Determination of Defect Depth and Size Using Virtual Heat Sources in Pulsed Infrared Thermography

Abstract

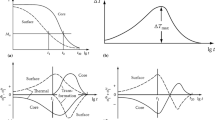
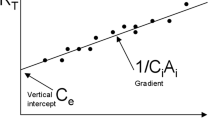
The determination of defect depth and size using Pulsed Infrared Thermography is a critical problem. The problem of defect depth estimation has been previously studied using 1D heat conduction models. Unfortunately, 1D heat conduction based models are generally inadequate in predicting heat flow around defects. In this study, a novel approach based on virtual heat sources is proposed to model heat flow around defects accounting for 2D axisymmetric heat conduction. The proposed approach is used to quantitatively determine the defect depth and size. The validity of the model is established using experiments performed on a stainless steel plate specimen with flat bottom holes at different depths.

Appendix

Solution of T ₁(r,t)

Let T ₁(r,t) = k ₁(r)k ₂(t). Then from equation (7),

$$ k_2\frac{\partial^2 k_1}{\partial r^2}+k_2\frac{1}{r}\frac{\partial k_1}{\partial r}=k_1\frac{1}{\alpha}\frac{\partial k_2}{\partial t} $$

(16)

The above equation can be written as,

$$ \frac{1}{k_1}\left[\frac{\partial^2 k_1}{\partial r^2}+\frac{1}{r}\frac{\partial k_1}{\partial r}\right]=\frac{1}{k_2}\frac{1}{\alpha}\frac{\partial k_2}{\partial t} $$

(17)

By letting both sides of the equation equal to the constant, − μ ², we have

$$ \frac{\partial^2 k_1}{\partial r^2}+\frac{1}{r}\frac{\partial k_1}{\partial r}+k_1\mu^2=0 $$

(18)

and

$$ \frac{\partial k_2}{\partial t}+k_2\alpha\mu^2=0 $$

(19)

Solving by standard methods yields, $k_2(t)=c_1 e^{-t\alpha\mu^2}$ and k ₁(r) = c ₂ J ₀(r μ) + c ₃ Y ₀(r μ). J ₀ is the Bessel’s function of the first kind with order 0 and Y ₀ is the Bessel’s function of the second kind with order 0. c ₁, c ₂, c ₃ and μ are constants that need to determined based on the prescribed initial and boundary conditions in the radial direction. It should be noted that Y ₀, the Bessel’s function of the second kind at order 0, is unbounded at r = 0. For boundedness of the solution, c ₃ = 0. No flux condition at r = R implies, $\frac{\partial T}{\partial r}=0$, and hence $\frac{\partial k_1}{\partial r}=0$. This implies − μc ₂ J ₁(r μ) = 0 as $\frac{\partial J_0(\mu r)}{\partial r}=-\mu J_1(\mu r)$. For non-trivial solutions, J ₁(μR) = 0. Using standard tables for roots of Bessel functions, the roots of the function are 3.83, 7.01, 10.17 .... Thus, T ₁(r,t) can be written as the product of k ₁(r) and k ₂(t).

$$ T_1(r,t)=c_1c_2 e^{-\mu^2 \alpha t} J_0(\mu r) $$

(20)

where $\mu \in \left\{ \frac{3.83}{R}, \frac{7.01}{R}, \frac{10.17}{R} \ldots \right\}$. By letting c ₁ c ₂ = A, a constant, and by using principle of superposition of the solutions, we have,

$$ T_1(r,t)= \sum \limits_{n=1}^{\infty} A_n e^{-\mu_n^2 \alpha t} J_0(\mu_n r) $$

(21)

A _n are a series of multiplicative constants that need to determined by using the initial conditions and properties of Bessel’s functions. At t = 0,

$$ \label{eq:bessel_ic} \displaystyle\sum \limits_{n=1}^{\infty} A_n J_0(\mu_n r)= \left\{ \begin{array}{l l} T_v & \quad 0\leq r \leq a \\ 0 & \quad a < r \leq R \\ \end{array} \right. $$

(22)

If μ _n and μ _m are roots of J ₀′(μR) = 0, it follows that [9],

$$ \label{eq:besselrelations} \begin{array}[b]{ll} \displaystyle \int_0^R r J_0(\mu_n r) J_0(\mu_m r) \,dr=0 & \forall m \neq n,\\[10pt] \displaystyle \int_0^R r \left\{J_0(\mu_n r)\right\}^2\,dr=\frac{R^2}{2}\left\{ J_0(\mu_n R)\right\}^2 & \\ [1ex] \end{array} $$

(23)

Multiplying both sides of equation (22) by r J ₀(μ _n r) dr and integrating between the limits 0 to R, we have,

$$ A_n \int_0^R \left\{J_0(\mu_n r)\right\}^2 r \,dr =\int_0^a T_v J_0(\mu_n r) r\,dr $$

(24)

On further simplifying using equation (23),

$$ A_n =\frac{2T_v }{R^2 J_0^2(\mu_n R)} \int_0^a J_0(\mu_n r) r\,dr $$

(25)

The radial part of the solution can be written as,

$$\begin{array}{lll}T_1(r,t)={}&\sum \limits_{n=1}^{\infty} \displaystyle \frac{2T_v}{R^2 J_0^2(\mu_n R)} \left(\int_0^a J_0(\mu_n r) r\,dr \right)\nonumber\\ &\times e^{-\mu_n^2 \alpha t} J_0(\mu_n r) \end{array}$$

(26)

where $\mu_n \in \left\{\frac{3.83}{R}, \frac{7.01}{R},\frac{10.17}{R} \ldots \right\}$. For solution completeness, a constant first term analogous to the Fourier series has to be added to the expansion [9]. The constant first term would be $\frac{2}{R^2}\int_0^R \zeta f(\zeta) \,d\zeta$ which reduces to $\frac{2}{R^2}\int_0^a \zeta T_v \,d\zeta$ for the given set of boundary and initial conditions. Thus, the constant first term equals $T_v\frac{a^2}{R^2}$. The radial part of the solution with the added constant term can be finally written as,

$$\begin{array}{lll}T_1(r,t)= T_v&\left[\frac{a^2}{R^2}+\sum \limits_{n=1}^{\infty} \displaystyle \frac{2}{R^2 J_0^2(\mu_n R)} \right.\nonumber\\ &\left.\times\left(\int_0^a J_0(\mu_n r) r\,dr \right)e^{-\mu_n^2 \alpha t} J_0(\mu_n r) \vphantom{\sum \limits_{n=1}^{\infty} \displaystyle \frac{2}{R^2 J_0^2(\mu_n R)}}\right] \end{array}$$

(27)

Solution of T ₂(z,t)

By letting T ₂(z,t) = k ₃(z)k ₄(t). Equation (9) simplifies to,

$$ k_4\frac{\partial^2k_3}{\partial z^2}=k_3\frac{1}{\alpha}\frac{\partial k_4}{\partial t} $$

(28)

By letting both sides of the equation equal to the constant, − λ ², and rearranging, we have,

$$\label{eq:temp1} \frac{\partial^2k_3}{\partial z^2}+k_3\lambda^2=0 $$

(29)

and

$$\label{eq:temp2} \frac{\partial k_4}{\partial t}+\alpha k_4 \lambda^2=0 $$

(30)

Solving equations (29) and (30) yields, k ₃(z) = c ₅cos(λz) + c ₆sin(λz) and $k_4(t)=c_4 e^{-t\alpha\lambda^2}$ and by using the boundary conditions in the z direction, we have $\frac{\partial k_3}{\partial z}=0$ at z = 0 and z = d.

$$ \displaystyle \frac{\partial k_3}{\partial z}=\lambda\left(-c_5 \sin(\lambda z) +c_6\cos(\lambda z) \right) $$

(31)

$\frac{\partial k_3}{\partial z}=0$ at z = 0 implies c ₆ = 0, and $\frac{\partial k_3}{\partial z}=0$ at z = d implies − c ₅ λsin(λd) = 0. For non-trivial solutions, sin(λd) = 0 which implies, $\lambda=\frac{m\pi}{d}$ for m = 1, 2, 3, .... Thus,

$$ k_3(z)=c_5\cos\left(\frac{m\pi z}{d} \right), \qquad m=1, 2, 3, \ldots $$

(32)

The resulting solution of the partial differential equation in the z direction is a product of k ₃(z) and k ₄(t),

$$ T_2(z,t)=B \cos\left(\frac{m\pi z}{d} \right)e^{-\left(\frac{m\pi}{d}\right)^2 \alpha t}, \qquad m=1, 2, 3, \ldots $$

(33)

where B = c ₄ c ₅ is an arbitrary multiplicative constant. By principle of superimposition, the above equation can be written as

$$ T_2(z,t)= \displaystyle\sum \limits_{m=0}^{\infty} B_m \cos\left(\frac{m\pi z}{d} \right)e^{-\left(\frac{m\pi}{d}\right)^2 \alpha t} $$

(34)

The series of constants, B _m will be determined using the initial condition in the z direction.

$$ \displaystyle\sum \limits_{n=0}^{\infty} B_n \cos\left(\frac{n\pi z}{d} \right) = \left\{ \begin{array}{l l} 1 & \quad z=0 \\[6pt] 0 & \quad 0<z \leq d \\ \end{array} \right. $$

(35)

By using,

$$ \int_0^d \cos\frac{k_1 \pi z}{d} \cos\frac{k_2 \pi z}{d}\,dz = \left\{ \begin{array}{l l} 0 & \quad k_1\neq k_2 \\[12pt] \dfrac{d}{2} & \quad k_1=k_2 \neq 0\\[12pt] d & \quad k_1=k_2=0\\ \end{array} \right. $$

(36)

Thus, B ₀ = 1 and B _m  = 2 for all m ≥ 1. The solution in the z direction can be written as,

$$ T_2(z,t)= 1+2\displaystyle\sum \limits_{m=1}^{\infty}\cos\left(\frac{m\pi z}{d}\right) e^{-\left(\frac{m\pi}{d}\right)^2 \alpha t}. $$

(37)