Phase Margin Determination in a Closed-Loop Configuration

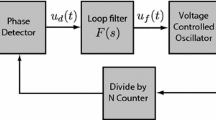
Abstract

A new and simple method is presented for determining the stability margin of a system with feedback. Contrary to classical and established methods the described procedure circumvents prior simulation of the loop gain. Instead, the phase margin is found by circuit simulation of the system in a closed-loop configuration. For this purpose, the phase slope of the closed-loop transfer function is evaluated after injecting some additional phase shift into the feedback loop. Moreover, the proposed method can also be applied to support the design of a system that requires a specified margin.

Appendix: On the Relation Between Group Delay and Phase Margin

This appendix contains the mathematical basics of the group delay for feedback circuits and its relation to the dominant system pole as well as the phase margin.

The frequency response H(jω) of a frequency dependent two-port network is a rational function and can be written as a product of first- and second-order terms:

(3)

The group delay τ _GR associated with each function H _i (jω) is defined as

(4)

The total phase shift has additive properties:

Therefore, the total group delay is

(5)

In (5) each group delay $\tau_{\mathrm{GR, i}}$ is connected with the corresponding H _i term in (3). The various terms H _i in (3) can also be expressed by the respective zero and pole locations (s _z,s _p) in the complex frequency plane s=σ+jω. In this case, the total frequency response H(jω) in (3) becomes the system function

(6)

The individual contribution of each pole and zero (real or conjugate-complex) to the total phase response can be found using simple geometric relations between the respective location of the singularity in the s-plane and the running frequency variable ω on the imaginary axis [4]:

(7)

where Re and Im denote the real and imaginary parts of the pole or zero location in the s-plane. Applying (4) and (5) the total group delay can now be written in terms of s-plane parameters:

(8)

Note that in (8) poles and zeros may be located in the left-half of the s-plane (LHP) as well as the right-half of the s-plane (RHP). At this stage, this concept ignores the fact that in causal and stable systems RHP poles must not appear. Evaluating (8) it can be concluded that

• LHP poles and RHP zeros increase the group delay;

• RHP poles and LHP zeros decrease the group delay;

• That particular singularity (pole or zero) with the shortest distance to the imaginary axis—expressed by the denominators in (8)—determines the maximum contribution to the total group delay.

If a transfer function is represented by a conjugate-complex pole pair $s_{\mathrm{p1,2}}$ only, the group delay is readily found by using (4) directly, rather than by applying (8) for both poles separately. For example, a second-order lowpass frequency response in terms of the data describing the pole

has the group delay

where ω _P (pole frequency) and ζ (damping ratio) are defined as

(9)

It can be shown [7] that for |ζ∣<0.866 the group delay exhibits peaking at

with a peak value of

(10)

Thus, the peak value of the group delay is large for a pole pair with a small damping ratio ζ and vice versa. Note that in accordance with (8), (9), and (10) the group delay calculation results in a negative value if the pole pair is located in the RHP with ζ<0.

On the other hand, it is well known that the phase margin of a feedback system decreases as the dominant pole pair moves closer to the imaginary axis due to the smaller amount of damping. Therefore, it is promising to investigate the relation between the phase margin φ _PM, the damping ratio ζ, and the group delay $\tau_{\mathrm{GR,}}$, which can be expressed by the slope of the phase function.

The relationship between the damping ratio and the phase margin for a second-order system is given in [1]:

It can be shown [1] that for φ _PM<65^∘ and ζ<0.707(1/2ζ=Q _P>0.707) this expression can be approximated with good accuracy by

(11)

Introducing (11) into (10) yields

(12)

where φ _PM is measured in degrees.

This relationship combines the phase margin φ _PM with the group delay peak value, which can be easily found by simulation. But a certain drawback of this simple method for rating the phase margin of a second-order system is the need to know the respective pole frequency ω _P. The approximation ω _peak≈ω _P may be used for underdamped systems only with a large group delay peak at ω=ω _peak (for example: ζ=0.1→ω _peak=0.995ω _P).

However, a graphical representation of (12) reveals a quasi-linear relationship between the inverse peak delay $\tau_{\mathrm{GR,peak}}$ and φ _PM. This property can be advantageously exploited for interpolation purposes without needing to know the pole frequency. With the aim of producing this graph, the phase margin of the circuit to be investigated is varied by introducing some additional negative phase shift φ _Z=−|φ _Z| into the feedback loop.

This results in an artificially reduced phase margin $\varphi_{\mathrm{PM,Z}}$, which becomes negative for |φ _Z|>φ _PM:

After replacing φ _PM by $\varphi_{\mathrm{PM,Z}}$ in (12) the function $(\mbox{1/}\tau_{\mathrm{GR,peak}})=f(|\varphi_{\mathrm{Z}}|)$ is shown in Fig. 4 for the normalized pole frequency ω _P=1 rad/s and φ _PM=45^∘. As can be seen, the curve exhibits good linearity properties and crosses the horizontal axis at |φ _Z|=φ _PM=45^∘. This is because the condition $\varphi_{\mathrm{PM,Z}} =0$ leads to a group delay peak approaching infinity with 1/$\tau_{\mathrm{GR, peak}} =0$.

Fig. 4

Inverse group delay peak value as a function of injected phase shift φ _Z. (a) Function according to (12), (b) Linear approximation (13). Parameters: ω _P=1 rad/s, φ _PM=45^∘

For practical applications, with an unknown phase margin φ _PM the quasi-linear properties of the function in Fig. 4 enable a simple interpolation between two user-defined φ _Z values and the respective group delay peak values. For this purpose, (12) is approximated by the linear expression

(13)

For comparison purposes, this approximation is also shown in Fig. 4.

Introducing two distinct points ($\varphi_{\mathrm{Z}}, \tau_{\mathrm{GR,peak}}$) into (13) and solving for φ _PM yields

(14)

Note that the range between the two selected φ _Z values must contain the unknown margin φ _PM.

As a consequence (see Fig. 4) it follows that

For the sake of uniqueness it is, therefore, convenient to introduce magnitudes into (14):

(15)

In summary, there are two possible approaches for determining the phase margin of a second-order system with feedback based on closed-loop ac simulations:

• 1. Evaluation of the interpolation algorithm (15) based on two appropriate φ _Z values below and above the expected phase margin φ _PM, respectively;

• 2. Graphical representation of ($\tau_{\mathrm{GR, peak}})^{-1} =f(|\varphi_{\mathrm{Z}}|$) based on at least two appropriate φ _Z values (following (13) and Fig. 4) and identification of the zero crossing at |φ _Z|=φ _PM. This graph is easily produced by exploiting the post-processor capabilities of the simulation program (Performance Analysis, PA). For this purpose, a suitable goal function $\mathrm{GF} =f(1/\tau_{\mathrm{GR, peak}})$ is provided in Sect. 3.2.

Remark

The mathematical expressions that contain the relation between group delay and phase margin, (13) and (15), have been derived for a second-order system. However, in most cases the response of a higher-order systems—with additional poles and/or zeros—is dominated by a single pole pair. Therefore, the above considerations apply also to the majority of other active circuits and feedback loops of practical interest. This is true, in particular, because the injected extra phase values shift the dominant pole pair even closer to the imaginary s-plane axis. For this reason, it can be beneficial to step through more than only two φ _Z values during the ac analysis. In this case, the second approach for evaluating the group delay extrema (Performance Analysis, goal function GF) is to be applied.