Skip to main content
SpringerLink
Log in

The Dynamics of Flat Surface Internal Geophysical Waves with Currents

  • Published:
Journal of Mathematical Fluid Mechanics Aims and scope Submit manuscript

Abstract

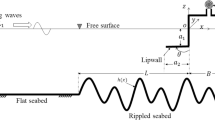
A two-dimensional water wave system is examined consisting of two discrete incompressible fluid domains separated by a free common interface. In a geophysical context this is a model of an internal wave, formed at a pycnocline or thermocline in the ocean. The system is considered as being bounded at the bottom and top by a flatbed and wave-free surface respectively. A current profile with depth-dependent currents in each domain is considered. The Hamiltonian of the system is determined and expressed in terms of canonical wave-related variables. Limiting behaviour is examined and compared to that of other known models. The linearised equations as well as long-wave approximations are presented.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Benjamin T.B., Bridges T.J.: Reappraisal of the Kelvin-Helmholtz problem. Part 1. Hamiltonian structure. J. Fluid Mech. 333, 301–325 (1997)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  2. Benjamin T.B., Bridges T.J.: Reappraisal of the Kelvin-Helmholtz problem. Part 2. Interaction of the Kelvin-Helmholtz, superharmonic and Benjamin-Feir instabilities. J. Fluid Mech. 333, 327–373 (1997)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  3. Benjamin T.B., Olver P.J.: Hamiltonian structure, symmetries and conservation laws for water waves. J. Fluid Mech. 125, 137–185 (1982)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  4. Compelli A.: Hamiltonian formulation of 2 bounded immiscible media with constant non-zero vorticities and a common interface. Wave Motion 54, 115–124 (2015). doi:10.1016/j.wavemoti.2014.11.015

    Article  MathSciNet  Google Scholar 

  5. Compelli A.: Hamiltonian approach to the modeling of internal geophysical waves with vorticity. Monatsh. Math. 179(4), 509–521 (2016). doi:10.1007/s00605-014-0724-1

    Article  MathSciNet  MATH  Google Scholar 

  6. Compelli, A., Ivanov, R.I.: On the dynamics of internal waves interacting with the Equatorial Undercurrent. J. Nonlinear Math. Phys. 22, 531–539 (2015). doi:10.1080/14029251.2015.1113052. arXiv:1510.04096 [math-ph]

  7. Compelli, A., Ivanov, R.I.: Hamiltonian approach to internal wave-current interactions in a two-media fluid with a rigid lid. Pliska Stud. Math. Bulgar. 25, 7–18 (2015). arXiv:1607.01358 [physics.flu-dyn]

  8. Constantin A.: On the deep water wave motion. J. Phys. A 34, 1405–1417 (2001). doi:10.1088/0305-4470/34/7/313

    Article  ADS  MathSciNet  MATH  Google Scholar 

  9. Constantin, A.: Nonlinear water waves with applications to wave-current interactions and tsunamis. In: CBMS-NSF Regional Conference Series in Applied Mathematics, vol. 81. SIAM, Philadelphia (2011)

  10. Constantin A., Escher J.: Symmetry of steady periodic surface water waves with vorticity. J. Fluid Mech. 498, 171–181 (2004). doi:10.1017/S0022112003006773

    Article  ADS  MathSciNet  MATH  Google Scholar 

  11. Constantin A., Escher J.: Analyticity of periodic traveling free surface water waves with vorticity. Ann. Math. 173, 559–568 (2011). doi:10.4007/annals.2011.173.1.12

    Article  MathSciNet  MATH  Google Scholar 

  12. Constantin A., Germain P.: Instability of some equatorially trapped waves. J. Geophys. Res. Oceans 118, 2802–2810 (2013). doi:10.1002/jgrc.20219

    Article  ADS  Google Scholar 

  13. Constantin, A., Ivanov, R.I.: On an integrable two-component Camassa-Holm shallow water system. Phys. Lett. A. 372, 7129–7132 (2008). doi:10.1016/j.physleta.2008.10.050. arXiv:0806.0868 [nlin.SI]

  14. Constantin A., Ivanov R.I.: A Hamiltonian approach to wave-current interactions in two-layer fluids. Phys. Fluids 27, 086603 (2015). doi:10.1063/1.4929457

    Article  ADS  MATH  Google Scholar 

  15. Constantin, A., Ivanov, R.I., Martin, C.I.: Hamiltonian formulation for wave-current interactions in stratified rotational flows. Arch. Ration. Mech. Anal. 221(3), 1417–1447 (2016). doi:10.1007/s00205-016-0990-2

  16. Constantin, A., Ivanov, R.I., Prodanov, E.M.: Nearly-Hamiltonian structure for water waves with constant vorticity. J. Math. Fluid Mech. 9, 1–14 (2007). doi:10.1007/s00021-006-0230-x. arXiv:math-ph/0610014

  17. Constantin A., Johnson R.S.: The dynamics of waves interacting with the equatorial undercurrent. Geophys. Astrophys. Fluid Dyn. 109, 311–358 (2015). doi:10.1080/03091929.2015.1066785

    Article  ADS  MathSciNet  Google Scholar 

  18. Constantin A., Sattinger D., Strauss W.: Variational formulations for steady water waves with vorticity. J. Fluid Mech. 548, 151–163 (2006). doi:10.1017/S0022112005007469

    Article  ADS  MathSciNet  Google Scholar 

  19. Constantin A., Strauss W.: Exact steady periodic water waves with vorticity. Commun. Pure Appl. Math. 57, 481–527 (2004). doi:10.1002/cpa.3046

    Article  MathSciNet  MATH  Google Scholar 

  20. Cotter, C.J., Holm, D.D., Percival, J.R.: The square root depth wave equations. Proc. R. Soc. A. 466, 3621–3633 (2010). doi:10.1098/rspa.2010.0124

  21. Craig W., Guyenne P., Kalisch H.: Hamiltonian long wave expansions for free surfaces and interfaces. Commun. Pure Appl. Math. 24, 1587–1641 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  22. Craig W., Guyenne P., Sulem C.: Coupling between internal and surface waves. Nat. Hazards 57, 617–642 (2011). doi:10.1007/s11069-010-9535-4

    Article  Google Scholar 

  23. Escher J., Henry D., Kolev B., Lyons T.: Two-component equations modelling water waves with constant vorticity. Annali di Matematica Pura ed Applicata 195, 249–271 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  24. Fan L., Gao H., Liu Y.: On the rotation-two-component Camassa–Holm system modelling the equatorial water waves. Adv. Math. 291, 59–89 (2016). doi:10.1016/j.aim.2015.11.049

    Article  MathSciNet  MATH  Google Scholar 

  25. Genoud F., Henry D.: Instability of equatorial water waves with an underlying current. J. Math. Fluid Mech. 16, 661–667 (2014). doi:10.1007/s00021-014-0175-4

    Article  MathSciNet  MATH  Google Scholar 

  26. Henry D., Hsu H.-C.: Instability of equatorial water waves in the f-plane. Discrete Contin. Dyn. Syst. 35, 906–916 (2015). doi:10.3934/dcds.2015.35.909

    MathSciNet  MATH  Google Scholar 

  27. Holm, D.D., Ivanov, R.I.: Two-component CH system: Inverse scattering, peakons and geometry. Inverse Probl. 27, 045013 (2011). doi:10.1088/0266-5611/27/4/045013. arXiv:1009.5374v1 [nlin.SI]

  28. Ivanov, R.I.: Two component integrable systems modelling shallow water waves: the constant vorticity case. Wave Motion 46, 389–396 (2009). doi:10.1016/j.wavemoti.2009.06.012. arXiv:0906.0780

  29. Ivanov, R.I. and Lyons, T.: Integrable models for shallow water with energy dependent spectral problems. Journal of Nonlinear Mathematical Physics 19, Suppl. 1, 1240008 (2012) (17 pages). doi:10.1142/S1402925112400086. arXiv:1211.5567 [nlin.SI]

  30. Johnson R.S.: Camassa-Holm Korteweg-de Vries and related models for water waves. J. Fluid. Mech. 457, 63–82 (2002). doi:10.1017/S0022112001007224

    ADS  MathSciNet  MATH  Google Scholar 

  31. Johnson R.S.: The Camassa-Holm equation for water waves moving over a shear flow. Fluid Dyn. Res. 33, 97–111 (2003). doi:10.1016/S0169-5983(03)00036-4

    Article  ADS  MathSciNet  MATH  Google Scholar 

  32. Johnson, R.S.: The classical problem of water waves: a reservoir of integrable and nearly-integrable equations. J. Nonlinear Math. Phys. 10(suppl. 1), 72–92 (2003)

  33. Jonsson, I.G.: Wave-current interactions. In: The sea, pp, 65–120. Willey, New York (1990)

  34. Kaup D.J.: A higher-order water-wave equation and the method for solving it. Progr. Theor. Phys. 54, 396–408 (1975)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  35. Korteweg, D., de Vries, G.: On the change of form of long waves advancing in a rectangular canal, and on a new type of long stationary waves. Philos. Mag. 39, 422–443 (1895) [reprint: Philos. Mag. 91, 1007–1028 (2011). doi:10.1080/14786435.2010.547337]

  36. Lamb K.: Internal wave breaking and dissipation mechanisms on the continental slope/shelf. Annu. Rev. Fluid Mech. 46, 231–254 (2014)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  37. Martin C.I.: Dispersion relations for gravity water flows with two rotational layers. Eur. J. Mech. B/Fluids 50, 9–18 (2015). doi:10.1016/j.euromechflu.2014.10.005

    Article  ADS  MathSciNet  Google Scholar 

  38. Milder D.M.: A note regarding On Hamilton‘s principle for water waves. J. Fluid Mech. 83, 159–161 (1977)

    Article  ADS  MATH  Google Scholar 

  39. Miles J.W.: Hamiltonian formulations for surface waves. Appl. Sci. Res. 37, 103–110 (1981)

    Article  MathSciNet  MATH  Google Scholar 

  40. Miles J.W.: On Hamilton’s principle for water waves. J. Fluid Mech. 83, 153–158 (1977)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  41. Peregrine D.H.: Interaction of water waves and currents. Adv. Appl. Mech. 16, 9–117 (1976)

    Article  MATH  Google Scholar 

  42. Sattinger D.H., Szmigielski J.: A Riemann-Hilbert problem for an energy dependent Schrödinger operator. Inverse Probl. 12, 1003–1025 (1996)

    Article  ADS  MATH  Google Scholar 

  43. Teles da Silva A.F., Peregrine D.H.: Steep, steady surface waves on water of finite depth with constant vorticity. J. Fluid Mech. 195, 281–302 (1988)

    Article  ADS  MathSciNet  Google Scholar 

  44. Thomas R., Kharif C., Manna M.: A nonlinear Schrödinger equation for water waves on finite depth with constant vorticity. Phys. Fluids. 24, 127102 (2012). doi:10.1063/1.4768530

    Article  ADS  Google Scholar 

  45. Thomas, G.P., Klopman, G.: Wave-current interactions in the near-shore region. In: Gravity waves in water of finite depth, edited by Hunt, J.N. (computational Mechanics, Southampton), pp. 215–319 (1997)

  46. Wahlén E.: A Hamiltonian formulation of water waves with constant vorticity. Lett. Math. Phys. 79, 303–315 (2007). doi:10.1007/s11005-007-0143-5

    Article  ADS  MathSciNet  MATH  Google Scholar 

  47. Wahlén E.: Hamiltonian Long Wave Approximations of Water Waves with Constant Vorticity. Phys. Lett. A. 372, 2597–2602 (2008). doi:10.1016/j.physleta.2007.12.018

    Article  ADS  MathSciNet  MATH  Google Scholar 

  48. Zakharov, V.E.: Stability of periodic waves of finite amplitude on the surface of a deep fluid. Zh. Prikl. Mekh. Tekh. Fiz. 9, 86–94 (1968, in Russian) [J. Appl. Mech. Tech. Phys. 9, 190–194 (1968, English translation)]

Download references

Author information

Authors and Affiliations

  1. School of Mathematical Sciences, Dublin Institute of Technology, Kevin Street, Dublin 8, Ireland

    Alan Compelli & Rossen I. Ivanov

Authors
  1. Alan Compelli
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Rossen I. Ivanov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Rossen I. Ivanov.

Additional information

Communicated by A. Constantin

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Compelli, A., Ivanov, R.I. The Dynamics of Flat Surface Internal Geophysical Waves with Currents. J. Math. Fluid Mech. 19, 329–344 (2017). https://doi.org/10.1007/s00021-016-0283-4

Download citation

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00021-016-0283-4

Mathematics Subject Classification

Keywords

Search

Navigation