Nonlinear model-based parameter estimation and stability analysis of an aero-pendulum subject to digital delayed control

Abstract

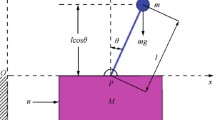
Digitization and time delay are known to modify the stability properties of feedback controlled systems. Although their effects have been widely investigated and they occur in most of the systems equipped with digital processors, they are usually neglected in industrial approaches, by virtue of the high sampling frequencies of modern processors. However, these approaches are not conservative with respect to stability. In this work, we investigate, first analytically, then numerically and experimentally, the stability properties of the so-called Aeropendulum. The Aeropendulum is a mechanical pendulum with a propeller at its free end. A motor, activating the propeller, allows an active control of the pendulum in a feedback loop. The system exhibits most of the difficulties encountered in more involved industrial robotic systems. The estimation of the parameter values is performed through a model-based estimation, which allows to successfully define damping coefficients of order zero, one and two. Stability charts obtained with different controllers are compared, showing the larger stability region obtainable with the act-and-wait controller under proper conditions, as predicted by the theory.

Appendix: Transformation of the system into a map

In order to transform the continuous time system in Eq. (16) into the map in Eq. (17), Eq. (16) must be solved in closed form within a single time step. The PWM signal is constant within each sampling interval. Thus, once PWM is considered to be constant, ${\varOmega }(t)$, with $t\in \left[ t_j,t_{j+1}\right)$ can be easily found, as the result of a first order linear differential equation. Substituting ${\varOmega }(t)$ into the first equation of Eq. (16) and solving the resultant linear differential equation, $\phi (t)$, with $t\in \left[ t_j,t_{j+1}\right)$ can be found. Considering the evolution of these functions from $t_j$ to $t_{j+1}$, the map in Eq. (17) can be defined. In the following, the explicit formulation of the coefficients of $\mathbf {A}$ is given.

$$\begin{aligned}&a_{11}=e^{-\tau \omega _n\left( \hat{\delta } +\sqrt{\hat{\delta }^2-c_{\varphi _0}}\right) }\left( \frac{\hat{\delta }\left( e^{2\tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}-1\right) }{2\sqrt{\hat{\delta }^2-c_{\varphi _0}}}+ \frac{1+e^{2\tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}}{2}\right) \\&a_{12}=\frac{e^{-\hat{\delta }\tau \omega _n -\tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}\left( e^{2\tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}-1\right) }{2\tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}\\&a_{13}=-\frac{1+\lambda \omega _n\left( \sqrt{\hat{\delta }^2-c_{\varphi _0}}-\hat{\delta }\right) + e^{2\tau \omega _n \sqrt{\hat{\delta }^2-c_{\varphi _0}}} \left( \lambda \omega _n\left( \sqrt{\hat{\delta }^2-c_{\varphi _0}}+\hat{\delta }\right) -1\right) }{\lambda ^{-1}e^{\hat{\delta }\tau \omega _n+ \tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}} \left( 2\omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}\left( 1 -2\hat{\delta }\lambda \omega _n+\lambda ^2\omega _n^2 c_{\varphi _0}\right) \right) }+\frac{\lambda ^2}{e^{\tau /\lambda } \left( 1 -2\hat{\delta }\lambda \omega _n+\lambda ^2\omega _n^2 c_{\varphi _0}\right) }\\&a_{14}= \sec \left( \varphi _0\right) \Bigg (\frac{\left( 2\hat{\delta }\lambda \omega _n-1\right) \hat{\delta }\left( e^{2\tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}-1\right) }{e^{\hat{\delta }\tau \omega _n+ \tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}\left( 2\omega _n^2\sqrt{\hat{\delta }^2 -c_{\varphi _0}} \left( 1-2\hat{\delta }\lambda \omega _n+ \lambda ^2\omega _n^2c_{\varphi _0}\right) \right) }\\&\quad +\frac{\left( 2\hat{\delta }\lambda \omega _n-1\right) \left( 1- 2e^{\hat{\delta }\tau \omega _n+\tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}} +e^{2\tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}\right) }{e^{\hat{\delta }\tau \omega _n+ \tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}2\omega _n^2 \left( 1-2\hat{\delta }\lambda \omega _n+ \lambda ^2\omega _n^2c_{\varphi _0}\right) }\\&\quad +\frac{\lambda ^2 c_{\varphi _0}}{1-2\hat{\delta }\lambda \omega _n +\lambda ^2\omega _n^2c_{\varphi _0}} +\frac{\lambda c_{\varphi _0}\left( e^{\tau /\lambda }-e^{\tau /\lambda +2\tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}} - 2e^{\hat{\delta }\tau \omega _n+ \tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}\lambda \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}\right) }{e^{\tau /\lambda + \hat{\delta }\tau \omega _n+ \tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}} 2\omega _n\sqrt{\hat{\delta }^2 -c_{\varphi _0}} \left( 1-2\hat{\delta }\lambda \omega _n+ \lambda ^2\omega _n^2c_{\varphi _0}\right) }\Bigg ) \end{aligned}$$

$$\begin{aligned} a_{21}= & {} \frac{e^{-\hat{\delta }\tau \omega _n -\tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}\left( 1-e^{2\tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}\right) \tau \omega _n c_{\varphi _0}\sqrt{\hat{\delta }^2-c_{\varphi _0}}}{2\left( \hat{\delta }^2-c_{\varphi _0}\right) }\\ a_{22}= & {} \frac{e^{-\hat{\delta }\tau \omega _n -\tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}}{2}\left( \frac{\hat{\delta }\left( 1-e^{2\tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}\right) }{\sqrt{\hat{\delta }^2 -c_{\varphi _0}}} +1+e^{2\tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}\right) \\ a_{23}= & {} \frac{\lambda \hat{\delta }^2\left( e^{\tau /\lambda }- 2e^{\hat{\delta }\tau \omega _n +\tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}+e^{\tau /\lambda +2\tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}\right) \tau }{e^{\tau /\lambda +\hat{\delta }\tau \omega _n +\tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}2\left( \hat{\delta }^2 -c_{\varphi _0}\right) \left( 1-2\hat{\delta }\lambda \omega _n +\lambda ^2\omega _n^2c_{\varphi _0}\right) }\nonumber \\&-\frac{\lambda \tau \left( \left( \hat{\delta } e^{2\tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}-\hat{\delta }+\lambda \omega _nc_{\varphi _0} \right) \sqrt{\hat{\delta }^2-c_{\varphi _0}}+c_{\varphi _0}\right) }{e^{\hat{\delta }\tau \omega _n +\tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}2\left( \hat{\delta }^2 -c_{\varphi _0}\right) \left( 1-2\hat{\delta }\lambda \omega _n +\lambda ^2\omega _n^2c_{\varphi _0}\right) }\nonumber \\&+\frac{\lambda \tau c_{\varphi _0}\left( 2e^{\hat{\delta }\tau \omega _n} +e^{\tau /\lambda + \tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}} }\left( \lambda \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}-\tau \right) \right) }{e^{\tau /\lambda +\hat{\delta }\tau \omega _n}2\left( \hat{\delta }^2 -c_{\varphi _0}\right) \left( 1-2\hat{\delta }\lambda \omega _n+ \lambda ^2\omega _n^2c_{\varphi _0}\right) }\\ a_{24}= & {} -\frac{\left( e^{\tau /\lambda }- 2e^{\hat{\delta }\tau \omega _n+\tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}+ e^{\tau /\lambda +2\tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}\right) \lambda \tau }{e^{\tau /\lambda +\hat{\delta }\tau \omega _n + \tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}} 2\left( 1 -2\hat{\delta }\lambda \omega _n+\lambda ^2 \omega _n^2c_{\varphi _0}\right) }\nonumber \\&+\frac{\left( e^{2\tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}}-1\right) \tau \left( 1-\hat{\delta }\lambda \omega _n\right) }{e^{\hat{\delta }\tau \omega _n + \tau \omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}} 2\omega _n\sqrt{\hat{\delta }^2-c_{\varphi _0}}\left( 1 -2\hat{\delta }\lambda \omega _n+\lambda ^2 \omega _n^2c_{\varphi _0}\right) }\\ a_{33}= & {} e^{-\tau /\lambda }\\ a_{34}= & {} 1-e^{-\tau /\lambda } \end{aligned}$$