Skip to main content
SpringerLink
Log in

Complex Backward–Forward Derivative Operator in Non-local-In-Time Lagrangians Mechanics

  • Published:
Qualitative Theory of Dynamical Systems Aims and scope Submit manuscript

Abstract

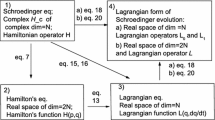
In this paper we introduce non-local-in-time complexified Lagrangians characterized by an expanded complex backward–forward derivative operator which generalize the classical complex derivative operator. We developed the Euler–Lagrange equations and solved them for some special case. We discuss their implications in Newtonian mechanics where a number of applications were illustrated.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Alber, S., Marsden, J.E.: Semiclassical monodromy and the spherical pendulum as a complex Hamiltonian system. Fields Inst. Commun. 8, 1–18 (1996)

    MathSciNet  MATH  Google Scholar 

  2. Ben Adda, F., Cresson, J.: Quantum derivatives and the Schrödinger equation. Chaos Solitons Fractals 19, 1323–1334 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  3. Bender, C.M., Holm, D.D., Hook, D.W.: Complexified dynamical systems. J. Phys. A 40, F793–F804 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  4. Cresson, J.: Fractional embedding of differential operators and Lagrangian system. J. Math. Phys. 48(3), 033504–044534 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  5. Dryl, M., Torres, D.F.M.: The delta–nabla calculus of variations for composition functionals on time scales. Int. J. Differ. Equ. 8, 27–47 (2013)

    MathSciNet  Google Scholar 

  6. El-Nabulsi, R.A.: Non-standard non-local-in-time Lagrangians in classical mechanics. Qual. Theor. Dyn. Syst. 13, 149–160 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  7. El-Nabulsi, R.A., Torres, D.F.M.: Fractional actionlike variational problems. J. Math. Phys. 49(5), 053521–053527 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  8. El-Nabulsi, R.A., Torres, D.F.M.: Necessary optimality conditions for fractional action-like integrals of variational calculus with Riemann-Liouville derivatives of order (\(\alpha \), \(\beta )\). Math. Methods Appl. Sci. 30(15), 1931–1939 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  9. El-Nabulsi, R.A.: Lagrangian and Hamiltonian dynamics with imaginary time. J. Appl. Anal. 18, 283–295 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  10. Feynman, R.P.: Space-time approach to relativistic quantum mechanics. Rev. Mod. Phys. 20, 367–387 (1948)

    Article  MathSciNet  Google Scholar 

  11. Feynman, R.P., Hibbs, A.: Quantum Mechanics and Path Integrals. MacGraw-Hill, New York (1965)

    MATH  Google Scholar 

  12. Kaushal, R.S.: Classical and quantum mechanics of complex Hamiltonian systems: an extended complex phase space approach. PRAMANA J. Phys. 73(2), 287–297 (2009)

    Article  Google Scholar 

  13. Li, Z.-Y., Fu, J.-L., Chen, L.-Q.: Euler–Lagrange equation from nonlocal-in-time kinetic energy of nonconservative system. Phys. Lett. A 374, 106–109 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  14. Malinowska, A.B., Torres, D.F.M.: Springer Briefs in Electrical and Computer Engineering: Control, Automation and Robotics. Quantum variational calculus. Springer, New York (2014)

    Google Scholar 

  15. Martins, N., Torres, D.F.M.: Higher-order infinite horizon variational problems in discrete quantum calculus. Comput. Math. Appl. 64, 2166–2175 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  16. Martins, N., Torres, D.F.M.: Calculus of variations on time scales with nabla derivatives. Nonlinear Anal. 71, e763–e773 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  17. Mohanasubha, R., Sheeba, J.H., Chandrasekar, V.K., Senthilvelan, M., Lakshmanan, M.: A nonlocal connection between certain linear and nonlinear ordinary differential equations—Part II: Complex nonlinear oscillators. Appl. Math. Comput. 224, 593–602 (2013)

    MathSciNet  MATH  Google Scholar 

  18. Nelson, E.: Derivation of the Schrödinger equation from Newtonian mechanics. Phys. Rev. 150, 1079–1085 (1966)

    Article  Google Scholar 

  19. Nottale, L.: Fractal Space-Time and Microphysics: Towards a Theory of Scale Relativity. World Scientific, New York (1993)

    Book  MATH  Google Scholar 

  20. Sbitnev, V.I.: Bohmian trajectories and the path integral paradigm. Complexified Lagrangian mechanics. Int. J. Bifurn. Chaos 19, 2335–2346 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  21. Suykens, J.A.K.: Extending Newton’s law from nonlocal-in-time kinetic energy. Phys. Lett. A 373, 1201–1211 (2009)

    Article  MATH  Google Scholar 

  22. Tritton, D.J.: Physical Fluid Dynamics, 2nd edn. Clarendon Press, Oxford (1988)

    MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. College of Mathematics and Information Science, Neijiang Normal University, Neijiang, 641112, Sichuan, China

    Rami Ahmad El-Nabulsi

Authors
  1. Rami Ahmad El-Nabulsi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Rami Ahmad El-Nabulsi.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

El-Nabulsi, R.A. Complex Backward–Forward Derivative Operator in Non-local-In-Time Lagrangians Mechanics. Qual. Theory Dyn. Syst. 16, 223–234 (2017). https://doi.org/10.1007/s12346-016-0187-y

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12346-016-0187-y

Keywords

Mathematics Subject Classification

Search

Navigation