Stress-Sensitive Permeability: Application to Fault Integrity During Gas Production

Abstract

The objective is to propose a simple theoretical approach and the associated numerical algorithm to capture the permeability evolution within a fractured region in response to a stress perturbation. The stress range of interest is typical of a reversible deformation such that the fractures have varying apertures but constant lengths and densities. It is the permeability evolution from a negligible value characteristic of flows on geological times to values more relevant for gas production which is important for the structural integrity of the fractured region. A simple 1D application related to the sealing capacity of a fault bounding a producing gas reservoir is proposed to illustrate the theory. The stress change on the two sides of the faults is obtained with a 2D finite-element simulation based on the theory of poro-elasticity and considering the fault as a material discontinuity. The 1D flow simulation is done in a second step, and the flux is assumed to occur through the fault thickness from the non-depleted (minus side) to the depleted (plus side) regions. It is shown how the depletion results in the fractures opening in the fault damaged zone close to the minus side and the fracture closure next to the plus side. This evolution could be non-monotonic in time because of the development and the thinning of a boundary layer in the fluid pressure at the plus side. The simulations end once a Coulomb criterion is reached, typically at the minus side of the fault. The presence of a low-permeability core in the fault center does not change these conclusions although a positive effective normal stress is detected in the damaged zone on the minus side of the core prior to the Coulomb criterion activation.

Appendices

Appendix 1: 1D Numerical Algorithm

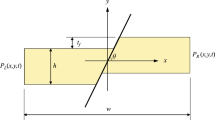
The objective is to present the weak form of the coupled problem at the basis of the finite-element method. The starting point is Eq. (8) which in this 1D setting and in the $(\xi _1,\xi _2)$ basis introduced in Fig. 3c reads

$$\begin{aligned}&\displaystyle \int _0^{2w} \varphi \rho _g \phi C_{eq} \frac{\partial p}{\partial t} - \varphi \Bigl ( K ( p_{,1} -\rho _g g \cos \gamma ) \Bigr )_{,1}\, d\xi _1 \nonumber \\&\quad = - \int _0^{2w} \varphi \rho _g R_\theta \frac{\partial \theta }{\partial t} \, d\xi _1\quad \mathrm{with} \quad K = \frac{ \rho _g k_{rg} K_{11}}{\mu _g} \, , \end{aligned}$$

(25)

once multiplied by the virtual scalar field $\varphi$ and summed over the fault width. The comma marks the partial differential with respect to the first coordinate $\xi _1$. Integration by parts of the second term in the left-hand side of (25) and application of the divergence theorem leads to

$$\begin{aligned} \displaystyle \int _0^{2w} \varphi \rho _g \phi C_{eq} \frac{\partial p}{\partial t} + \varphi _{,1} K p_{,1} \, d\xi _1 = \int _0^{2w} -\varphi \rho _g R_\theta \frac{\partial \theta }{\partial t} + \varphi _{,1} K \rho _g g \cos \gamma \, d\xi _1 \, , \end{aligned}$$

(26)

having selected virtual fields equal to zero at the two ends of the fault where the pressure is prescribed.

Let us now discretize the region [0; 2w] in a set of $\mathtt numel$ elements having each two nodes and consider the following interpolation of the pressure and virtual field over each element

$$\begin{aligned} \varphi (\zeta ) = \sum _{a=1}^2 N_a(\zeta ) \varphi _a, \quad p(\zeta ) = \sum _{a=1}^2 N_a(\zeta ) p_a, \end{aligned}$$

(27)

in which $p_a$, $\varphi _a$ and $N_a$ are the nodal values of the pressure and of the virtual field and the classical linear shape functions of the element of length 2 in the computational domain and of length $L_e$ in the physical space, respectively. The coordinate $\zeta$ introduced in (27) is in the computational domain and ranges from −1 to +1. Spatial interpolation (27) is now introduced in the governing equation, and the local contribution of an element is

$$\begin{aligned}&\displaystyle \int _{-1}^{+1} N_a \rho _g \phi C_{eq} N_b J d\zeta \, \frac{\partial p_b}{\partial t} + \int _{-1}^{+1} N_{a,\zeta } K N_{b,\zeta } J^{-1} \, d\zeta \, p_{b} \nonumber \\&\quad = \int _{-1}^{+1} - N_a \rho _g R_\theta \frac{\partial \theta }{\partial t} J + N_{a,\zeta } K \rho _g g \cos \gamma \, d\zeta , \end{aligned}$$

(28)

all quadratures being computed in the computational domain and J standing for the Jacobian of the transformation to the physical domain ($J = L_e/2$). This equation is now presented in a synthetic manner with the help of a matrix notation

$$\begin{aligned} \displaystyle [ C ] \, \left\{ \frac{\partial p}{\partial t} \right\} + [ K] \, \{ p \} = \{ F\}\, , \end{aligned}$$

(29)

with the introduction of the local storativity, permeability and of a source term due to the coupling with the elastic deformation and the action of gravity:

$$\begin{aligned} \displaystyle [ C ]&= \rho _g \phi C_{eq} \frac{J}{3} \left[ \begin{array}{cc} 2 &{} 1 \\ 1 &{} 2 \end{array} \right] ,\nonumber \\ [ K ]&= \frac{K}{2J} \left[ \begin{array}{rr} 1 &{} -1 \\ -1 &{} 1 \end{array}\right] \nonumber , \\ \displaystyle \{F\}&= -\rho _g R_\theta \frac{\partial \theta }{\partial t} J \left\{ \begin{array}{c} 1 \\ 1 \end{array}\right\} + K \rho _g g \cos \gamma \left\{ \begin{array}{r} -1 \\ 1 \end{array}\right\} . \end{aligned}$$

(30)

The parameters pre-multiplying the matrices and vectors in (30) are assumed constant over each element. Equation (29) is integrated in time using the trapezoidal rule which is second-order accurate and unconditionally stable, at least for the classical heat equation.

Appendix 2: Poro-Elasticity and Undrained Conditions

The objective is to complement the poro-elasticity theory presented in Sect. 2.2 with the undrained condition. It is defined by the absence of gas mass exchange between the representative volume element and the external domain. Two approaches are considered, the first at the macroscale and the second based on a thought experiment with two interpretations.

At the macroscale, experiences with undrained conditions lead to the interpretation that the relation between stress change and strain is linear

(31)

with an undrained, isotropic stiffness characterized by the incompressibility $\kappa _u$ and a shear modulus G. The former material parameter is larger than $\kappa$ since the fluid phase participates to the elastic stiffness of the whole. The latter parameter is identical for drained and undrained conditions since it is associated with the deviatory deformation which is not sensitive to the nature of the fluid phase. For a spherical stress change, relation (31) simplifies to

$$\begin{aligned} \Delta P = \kappa _u \theta . \end{aligned}$$

(32)

The volumetric strain $\theta$ leads to a pressure change which is obtained by setting to zero the mass change in (6)

$$\begin{aligned} \phi _0 C_{eq0} \Delta p + R_\theta \theta = 0, \end{aligned}$$

(33)

considering a stress change and a fluid pressure change from the initial conditions assumed for the linearization.

Consider now a thought experiment where the force density on the external boundary is $\Delta P \underline{{n}}$. The macroscale interpretation for undrained conditions is that the deformation is spherical and provided by (32). This experiment is now repeated under drained conditions and the same external loading. The free parameter is the pressure change which is adjusted such that the spherical deformation is the same in the two experiments. This pressure change is thus given by (10). Elimination of the deformation from (32) and (10) provides

$$\begin{aligned} \Delta p = - \Delta P B \quad \mathrm{with}\quad B = \frac{\kappa _u-\kappa }{\alpha \kappa _u}, \end{aligned}$$

(34)

in which B is Skempton coefficient.

We thus have derived two expressions relating the fluid pressure change to the hydrostatic stress change under drained conditions which are (33) and (34) once the volumetric strain is replaced by $\Delta P/\kappa _u$ in the former relation. The determinant of this system of equations has to be zero for a non-trivial solution to exist, a condition from which the following relation between the undrained and the drained incompressibilities is derived

$$\begin{aligned} \kappa _u = \kappa + \frac{\alpha (\alpha -\phi _0)}{\phi _0 C_{eq0}} \, , \end{aligned}$$

(35)

having made use of (13).