Volume Averaging Study of the Capacitive Deionization Process in Homogeneous Porous Media

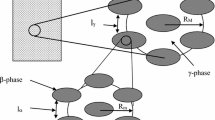
Abstract

Ion storage in porous electrodes is important in applications such as energy storage by supercapacitors, water purification by capacitive deionization, extraction of energy from a salinity difference and heavy ion purification. A model is presented to simulate the charge process in homogeneous porous media comprising big pores. It is based on a theory for capacitive charging by ideally polarizable porous electrodes without faradaic reactions or specific adsorption of ions. A volume averaging technique is used to derive the averaged transport equations in the limit of thin electrical double layers. Transport between the electrolyte solution and the charged wall is described using the Gouy–Chapman–Stern model. The effective transport parameters for isotropic porous media are calculated solving the corresponding closure problems. The source terms that appear in the average equations are calculated using numerical computations. An alternative way to deal with the source terms is proposed.

Appendix

1.1 Order of Magnitude Analysis in Eq. (18)

$$\begin{aligned} \varepsilon _{\upalpha } \partial \langle {c}_\upalpha \rangle ^{\upalpha }/\partial t= & {} \underline{\nabla } \bullet ({\underline{\underline{D}}}_{\mathrm{eff}} \bullet \underline{\nabla }\langle {c}_\upalpha \rangle ^{\upalpha }) + \underline{\nabla } \bullet (D \varepsilon _{\upalpha } \underline{u} \partial \langle {w}\rangle _{\upalpha \upbeta } /\partial t) - a_{v} \partial \langle {w}\rangle _{\upalpha \upbeta } /\partial t, \\&O\left( \frac{{D}{\left\langle {{c}_{\upalpha }} \right\rangle }^{\upalpha }}{{L L}_{c}}\right) , \quad O\left( \frac{{1}}{{L}}\frac{\partial {\left\langle {w} \right\rangle }_{{\upalpha \upbeta }}}{\partial {t}}\right) , \quad O\left( \frac{{1}}{{l}_\upalpha }\frac{\partial {\left\langle {w} \right\rangle }_{{\upalpha \upbeta }}}{\partial {t}}\right) .\nonumber \end{aligned}$$

(18)

Using, $- \underline{n}_{\upalpha \upbeta }\bullet \underline{\nabla }{f}_2 = 1/D$, we get ${f}_2 \approx O({l}_\upalpha /D)$ because $f_{2}$ varies with $l_{\upalpha }$. From $\underline{{u}}=\frac{{1}}{{V}_{\upalpha }}\int \limits _{{A}_{{\upalpha \upbeta }}} {{\underline{{n}}}_{{\upalpha \upbeta }} {f}_{2} { \hbox { d}A}}$, using mean value theorem and ${f}_2 \approx O({l}_\upalpha /D)$ we get, $\underline{{u}}\approx O{(1 / D)}$. Here, we used $A/V_{\upalpha } = a_{V}$ and ${a}_{V} \approx O({l}_\upalpha ^{{-1}})$.

1.2 Order of Magnitude Analysis in Eq. (32)

$$\begin{aligned}&\underline{\nabla } \bullet \{{\underline{\underline{U}}}_{\mathrm{eff}} \bullet \langle {c}_\upalpha \rangle ^{\upalpha } \underline{\nabla }\langle \phi _\upalpha \rangle ^{{\upalpha }}\} + \underline{\nabla }{\bullet }\left\{ \frac{{\varepsilon }_{\upalpha } { U}{\left\langle {{c}_{\upalpha }} \right\rangle }^{\upalpha }}{{V}_{\upalpha }}\left( \int \limits _{{A}_{{\upalpha \upbeta }}} {\underline{{n}}}_{{\upalpha \upbeta }} { g}_{2} \hbox { d}A\right) \frac{\partial {\left\langle {q} \right\rangle }_{{\upalpha \upbeta }}}{\partial {t}}\right\} \nonumber \\&\quad O\left( \frac{{U \varepsilon }_\upalpha {\left\langle {{c}_{\upalpha }} \right\rangle }^{\upalpha } {\left\langle \phi \right\rangle }^{\upalpha }}{{L L}_{c}}\right) , \quad O\left( \frac{{1}}{{L}}\frac{\partial {\left\langle {q} \right\rangle }_{{\upalpha \upbeta }}}{\partial {t}}\right) ,\nonumber \\&\qquad +\, \underline{\nabla } \bullet \left\{ \frac{{\varepsilon }_{\upalpha } { U}}{{V}_{\upalpha }}\int \limits _{{V}_\upalpha } {{{\tilde{c}}}_\upalpha \underline{\nabla }{\tilde{\phi }}_\upalpha { \hbox { d}V}} \right\} - a_{v} \quad \frac{\partial {\left\langle {q} \right\rangle }_{{\upalpha \upbeta }}}{\partial {t}} = 0,\\&\qquad O\left( \frac{{U \varepsilon }_\upalpha {\left\langle {{c}_{\upalpha }} \right\rangle }^{\upalpha } {\left\langle \phi \right\rangle }^{\upalpha }}{{L L}_{c}}\frac{{l}_\upalpha }{{L}}\right) , \quad O\left( \frac{{1}}{{l}_\upalpha }\frac{\partial {\left\langle {q} \right\rangle }_{{\upalpha \upbeta }}}{\partial {t}}\right) .\nonumber \end{aligned}$$

(32)

The order of magnitude for the deviation variables ${\tilde{c}}_{\upalpha }$ and $\tilde{\phi }_{\upalpha }$ were obtained using Eqs. (23) and (36). We use ${g}_2 \approx O\left( {l}_\upalpha / \left( U{\left\langle {{c}_{\upalpha }} \right\rangle }^\upalpha \right) \right)$ estimated from Eq. (41).