Upscaling for Adiabatic Solid–Fluid Reactions in Porous Medium Using a Volume Averaging Theory

Abstract

In this paper, an upscaling study of solid–fluid combustion in porous medium with homogeneous and heterogeneous heat sources is carried out using a volume averaging theory. For the sake of simplicity, the reaction rate is assumed to be of first-order Arrhenius type and convection is not taken into account. Local thermal non-equilibrium is considered between the solid and fluid phases. During the resolution of closure problems, periodic boundary condition is utilized in order to determine the effective coefficients in the upscaled model.The obtained macroscale theory is validated against direct numerical simulation results for two typical porous medium geometries made of simple unit cells, namely unconsolidated and consolidated porous media. The comparisons between the present upscaled and microscale results are conducted for various Damköhler numbers for both homogeneous and heterogeneous reaction cases. It has been found that, for the low Damköhler number cases, the temperature profiles generated from the derived upscaled model are in accordance with that of the microscale model. For the high Damköhler number cases, however, the macroscale model fails to predict the combustion front and temperature profile, which evidently suggests that the effects of neglected terms during the upscaling process should be re-examined carefully in further investigations.

Appendices

Appendix 1: Closure Problems

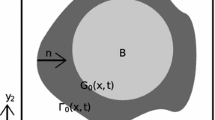
Here, we followed the same manipulations conducted by Quintard and Whitaker (1993) except that, in our upscaled equation, we have an extra reaction term for the solid phase. The closure variables, defined in Eqs. (27a) and (27b), are introduced in Eqs. (24) and (25) and for these equations to be satisfied at the leading order in terms of ${\left\langle T_{{m}}\right\rangle }^m$, ${\left\langle T_{{f}}\right\rangle }^f$, $\nabla {\left\langle T_{{m}}\right\rangle }^m$ and $\nabla {\left\langle T_{{f}}\right\rangle }^f$, they must satisfy the closure problems discussed below.

In the next step, we only need to determine these variables in some representative region in order to evaluate the terms in macroscopic equations that contain the spatial deviation variables. The first closure problem is associated with $\nabla {\left\langle T_{{m}} \right\rangle }^m$ and takes the following form.

$$\begin{aligned} k_{{m}}\nabla ^2\mathbf {b}_{mm}=\epsilon ^{-1}_{{m}}\mathbf {C}_{mm} \quad \quad \quad \text {in the solid} \end{aligned}$$

(57)

$$\begin{aligned} \mathbf {b}_{mm}&=\mathbf {b}_{{fm}} \quad \quad \quad \text {at}\, A_{{fm}} \end{aligned}$$

(58a)

$$\begin{aligned} \mathbf {n}_{mf}\cdot k_{{m}} \nabla \mathbf {b}_{mm}&= \mathbf {n}_{mf}\cdot k_{{f}} \nabla \mathbf {b}_{{fm}}-\mathbf {n}_{mf}k_{{m}} \quad \quad \quad \text {at}\, A_{{fm}} \end{aligned}$$

(58b)

$$\begin{aligned} 0=k_{{f}}\nabla ^2\mathbf {b}_{{fm}}+\epsilon ^{-1}_{{f}}\mathbf {C}_{mm} \quad \quad \quad \text {in the fluid} \end{aligned}$$

(59)

$$\begin{aligned}&\text {Periodicity:} \quad \mathbf {b}_{{fm}} \left( \mathbf {r} + \ell _i\right) = \mathbf {b}_{{fm}} \left( \mathbf {r} \right) , \quad \quad \mathbf {b}_{mm} \left( \mathbf {r} + \ell _i\right) = \mathbf {b}_{mm} \left( \mathbf {r} \right) , \quad \quad i=1,2,3 \end{aligned}$$

(60a)

$$\begin{aligned}&\text {Average:} \quad {\left\langle \mathbf {b}_{{fm}} \right\rangle }^f=0, \quad \quad {\left\langle \mathbf {b}_{mm} \right\rangle }^m=0 \end{aligned}$$

(60b)

Here, $\mathbf {C}_{mm}$ is the unknown integral represented by

$$\begin{aligned} \mathbf {C}_{mm}=\frac{1}{V}\int _{A_{mf}}\mathbf {n}_{mf}\cdot k_{{m}} \nabla \mathbf {b}_{mm} dA = \frac{1}{V}\int _{A_{mf}}\mathbf {n}_{mf}\cdot k_{{f}} \nabla \mathbf {b}_{{fm}} dA \end{aligned}$$

(61)

A detailed description of the evaluation of this unknown integral is given by Quintard et al. (1997).

The term $\nabla {\left\langle T_{{f}} \right\rangle }^f$ is also a source in the closure problem for $\widetilde{T}_{{m}}$ and $\widetilde{T}_{{f}}$ . The boundary value problem associated with the closure variable for $\nabla {\left\langle T_{{f}} \right\rangle }^f$ is given by

$$\begin{aligned} k_{{m}}\nabla ^2\mathbf {b}_{mf}=\epsilon ^{-1}_{{m}}\mathbf {C}_{mf} \quad \quad \quad \text {in the solid} \end{aligned}$$

(62)

$$\begin{aligned} \mathbf {b}_{mf}= & {} \mathbf {b}_{ff} \quad \quad \quad \text {at }A_{{fm}} \end{aligned}$$

(63a)

$$\begin{aligned} \mathbf {n}_{mf}\cdot k_{{m}} \nabla \mathbf {b}_{mf}= & {} \mathbf {n}_{mf}\cdot k_{{f}} \nabla \mathbf {b}_{ff}+\mathbf {n}_{mf}k_{{f}}\quad \quad \quad \text {at }A_{{fm}} \end{aligned}$$

(63b)

$$\begin{aligned} 0=k_{{f}}\nabla ^2\mathbf {b}_{ff}+\epsilon ^{-1}_{{f}}\mathbf {C}_{mf} \quad \quad \quad \text {in the fluid} \end{aligned}$$

(64)

$$\begin{aligned}&\text {Periodicity:} \quad \mathbf {b}_{ff} \left( \mathbf {r} + \ell _i\right) = \mathbf {b}_{ff} \left( \mathbf {r} \right) , \quad \quad \mathbf {b}_{mf} \left( \mathbf {r} + \ell _i\right) = \mathbf {b}_{mf} \left( \mathbf {r} \right) , \quad \quad i=1,2,3 \end{aligned}$$

(65a)

$$\begin{aligned}&\text {Average:} \quad {\left\langle \mathbf {b}_{ff} \right\rangle }^f=0, \quad \quad {\left\langle \mathbf {b}_{mf} \right\rangle }^m=0 \end{aligned}$$

(65b)

Here, $\mathbf {C}_{mf}$ is the unknown integral represented by

$$\begin{aligned} \mathbf {C}_{mf}=\frac{1}{V}\int _{A_{mf}}\mathbf {n}_{mf}\cdot k_{{m}} \nabla \mathbf {b}_{mf} dA = \frac{1}{V}\int _{A_{mf}}\mathbf {n}_{mf}\cdot k_{{f}} \nabla \mathbf {b}_{ff} dA \end{aligned}$$

(66)

Moving on to the source represented by $\left\langle T_{{f}}\right\rangle ^f-\left\langle T_{{m}}\right\rangle ^m$ in Eq. (26a), we construct the following boundary problem for the closure scalars $s_{{m}}$ and $s_{{f}}$.

$$\begin{aligned} 0=k_{{m}}\nabla ^2 s_{{m}}+\epsilon ^{-1}_{{m}}h \quad \quad \quad \text {in the solid} \end{aligned}$$

(67)

$$\begin{aligned} s_{{f}}=&s_{{m}} +1 \quad \quad \quad \text {at }A_{{fm}} \end{aligned}$$

(68a)

$$\begin{aligned} \mathbf {n}_{mf}\cdot k_{{m}} \nabla s_{{m}} =&\mathbf {n}_{mf}\cdot k_{{f}} \nabla s_{{f}} \quad \quad \quad \text {at }A_{{fm}} \end{aligned}$$

(68b)

$$\begin{aligned} 0=k_{{f}}\nabla ^2s_{{f}} - \epsilon ^{-1}_{{f}}h \quad \quad \quad \text {in the fluid} \end{aligned}$$

(69)

$$\begin{aligned}&\text {Periodicity:} \quad s_{{f}} \left( \mathbf {r} + \ell _i\right) = s_{{f}} \left( \mathbf {r} \right) , \quad \quad s_{{m}} \left( \mathbf {r} + \ell _i\right) = s_{{m}} \left( \mathbf {r} \right) , \quad \quad i=1,2,3 \end{aligned}$$

(70a)

$$\begin{aligned}&\text {Average:} \quad {\left\langle s_{{f}} \right\rangle }^f=0, \quad \quad {\left\langle s_{{m}} \right\rangle }^m=0 \end{aligned}$$

(70b)

In this closure problem, the undetermined constant is represented by

$$\begin{aligned} h=\frac{1}{V}\int _{A_{mf}}\mathbf {n}_{{fm}}\cdot k_{{m}} \nabla s_{{m}} dA = \frac{1}{V}\int _{A_{mf}}\mathbf {n}_{{fm}}\cdot k_{{f}} \nabla s_{{f}} dA \end{aligned}$$

(71)

Appendix 2: Large Conductivity Ratio Behavior of the Closure Problems

In this appendix, we are interested in the behavior of the closure problems in the limit of $k_{{m}}/k_{{f}}$ (or $k_{{f}}/k_{{m}}$) going to infinity. The results are clear for the mapping variables and the distribution coefficient as illustrated in Fig. 7.

For the closure problem involving the mapping variable $s_{{m}}$ and $s_{{f}}$, we have by looking at the limit of Eqs. (67) through (71) when $k_{{m}}/k_{{f}} \rightarrow +\infty$:

$$\begin{aligned} s_{{m}}=0 \end{aligned}$$

(72)

and

$$\begin{aligned} \frac{h l_{{f}}^{2}}{k_{{f}}} = constant \end{aligned}$$

(73)

Similarly, when $k_{{f}}/k_{{m}} \rightarrow +\infty$, we have

$$\begin{aligned} s_{{f}}=0 \end{aligned}$$

(74)

and,

$$\begin{aligned} \frac{h l_{{f}}^{2}}{k_{{m}}} = \hbox {constant} \end{aligned}$$

(75)

This simply gives the obvious physical result that the heat transfer resistance is due to the less conductive material in these limiting cases.

For the closure problems involving the $\mathbf {b}_{\alpha \beta }$ mapping variables, we can develop the following estimates. Eqs. (57) through (61) lead to in the limit $k_{{m}}/k_{{f}} \rightarrow +\infty$:

$$\begin{aligned} \mathbf {b}_{mm}= & {} 0 \quad \quad \quad \quad \text {in }V_{{m}} \end{aligned}$$

(76)

$$\begin{aligned} \mathbf {n}_{mf}\cdot k_{{f}} \nabla \mathbf {b}_{mm}= & {} - \mathbf {n}_{mf} \quad \quad \text {at }A_{{fm}} \end{aligned}$$

(77)

$$\begin{aligned}&\text {Periodicity:} \quad \quad \mathbf {b}_{mm} \left( \mathbf {r} + \ell _i\right) = \mathbf {b}_{mm} \left( \mathbf {r} \right) , \quad \quad i=1,2,3 \end{aligned}$$

(78a)

$$\begin{aligned}&\text {Average:} \quad \quad {\left\langle \mathbf {b}_{mm} \right\rangle }^m=0 \end{aligned}$$

(78b)

which is the typical effective diffusion problem for the $m$-phase. Its solution gives for a percolating $m$-phase, and in the isotropic case:

$$\begin{aligned} \mathbf {K}_{mm} =\epsilon _{{m}} k_{{m}} \mathbf {I}+\frac{k_{{m}}}{V}\int _{A_{mf}}\mathbf {n}_{mf} \mathbf {b}_{mm}dA=\frac{\epsilon _{{m}} k_{{m}}}{\tau _{{m}}} \mathbf {I} \end{aligned}$$

(79)

where $\tau _{{m}}$ is the tortuosity given by the solution of the closure problem over a representative unit cell.

Similarly, in the case $k_{{f}}/k_{{m}} \rightarrow +\infty$, we obtain by looking at Eqs. (62) through (66)

$$\begin{aligned} \mathbf {K}_{ff}=\epsilon _{{f}} k_{{f}} \mathbf {I}+\frac{k_{{f}}}{V}\int _{A_{mf}}\mathbf {n}_{mf}\mathbf {b}_{ff}dA =\frac{\epsilon _{{f}} k_{{f}}}{\tau _{{f}}} \mathbf {I} \end{aligned}$$

(80)

where this time $\tau _{{f}}$ is the calculated tortuosity of the percolating isotropic $f$-phase.

Appendix 3: Normalization of Governing Equations

In this appendix, the microscale and macroscale governing equations for both homogeneous and heterogeneous reactions presented in this paper are normalized. Firstly, the dimensionless governing equations for homogeneous reaction are written as:

$$\begin{aligned} \frac{\partial T^{*}_{{m}}}{\partial t^*}=\nabla ^2 T^{*}_{{m}}+\frac{Da}{E/RT_{\mathrm{in}}}e^{-\frac{E}{RT_{\mathrm{in}}} \left( \frac{1}{T^{*}_{{m}}}-1 \right) } \end{aligned}$$

(81)

and

$$\begin{aligned} \frac{\partial T^{*}_{{f}}}{\partial t^*}\frac{\left( \rho c_p \right) _{{f}}/k_{{f}}}{\left( \rho c_p \right) _{{m}}/k_{{m}}}=\nabla ^2 T^{*}_{{f}} \end{aligned}$$

(82)

The interfacial boundary condition is expressed by

$$\begin{aligned} T^*_{{m}}&=T^*_{{f}} \end{aligned}$$

(83a)

$$\begin{aligned} \mathbf {n}_{mf} \cdot \frac{k_{{m}}}{k_{{f}}} \nabla T^*_{{m}}&= \mathbf {n}_{mf} \cdot \nabla T^*_{{f}} \end{aligned}$$

(83b)

where

$$\begin{aligned} T^*_{{m}}=T_{{m}}/T_{\mathrm{in}}, \quad \quad T^*_{{f}}&=T_{{f}}/T_{\mathrm{in}}, \quad \quad t^*=t\left( k_{{m}}/\left( \rho c_p \right) _{{m}} \right) /L^{2}_{\mathrm{ref}} \end{aligned}$$

(84a)

$$\begin{aligned} Da&= \frac{H_{rxn}}{(\rho c_p)_{{m}}} \frac{E}{R T^2_{\mathrm{in}} } \frac{A_0 e^{-E/R T_{\mathrm{in}} } L^{2}_{\mathrm{ref}} }{k_{{m}}/(\rho c_p)_{{m}}} \end{aligned}$$

(84b)

The corresponding dimensionless macroscopic governing equations based on Eqs. (28) and (29) are written as:

$$\begin{aligned} \epsilon _{{m}}\frac{\partial \left\langle T^*_{{m}} \right\rangle ^m}{\partial t^*}= & {} \nabla \cdot \left( \mathbf {K}^*_{mm}\cdot \nabla {\left\langle T^*_{{m}} \right\rangle }^m+\mathbf {K}^*_{mf}\cdot \nabla {\left\langle T^*_{{f}} \right\rangle }^f\right) +\mathbf {u}^*_{mm}\cdot \nabla {\left\langle T^*_{{m}} \right\rangle }^m\nonumber \\&+\,\mathbf {u}^*_{mf}\cdot \nabla {\left\langle T^*_{{f}} \right\rangle }^f-h^*\left( {\left\langle T^*_{{m}} \right\rangle }^m-{\left\langle T^*_{{f}} \right\rangle }^f\right) \nonumber \\&+\,\epsilon _{{m}} \frac{Da}{E/RT_{\mathrm{in}}}e^{-\frac{E}{RT_{\mathrm{in}}}\left( \frac{1}{{\left\langle T^*_{{m}} \right\rangle }^m }-1 \right) } \end{aligned}$$

(85)

$$\begin{aligned} \epsilon _{{f}}\frac{\left( \rho c_p \right) _{{f}}/k_{{f}}}{\left( \rho c_p \right) _{{m}}/k_{{m}}}\frac{\partial \left\langle T^*_{{f}} \right\rangle ^f}{\partial t^*}= & {} \nabla \cdot \left( \mathbf {K}^*_{ff}\cdot \nabla {\left\langle T^*_{{f}} \right\rangle }^f+\mathbf {K}^*_{{fm}}\cdot \nabla {\left\langle T^*_{{m}} \right\rangle }^m\right) \nonumber \\&+\,\mathbf {u}^*_{{fm}}\cdot \nabla {\left\langle T^*_{{m}} \right\rangle }^m+\mathbf {u}^*_{ff}\cdot \nabla {\left\langle T^*_{{f}} \right\rangle }^f+h^*\frac{k_{{m}}}{k_{{f}}}\left( {\left\langle T^*_{{m}} \right\rangle }^m-{\left\langle T^*_{{f}} \right\rangle }^f\right) \nonumber \\ \end{aligned}$$

(86)

where

$$\begin{aligned} {\left\langle T^*_{{m}} \right\rangle }^m= & {} {\left\langle T_{{m}} \right\rangle }^m/T_{\mathrm{in}}, \quad {\left\langle T^*_{{f}} \right\rangle }^f={\left\langle T_{{f}} \right\rangle }^f/T_{\mathrm{in}}, \quad \mathbf {K}^*_{mm}=\mathbf {K}_{mm}/k_{{m}} \end{aligned}$$

(87a)

$$\begin{aligned} \mathbf {K}^*_{mf}= & {} \mathbf {K}_{mf}/k_{{m}}, \quad \mathbf {K}^*_{ff}=\mathbf {K}_{ff}/k_{{f}}, \quad \mathbf {K}^*_{{fm}}=\mathbf {K}_{{fm}}/k_{{f}} \end{aligned}$$

(87b)

$$\begin{aligned} \mathbf {u}^*_{mm}= & {} \mathbf {u}_{mm}L_{\mathrm{ref}}/k_{{m}}, \quad \mathbf {u}^*_{mf}=\mathbf {u}_{mf}L_{\mathrm{ref}}/k_{{m}}, \quad \mathbf {u}^*_{{fm}}=\mathbf {u}_{{fm}}L_{\mathrm{ref}}/k_{{f}} \end{aligned}$$

(87c)

$$\begin{aligned} \mathbf {u}^*_{ff}= & {} \mathbf {u}_{ff}L_{\mathrm{ref}}/k_{{f}}, \quad \quad \quad h^*=hL^2_{\mathrm{ref}}/k_{{m}} \end{aligned}$$

(87d)

Based on the parameters given in Eq. (84a), the dimensionless microscopic governing equations for heterogeneous reaction are obtained as follows:

$$\begin{aligned} \frac{\partial T^{*}_{{m}}}{\partial t^*}=\nabla ^2 T^{*}_{{m}} \quad \quad \quad \quad \text {in }V_{{m}} \end{aligned}$$

(88)

and

$$\begin{aligned} \frac{\partial T^{*}_{{f}}}{\partial t^*}\frac{\left( \rho c_p \right) _{{f}}/k_{{f}}}{\left( \rho c_p \right) _{{m}}/k_{{m}}}=\nabla ^2 T^{*}_{{f}} \quad \quad \quad \quad \text {in }V_{{f}} \end{aligned}$$

(89)

The adapted boundary conditions are written as

$$\begin{aligned} T^*_{{m}}&=T^*_{{f}} \quad \quad \quad \text {at }A_{{fm}} \end{aligned}$$

(90a)

$$\begin{aligned} \mathbf {n}_{{fm}} \cdot \frac{k_{{f}}}{k_{{m}}}\nabla T^*_{{f}}&= \mathbf {n}_{{fm}} \cdot \nabla T^*_{{m}}+ Da\frac{H_{rxn}}{\left( \rho c_p\right) _{{m}}T_{\mathrm{in}}}e^{-\frac{E}{RT_{\mathrm{in}}}\left( \frac{1}{T^{*}_{{m}}}-1 \right) }\quad \text {at }A_{{fm}} \end{aligned}$$

(90b)

where the Damköhler number for heterogeneous reaction in this paper is different with Eq. (84b) and defined as below

$$\begin{aligned} Da=\frac{A_0 e^{-E/R T_{\mathrm{in}} } L_{\mathrm{ref}} }{k_{{m}}/(\rho c_p)_{{m}}} \end{aligned}$$

(91)

Moreover, we can use Eqs. (87a)–(87d) to normalize Eqs. (55) and (56) and obtain the following dimensionless macroscopic governing equations:

$$\begin{aligned}&\epsilon _{{m}}\frac{\partial \left\langle T^*_{{m}} \right\rangle ^m}{\partial t^*}=\nabla \cdot \left( \mathbf {K}^*_{mm}\cdot \nabla {\left\langle T^*_{{m}} \right\rangle }^m+\mathbf {K}^*_{mf}\cdot \nabla {\left\langle T^*_{{f}} \right\rangle }^f\right) +\mathbf {u}^*_{mm}\cdot \nabla {\left\langle T^*_{{m}} \right\rangle }^m\nonumber \\&\quad +\,\mathbf {u}^*_{mf}\cdot \nabla {\left\langle T^*_{{f}} \right\rangle }^f-h^*\left( {\left\langle T^*_{{m}} \right\rangle }^m-{\left\langle T^*_{{f}} \right\rangle }^f\right) \nonumber \\&\quad +\,a_VL_{\mathrm{ref}}\xi _{{m}} \left( 1 + w^* \frac{E}{R T_{\mathrm{in}}\left( {\left\langle T^*_{{m}}\right\rangle }^m \right) ^2 } \right) Da\frac{H_{rxn}}{\left( \rho c_p\right) _{{m}}T_{\mathrm{in}}}e^{-\frac{E}{RT_{\mathrm{in}}}\left( \frac{1}{{\left\langle T^*_{{m}} \right\rangle }^m}-1 \right) } \end{aligned}$$

(92)

$$\begin{aligned}&\epsilon _{{f}}\frac{\left( \rho c_p \right) _{{f}}/k_{{f}}}{\left( \rho c_p \right) _{{m}}/k_{{m}}}\frac{\partial \left\langle T^*_{{f}} \right\rangle ^f}{\partial t^*}=\nabla \cdot \left( \mathbf {K}^*_{ff}\cdot \nabla {\left\langle T^*_{{f}} \right\rangle }^f+\mathbf {K}^*_{{fm}}\cdot \nabla {\left\langle T^*_{{m}} \right\rangle }^m\right) \nonumber \\&\quad +\,\mathbf {u}^*_{{fm}}\cdot \nabla {\left\langle T^*_{{m}} \right\rangle }^m+\mathbf {u}^*_{ff}\cdot \nabla {\left\langle T^*_{{f}} \right\rangle }^f+h^*\frac{k_{{m}}}{k_{{f}}}\left( {\left\langle T^*_{{m}} \right\rangle }^m-{\left\langle T^*_{{f}} \right\rangle }^f\right) \nonumber \\&\quad +\,a_VL_{\mathrm{ref}}\xi _{{f}} \left( 1 + w^* \frac{E}{R T_{\mathrm{in}}\left( {\left\langle T^*_{{m}}\right\rangle }^m \right) ^2 } \right) Da\frac{H_{rxn}}{\left( \rho c_p\right) _{{m}}T_{\mathrm{in}}}e^{-\frac{E}{RT_{\mathrm{in}}}\left( \frac{1}{{\left\langle T^*_{{m}} \right\rangle }^m}-1 \right) } \end{aligned}$$

(93)

where the spatial deviation dimensionless temperature of solid phase at the interface is given by

$$\begin{aligned}&w^*=w/T_{\mathrm{in}}\nonumber \\&\quad =\frac{ \frac{E}{RT_{\mathrm{in}}\left( \left\langle T^*_{{f}}\right\rangle ^f \right) ^2} e^{\frac{E}{RT_{\mathrm{in}}\left\langle T^*_{{f}}\right\rangle ^f}} \left( \left\langle T^*_{{m}}\right\rangle ^m - \left\langle T^*_{{f}}\right\rangle ^f \right) - \left( e^{\frac{E}{RT_{\mathrm{in}}\left\langle T^*_{{m}}\right\rangle ^m}} - e^{\frac{E}{RT_{\mathrm{in}}\left\langle T^*_{{f}}\right\rangle ^f}} \right) }{ \frac{E}{RT_{\mathrm{in}}\left( \left\langle T^*_{{m}}\right\rangle ^m \right) ^2} e^{\frac{E}{RT_{\mathrm{in}}\left\langle T^*_{{m}}\right\rangle ^m}} - \frac{E}{RT_{\mathrm{in}}\left( \left\langle T^*_{{f}}\right\rangle ^f \right) ^2} e^{\frac{E}{RT_{\mathrm{in}}\left\langle T^*_{{f}}\right\rangle ^f}}} \qquad \end{aligned}$$

(94)