Abstract
A review is given for cyclotron resonant interactions in space plasmas. After giving a simple formulation for the test particle approach, illustrative examples for resonant interactions are given. It is shown that for obliquely propagating whistler waves, not only fundamental cyclotron resonance, but also other resonances, such as transit-time resonance, anomalous cyclotron resonance, higher-harmonic cyclotron resonance, and even subharmonic resonance can come into play. A few recent topics of cyclotron resonant interactions, such as electron injection in shocks, cyclotron resonant heating of solar wind heavy ions, and relativistic modifications, are also reviewed.










Similar content being viewed by others
Notes
Note, however, that there still remains self nonlinearity in (3) for the mode #, where \({\mathbf{E}_{w}^{\#}}\) and \({\mathbf{B}_{w}^{\#}}\) are evaluated at the particle position r e (t), which is affected by \({\mathbf{E}_{w}^{\#}}\) and \({\mathbf{B}_{w}^{\#}}\) themselves. This nonlinearity is the origin of subharmonic resonances to be discussed in Sect. 4.5.
From this consideration it is naturally understood why there is no anomalous resonance effect for a parallel propagating whistler wave (Fig. 1): An electron satisfying the n=−1 resonance condition feels the whistler wave left-hand polarized in its own comoving frame and does not exchange energy and momentum with the wave efficiently.
In this and next subsections we consider the resonant interaction of ions and set the sign of ω positive for the left-hand polarized waves.
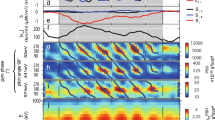
The authors took the z axis along B 0. The limiting velocity v 0 for the multiple interactions corresponds to the condition tangential to the wave dispersion relation (Fig. 8(c)).
Note the following relation for the Lorentz factor,
$$\gamma\equiv\bigl\{1-\bigl(v_x^2+v_\perp^2\bigr)/c^2 \bigr\}^{-1/2} =\bigl(1+u_\parallel^2+u_\perp^2\bigr)^{1/2}.$$
References
T. Amano, M. Hoshino, A critical Mach number for electron injection in collisionless shocks. Phys. Rev. Lett. 104, 181102 (2010)
D.D. Barbosa, W.S. Kurth, Suprathermal electrons and Bernstein waves in Jupiter’s inner magnetosphere. J. Geophys. Res. 85, 6729 (1980)
N. Brice, Fundamentals of very low frequency emission generation mechanisms. J. Geophys. Res. 69, 4515 (1964)
C. Cattell, J.R. Wygant, K. Goetz et al., Discovery of very large amplitude whistler-mode waves in Earth’s radiation belt. Geophys. Res. Lett. 35, L01105 (2008)
L. Chen, Z. Lin, R. White, On resonant heating below the cyclotron frequency. Phys. Plasmas 8, 4713 (2001)
C.M. Cully, J.W. Bonnell, R.E. Ergun, THEMIS observations of long-lived regions of large-amplitude whistler waves in the inner magnetosphere. Geophys. Res. Lett. 35, L17S16 (2008)
D.H. Fairfield, Bow shock associated waves observed in the far upstream interplanetary medium. J. Geophys. Res. 74, 3541 (1969)
N. Furuya, Y. Omura, D. Summers, Relativistic turning acceleration of radiation belt electrons by whistler mode chorus. J. Geophys. Res. 113, A04224 (2008)
V.L. Ginzburg, Certain theoretical aspects of radiation due to superluminal motion in a medium. Sov. Phys. Usp. 2, 874 (1960)
K. Hasselmann, G. Wibberenz, Scattering of charged particles by random electromagnetic fields. Z. Geophys. 34, 353 (1968)
D.E. Hall, P.A. Sturrock, Diffusion, scattering, and acceleration of particles by stochastic electromagnetic field. Phys. Fluids 10, 2620 (1967)
A. Hasegawa, L. Chen, Kinetic processes in plasma-heating by resonant mode conversion of Alfvén wave. Phys. Fluids 19, 1924 (1976)
R.A. Helliwell, Controlled VLF wave injection experiments in the magnetosphere. Space Sci. Rev. 15, 781 (1974)
M. Hillas, The origin of ultra-high-energy cosmic rays. Annu. Rev. Astron. Astrophys. 22, 425 (1984)
J.V. Hollweg, P.A. Isenberg, Generation of fast solar wind: a review with emphasis on the resonant cyclotron interaction. J. Geophys. Res. 107, 1147 (2002)
M. Hoshino, T. Terasawa, Numerical study of the upstream wave excitation mechanism. 1. Nonlinear phase bunching of beam ions. J. Geophys. Res. 90, 57 (1985)
P.A. Isenberg, B.J. Vasquez, Preferential perpendicular heating of coronal hole minor ions by the Fermi mechanism. Astrophys. J. 668, 546 (2007)
P.A. Isenberg, B.J. Vasquez, Preferential acceleration and perpendicular heating of minor ions in a collisionless coronal hole. Astrophys. J. 696, 591 (2009)
J.R. Jokipii, Propagation of cosmic rays in the solar wind. Rev. Geophys. Space Sci. 9, 27 (1971)
V.I. Karpman, Nonlinear effects in the ELF waves propagating along the magnetic field in the magnetosphere. Space Sci. Rev. 16, 361 (1974)
C.F. Kennel, F. Engelmann, Velocity space diffusion from weak plasma turbulence in a magnetic field. Phys. Fluids 9, 2377 (1966)
C.F. Kennel, H.E. Petschek, Limit on stably trapped particle fluxes. J. Geophys. Res. 71, 1 (1966)
Y. Kuramitsu, V. Krasnoselskikh, Gyroresonant surfing acceleration. Phys. Rev. Lett. 94, 031102 (2005a)
Y. Kuramitsu, V. Krasnoselskikh, Acceleration of charged particles by gyroresonant surfing at quasi-parallel shocks. Astron. Astrophys. 438, 391 (2005b)
R.M. Kulsrud, A. Ferrari, The relativistic quasilinear theory of particle acceleration by hydromagnetic turbulence. Astrophys. Space Sci. 12, 302 (1971)
R.J. Leamon, C.W. Smith, N.F. Ness, H.K. Wong, Dissipation range dynamics: kinetic Alfvén waves and the importance of β e . J. Geophys. Res. 104, 22331 (1999)
M.A. Lee, I. Lerche, Waves and irregularities in the solar wind. Rev. Geophys. Space Sci. 12, 671 (1974)
A. Levinson, Electron injection in collisionless shocks. Astrophys. J. 401, 73 (1992)
Q. Lu, L. Chen, Ion heating by a spectrum of obliquely propagating low-frequency Alfvén waves. Astrophys. J. 704, 743 (2009)
R.L. Lysak, W. Lotko, On the kinetic dispersion relation for shear Alfvén waves. J. Geophys. Res. 101, 5085 (1996)
S. Matsukiyo, T. Hada, Relativistic particle acceleration in developing Alfvén turbulence. Astrophys. J. 692, 1004 (2009)
H. Matsumoto, Nonlinear Whistler-mode interaction and triggered emissions in the magnetosphere, in Wave Instabilities in Space Plasmas, ed. by P.J. Palmadesso, K. Papadopoulos (Reidel, Dordrecht, 1979)
M. Oka, T. Terasawa, Y. Seki, M. Fujimoto, Y. Kasaba, H. Kojima, I. Shinohara, H. Matsui, H. Matsumoto, Y. Saito, T. Mukai, Whistler critical Mach number and electron acceleration at the bow shock: geotail observation. Geophys. Res. Lett. 33, L24104 (2006)
Y. Omura, N. Furuya, D. Summers, Relativistic turning acceleration of resonant electrons by coherent whistler mode waves in a dipole magnetic field. J. Geophys. Res. 112, A06236 (2007)
S. O’Sullivan, B. Reville, A.M. Taylor, Stochastic particle acceleration in the lobes of giant radio galaxies. Mon. Not. R. Astron. Soc. 400, 248 (2009)
P. Palmadesso, G. Schmidt, Collisionless damping of a large amplitude whistler wave. Phys. Fluids 14, 1411 (1971)
V. Petrosian, S. Liu, Stochastic acceleration of electrons and protons. I. Acceleration by parallel-propagating waves. Astrophys. J. 610, 550 (2004)
R. Schlickeiser, Cosmic-ray transport and acceleration. I. Derivation of the kinetic equation and application to cosmic rays in static cold media. Astrophys. J. 336, 243 (1989a)
R. Schlickeiser, Cosmic-ray transport and acceleration. II. Cosmic rays in moving cold media with application to diffusive shock wave acceleration. Astrophys. J. 336, 264 (1989b)
R. Schlickeiser, Cosmic Ray Astrophysics (Springer, Berlin, 2002)
R. Schlickeiser, J.A. Miller, Quasi-linear theory of cosmic ray transport and acceleration: the role of oblique magnetohydrodynamic waves and transit-time damping. Astrophys. J. 492, 352 (1998)
J.P.M. Schmitt, Nonlinear theory of rf heating at cyclotron harmonics. Phys. Fluids 19, 245 (1976)
A. Shalchi, Nonlinear Cosmic Ray Diffusion Theories (Springer, Berlin, 2009)
J. Skilling, Cosmic ray streaming. I. Effect of Alfvén waves on particles. Mon. Not. R. Astron. Soc. 172, 557 (1975)
Y.N. Smirnov, D.A. Frank-Kamenetskii, Nonlinearity and parametric resonance in a plasma. Sov. Phys. JETP 26, 627 (1968)
T.H. Stix, Waves in Plasmas (Springer, New York, 1992)
X. Tao, J. Bortnik, Nonlinear interactions between relativistic radiation belt electrons and oblique whistler mode waves. Nonlinear Process. Geophys. 17, 599 (2010)
T. Terasawa, M. Nambu, Ion heating and acceleration by magnetosonic waves via cyclotron subharmonic resonance. Geophys. Res. Lett. 16, 357 (1989)
J.J.P. Viratanen, R. Vainio, Stochastic acceleration in relativistic parallel shocks. Astrophys. J. 621, 313 (2005)
Y. Watanabe, T. Terasawa, On the excitation mechanism of the low-frequency upstream waves. J. Geophys. Res. 89, 6623 (1984)
R. White, L. Chen, Z. Lin, Resonant plasma heating below the cyclotron frequency. Phys. Plasmas 9, 1890 (2002)
D. Winske, M.M. Leroy, Diffuse ions produced by electromagnetic ion beam instabilities. J. Geophys. Res. 89, 2673 (1984)
Acknowledgements
We would like to thank for valuable discussions and comments to T. Hada, M. Scholer, M.A. Lee, and Y. Ohira. The works by T.T. and S.M. get partial supports from the grants-in-aids 21540259 and 22740323 from Japan Society for the Promotion of Science, respectively.
Author information
Authors and Affiliations
Corresponding author
Appendices
Appendix A
For the j species (j=i or e), the plasma frequency is defined as \(\omega_{pj} = \sqrt{4\pi n_{0} e^{2}/m_{j}}\), and the cyclotron frequency as Ω cj =eB 0/m j c. The Alfvén velocity V A is defined as \(B_{0}/\sqrt{4\pi n_{0} (m_{i}+m_{e})}\), which is chosen to be 10−4 c in the test particle calculation. The ion mass m i is taken 1836 times m e .
The wave electric field E w ∝expi(kr−ωt) should satisfy the following matrix relation (see, e.g., Chap. 2 of Stix 1992),
with a matrix \({\tilde{M}}\) given as
where N≡kc/ω is a refractive index, and P, S, and D are defined as

Note that the condition \(\det {\tilde{M}} =0\) gives the wave dispersion relation.
From the first and second column of the matrix equation (17), we have

Now switching from the complex formulation to the real formulation, we write the xyz components of the electric field, (E w,x , E w,y , E w,z ), as
where the relative amplitudes of (g x , g y , g z ) are determined by (20), and φ is the phase angle,
where φ 0 is the initial phase angle, respectively. From the Faraday’s law, we have
from which the xyz components of the magnetic field, (B w,x , B w,y , B w,z ), are obtained as

Appendix B
In a finite amplitude monochromatic electromagnetic wave propagating parallel to the background magnetic field B 0 charged particles can be phase-space trapped around the resonant velocity (see e.g., Palmadesso and Schmidt 1971; Helliwell 1974; Schmitt 1976; Karpman 1974; Matsumoto 1979; Hoshino and Terasawa 1985; Kuramitsu and Krasnoselskikh 2005b). Here we follow the description in a recent article by Kuramitsu and Krasnoselskikh (2005b). Firstly, the gyrophase angle for an ion is defined as ϕ p ≡tan−1(v z /v y ), the wave phase angle as ϕ w ≡kx−ωt+α w (α w : the initial value), and their difference as ψ≡ϕ w −ϕ p (Fig. 11). Next, with the velocity of ions in the wave rest frame (u x ,u ⊥)=(v x −ω/k,v ⊥) the cosine of the pitch angle is defined as μ≡u x /|u|, where \(|u| = (u_{x}^{2} + u_{\perp}^{2})^{1/2}\) is the constant of motion (namely, the ion energy is conserved in the wave rest frame). The second integral of the ion motion χ can be written as,
where κ=ku/Ω ci , and b the wave amplitude normalized by |B 0|. With χ, the equation of motion is simplified as
Figure 12 shows the ion trajectories in the μ−ψ phase space with κ=3 and b=0.01, 0.1, 1, 10. When the wave amplitude is small ((a): b=0.01), ions are trapped around (μ,ψ)=(−1/κ,π), which is due to the linear cyclotron resonant interaction. As the wave amplitude b becomes larger ((c) and (d)), the trapping region also becomes larger and a new trapping region is born in a location far from the original point (−1/κ,π).
Figure from Kuramitsu and Krasnoselskikh (2005b) showing the definition of angles, ϕ p , ϕ w , and ψ
Figure from Kuramitsu and Krasnoselskikh (2005b) showing particle trajectories in the μ–ψ phase space with κ=3 when (a) b=0.01, (b) b=0.1, (c) b=1, and (d) b=10. One curve corresponds to one constant of motion χ
Rights and permissions
About this article
Cite this article
Terasawa, T., Matsukiyo, S. Cyclotron Resonant Interactions in Cosmic Particle Accelerators. Space Sci Rev 173, 623–640 (2012). https://doi.org/10.1007/s11214-012-9878-0
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11214-012-9878-0