Abstract
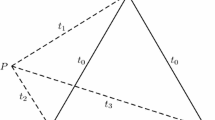
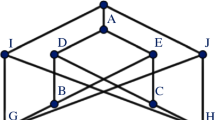
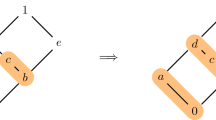
We call a finite lattice crosscut-simplicial if the crosscut complex of every nuclear interval is equal to the boundary of a simplex. Every interval of such a lattice is either contractible or homotopy equivalent to a sphere. Recently, Hersh and Mészáros introduced SB-labelings and proved that if a lattice has an SB-labeling then it is crosscut-simplicial. Some known examples of lattices with a natural SB-labeling include the join-distributive lattices, the weak order of a Coxeter group, and the Tamari lattice. Generalizing these three examples, we prove that every meet-semidistributive lattice is crosscut-simplicial, though we do not know whether all such lattices admit an SB-labeling. While not every crosscut-simplicial lattice is meet-semidistributive, we prove that these properties are equivalent for chamber posets of real hyperplane arrangements.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Björner, A., Edelman, P.H., Ziegler, G.M.: Hyperplane arrangements with a lattice of regions. Discrete Comput. Geom. 5(3), 263–288 (1990)
Björner, A.: Orderings of Coxeter groups. Combinatorics and Algebra 34, 175–195 (1984)
Bjorner, A.: Topological methods. Handbook of Combinatorics 2, 1819–1872 (1995)
Björner, A., Brenti, F.: Combinatorics of Coxeter Groups, vol. 231. Springer Science+ Business Media (2005)
Björner, A., Las Vergnas, M., Sturmfels, B., White, N., Ziegler, G.M.: Oriented Matroids, vol. 46. Cambridge University Press (1999)
Caspard, N., Le Conte de Poly-Barbut, C., Morvan, M.: Cayley lattices of finite coxeter groups are bounded. Adv. Appl. Math. 33(1), 71–94 (2004)
Day, A.: Congruence normality: the characterization of the doubling class of convex sets. Algebra Universalis 31(3), 397–406 (1994)
Edelman, P.H.: Meet-distributive lattices and the anti-exchange closure. Algebra Universalis 10(1), 290–299 (1980)
Edelman, P.H.: A partial order on the regions of ℝn dissected by hyperplanes. Trans. Am. Math. Soc. 283(2), 617–631 (1984)
Edelman, P.H., Walker, J.W.: The homotopy type of hyperplane posets. Proc. Am. Math. Soc. 94(2), 221–225 (1985)
Funayama, N., Nakayama, T., et al.: On the distributivity of a lattice of lattice-congruences. Proceedings of the Imperial Academy 18(9), 553–554 (1942)
Hersh, P., Meszaros, K.: SB-labelings and posets with each interval homotopy equivalent to a sphere or a ball. arXiv:1407.5311 (2014)
Jambu, M., Paris, L.: Combinatorics of inductively factored arrangements. Eur. J. Comb. 16(3), 267–292 (1995)
Le Conte de Poly-Barbut, C.: Sur les treillis de coxeter finis. Mathématiques, informatique et Sciences Humaines 125, 41–57 (1994)
Mühle, H.: SB-Labelings, distributivity, and Bruhat order on sortable elements ArXiv e-prints (2014)
Reading, N.: Lattice and order properties of the poset of regions in a hyperplane arrangement. Algebra Universalis 50(2), 179–205 (2003)
Reading, N.: Lattice congruences, Fans and Hopf algebras. Journal of Combinatorial Theory, Series A 110(2), 237–273 (2005)
Reading, N.: Cambrian lattices. Adv. Math. 205(2), 313–353 (2006)
Stanley, R.P.: Enumerative combinatorics. Wadsworth Publ. Co., Belmont, CA, USA (1986)
Walker, J.W.: Canonical homeomorphisms of posets. Eur. J. Comb. 9(2), 97–107 (1988)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
McConville, T. Crosscut-Simplicial Lattices. Order 34, 465–477 (2017). https://doi.org/10.1007/s11083-016-9409-9
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11083-016-9409-9