Onset of colored-noise-induced chaos in the generalized Duffing system

Abstract

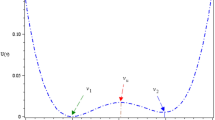
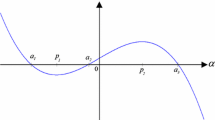
The effects of colored noise, red noise and green noise, on the onset of chaos are investigated theoretically and confirmed numerically in the generalized Duffing system with a fractional-order deflection. Analytical predictions concerning the chaotic thresholds in the parameter space are derived by using the stochastic Melnikov method combined with the mean-square criterion. To qualitatively confirm the analytical results, numerical simulations obtained from the mean largest Lyapunov exponent are used as test beds. We show that colored noise can induce chaos, and the effects for the case of red noise on the onset of chaos differ from those for the case of green noise. The most noteworthy result of this work is the formula, which relates the chaotic thresholds among red, green and white noise, holds for noise-induced chaos in the Duffing system. We also show that Gaussian white noise can induce chaos more easily than colored noise.

Appendix

Here, we will clarify the proposed second-order stochastic Runge–Kutta algorithm for integrating the differential equations under green noise. The basic idea of the algorithm is similar to that in [37] where white or red noise is treated.

We start with formally integrating Eq. (7) from time 0 to time $\Delta t$ :

$$\begin{aligned} z(\Delta t)= & {} {z_0} + \int _0^{\Delta t} {f(z(t'))} dt' + \int _0^{\Delta t} {\xi (t')} dt' \nonumber \\&- \int _0^{\Delta t} {\eta (t')} dt',\nonumber \\ \xi (\Delta t)= & {} {\xi _0} + \int _0^{\Delta t} {( - \gamma \xi (t'))} dt' + \int _0^{\Delta t} {\gamma \eta (t')} dt'.\nonumber \\ \end{aligned}$$

(26)

Defining

$$\begin{aligned} {\varGamma _0}(t)\equiv & {} \int _0^{t} {\eta (t')} dt',\\ {\varGamma _i}(t)\equiv & {} \int _0^{t} {{\varGamma _{i - 1}}(t')} dt',i = 1,2, \ldots , \end{aligned}$$

and using the identity [37]

$$\begin{aligned} < {\varGamma _m}(t){\varGamma _n}(t) > = {D^2}\frac{{{t^{m + n + 1}}}}{{m!n!(m + n + 1)}}, \end{aligned}$$

we can expand Eq. (26) about ${z_0}$ to ${(\Delta t)^2}$ . Then, we have

$$\begin{aligned} z(\Delta t)= & {} {z_0} + {f_0}\Delta t + \frac{1}{2}{f_0}{f'_0}{(\Delta t)^2} + \frac{1}{2}{\xi _0}{f'_0}{(\Delta t)^2}\\&+\, {\xi _0}\Delta t - \frac{1}{2}\gamma {\xi _0}{(\Delta t)^2} + {S_z},\\ \xi (\Delta t)= & {} {\xi _0} - \gamma {\xi _0}\Delta t + \frac{1}{2}{\gamma ^2}{\xi _0}{(\Delta t)^2} + {S_\xi }, \end{aligned}$$

in which ${f_0} = f({z_0})$, and

$$\begin{aligned} {S_z}= & {} - {\varGamma _0}(\Delta t) + \frac{1}{2}{f''_0}\int _0^{\Delta t} {dt'} \varGamma _0^2(t') \\&+\, \gamma {\varGamma _1}(\Delta t) - {f'_0}{\varGamma _1}(\Delta t),\\ {S_\xi }= & {} \gamma {\varGamma _0}(\Delta t) - {\gamma ^2}{\varGamma _1}(\Delta t). \end{aligned}$$

Then, we get the mean and variance of ${S_z}$ and ${S_\xi }$ to the order of ${(\Delta t)^2}$ :

$$\begin{aligned}< {S_z}>= & {} \frac{{{D^2}}}{4}{f''_0}{(\Delta t)^2},\nonumber \\< S_z^2>= & {} {D^2}\Delta t - {D^2}\gamma {(\Delta t)^2} + {D^2}{f'_0}{(\Delta t)^2},\nonumber \\< {S_\xi }>= & {} 0,\nonumber \\ < S_\xi ^2 >= & {} {D^2}{\gamma ^2}\Delta t - {D^2}{\gamma ^3}{(\Delta t)^2}. \end{aligned}$$

(27)

Parallelly, starting directly from Eq. (7) by using a second-order Runge–Kutta algorithm, we have

$$\begin{aligned} z(\Delta t)= & {} {z_0} + \frac{1}{2}({F_1} + {F_2})\Delta t - D\sqrt{\Delta t} {\phi _0},\nonumber \\ \xi (\Delta t)= & {} {\xi _0} + \frac{1}{2}({H_1} + {H_2})\Delta t + D\gamma \sqrt{\Delta t} {\phi _0}, \end{aligned}$$

(28)

in which

$$\begin{aligned} {H_1}= & {} - \gamma \left( {\xi _0} + D\gamma \sqrt{\Delta t} {\phi _1}\right) ,\\ {H_2}= & {} - \gamma \left( {\xi _0} + \Delta t{H_1} + D\gamma \sqrt{\Delta t} {\phi _2}\right) ,\\ {F_1}= & {} f\left( {z_0} - D\sqrt{\Delta t} {\phi _1}\right) + {\xi _0} + D\gamma \sqrt{\Delta t} {\phi _1},\\ {F_2}= & {} f\left( {z_0} + \Delta t{F_1} - D\sqrt{\Delta t} {\phi _2}\right) + {\xi _0} + \Delta t{H_1} \\&+\,D\gamma \sqrt{\Delta t} {\phi _2}, \end{aligned}$$

and ${\phi _0}$, ${\phi _1}$, and ${\phi _2}$ are three independent standard Gaussian random numbers, each of which has zero mean and unit variance. Expanding Eq. (28) about ${z_0}$ to order ${(\Delta t)^2}$, we obtain

$$\begin{aligned} z(\Delta t)= & {} {z_0} + {f_0}\Delta t + \frac{1}{2}{f_0}{f'_0}{(\Delta t)^2} + \frac{1}{2}{f'_0}{\xi _0}{(\Delta t)^2}\\&+ {\xi _0}\Delta t - \frac{1}{2}\gamma {\xi _0}{(\Delta t)^2} + {S'_z},\\ \xi (\Delta t)= & {} {\xi _0} - \gamma {\xi _0}\Delta t + \frac{1}{2}{\gamma ^2}{\xi _0}{(\Delta t)^2} + {S'_\xi }, \end{aligned}$$

where

$$\begin{aligned} {S'_z}= & {} - D\sqrt{\Delta t} {\phi _0} - \frac{1}{2}D({f'_0} - \gamma ){(\sqrt{\Delta t} )^3}{\phi _1} \\&+\frac{{{D^2}}}{4}{f''_0}{(\Delta t)^2}\phi _1^2 \\&- \frac{1}{2}D({f'_0} - \gamma ){(\sqrt{\Delta t} )^3}{\phi _2} + \frac{{{D^2}}}{4}{f''_0}{(\Delta t)^2}\phi _2^2,\\ {S'_\xi }= & {} D\gamma \sqrt{\Delta t} {\phi _0} - \frac{1}{2}D{\gamma ^2}{(\sqrt{\Delta t} )^3}{\phi _1} \\&- \frac{1}{2}D{\gamma ^2}{(\sqrt{\Delta t} )^3}{\phi _2}. \end{aligned}$$

The means and the variances of ${S'_z}$ and ${S'_\xi }$ are calculated to be

$$\begin{aligned}< {S'_z}>= & {} \frac{{{D^2}}}{4}{f''_0}{(\Delta t)^2}< \phi _1^2> \nonumber \\&+ \frac{{{D^2}}}{4}{f''_0}{(\Delta t)^2}< \phi _2^2> ,\nonumber \\< {S_z'^2}>= & {} {D^2}\Delta t< \phi _0^2> \nonumber \\&+ {D^2}({f'_0} - \gamma ){(\Delta t)^2}< {\phi _0}({\phi _1} + {\phi _2})> ,\nonumber \\< {S'_\xi }>= & {} 0,\nonumber \\< {S_\xi '^2}>= & {} {D^2}{\gamma ^2}\Delta t< \phi _0^2> \nonumber \\&- {D^2}{\gamma ^3}{(\Delta t)^2} < {\phi _0}({\phi _1} + {\phi _2}) > . \end{aligned}$$

(29)

Equating Eqs. (27)–(29) leads to

$$\begin{aligned}&< \phi _0^2> = 1,\\&< \phi _1^2> +< \phi _2^2> = 1,\\&< {\phi _0}({\phi _1} + {\phi _2}) > = 1. \end{aligned}$$

Due to the reason that there are three equations and three unknowns, it is possible for us to define a standard Gaussian random number $\psi$ so that ${\phi _i} = {a_i}\psi ,i = 0,1,2$. To maintain the structure, we can conveniently choose ${a_0} = {a_2} = 1$ and ${a_1} = 0$. Then, these considerations lead to the second-order stochastic Runge–Kutta algorithm as represented by Eq. (10).