Size-dependent elastic interaction of screw dislocations with semi-infinite coating materials revealed by atomistic simulation and two dimensional analysis

Abstract

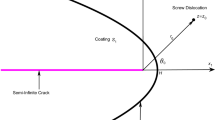
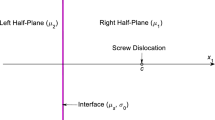
The aim of this work is to study the size-dependent elastic interaction of a screw dislocation with an elastic isotropic layered semi-infinite matrix using molecular dynamics analysis. The analytic solutions of complex functions of a screw dislocation in the matrix and in the thin layer are solved by means of applying complex potential approach in conjunction with the techniques of the image method. With the aid of the obtained stress fields and the Peach–Koehler formula, the explicit expressions for the image force acting on the screw dislocation are obtained. The results indicate that a large change in distance between dislocation and interface leads to a slight change in potential energy and stress. The material elastic dissimilarity, the thin layer thickness and the dislocation position play a crucial role in the image force acting on the dislocation. When a screw dislocation is in the matrix or in the layer, the image force approaches a constant value with the increasing thickness of thin layer. In addition, when the matrix is softer than the layer and the dislocation is in the matrix, there is a stable equilibrium position for dislocation; while, when the matrix is softer than the layer and the dislocation is in the layer, there exists a non-stable equilibrium position for dislocation.

Appendix 1

When the screw dislocation is in the thin layer, the explicit expressions for the image force acting on the screw dislocation can be obtained. As shown in Fig. 6b, a screw dislocation is fixed at the distance d away from the surface, and the global coordinate is setted as originating at a screw dislocation. We also only need to study the image force of the y-direction force variation when a screw dislocation motives along the direction parallel with the y-axis. In addition, we can change the distance d to study a variation of the image force ($z_{*} = 0$). The task now is to determine the complex potential in the layer under the boundary conditions described by Eqs. (1), (2) and (3).

Based on the work of Yang and Xu [14], by means of the image method, two series of infinite image points are produced from the screw dislocation point due to the surface and the interface. The first series of image points are illustrated in Fig. 12a, an image point is first produced by the interface and then reflected by the free surface, and so on. The image points reflected by the interface are denoted by $O_{i}$, and the corresponding local coordinate is $z_{k}$. The image points reflected by the free surface are denoted by $C_{i}$, and the corresponding local coordinate is $\zeta_{k}$. The relationships between the local coordinates and the global coordinates are

$$ \left\{ {\begin{array}{*{20}l} {z_{k} = z - \left( {2kh - d} \right){\text{i}}} \hfill \\ {\zeta_{k} = z + \left[ {2h\left( {k - 1} \right) - d} \right]{\text{i}}} \hfill \\ \end{array} } \right. $$

(22a)

Fig. 12

First series of image points in (a), and second series of image points in (b) for dislocation in the thin layer

The second series of infinite image points are shown in Fig. 12b. First, an image point is produced by the free surface and then reflected by the interface, and so on. The image points reflected by the interface are denoted by $A_{i}$, and the corresponding local coordinate is $\eta_{k}$. The image points reflected by the free surface are denoted by $B_{i}$, and the corresponding local coordinate is $\xi_{k}$. The relationships between the local coordinates and the global coordinates are

$$ \left\{ {\begin{array}{*{20}l} {\xi_{k} = z + \left( {2hk + d} \right){\text{i}}} \hfill \\ {\eta_{k} = z + \left( {2hk - 2d} \right){\text{i}}} \hfill \\ \end{array} } \right. $$

(22b)

When a screw dislocation with Burger’s vector $b_{z}$ is located at an arbitrary point $z_{*}$ in the layer, the complex potentials can take the following forms [16]

$$ f_{1} = f_{\rm I} (z_{0} ) + \sum\limits_{k = 1}^{\infty } {\left[ {a_{k} \left( {z_{k} } \right) + b_{k} \left( {\zeta_{k} } \right) + c_{k} \left( {\xi_{k} } \right) + d_{k} \left( {\eta_{k} } \right)} \right]} ,\quad z \in \,\,\,{\text{the layer}} $$

(23)

$$ f_{2} = f_{\Pi } (z_{0} ) + \sum\limits_{k = 1}^{\infty } {\left[ {f_{k} \left( {z_{k} } \right) + g_{k} \left( {\eta_{k} } \right)} \right]} ,\quad z \in {\text{the matrix}} $$

(24)

where the complex potential $f_{\rm I} (z_{0} ) = \gamma \ln \left( {z - z_{*} } \right)$, γ = b _z /(2πi) and $z_{0} = z - z_{*}$. The complex potentials $f_{\Pi } (z)$, $a_{k} \left( {z_{k} } \right)$, $b_{k} \left( {\zeta_{k} } \right)$, $c_{k} \left( {\xi_{k} } \right)$, $d_{k} \left( {\eta_{k} } \right)$, $f_{k} \left( {z_{k} } \right)$ and $g_{k} \left( {\eta_{k} } \right)$ are holomorphic in the regions where they are defined, respectively.

First, the continuity conditions should be satisfied along the interface. It is noted that at the interface one has $z_{k} = \overline{{\zeta_{k} }}$ and $\xi_{k + 1} = \overline{{\eta_{k} }}$. Substituting the stress functions expressed in Eqs. (23) and (24) into formula expressed in Eqs. (4) and (5) to obtain the stresses and the displacements, then into the continuity conditions of Eqs. (1) and (2), at the mean time considering the corresponding relationships of the image points, it yields

$$ \left\{ {\begin{array}{*{20}l} {\overline{{f_{\rm I}^{{\prime }} (z_{0} )}} + \overline{{c_{1}^{{\prime }} \left( {\xi_{1} } \right)}} - f_{\rm I}^{{\prime }} (z_{0} ) - c_{1}^{{\prime }} \left( {\xi_{1} } \right) =\Gamma _{2} \left[ {\overline{{f_{\Pi }^{\prime } (z_{0} )}} - f_{\Pi }^{\prime } (z_{0} )} \right]} \hfill \\ {\overline{{a_{k}^{{\prime }} \left( {z_{k} } \right)}} + \overline{{b_{k}^{{\prime }} \left( {\zeta_{k} } \right)}} - a_{k}^{{\prime }} \left( {z_{k} } \right) - b_{k}^{{\prime }} \left( {\zeta_{k} } \right) =\Gamma _{2} \left[ {\overline{{f_{k}^{{\prime }} \left( {z_{k} } \right)}} - f_{k}^{{\prime }} \left( {z_{k} } \right)} \right]} \hfill \\ {\overline{{c_{k + 1}^{{\prime }} \left( {\xi_{k + 1} } \right)}} + \overline{{d_{k}^{{\prime }} \left( {\eta_{k} } \right)}} - c_{k + 1}^{{\prime }} \left( {\xi_{k + 1} } \right) - d_{k}^{{\prime }} \left( {\eta_{k} } \right) =\Gamma _{2} \left[ {\overline{{g_{k}^{{\prime }} \left( {\eta_{k} } \right)}} - g_{k}^{{\prime }} \left( {\eta_{k} } \right)} \right]} \hfill \\ {f_{\rm I} (z_{0} ) + \overline{{f_{\rm I} (z_{0} )}} + c_{1} \left( {\xi_{1} } \right) + \overline{{c_{1} \left( {\xi_{1} } \right)}} = f_{\Pi } (z_{0} ) + \overline{{f_{\Pi } (z_{0} )}} } \hfill \\ {a_{k} \left( {z_{k} } \right) + \overline{{a_{k} \left( {z_{k} } \right)}} - f_{k} \left( {z_{k} } \right) - \overline{{f_{k} \left( {z_{k} } \right)}} = - b_{k} \left( {\zeta_{k} } \right) - \overline{{b_{k} \left( {\zeta_{k} } \right)}} } \hfill \\ {c_{k + 1} \left( {\xi_{k + 1} } \right) + \overline{{c_{k + 1} \left( {\xi_{k + 1} } \right)}} = g_{k} \left( {\eta_{k} } \right) + \overline{{g_{k} \left( {\eta_{k} } \right)}} - d_{k} \left( {\eta_{k} } \right) - \overline{{d_{k} \left( {\eta_{k} } \right)}} } \hfill \\ \end{array} } \right. $$

(25)

where the shear modulus ratio $\Gamma _{2} = \mu_{2} /\mu_{1}$.

Based on interchange theorem, at the interface, we have

$$ \left\{ {\begin{array}{*{20}l} {\frac{\partial L}{{\partial z_{k} }} = - \frac{\partial R}{{\partial \overline{{\zeta_{k} }} }},\int {Ldz_{k} } = - \int {Rd\overline{{\zeta_{k} }} } } \hfill \\ {\frac{\partial L}{{\partial \xi_{k + 1} }} = - \frac{\partial R}{{\partial \overline{{\eta_{k} }} }},\int {Ld\xi_{k + 1} } = - \int {Rd\overline{{\eta_{k} }} } } \hfill \\ {\frac{\partial L}{{\partial \xi_{1} }} = - \frac{\partial R}{{\partial \overline{{z_{0} }} }},\int {Ld\xi_{1} } = - \int {Rd\overline{{z_{0} }} } } \hfill \\ \end{array} } \right. $$

(26)

where L and R represent the left- and right-hand side of an equation like $L(x,y) = R(x,y)$, respectively. Using Eq. (26), from Eq. (25) we can obtain

$$ \left\{ {\begin{array}{*{20}l} {c_{1} (\xi_{1} ) = \overline{{f_{\rm I} (z_{0} )}} - \Gamma_{2} \overline{{f_{\Pi } (z_{0} )}} } \hfill \\ {f_{\Pi } (z_{0} ) = \frac{2}{{1 + \Gamma_{2} }}f_{\rm I} (z_{0} )} \hfill \\ {f_{k} (z_{k} ) = \frac{2}{{1 + \Gamma_{2} }}a_{k} (z_{k} )} \hfill \\ {b_{k} (\zeta_{k} ) = \overline{{a_{k} (z_{k} )}} - \Gamma_{2} \overline{{f_{k} (z_{k} )}} } \hfill \\ {g_{k} (\eta_{k} ) = \frac{2}{{1 + \Gamma_{2} }}d_{k} (\eta_{k} )} \hfill \\ {c_{k + 1} (\xi_{k + 1} ) = \overline{{g_{k} (\eta_{k} )}} - \Gamma_{2} \overline{{d_{k} (\eta_{k} )}} } \hfill \\ \end{array} } \right. $$

(27)

Second, at the free surface $z_{k + 1} = \overline{{\zeta_{k} }}$ and $\xi_{k} = \overline{{\eta_{k} }}$.

$$ \left\{ {\begin{array}{*{20}l} {f_{\rm I}^{{\prime }} (z_{0} ) + a_{1}^{{\prime }} \left( {z_{1} } \right) + \overline{{f_{\rm I}^{{\prime }} (z_{0} )}} + \overline{{a_{1}^{{\prime }} \left( {z_{1} } \right)}} = 0} \hfill \\ {a_{k + 1}^{{\prime }} \left( {z_{k + 1} } \right) + b_{k}^{{\prime }} \left( {\zeta_{k} } \right) + \overline{{a_{k + 1}^{{\prime }} \left( {z_{k + 1} } \right)}} + \overline{{b_{k}^{{\prime }} \left( {\zeta_{k} } \right)}} = 0} \hfill \\ {c_{k}^{{\prime }} \left( {\xi_{k} } \right) + d_{k}^{{\prime }} \left( {\eta_{k} } \right) + \overline{{c_{k}^{{\prime }} \left( {\xi_{k} } \right)}} + \overline{{d_{k}^{{\prime }} \left( {\eta_{k} } \right)}} = 0} \hfill \\ \end{array} } \right. $$

(28)

Based on interchange theorem, at the interface, we have

$$ \left\{ {\begin{array}{*{20}l} {\frac{\partial L}{{\partial z_{k + 1} }} = \frac{\partial R}{{\partial \overline{{\zeta_{k} }} }},\int {Ldz_{k + 1} } = \int {Rd\overline{{\zeta_{k} }} } } \hfill \\ {\frac{\partial L}{{\partial \xi_{k} }} = \frac{\partial R}{{\partial \overline{{\eta_{k} }} }},\int {Ld\xi_{k} } = \int {Rd\overline{{\eta_{k} }} } } \hfill \\ {\frac{\partial L}{{\partial z_{1} }} = \frac{\partial R}{{\partial \overline{{z_{0} }} }},\int {Ldz_{1} } = \int {Rd\overline{{z_{0} }} } } \hfill \\ \end{array} } \right. $$

(29)

Using Eq. (29), from Eq. (28) we can obtain

$$ \left\{ {\begin{array}{*{20}l} {a_{1} \left( {z_{1} } \right) = \overline{{f_{\rm I} (z_{0} )}} } \hfill \\ {a_{k + 1} \left( {z_{k + 1} } \right) = \overline{{b_{k} \left( {\zeta_{k} } \right)}} } \hfill \\ {d_{k} \left( {\eta_{k} } \right) = \overline{{c_{k} \left( {\xi_{k} } \right)}} } \hfill \\ \end{array} } \right. $$

(30)

According to Eqs. (23), (27) and (30), the displacement boundary conditions and the stress boundary conditions should be satisfied along the interface and the free surface, the complex potential function can be expressed as

$$ \begin{aligned} f_{1} & = \frac{{b_{z} }}{2\pi i}\ln \left( {z - z_{*} } \right) + \overline{\gamma } \ln \left( {z - 2di} \right) + \frac{{1 - \Gamma_{2} }}{{1 + \Gamma_{2} }}\overline{\gamma } \ln \left[ {z + \left( {2h - 2d} \right)i} \right] + \frac{{1 - \Gamma_{2} }}{{1 + \Gamma_{2} }}\gamma \ln \left( {z - 2hi} \right) \\ & \quad + \sum\limits_{k = 2}^{\infty } {\frac{{\left( {1 - \Gamma_{2} } \right)^{k - 2} }}{{\left( {1 + \Gamma_{2} } \right)^{k - 2} }}\overline{\gamma } \ln \left\{ {z - \left[ {2d + 2h(k - 1)} \right]i} \right\}} + \sum\limits_{k = 1}^{\infty } {\frac{{\left( {1 - \Gamma_{2} } \right)^{k - 1} }}{{\left( {1 + \Gamma_{2} } \right)^{k - 1} }}\gamma \ln \left( {z + 2hki} \right)} \\ & \quad + \sum\limits_{k = 2}^{\infty } {\frac{{2^{k - 1} \left( {1 - \Gamma_{2} } \right)^{k} }}{{\left( {1 + \Gamma_{2} } \right)^{2k - 1} }}\gamma \ln \left[ {z + \left( {2hk - 2d} \right)i} \right]} + \sum\limits_{k = 2}^{\infty } {\frac{{2^{k - 1} \left( {1 - \Gamma_{2} } \right)^{k} }}{{\left( {1 + \Gamma_{2} } \right)^{2k - 1} }}\overline{\gamma } \ln \left( {z - 2hki} \right)\quad z \in \,\,\,{\text{the film}}} \\ \end{aligned} $$

(31)