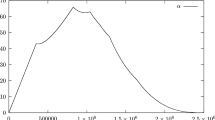
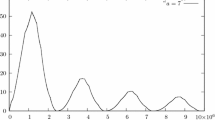
Abstract
We prove that the “quadratic irrational rotation” exhibits a central limit theorem. More precisely, let α be an arbitrary real root of a quadratic equation with integer coefficients; say, α = \( \sqrt 2 \). Given any rational number 0 < x < 1 (say, x = 1/2) and any positive integer n, we count the number of elements of the sequence α, 2α, 3α, …, nα modulo 1 that fall into the subinterval [0, x]. We prove that this counting number satisfies a central limit theorem in the following sense. First, we subtract the “expected number” nx from the counting number, and study the typical fluctuation of this difference as n runs in a long interval 1 ≤ n ≤ N. Depending on α and x, we may need an extra additive correction of constant times logarithm of N; furthermore, what we always need is a multiplicative correction: division by (another) constant times square root of logarithm of N. If N is large, the distribution of this renormalized counting number, as n runs in 1 ≤ n ≤ N, is very close to the standard normal distribution (bell shaped curve), and the corresponding error term tends to zero as N tends to infinity. This is the main result of the paper (see Theorem 1.1). The proof is rather complicated and long; it has many interesting detours and byproducts. For example, the exact determination of the key constant factors (in the additive and multiplicative norming), which depend on α and x, requires surprisingly deep algebraic tools such as Dedeking sums, the class number of quadratic fields, and generalized class number formulas. The crucial property of a quadratic irrational is the periodicity of its continued fraction. Periodicity means self-similarity, which leads us to Markov chains: our basic probabilistic tool to prove the central limit theorem. We also use a lot of Fourier analysis. Finally, I just mention one byproduct of this research: we solve an old problem of Hardy and Littlewood on diophantine sums.
The whole paper consists of an introduction and 17 sections. Part 1 contains the Introduction and Sections 1–7.
Similar content being viewed by others
References
T. van Aardenne-Ehrenfest, Proof of the impossibility of a just distribution of an infinite sequence of points over an interval, Proc. Kon. Ned. Akad. v. Wetensch., 48 (1945), 266–271.
T. van Aardenne-Ehrenfest, On the impossibility of a just distribution, Proc. Kon. Ned. Akad. v. Wetensch., 52 (1949), 734–739.
J. Beck, Randomness of n\( \sqrt 2 \) mod 1 and a Ramsey property of the hyperbola, Sets, Graphs and Numbers, Colloq. Math. Soc. János Bolyai 60, Budapest, 1992, 23–66.
J. Beck, From probabilistic diophantine approximation to quadratic fields, Random and Quasi-Random Point Sets, Lecture Notes in Statistics 138, Springer-Verlag, New York, 1998, 1–49.
J. Beck, Randomness in lattice point problems, Discrete Math., 229 (2001), 29–45.
J. Beck, Lattice point problems: crossroads of number theory, probability theory, and Fourier analysis, Fourier Analysis and Convexity (eds. L. A. Brandolini et al.), Birkhauser, Boston, 2004, 1–35.
J. Beck and W. W. L. Chen, Irregularities of Distribution, Cambridge Tracts in Mathematics 89, Cambridge University Press, Cambridge, 1987.
B. Chazelle, The Discrepancy Method, Cambridge University Press, Cambridge, 2000.
J. G. van der Corput, Verteilungsfunktionen. I and II., Proc. Kon. Ned. Akad. v. Wetensch., 38 (1935), 813–821 and 1058–1066.
H. Davenport, Note on irregularities of distribution, Mathematika, 3 (1956), 131–135.
U. Dieter, Das Verhaltender Kleinschen Functionen gegenüber Modultransformationen und verallgemeinerte Dedekindsche Summen, J. Reine Angew. Math., 201 (1959), 37–70.
Y. Dupain, Discrépance de la suite, Ann. Inst. Fourier (Grenoble), 29 (1979), 81–106.
Y. Dupain and Vera T. Sós, On the discrepancy of nα sequences, Topics in classical number theory, Colloquium, Budapest 1981, vol. 1, Colloq. Math. Soc. János Bolyai 34, 1984, 355–387.
W. Feller, An Introduction to Probability Theory and its Applications, Vol. 1 (3rd edn), Wiley, New York, 1969.
W. Feller, An Introduction to Probability Theory and its Applications, Vol. 2 (2nd edn), Wiley, New York, 1971.
G. H. Hardy and J. Littlewood, The lattice-points of a right-angled triangle. I, Proc. London Math. Soc., 3 (1920), 15–36.
G. H. Hardy and J. Littlewood, The lattice-points of a right-angled triangle. II, Abh. Math. Sem. Hamburg, 1 (1922), 212–249.
G. H. Hardy and J. Littlewood, Some problems of Diophantine approximation: A series of cosecants, Bull. Calcutta Math. Soc., 20 (1930), 251–266.
G. H. Hardy and E. M. Wright, An introduction to the theory of numbers, 5th edition, Clarendon Press, Oxford, 1979.
A. Khintchine, Continued Fractions, English translation, P. Noordhoff, Groningen, The Netherlands, 1963.
D. E. Knuth, Notes on generalized Dedekind sums, Acta Arithmetica, 33 (1977), 297–325.
S. Lang, Introduction to Diophantine Approximations, Addison-Wesley, 1966.
J. Matousek, Geometric Discrepancy, Algorithms and Combinatorics 18, Springer-Verlag, Berlin, 1999.
A. Ostrowski, Bemerkungen zur Theorie der Diophantischen Approximationen. I., Abh. Hamburg Sem., 1 (1922), 77–99.
H. Rademacher and E. Grosswald, Dedekind Sums, Carus Monograph No. 16, Math. Assoc. Amer., 1972.
K. F. Roth, Irregularities of distribution, Mathematika, 1 (1954), 73–79.
W. M. Schmidt, Irregularities of distribution. VII, Acta Arithmetica, 21 (1972), 45–50.
J. Schoissengeier, Another proof of a theorem of Beck, Monatsh. Math., 129 (2000), 147–151.
Vera T. Sós, On the distribution mod 1 of the sequence {nα}, Ann. Univ. Sci. Budapest, 1 (1958), 127–234.
Vera T. Sós and S. K. Zaremba, The mean-square discrepancies of some two-dimensional lattices, Studia Sci. Math. Hungarica, 14 (1979), 255–271.
S. Swierczkowski, On successive settings of an arc on the circumeference of a circle, Fund. Math., 46 (1958), 187–189.
H. Weyl, Über die Gleichverteilung von Zahlen mod Eins, Math. Ann., 77 (1916), 313–352.
D. B. Zagier, Nombres de classes at fractions continues, J. Arithmetiques de Bordeaux, Asterisque, 24–25 (1975), 81–97.
D. B. Zagier, Zeta-funktionen und quadratische Körper, Hochschultext, Springer, 1981.
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by Attila Pethő
Rights and permissions
About this article
Cite this article
Beck, J. Randomness of the square root of 2 and the Giant Leap, Part 1. Period Math Hung 60, 137–242 (2010). https://doi.org/10.1007/s10998-010-2137-9
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10998-010-2137-9