Abstract
The Poisson-Boltzmann equation for a strongly charged plate inside a generic charge-asymmetric electrolyte is solved using the method of asymptotic matching. Both near field and far field asymptotic behaviors of the potential are systematically analyzed. Using these expansions, the renormalized surface charge density is obtained as an asymptotic series in terms of the bare surface charge density.



Similar content being viewed by others
Notes
We will use SI units in this work.
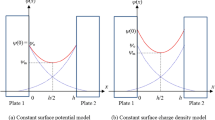
In principle, one can also study plate with fixed surface potential, or with more general linear relation between surface charge density and surface potential. It has been debated in literatures which is the most appropriate boundary conditions for charged surfaces. For an isolated plate, however, there is no difference of physical significance between different boundary conditions: They are all physically equivalent to each other.
Our definition of Gouy-Chapman length differs from that in other literatures by a factor of two.
Even though the potential Ψ(z), and hence also ϒ(s)=e Ψ(z) as well, is finite for all s∈(0,1), their Taylor expansions around s=0 may diverge.
We have also tried to use the difference between the current order and the second nearest order approximations. The resulting error bounds only change slightly from what we discuss below.
For a more realistic primitive model, there are more length scales: the diameters of counter-ions and co-ions.
References
Andelman, D.: Electrostatic Properties of Membranes: The Poisson-Boltzmann Theory (Chap. 12). Structure and Dynamics of Membranes Generic and Specific Interactions. Elsevier, Amsterdam (1995)
Levin, Y.: Electrostatic correlations: from plasma to biology. Rep. Prog. Phys. 65(11), 1577–1632 (2002)
Grosberg, A.Yu., Nguyen, T.T., Shklovskii, B.I.: Colloquium: the physics of charge inversion in chemical and biological systems. Rev. Mod. Phys. 74(2), 329–345 (2002)
Boroudjerdi, H., Kim, Y.-W., Naji, A., Netz, R.R., Schlagberger, X., Serr, A.: Statics and dynamics of strongly charged soft matter. Phys. Rep. 416(3–4), 129–199 (2005)
Grahame, D.C.: Diffuse double layer theory for electrolytes of unsymmetrical valence types. J. Chem. Phys. 21(6), 1054–1060 (1953)
Andrietti, F., Peres, A., Pezzotta, R.: Exact solution of the unidimensional Poisson-Boltzmann equation for a 1:2 (2:1) electrolyte. Biophys. J. 16(9), 1121–1124 (1976)
Tracy, C.A., Widom, H.: On exact solutions to the cylindrical Poisson-Boltzmann equation with application to polyelectrolytes. Physica A 244, 402–413 (1997)
Téllez, G., Trizac, E.: Nonlinear screening of spherical and cylindrical colloids: the case of 1:2 and 2:1 electrolytes. Phys. Rev. E 70(1), 11404 (2004)
Téllez, G., Trizac, E.: Exact asymptotic expansions for the cylindrical Poisson–Boltzmann equation. J. Stat. Mech. Theory Exp. 2006(06), P06018 (2006)
Téllez, G.: Nonlinear screening of charged macromolecules. Philos. Trans. R. Soc., Math. Phys. Eng. Sci. 369(1935), 322–334 (2011)
Xing, X.: Poisson-Boltzmann theory for two parallel uniformly charged plates. Phys. Rev. E 83(4), 041410 (2011)
Trizac, E., Bocquet, L., Aubouy, M., von Grünberg, H.H.: Alexander’s prescription for colloidal charge renormalization. Langmuir 19(9), 4027–4033 (2003)
Trizac, E., Bocquet, L., Aubouy, M.: Simple approach for charge renormalization in highly charged macroions. Phys. Rev. Lett. 89(24), 248301 (2002)
Belloni, L.: A hypernetted chain study of highly asymmetrical polyelectrolytes. Chem. Phys. 99(1), 43–54 (1985)
Palberg, T., Mönch, W., Bitzer, F., Piazza, R., Bellini, T.: Freezing transition for colloids with adjustable charge: a test of charge renormalization. Phys. Rev. Lett. 74(22), 4555–4558 (1995)
Belloni, L.: Colloids Surf. A 140, 227 (1998)
Alexander, S., Chaikin, P.M., Grant, P., Morales, G.J., Pincus, P., Hone, D.: Charge renormalization, osmotic pressure, and bulk modulus of colloidal crystals: theory. J. Chem. Phys. 80(11), 5776–5781 (1984)
Borukhov, I., Andelman, D., Orland, H.: Steric effects in electrolytes: a modified Poisson-Boltzmann equation. Phys. Rev. Lett. 79(3), 435–438 (1997)
Zhou, S., Wang, Z., Li, B.: Mean-field description of ionic size effects with nonuniform ionic sizes: a numerical approach. Phys. Rev. E 84(2), 021901 (2011)
Bender, C.M., Orszag, S.A.: Advanced Mathematical Methods for Scientists and Engineers. Springer, Berlin (1999)
Acknowledgements
The authors thank NSFC (grant numbers 11174196, 91130012) for financial support.
Author information
Authors and Affiliations
Corresponding author
Appendices
Appendix A: Coefficients of Near Field Expansions
In Table 5, we list nine lowest order coefficients in the near field expansion of the potential. The form of expansion is displayed in Table 1.
Appendix B: Basics of Shanks Transform
In this appendix, we present a heuristic discussion of Shanks transform. The readers are referred to the classical monograph by Bender and Orszag [20] for details. Originally Shanks transformation was invented to speed up convergence of slowly converging series. Its most powerful application is however to sum up divergent series and yield finite result. For simple geometric series, Shanks transformation is equivalent to the traditional procedure, where one first sum up the series within its radius of convergence, and then analytically continuate beyond this domain.
Consider a simple geometric series
which is convergent for |z|<z 1. Within this domain of convergence, the series can be summed up to yield a simple rational function with one simple pole at z=z 1:
Let us define the remainder R n as
which is the error if we use the partial sum A n to approximate the original series. For the simple geometric series (41) the remainder has the following simple form
which is also geometric in n. The remainder goes to zero as long as the original series converges.
Suppose we are interested in the domain |z|>1, i.e. outside the radius of convergence of A, and suppose that for some reason, we are only able to calculate the partial sums \(A_{n} = \sum_{0}^{n} a\, z^{k}\) only up to some finite order n. The conventional approach fails here because we can not sum up the series to infinite order for small z. On the other hand, the partial sum A n is not a good approximation to the exact because the error (i.e. the remainder R n ) diverges in the domain of interest. So the question is how we can obtain a good approximation to the series outside its domain of convergence, with the knowledge of only finite terms of the series?
For the geometric series (41), we have the following relations for arbitrary integer n:



Solving these three equations, we can express A in terms of three parameters A n+1,A n ,A n−1 (details ignored here):

where ΔA n =A n+1−A n . Therefore knowing that the series is geometric, we only need three terms of partial sums to obtain the exact sum of the series.
The Shanks transformation is a nonlinear transformation acting on the partial sums S 1(A n ) (the subscript 1 means it is the first order Shanks transformation).
Note however, in computing S 1(A n ), we actually need three partial sums A n+1, A n , and A n−1. Strictly speaking, therefore, Shanks transformation acts on the whole sequence of partial sums, and return a new sequence of partial sums. It is defined such that it returns the exact A if the original series is geometric.
The utility of Shanks transformation can be best understood by looking at its effect on the remainder. Let A n =A−R n in the above equation, where R n is the remainder of the original series. Further let \(R^{S}_{n}\) be the remainder of the Shanks transformed series:
We easily see the following
If the original series is geometric, then R n has the form of Eq. (44), and \(R^{S}_{n}\) simply vanishes, indicating that the transformed partial sum is actually the exact result.
For a more general series, let us assume that we can separate the dominant part of the remainder which is again geometric:
where \(\tilde{R}_{n} /z^{n} \rightarrow0\) as n→∞. Our analysis below applies regardless of the convergence of the remainders R n , \(\tilde{R}_{n}\). Using Eq. (51), the Shanks transformed remainder then becomes:

In the limit n→∞, therefore, the dominant part z n no longer appear in the transformed version of the remainder. As a consequence the transformed remainder becomes much smaller than the original remainder. In particular, if z>1 but \(\tilde{R}_{n} \rightarrow0\), the original series diverges R n →∞ but the Shanks transformed series converges, i.e. \(R^{S}_{n} \rightarrow 0\). This shows how Shanks transformation can be used to achieve the purpose of analytic continuation with only finite number of terms of the series. Note that for the case we studied here, the summation (42) contains a simple pole at z=1. The effect of Shanks transformation can also be understood as removing this singularity from the original series. It is easy to see that the Shanks transformation recovers the exacts from Taylor series of all rational functions with only one pole, in the form of P(z)/(z−z 0), where P(z) is a polynomial of arbitrary (but finite) order.
The first order Shanks transformation discussed above does not work if the remainders contain second order poles. To deal with these series, we have to introduce second order Shanks transformation, which removes two poles at once. In this case, it can be shown that the exact sum A can be calculated using five terms of the partial sums: A n+2, A n+1, A n , A n−1, A n−2:
For a general series, the second order Shanks transformation is then defined as

This second order transformation returns the exacts when applying to the Taylor expansions of rational functions with two poles.
Appendix C: Estimation of Error δc 1
To estimate the error of c 1, we note that if we use the exact near field and far field expansions in Eq. (36), we would have found the exact value for the coefficient \(c_{1}^{\mathrm{ex}}\), independent of the matching point z ∗. Mathematically we have:

where the superscript ex stands for “exact”. By contrast, because of the approximate nature of the expansions we use, the coefficient c 1 we find is different from its exact value. Let \(c_{1} = c_{1}^{\mathrm{ex}} + \delta c_{1}\), where δc 1 is the error, we can rewrite Eq. (36) in the following form:
Expanding the left hand side to the linear order of δc 1, we have:

The derivative of \(\varPsi^{\mathrm{app}}_{\mathrm{far}}\) with respect to c 1 can be easily calculated using Mathematica. We further introduce the error of the far field and near field expansions (at the matching point z ∗) as following:


These errors can be estimated by the difference between the approximation at the current order and that at the next order, which can be conveniently calculated using Mathematica. Using Eqs. (56), (58), (59a), (59b), we can estimate the upper bound of the error δc 1 as
Rights and permissions
About this article
Cite this article
Han, M., Xing, X. Renormalized Surface Charge Density for a Strongly Charged Plate in Asymmetric Electrolytes: Exact Asymptotic Expansion in Poisson Boltzmann Theory. J Stat Phys 151, 1121–1139 (2013). https://doi.org/10.1007/s10955-013-0751-7
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10955-013-0751-7