1 Introduction

High T c superconductors (HTSC) are mainly layered copper oxide superconductors with a weak interaction between the superconducting CuO2 planes which leads to strong anisotropy of their properties. For example, the homologous series of the Bi-based superconductors can be expressed by the formula Bi2Sr2Ca n−1Cu n O 4+2n . The groups of the n tightly spaced identical CuO2 planes form the superconducting layers. In the Lawrence-Doniach theory [1], which is often used for theoretical studies of the layered superconductors, the two-dimensional Ginzburg-Landau (GL) equations [2] describe the properties of the superconducting CuO2 layers, and the interaction between them is taken into account by additional Josephson term in the free energy. It will be noted that the Josephson term has little effect on the order parameter in the superconducting layers. Thus, the model describes the Ginzburg-Landau order parameter as two-dimensional function in the CuO2 layers which changes only in the plane of the layers and is zero between the superconducting layers. The gradient term in the free energy along normal to the layers direction is replaced by a finite difference which defined by Josephson term. One of the challenges in high-temperature superconductivity is to understand the properties of the superconducting CuO2 planes that are within a short distance of each other in unit cell and doped with a small concentration of holes. This is confirmed by the experimental fact that in cuprate HTSC, superconductivity persists in ultrathin slabs (one half unit cell thick) and, for example, T c of the similar Bi2Sr2CaCu2 O 8 ultrathin slabs is equal to that of the high T c phase itself [3]. Therefore, the study of the properties of thin superconducting plate in the framework of the GL theory is relevant and important for understanding the properties of HTSC.

Shapiro steps on I-V curves of Josephson junctions made on high T c superconductors [4] and flux quantization experiments in these superconductors [5] demonstrate that superconductivity in these materials involves the pairing of electrons as in conventional Bardeen-Cooper-Schrieffer (BCS) superconductors. It enables to use the results of the microscopic theory of Gor’kov [6] in case of HTSC. But it should be noted, in spite of the wealth of results, we still do not have a consensus on the mechanism of the pairing interaction leading to superconductivity in the cuprates. As shown in the work [6], the GL equations can be obtained by using the microscopic BCS theory [7], the role of the order parameter realizes the pairing potential Δ. In the absence of fields and currents in bulk homogeneous superconductor the pairing potential is equal to the BCS energy gap Δ m .

The boundary conditions turned out to depress the GL order parameter and to decrease T c of the thin layers compared to the critical temperature of the massive superconductor (T cm) [8,9,10,11]. For example, the origin of the effect in the layered HTSCs is the depression of the superconducting order parameter in the tightly spaced superconducting CuO2 planes by the neighboring insulating layers in the unit cell. The critical temperature dependence of the HTSC copper oxide superconductors as a function of the number of identical superconducting CuO2 planes in the unit cell was explained in [12, 13] by using this approach. Thus, the GLG theory is important to study of the HTSCs. Here, we have examined the spectrum of elementary excitations in high T c superconductors taking into account the boundary conditions.

2 Statement of the Problem

Results of numerical study of the effect of the boundary conditions on the GL equations were reported in [11] for superconducting plates in a magnetic field. In the absence of magnetic field, the GL equation describing the order parameter can be written in the form:

$$ \nabla^{2}\psi +\frac{1}{\xi^{2}}\left( {\psi -\psi^{3}} \right)=0 $$
(1)

where ξ is the coherence length. Here, the direction perpendicular to the plane of the superconducting plate is denoted by x, and the plate is located in the region − d/2 ≤ x ≤ d/2. The order parameter in the GL theory is written as Ψ = |Ψ|e i𝜃, where |Ψ| is the module and 𝜃 is the phase of the order parameter. In (1), we use the normalized order parameter ψ = Ψ/Ψ0, where Ψ0 is the equilibrium value of the order parameter in the bulk superconductor without magnetic field. For numerical solution of (1), it is necessary to define boundary conditions. The coherence length ξ(0) = ξ(T = 0) is known to be short in HTSCs. Therefore, the role of the surface member in the expression for the free energy increases in these superconductors. As a result, the boundary condition for the order parameter is shown in [14] to be written in the form:

$$ \partial \psi /\partial x=1/{\Lambda}\psi \vert_{s} $$
(2)

where Λ is a phenomenological parameter, which was called as extrapolation length in [14]. It is shown in [8, 14] that the Λ is defined by the properties of the material to witch the superconductor is bordered. The phenomenological approach used in [14] gives the following relation for Λ:

$$ {\Lambda} =\frac{\xi^{2} (0)T_{\text{cm}}} {l(T_{\text{cm}} -T_{\text{cs}} )}, $$
(3)

where l is a lattice parameter and T cs is the critical temperature of the surface layer, whose thickness is of the order of the lattice constant l.

Microscopic analysis of the extrapolation length was given in the works [8, 9] in the framework of the BCS theory. In case of dirty superconductors, Simonin [9] obtained that

$$ {\Lambda} =\frac{N(0)V_{\text{ep}} \xi^{2} (0)}{a} $$
(4)

where a is the Thomas Fermi screening length, which in metals is of the order of the lattice parameter l, N(0) and V ep are the bulk values of the density of states at the Fermi energy and of electron-phonon interaction energy of the electrons in the Cooper pares. The interaction potential N(0)V ep defines the critical temperature in the BCS theory. For classical low-temperature superconductors (LTSC) N(0)V ep ≤ 0.3. Simonin showed that the relation (4) successfully explains the decrease in the critical temperature with decreasing of their thickness observed in thin films of Nb, Pb and Bi.

It will be noted that the extrapolation length is very long in the LTSC [8], this explains the validity of the commonly used boundary condition in this case:

$$ \partial \psi /\partial x=0\vert_{s} . $$
(5)

The order parameter is equal to Ψ0 on the boundary of the LTSC with a large coherence length ξ(0) [8], and hence, the energy gap in the spectrum of excitations is equal to Δ0 near the boundary. In this case, the extrapolation length aspires to infinity. It provides the ability to measure the energy gap using conventional tunneling methods, in which only the surface layer with thickness of the order of ξ(0) plays a main role.

On the other hand, the order parameter is suppressed at the boundary of the high T c superconductor due to the boundary conditions (2). Moreover, it is suppressed in thin layers containing n tightly spaced identical superconducting CuO2 planes, of which copper oxide superconductors are composed. The pairing potential determined from (1) with boundary conditions (2), will be space-dependent Δ(x), which will behave as ψ(x). In our work, (1) with boundary conditions (2) are solved by numerical methods. The equation was transformed for the purpose as:

$$ (\partial \psi /\partial x)^{2}=\frac{1}{\xi^{2}}\left[ {\psi (0)^{2}-\psi (x)^{2}-0.5(\psi (0)^{4}-\psi (x)^{4})} \right], $$
(6)

and the boundary conditions at x = ±d/2 can be written as:

$$ \psi^{2}(\pm d/2)=1+(\xi /{\Lambda} )^{2}-\left[ {\left( {1+(\xi /{\Lambda} )^{2}} \right)^{2}-2\psi^{2}(0)+\psi^{4}(0)} \right]^{0.5}, $$
(7)

where ψ is the normalized order parameter or the normalized pairing potential ψ = Δ(x)/Δ m . In this case, Δ m is the pairing potential of a hypothetical bulk superconductor consisting only of the superconducting CuO2 planes and the order parameter is not suppressed by neighboring nonsuperconducting layers. The pairing potential accepts this value in the depths of such a superconductor at a great distance from the surface.

The coherence length ξ depends on temperature, so (6) is formally valid at any temperature T, where the order parameter is small ψ << 1. Therefore, we present some results of the calculations for temperatures sufficiently remote from the T cm to analyze experimental results. For this purpose, we use the empirical temperature dependence of ξ(T):

$$ \xi =\frac{\xi (0)}{\sqrt {1-\left( {\frac{T}{T_{\text{cm}}} } \right)^{2}}} . $$
(8)

The dependence is based on the empirical temperature dependence of the upper critical magnetic field:

$$ H_{c2} =H_{c2} (0)\left[ {1-\left( {\frac{T}{T_{\text{cm}}} } \right)^{2}} \right], $$
(9)

which fits well the experimental dependences. As a result, the dependence (8) can be obtained by using the well known expression for the upper critical magnetic field:

$$H_{c2} =\frac{{\Phi}_{0}} {2\pi \xi (T)^{2}} $$

where Φ0 is the magnetic flux quantum. At high temperatures T → T cm, the dependence (5) is close to the usual GL temperature dependence for ξ(T):

$$\xi =\frac{\xi (0)}{\sqrt {1-\frac{T}{T_{\text{cm}}} }} , $$

In addition, the standard BCS expression is used to find the temperature dependence of the energy gap in the limit of the weak electron-phonon interaction

$$ \frac{1}{N(0)V_{\text{ep}}} =\int\limits_{0}^{k_{B} {\Theta}_{\mathrm{D}}} {\frac{\mathrm{d}\varepsilon} {\sqrt {\varepsilon^{2}+{{\Delta}_{m}^{2}} (T)}} } th\frac{\sqrt {\varepsilon^{2}+{{\Delta}_{m}^{2}} (T)}} {2k_{B} T}, $$
(10)

where k B is the Boltzmann constant, ΘD is the Debye temperature, and ε is the energy of the free electrons. In this case, Δm(T) is the temperature dependence of the gap in the spectrum of excitations of a hypothetical bulk superconductor consisting only of the CuO2 planes. Also, we assume that usual BCS relation connecting Δm and T cm is valid:

$$ 2{\Delta}_{m} (0)=3.52k_{B} T_{\text{cm}} . $$
(11)

In the BCS theory, the T cm is the mean-field critical temperature of the hypothetical superconductor consisting only of the CuO2 planes where n = ∞. The estimations, which were made in the works [12, 13] by using the method, demonstrated that the best agreement the theoretical and experimental dependencies of the critical temperature as a function of the number of superconducting CuO2 planes was achieved for the T cm = T c(n = ∞) = 155 K. For this reason, the value of T cm is used in the present study.

3 Results of the Calculations

In thin superconducting layers, the boundary condition (2) results in the order parameter depression compared to the order parameter of the massive superconductor [9,10,11]. Figure 1 shows the dependence of the parameter in the center of a layer obtained from (6) as a function of the thickness of the layer at zero temperature T = 0 for different Λ. We denote ψ(x = 0,T = 0) as ψ 0. It will be noted that the dependence ψ(x) reaches a maximum in the center of the layer [11]. Since the GL theory is applicable only for small-order parameters, it is usually believed, that the temperature range of its applicability is restricted to the temperature region close to the critical temperature. But Fig. 1 demonstrates that there is a thickness range where 0 < ψ 0 << 1 for different finite Λ. It is very important, because small value of the order parameter is the main condition for the validity of the GLG theory [6, 8]. In this case, (6) can be used and the superconducting order parameter can be interpreted as pairing potential. Thus, the pairing potential of the thin superconducting layers is determined by the simple (6) not only near T cm but in a wide temperature range in this limit. The approach can be used in case of the cupper oxide HTSC.

Fig. 1
figure 1

The dependences of the order parameter in the center of a layer ψ(x = 0) at zero temperature and critical temperature T c (dashed lines) as a function of the layer thickness for different Λ

Full size image

We also show in Fig. 1 the thickness dependence of the critical temperature T c for two Λ by using dashed lines. Early works [9, 10, 14] had shown that the boundary condition (2) fixes the critical temperature of the superconducting film through:

$$ \tan [d/2\xi (T_{c} )]=\xi (T_{c} )/{\Lambda} $$
(12)

In case d << ξ(T c), the relation can be written as

$$ d{\Lambda} =2\xi^{2}(T_{c} ). $$
(13)

Using the empirical temperature dependence of ξ(T) (8), we can write

$$ T_{c} (d)=T_{\text{cm}} \left( {1-\frac{2\xi^{2}(0)}{d{\Lambda}} } \right)^{0.5} $$
(14)

Figure 1 shows the T c(d)/T cm dependences obtained from the relation (12). It should be noted, the T c(d)/T cm are close to the ψ 0(d) dependences for different Λ.

Temperature dependence of the order parameter can be obtained from the GL free energy to second order in the ψ 0, that is correct in the limit ψ 0 << 1 [9]. Evidently, in the case d << ξ(T c), the ψ 0(x) = const. Thus, in normalized units, the superconducting GL free energy, in absence of external magnetic field, reads as:

$$ F\sim {\psi_{0}^{2}} \left[ {-d/\xi^{2}(T)+2/{\Lambda}} \right] $$
(15)

Second term in the formula is added to take into account an influence of the superconducting thin film surface. Taking into account (13), we have

$$ F\sim d{\psi_{0}^{2}} \left[ {\xi^{-2}(T_{c} )-\xi^{-2}(T)} \right] $$
(16)

Using the formula (8) for the ξ(T), we receive

$$ F\sim \frac{d{\psi_{0}^{2}} {T_{c}^{2}}} {{\xi_{0}^{2}} T_{\text{cm}}^{2}} \left[ {1-\frac{T^{2}}{{T_{c}^{2}}} } \right] $$
(17)

In case of the usual boundary condition (5), the GL theory gives

$$ F\sim -d{\psi_{0}^{2}} \left[ {\xi^{-2}(T)} \right]. $$
(18)

Thus, the additional term appears in (16) in comparison with (18), and the ψ 0 transforms into T c ψ 0/T cm, which explains the correlation between T c(d) and ψ 0(d).

Since the main aim of my work is to study the effect of the new boundary condition (2) on the properties of high T c copper oxides superconductors, the superconducting plate is assumed to be formed by closely spaced CuO2 layers in the calculations. The number (n) in each unit cell varies from 1 to 3. The parameters T c and Λ were taken close to the values used in [12, 13] in which the T c(n) dependence was explained. Using the empirical formula (3) for the determination of the extrapolation length and assuming that T cs = T c (n = 1), we found that Λ/ξ(0) = 2 for Bi-based superconductor Bi2Sr2CaCu2 O 8. On the hand, the value T c (n = 2) = 90 K using (12) leads to Λ/ξ(0) = 2.3. The two values of the Λ determined by different methods which are close enough indicates that the used approach is enough reason for the study of cuprate superconductors. On the other hand, the microscopic formula which works well in the case of classical metallic superconductors, makes it possible to define the interaction potential N(0)V in cuprate superconductors. In the case of Λ/ξ(0) = 2.3 and a = l, this formula gives N(0)V ep ≈ 1.4 that indicates the presence of a strong coupling interaction of the electrons in Cooper pairs.

Figure 2a, b show examples of the distribution of the order parameter on the thickness of the superconducting layer, calculated by solving (6) with boundary conditions (7). In the calculations, we use the parameters of Bi-based superconductors. The origin of the abscissa axis corresponds to the center of the plate. Figure 2a corresponds to n = 1, and Fig. 2b to n = 2. As can be seen from the figures, new boundary conditions lead to the suppression of the order parameter as well as pairing potential in vicinity of the boundaries of the plate. The figures demonstrate the thinner superconducting layers the stronger-order parameter suppression, but the change of the order parameter across the plate is more significant in the thicker plates with n = 2 and 3. The thickness of a single CuO2 layer is assumed to be 2.4 Å. Hence, the GLG approach for Bi-based superconductors with n = 1 is more acceptable than that one for these superconductors with n = 2 and 3.

Fig. 2
figure 2

a, b Typical space dependences of the pairing potential in the superconducting layers. In this case, we use the parameters of Bi-based superconductors like Bi2Sr2Can−1Cun O 4+2n. a The superconducting layer with n = 1 (one CuO2 plane per unit cell), Λ/ξ(0) = 3.3, and b n = 2, Λ/ξ(0) = 2.3

Full size image

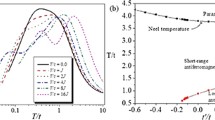
Evidently, the effect of the boundaries on pairing remains in the depth of the layered superconductors. This suggests that the thickness averaged pairing potential plays the role of the energy gap in these superconductors. Figure 3 shows an example of the temperature dependences of pairing potential < Δ(x) > averaged across the plate. It follows from (10) that the BCS dependence Δm(T) behaves as (T cm − T)1/2 near the critical temperature. At finite values of Λ, the root law of the temperature dependence of the thickness averaged pairing potential < Δ(x) >∼ (T c − T)1/2 is saved according to the calculations. It is worthy to note that this result is not trivial because the character of the dependence is defined by the temperature dependency of the coherence length ξ and the energy gap in the spectrum of excitations of a hypothetical massive superconductor consisting only of the CuO2 layers in the vicinity of the T cm. The influence of boundaries results in the decreasing critical temperature of the plate, but in the vicinity of the transition temperature T c, ξ(T), and Δm(T) are essentially constants due to the relations (8, 10). This temperature dependence of the averaged pairing potential is explained with regard to the additional surface term in the Ginzburg-Landau free energy functional, which leads to the formula (17) which is derived under the assumption that the plate thickness is much less than the coherence length ξ. Hence, the GL order parameter as well as the pairing potential has the root temperature dependence near the T c. Our numerical calculations show that the root law of the dependence Δ(T) is maintained in a wide temperature range. For comparison, Fig. 3 shows the temperature dependence of the BCS energy gap Δ(T) obtained from the relations (10, 11). At the same time, T c(n) are used instead of the T cm. Figure 4 shows the temperature dependences of the (< Δ(x) >)2 to demonstrate the root law temperature dependences more evidently. This figure corresponds to n = 1. It would be noted, in vicinity of the transition temperature, the BCS energy gap dependence for weak coupling is:

$$ {\Delta} (T)\approx 3.2k_{B} T_{c} \left( {\frac{T_{c} -T}{T_{c}} } \right)^{0.5} $$
(19)

At low temperatures, the calculated ratio \(\frac {2<{\Delta } (x)>}{k_{B} T_{\tilde {n}}} \) is approximately equal to 3.52, which is obtained in the BCS theory for weak coupling. It will be noted that our calculations give only the ratio of the Δ(T) to Δm(0); moreover, we believe that usual BCS relation (11) for weak coupling is valid. In case of strong coupling, the Δ(T) should be increased with increasing the Δ m (0).

Fig. 3
figure 3

Temperature dependence of the thickness averaged pairing potential < Δ > for Bi2Sr2CuO6 (scatter graph). The dashed curve shows the temperature dependence of the BCS energy gap Δ(T)

Full size image
Fig. 4
figure 4

The upper curves in both figures show the temperature dependences of the < Δ >2 to the superconducting layer with n = 1. The lower curve show the temperature dependence of the Δ2(T) obtained in the BCS theory

Full size image

4 Conclusions

We found the space dependence of the pairing potential across the thickness of the superconducting CuO2 layers in copper oxides HTSC. The approach proposed in this paper is applicable also for the S–N–S structures with weak Josephson interaction between superconducting layers made of classical low-temperature superconductors. It is established that the pairing potential is significantly depressed due to the influence of non-superconducting layers, which leads also to the reduction of the critical temperature of these superconductors. The temperature dependence of the effective energy gap was calculated in the work by using GLG approach. The gap appears to increase with the number n of CuO2 planes per unit cell. Comparison is performed of the obtained dependences with the similar dependences following from the BCS theory. The bulk interaction potential in cuprate HTSC is estimated in this work by using BCS theory. The value of N(0) V ep indicates the presence of a strong coupling interaction of the electrons in Cooper pairs.

Of course, the equation of the macroscopic GL theory extends to a microscopic length range to analyze the high-temperature cupper oxide superconductors in this work which requires further study.