Abstract
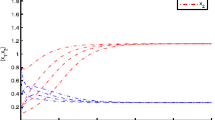
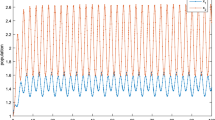
This article deals with a stage-structured model with state-dependent delay which is assumed to be an increasing function of the population density with lower and upper bound. Firstly, according to the principle of linearized stability (Theorem 3.6, Hartung et al. in Handbook of differential equations: ordinary differential equations, 2006), we study the local stability of system in combination with the positivity and boundedness of solutions. By using the comparison principle obtained and an iterative method, the global stability of the equilibria is completely analyzed. Our results show how the interaction between interspecific and intraspecific competition affects the coexistence of both species.

Similar content being viewed by others
References
Das, K., Ray, S.: Effect of delay on nutrient cycling in phytoplankton–zooplankton interactions in estuarine system. Ecol. model. 215, 69–76 (2008)
Kuang, Y.: Delay Differential Equation with Applications in Population Dynamics. Academic Press, New York (1993)
Hale, J.: Theory of Functional Differential Equations. Springer, New York (1977)
Yang, Y.: Hopf bifurcation in a two-competitor, one-prey system with time delay. Appl. Math. Comput. 214, 228–235 (2009)
Barclay, H., Driessche, P.: A model for a single species with two life history stages and added mortality. Ecol. Model 11, 157–166 (1980)
Gurney, W., Nisbet, R., Lawton, J.: The systematic formulation of tractible single species population models incorporating age structure. J. Anim. Ecol. 52, 479–485 (1983)
Tognetti, K.: The two stage stochastic model. Math. Biosci. 25, 195–204 (1975)
Landahl, H., Hanson, B.: A three stage population model with cannibalism. Bull. Math. Biol. 37, 11–17 (1975)
Wood, S., Blythe, S., Gurney, W., Nisbet, R.: Instability in mortality estimation schemes related to stage-structure population models. IMA J. Math. Appl. Med. Biol. 6, 47–68 (1989)
Aiello, W., Freedman, H., Wu, J.: Analysis of a model representing stage-structured population growth with state-dependent time delay. SIAM J. Appl. Math 52, 855–869 (1992)
Gambell, R.: Birds and mammals-Antarctic whales. In: Bonner, W., Walton, D. (eds.) Antarctica, pp. 223–241. Pergamon Press, New York (1985)
Andrewartha, H., Birch, L.: The Distribution and Abundance of Animals, p. 370. University of Chicago Press, Chicago, IL (1954)
Zaghrout, A., Attalah, S.: Analysis of a model of stage-structured population dynamics growth with state-dependent time delay. Appl. Math. Comput. 77, 185–194 (1996)
Al-Omari, J., Gourley, S.: Dynamics of a stage-structured population model incorporating a state-dependent maturation delay. Nonlinear Anal. Real World Appl. 6, 13–33 (2005)
Al-Omari, J., Tallafha, A.: Modelling and analysis of stage-structured population model with state-dependent maturation delay and harvesting. Int. J. Math. Anal. 1, 391–407 (2007)
Adimy, M., Crauste, F., Hbid, M., Qesmi, R.: Stability and Hopf bifurcation for a cell population model with state-dependent delay. SIAM J. Appl. Math. 70, 1611–1633 (2010)
Hu, Q., Zhao, X.: Global dynamics of a state-dependent delay model with unimodal feedback. J. Math. Anal. Appl. 399, 133–146 (2013)
Gause, G.: The Struggle for Existence. Williams and Wilkins, Baltimore (1934)
Kareiva, P.: Renewing the dialogue between theory and experiments in population ecology. In: Roughgarden, J., May, R., Levin, S. (eds.) Perspectives in Ecological Theory, pp. 68–88. Princeton University Press, Princeton (1989)
Gopalsamy, K.: Time lags and global stability in two-species competition. Bull. Math. Biol. 42, 729–737 (1980)
Bartoszewski, Z., Jackiewicz, Z., Kuang, Y.: Numerical solution of threshold problems in epidemics and population dynamics. J. Comput. Appl. Math. 279, 40–56 (2015)
Smith, H., Kuang, Y.: Periodic solutions of delay differential equations of threshold-type delay. In: Graef, Hale, (eds.) Oscillation and Dynamics in Delay Equations. Contemporary Mathematics, vol. 129, pp. 153–176. AMS, Providence, RI (1992)
Kuang, Y.: Delay differential equations. In: Hastings, A., Gross, L.J. (eds.) Encyclopedia of Theoretical Ecology, pp. 163–166. University of California Press, Berkeley, CA (2012)
Al-Omari, J., Gourley, S.: Stability and traveling fronts in Lotka–Vloterra competition models with stage structure. SIAM J. Appl. Math. 63, 2063–2086 (2003)
Hartung, F., Krisztin, T., Walther, H., Wu, J.: Functional differential equations with state-dependent delay: theory and applications. In: Canada, A., Drabek, P., Fonda, A. (eds.) Handbook of Differential Equations: Ordinary Differential Equations, vol. 3, pp. 435–545. Elsevier Science B. V., North-Holland, Amsterdam (2006)
Walther, H.: The solution manifold and \(C^1\)-smoothness of solution operators for differential equations with state dependent delay. J. Differ. Equ. 195, 46–65 (2003)
Gopalsamy, K.: Stability and Oscillations in Delay Differential Equations of Population Dynamics. Kluwer, Dordrecht (1992)
Hirsch, W., Hanisch, H., Gabriel, J.: Differential equations models of some parasitic infections, methods for the study of asymptotic behavior. Comm. Pure. Appl. Math. 38, 733–753 (1985)
Martin, R., Smith, H.: Abstract functional differential equations and reaction–diffusion systems. Trans. Am. Math. Soc. 321, 1–44 (1990)
Hale, J., Lunel, S.: Introduction to Functional Differential Equations. Springer, New York (1993)
Acknowledgments
We are extremely grateful to the critical comments and invaluable suggestions made by anonymous honorable reviewers. This work is supported by the National Nature Science Foundation of China, RFDP.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Lv, Y., Yuan, R., Pei, Y. et al. Global Stability of a Competitive Model with State-Dependent Delay. J Dyn Diff Equat 29, 501–521 (2017). https://doi.org/10.1007/s10884-015-9475-5
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10884-015-9475-5