The Characteristic Curves of Water

Abstract

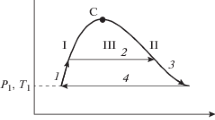
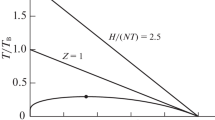
In 1960, E. H. Brown defined a set of characteristic curves (also known as ideal curves) of pure fluids, along which some thermodynamic properties match those of an ideal gas. These curves are used for testing the extrapolation behaviour of equations of state. This work is revisited, and an elegant representation of the first-order characteristic curves as level curves of a master function is proposed. It is shown that Brown’s postulate—that these curves are unique and dome-shaped in a double-logarithmic p, T representation—may fail for fluids exhibiting a density anomaly. A careful study of the Amagat curve (Joule inversion curve) generated from the IAPWS-95 reference equation of state for water reveals the existence of an additional branch.

Appendices

Appendix 1: Thermodynamic Derivatives

All derivatives are computed at constant amount of substance n.

isochoric tension coefficient:

$$\begin{aligned} \beta _V \equiv \left( \frac{\partial p}{\partial T} \right) _{V} = -\left( \frac{\partial p}{\partial V} \right) _{T} \left( \frac{\partial V}{\partial T} \right) _{P} = \frac{\alpha _p}{\kappa _T}. \end{aligned}$$

(26)

derivatives appearing in Eq. 19:

$$\begin{aligned} \left( \frac{\partial Z}{\partial T} \right) _{V}= & {} \frac{V}{n R T} \left( \frac{\partial p}{\partial T} \right) _{V} - \frac{p V}{n R T^2} = \frac{Z \alpha _p}{p \kappa _T} - \frac{Z}{T} = \frac{c_\kappa c_T - Z}{T},\nonumber \\ \left( \frac{\partial Z}{\partial p} \right) _{V}= & {} -\frac{p V}{n R T^2} \left( \frac{\partial T}{\partial p} \right) _{V} + \frac{V}{n R T} = \frac{Z}{p} \left( 1 - \frac{p \kappa _T}{T \alpha _p} \right) = \frac{Z}{p} \left( 1 - \frac{Z}{c_\kappa c_T} \right) ,\nonumber \\ \left( \frac{\partial Z}{\partial p} \right) _{T}= & {} \frac{p}{n R T} \left( \frac{\partial V}{\partial p} \right) _{T} + \frac{V}{n R T} = \frac{Z}{p} (1 - p \kappa _T) = \frac{Z}{p} \left( 1 - \frac{Z}{c_\kappa } \right) ,\nonumber \\ \left( \frac{\partial Z}{\partial V} \right) _{T}= & {} \frac{V}{n R T} \left( \frac{\partial p}{\partial V} \right) _{T} + \frac{p}{n R T} = \frac{p - \kappa _T^{-1}}{n R T} = \frac{Z - c_\kappa }{V},\nonumber \\ \left( \frac{\partial Z}{\partial T} \right) _{p}= & {} \frac{p}{n R T} \left( \frac{\partial V}{\partial T} \right) _{p} - \frac{p V}{n R T^2} = Z \alpha _p - \frac{Z}{T} = \frac{Z}{T} (c_T - 1),\nonumber \\ \left( \frac{\partial Z}{\partial V} \right) _{p}= & {} -\frac{p V}{n R T^2} \left( \frac{\partial T}{\partial V} \right) _{p} + \frac{p}{n R T} = \frac{Z}{V} \left( 1 - \frac{1}{T \alpha _p} \right) = \frac{Z}{V} \left( 1 - \frac{1}{c_T} \right) .\nonumber \\ \end{aligned}$$

(27)

Appendix 2: The Terminal Slopes of the Characteristic Curves at High Temperatures

Schaber presents expressions for the terminal slopes of Brown’s characteristic curves in his thesis [16], but does not give the proofs. For the readers’ convenience, these proofs are offered here.

For small pressures and large molar volumes the fluid can be described with a 3-term virial equation (cf. Eq. 20),

$$\begin{aligned} Z = 1 + \frac{B_2(T)}{V_\mathrm{m}} + \frac{B_3(T)}{V_\mathrm{m}^2}. \end{aligned}$$

(28)

The molar volume as a function of pressure is then, neglecting higher-order terms,

$$\begin{aligned} V_\mathrm{m}\approx \frac{R T}{p} + B_2(T) + \frac{p B_3(T)}{R T}. \end{aligned}$$

(29)

1.1 (a) The Terminal Slope of the Amagat Curve

Applying the first one of the Amagat criteria in Eq. 2 to the virial equation yields

$$\begin{aligned} \left( \frac{\partial Z}{\partial T} \right) _{V_\mathrm{m}} = \frac{B_2^\prime (T)}{V_\mathrm{m}} + \frac{B_3^\prime (T)}{V_\mathrm{m}^2} = 0 \end{aligned}$$

or

$$\begin{aligned} B_2^\prime (T) + \frac{B_3^\prime (T)}{V_\mathrm{m}} = 0. \end{aligned}$$

(30)

In the limit $p \rightarrow 0$, $V_\mathrm{m}\rightarrow \infty$ the second term can be neglected, and therefore $B_2^\prime (T) = 0$ is the criterion for the endpoint of the Amagat curve, which corresponds to a maximum of the second virial coefficient.

Let $T_\mathrm{A}$ denote the temperature of this endpoint, and $\Delta T = T - T_\mathrm{A}$ and $\Delta p = p$ the temperature and pressure deviations, respectively, from this point. Then, in the vicinity of $T_\mathrm{A}$, $B_2^\prime (T)$ can be approximated by

$$\begin{aligned} B_2^\prime (T) \approx B_2^{\prime \prime }(T_\mathrm{A}) \Delta T , \end{aligned}$$

where $B_2^{\prime \prime }(T_\mathrm{A})$ denotes the curvature of the second virial coefficient function at the Amagat endpoint. Substituting this into Eq. 30 and switching from molar volume to pressure yields

$$\begin{aligned} B_2^{\prime \prime }(T_\mathrm{A}) \Delta T + \frac{B_3^\prime (T)}{\frac{R T}{\Delta p} + B_2 + \ldots } = 0 \end{aligned}$$

and, after some rearrangements,

$$\begin{aligned} \frac{\Delta p}{\Delta T} = - \frac{B_2^{\prime \prime }(T_\mathrm{A})}{B_3^\prime (T)} \left( R T + \Delta p B_2(T) + \ldots \right) . \end{aligned}$$

In the limit $\Delta p \rightarrow 0, T \rightarrow T_\mathrm{A}$ this reduces to

$$\begin{aligned} \lim _{T \rightarrow T_\mathrm{A}} \frac{\mathrm{d}p}{\mathrm{d}T} \Big \vert _{q_\mathrm{R} = -\frac{1}{2}} = -\frac{B_2^{\prime \prime }(T_\mathrm{A}) R T_\mathrm{A}}{B_3^\prime (T_\mathrm{A})}. \end{aligned}$$

(31)

1.2 (b) The Terminal Slope of the Boyle Curve

Applying the criterion Eq. 5 to the virial equation gives

$$\begin{aligned} \left( \frac{\partial Z}{\partial V_\mathrm{m}} \right) _{T} = - \frac{B_2(T)}{V_\mathrm{m}^2} - \frac{2 B_3(T)}{V_\mathrm{m}^3} = 0 \end{aligned}$$

and hence

$$\begin{aligned} B_2(T) + \frac{2 B_3(T) p}{R T + B_2(T) p + \ldots } = 0. \end{aligned}$$

(32)

In the limit $p \rightarrow 0$, this reduces to $B_2 = 0$: At the endpoint of the Boyle curve, at the Boyle temperature $T_\mathrm{B}$, the second virial coefficient vanishes.

Again using deviation variables, we can write the previous equation as

$$\begin{aligned} B_2^\prime (T_\mathrm{B}) \Delta T + \frac{2 B_3(T) \Delta p}{R T + \Delta p B_2(T) + \ldots } = 0. \end{aligned}$$

or

$$\begin{aligned} \frac{\Delta p}{\Delta T} = -\frac{B_2^\prime (T_\mathrm{B})}{2 B_3(T)} \left( R T + \Delta p B_2(T) + \ldots \right) . \end{aligned}$$

In the limit $\Delta p \rightarrow 0, T \rightarrow T_\mathrm{B}$ this reduces to

$$\begin{aligned} \lim _{T \rightarrow T_\mathrm{B}} \frac{\mathrm{d}p}{\mathrm{d}T} \Big \vert _{q_\mathrm{R} = 0} = -\frac{B_2^\prime (T_\mathrm{B}) R T_\mathrm{B}}{2 B_3(T_\mathrm{B})}. \end{aligned}$$

(33)

1.3 (c) The Terminal Slope of the Charles Curve

For the derivation of this property, it is advantageous to start from the pressure-based virial equation of state,

$$\begin{aligned} Z = 1 + \bar{B}_2(T) p + \bar{B}_3(T) + p^2. \end{aligned}$$

(34)

The pressure-based virial coefficients are related to the volume-based ones by

$$\begin{aligned} \bar{B}_2(T) = \frac{B_2(T)}{R T} \quad \text {and} \quad \bar{B}_3(T) = \frac{B_3(T) - B_2^2(T)}{(R T)^2}. \end{aligned}$$

(35)

Applying the appropriate criterion in Eq. 8 gives

$$\begin{aligned} \left( \frac{\partial Z}{\partial T} \right) _{p} = \frac{p}{R T}\left( B_2^\prime (T) - \frac{B(T)}{T} \right) + \left( \frac{p}{R T} \right) ^2 \left( B_3^\prime (T) - \frac{2 B_3(T)}{T} - 2 B_2(T) B_2^\prime (T) + \frac{2 B_2^2(T)}{T} \right) = 0. \end{aligned}$$

or

$$\begin{aligned} B_2^\prime (T) - \frac{B_2(T)}{T} + \frac{p}{R T} \left( B_3^\prime (T) - \frac{2 B_3(T)}{T} - 2 B_2(T) B_2^\prime (T) + \frac{2 B_2^2(T)}{T} \right) = 0. \end{aligned}$$

(36)

The endpoint, at $p \rightarrow 0$, is evidently characterized by $B_2^\prime (T) - B_2(T)/T = 0$.

In order to obtain the terminal slope, we expand this criterion around the endpoint temperature $T_\mathrm{C}$,

$$\begin{aligned} B_2^\prime (T) - \frac{B_2(T)}{T} \approx \left( B_2^{\prime \prime }(T_\mathrm{C}) - \frac{B_2^\prime (T_\mathrm{C})}{T_\mathrm{C}} + \frac{B_2(T_\mathrm{C})}{T_\mathrm{C}^2} \right) \Delta T = B_2^{\prime \prime }(T_\mathrm{C}) \Delta T. \end{aligned}$$

Then Eq. 36 becomes

$$\begin{aligned} B_2^{\prime \prime }(T_\mathrm{C}) \Delta T = -\frac{\Delta p}{R T} \left( B_3^\prime (T) -\frac{2 B_3(T)}{T} - 2 B_2(T) \left[ B_2(T)^\prime - \frac{B_2(T)}{T}\right] \right) = 0. \end{aligned}$$

In the limit $\Delta p \rightarrow 0, T \rightarrow T_\mathrm{C}$, where the term in brackets vanishes, this yields the terminal slope,

$$\begin{aligned} \lim _{T \rightarrow T_\mathrm{C}} \frac{\mathrm{d}p}{\mathrm{d}T} \Big \vert _{q_\mathrm{R} = -\frac{1}{3}} = -\frac{B_2^{\prime \prime }(T_\mathrm{C}) R T_\mathrm{C}}{B_3^\prime (T_\mathrm{C}) - \frac{2}{T_\mathrm{C}} B_3(T_\mathrm{C})} . \end{aligned}$$

(37)