Optimal incentives for allocating HIV/AIDS prevention resources among multiple populations

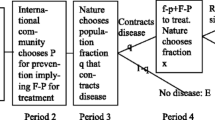
Abstract

Many agencies, such as the United Nations Program on HIV/AIDS (UNAIDS), the World Health Organization (WHO), the World Bank, the U.S. President’s Emergency Plan for AIDS Relief (PEPFAR), and the Global Fund to Fight AIDS, Tuberculosis and Malaria, provide funding to prevent HIV/AIDS infections worldwide. These funds are allocated at multiple levels, resulting in a highly complicated distribution process. An oversight agency allocates funds to various national-level decision-makers who then allocate funds to regional-level decision-makers who in turn distribute the monies to local organizations, programs, or risk groups. Simple allocation techniques are often preferred by the decision-makers at each administrative level, but such methods can lead to sub-optimal allocation of funds. Thus, incentives could be provided to decisionmakers in order to encourage optimal allocation of HIV/AIDS prevention resources. We formulate an incentive-based resource allocation model that takes into consideration strategic interactions between decision-makers in a multiple-level resource-allocation process. We analyze each decision-maker’s behavior at the equilibrium and summarize the results that characterize the optimal solution to the resource-allocation problem. Our intended audiences are technical experts, decision-makers, and policy-makers in governments who can make use of incentives to encourage effective decisions regarding HIV/AIDS policy modeling and budget allocation at local levels.

Appendix

1.1 A.1. Optimal solution of the “Knapsack Continuos Problem” of Stage 3

$$ \matrix{{*{20}{c}} {{ \max }\,I{A_i} = \sum\limits_{{j = 1}}^m {{h_{{ij}}}{y_{{ij}}}}, i = 1,2} \hfill \\ {{\text{s}}{\text{.t}}{.}\,\sum\limits_{{j = 1}}^m {{y_{{ij}}}} \leqslant {Z_i}} \hfill \\ {{y_{{ij}}} \geqslant {r_i}{Z_i}\frac{{{n_{{ij}}}}}{{{N_i}}},\,\,\,\,\,\,j = 1,2, \ldots, m} \hfill \\ } $$

The result of Stage 3 is the function IA _i (r _i ), the optimal solution of this “Knapsack Continuos Problem” is of the following form:

$$ \matrix{{*{20}{c}} {{y_{{i1}}} = {Z_i} - {r_i}{Z_i}\frac{{{n_{{i2}}}}}{{{N_i}}} - \ldots - {r_i}{Z_i}\frac{{{n_{{im}}}}}{{{N_i}}},i = 1,2} \hfill \\ {{y_{{ij}}} = {r_i}{Z_i}\frac{{{n_{{ij}}}}}{{{N_i}}},\,\,\,\,\,j = 2, \ldots, m} \hfill \\ } $$

The result of Stage 3 is the function IA _i (r _i ),

$$ \matrix{{*{20}{c}} {I{A_i}\left( {{r_i}} \right) = {Z_i}\left( {{h_{{i1}}} - {r_i}\left( {{h_{{i1}}} - {h_{{i2}}}} \right)\frac{{{n_{{i2}}}}}{{{N_i}}} - \ldots - {r_i}\left( {{h_{{i1}}} - {h_{{im}}}} \right)\frac{{{n_{{im}}}}}{{{N_i}}}} \right)} \\ { = \left( {\frac{{\left( {1 - {r_i} + {r_{{-i }}}} \right)}}{2}\left( {1 - f} \right)B + \left( {\frac{{{N_i}}}{{{N_1} + {N_2}}}} \right)fB} \right)\left( {{h_{{i1}}} - {k_i}} \right),} \\ } $$

where ${k_i} = \left( {{h_{{i1}}} - {h_{{i2}}}} \right)\frac{{{n_{{i2}}}}}{{{N_i}}} + \ldots + \left( {{h_{{i1}}} - {h_{{im}}}} \right)\frac{{{n_{{im}}}}}{{{N_i}}} < {h_{{i1}}},i = 1,2$.

We define the following terms before presenting the proof:

$$ \matrix{{*{20}{c}} {{f_{{ti}}} = \frac{{\frac{{\left( {{h_{{i1}}} - {k_i}} \right)\left( {1 - 2{b_i}} \right) + {c_i}{k_i}}}{{{b_i}\left( {{h_{{i1}}} - {k_i}} \right) - {c_i}{k_i}}}}}{{\frac{{2{N_i}}}{{{N_1} + {N_2}}} + \frac{{\left( {{h_{{i1}}} - {k_i}} \right)\left( {1 - 2{b_i}} \right) + {c_i}{k_i}}}{{{b_i}\left( {{h_{{i1}}} - {k_i}} \right) - {c_i}{k_i}}}}},} \\ {{r_{{t - i}}} = \frac{{\left( {{c_i}{k_i} - {b_i}\left( {{h_{{i1}}} - {k_i}} \right)} \right)\frac{{fB{N_i}}}{{{N_1} + {N_2}}} + \left( {{h_{{i1}}} - {k_i}} \right)\left( {1 - f} \right)\frac{B}{2}\left( {1 - {b_i}} \right)}}{{\left( {1 - f} \right)\frac{B}{2}\left( {{b_i}\left( {{h_{{i1}}} - {k_i}} \right) - {c_i}{k_i}} \right)}}.} \\ } $$

1.2 A.2. Proposition 1: For problem L _i with three the sub-populations:

• 1) U _i is a concave function of r _i and therefore the optimal solution is either $r_i^{*} = r_i^l$ or $r_i^{*} = 1$.

• 2) Table 1 specifies conditions for existence of Nash equilibria $\left( {r_i^l,r_{{ - i}}^l} \right),\left( {r_i^l,1} \right),\left( {1,r_{{ - i}}^l} \right)$, and (1,1).

Proof of Proposition 1

• (i) The lower-level utility function is ${\left( {{Z_i}} \right)^{{{a_i}}}}{\left( {{r_i}} \right)^{{{b_i}}}}{\left( {I{A_i}} \right)^{{{c_i}}}}$, where ${Z_i} = \frac{{\left( {1 - {r_i} + {r_{{ - i}}}} \right)}}{2}\left( {1 - f} \right)B + \frac{{{N_i}}}{{{N_1} + {N_2}}}fB$ and $I{A_i} = \left( {\frac{{\left( {1 - {r_i} + {r_{{ - i}}}} \right)}}{2}\left( {1 - f} \right)B + \left( {\frac{{{N_i}}}{{{N_1} + {N_2}}}} \right)fB} \right)\left( {{h_{{i1}}} - {r_i}\left( {{h_{{i1}}} - {h_{{i2}}}} \right)\frac{{{n_{{i2}}}}}{{{N_2}}} - {r_i}\left( {{h_{{i3}}} - {h_{{i3}}}} \right)\frac{{{n_{{i3}}}}}{{{N_3}}}} \right)$ are substituted to obtain:

  $$ \matrix{{*{20}{c}} {{L_i}:\mathop{{\max }}\limits_{{{r_i}}} {U_i}\left( {{r_i}} \right) = {{\left( {{Z_i}} \right)}^{{{a_i}}}}{{\left( {{r_i}} \right)}^{{{b_i}}}}{{\left( {I{A_i}} \right)}^{{{c_i}}}},\,{\text{where}}\,{a_i} + {b_i} + {c_i} = 1} \hfill \\ {{\text{s}}{\text{.t}}{.}\,0 \leqslant {r_i} \leqslant 1} \hfill \\ } $$

  The first derivative of ln U _i (r _i ) with respect to r _i is given by,

  $$ \matrix{{*{20}{c}} {\frac{d}{{d{r_i}}}\left( {\ln {U_i}\left( {{r_i}} \right)} \right) = \frac{d}{{d{r_i}}}\left( {{a_i}\ln {Z_i} + {b_i}\ln {r_i} + {c_i}\ln I{A_i}} \right)} \hfill \\ { = - \frac{{{a_i}}}{{{Z_i}}}\left( {1 - f} \right)\frac{B}{2} + \frac{{{b_i}}}{{{r_i}}} - \frac{{{c_i}}}{{I{A_i}}}\left( {\left( {1 - f} \right)\frac{B}{2}\left( {{h_{{i1}}} - {r_i}{k_i}} \right) + {k_i}{Z_i}} \right)} \hfill \\ } $$

  where, $\frac{{d{Z_i}}}{{d{r_i}}} = - \left( {1 - f} \right)\frac{B}{2},\frac{{dI{A_i}}}{{d{r_i}}} = - \left( {1 - f} \right)\frac{B}{2}\left( {{h_{{i1}}} - {r_i}{k_i}} \right) - {k_i}{Z_i}$. The second derivative of ln U _i (r _i ) with respect to r _i is given by,

  $$ \matrix{{*{20}{c}} {\frac{{{d^2}}}{{d{r_i}^2}}\left( {\ln {U_i}\left( {{r_i}} \right)} \right) = \frac{{{a_i}}}{{{Z_i}^2}}\left( {1 - f} \right)\frac{B}{2}\frac{d}{{d{r_i}}}\left( {{Z_i}} \right) - \frac{{{b_i}}}{{{r_i}^2}} - {c_i}\frac{d}{{d{r_i}}}\left( {\frac{{\left( {1 - f} \right)\frac{B}{2}\left( {{h_{{i1}}} - {r_i}{k_i}} \right) + {k_i}{Z_i}}}{{I{A_i}}}} \right)} \hfill \\ { = - \frac{{{a_i}}}{{{Z_i}^2}}{{\left( {1 - f} \right)}^2}\frac{{{B^2}}}{4} - \frac{{{b_i}}}{{{r_i}^2}} - {c_i}\frac{{ - \left( {1 - f} \right)\frac{B}{2}\left( {{h_{{i1}}} - {r_i}{k_i}} \right){k_i}{Z_i} + {{\left( {\left( {1 - f} \right)\frac{B}{2}\left( {{h_{{i1}}} - {r_i}{k_i}} \right) + {k_i}{Z_i}} \right)}^2}}}{{I{A_i}^2}}} \hfill \\ { = - \frac{{{a_i}}}{{{Z_i}^2}}{{\left( {1 - f} \right)}^2}\frac{{{B^2}}}{4} - \frac{{{b_i}}}{{{r_i}^2}} - {c_i}\frac{{{{\left( {1 - f} \right)}^2}\frac{{{B^2}}}{4}{{\left( {{h_{{i1}}} - {r_i}{k_i}} \right)}^2} + {k_i}^2{Z_i}^2}}{{I{A_i}^2}} \leqslant 0.} \hfill \\ } $$

  Clearly, $\ln \left( {{U_i}\left( {{r_i}} \right)} \right)\prime \prime \leqslant 0$. Thus, U _i (r _i ) is a concave function of r _i and the optimal solution is either $r_i^{*} = r_i^l$ or 1.

• (ii) To obtain specific conditions for existence of Nash equilibria, we solve for r _i * by setting, $\ln \left( {{U_i}\left( {{r_i}} \right)} \right)\prime = 0$.

The first derivative of lnU _i (r _i ) is

$$ \matrix{{*{20}{c}} { - \frac{{{a_i}}}{{{Z_i}}}\left( {1 - f} \right)\frac{B}{2} + \frac{{{b_i}}}{{{r_i}}} - \frac{{{c_i}}}{{I{A_i}}}\left( {\left( {1 - f} \right)\frac{B}{2}\left( {{h_{{i1}}} - {r_i}{k_i}} \right) + {k_i}{Z_i}} \right) = 0} \hfill \\ {\frac{{{b_i}}}{{{r_i}}} = \frac{{{a_i}}}{{{Z_i}}}\left( {1 - f} \right)\frac{B}{2} + \frac{{{c_i}}}{{{Z_i}\left( {{h_{{i1}}} - {r_i}{k_i}} \right)}}\left( {\left( {1 - f} \right)\frac{B}{2}\left( {{h_{{i1}}} - {r_i}{k_i}} \right) + {k_i}{Z_i}} \right)} \hfill \\ {{b_i}{Z_i} = \left( {{a_i} + {c_i}} \right){r_i}\left( {1 - f} \right)\frac{B}{2} + \frac{{{c_i}{r_i}}}{{\left( {{h_{{i1}}} - {r_i}{k_i}} \right)}}{k_i}{Z_i}} \hfill \\ } $$

On substituting value of Z _i and rearranging terms in the above equation, we obtain

$$ r_i^2{a_q} + {r_i}{b_q} + {c_q} = 0 $$

(11)

where ${a_q} = {k_i}\left( {1 + {c_i}} \right)\left( {1 - f} \right)\frac{B}{2}$, ${b_q} = - \left( {{b_i} + {c_i}} \right){k_i}f\frac{{B{N_i}}}{{{N_1} + {N_2}}} - \left( {{h_{{i1}}} + \left( {{b_i} + {c_i}} \right)\left( {1 + {r_{{ - i}}}} \right){k_i}} \right)\left( {1 - f} \right)\frac{B}{2}$, ${c_q} = {b_i}{h_{{i1}}}\left( {f\frac{{B{N_i}}}{{{N_1} + {N_2}}} + \left( {1 + {r_{{ - i}}}} \right)\left( {1 - f} \right)\frac{B}{2}} \right)$.

Equation (11) has two positive real roots, since $b_q^2 - 4{a_q}{c_q} > 0$ as shown below.

$$ \matrix{{*{20}{c}} {b_q^2 - 4{a_q}{c_q} = {{\left( {\left( {{b_i} + {c_i}} \right){k_i}f\frac{{B{N_i}}}{{{N_1} + {N_2}}} + \left( {{h_{{i1}}} + \left( {{b_i} + {c_i}} \right)\left( {1 + {r_{{ - i}}}} \right){k_i}} \right)\left( {1 - f} \right)\frac{B}{2}} \right)}^2} - } \hfill \\ {4\left( {{k_i}\left( {1 + {c_i}} \right)\left( {1 - f} \right)\frac{B}{2}} \right)\left( {{b_i}{h_{{i1}}}\left( {f\frac{{B{N_i}}}{{{N_1} + {N_2}}} + \left( {1 + {r_{{ - i}}}} \right)\left( {1 - f} \right)\frac{B}{2}} \right)} \right)} \hfill \\ { = {{\left( {\left( {{b_i} + {c_i}} \right){k_i}\left( {f\frac{{B{N_i}}}{{{N_1} + {N_2}}} + \left( {1 + {r_{{ - i}}}} \right)\left( {1 - f} \right)\frac{B}{2}} \right) + {h_{{i1}}}\left( {1 - f} \right)\frac{B}{2}} \right)}^2} - } \hfill \\ {4{k_i}\left( {1 - f} \right)\frac{B}{2}{b_i}{h_{{i1}}}\left( {f\frac{{B{N_i}}}{{{N_1} + {N_2}}} + \left( {1 + {r_{{ - i}}}} \right)\left( {1 - f} \right)\frac{B}{2}} \right) - {c_i}4{k_i}\left( {1 - f} \right)\frac{B}{2}{b_i}{h_{{i1}}}\left( {f\frac{{B{N_i}}}{{{N_1} + {N_2}}} + \left( {1 + {r_{{ - i}}}} \right)\left( {1 - f} \right)\frac{B}{2}} \right)} \hfill \\ { = {{\left( {{b_i}{k_i}\left( {f\frac{{B{N_i}}}{{{N_1} + {N_2}}} + \left( {1 + {r_{{ - i}}}} \right)\left( {1 - f} \right)\frac{B}{2}} \right) + {c_i}{k_i}\left( {f\frac{{B{N_i}}}{{{N_1} + {N_2}}} + \left( {1 + {r_{{ - i}}}} \right)\left( {1 - f} \right)\frac{B}{2}} \right) + {h_{{i1}}}\left( {1 - f} \right)\frac{B}{2}} \right)}^2} - } \hfill \\ {4{k_i}\left( {1 - f} \right)\frac{B}{2}{b_i}{h_{{i1}}}\left( {f\frac{{B{N_i}}}{{{N_1} + {N_2}}} + \left( {1 + {r_{{ - i}}}} \right)\left( {1 - f} \right)\frac{B}{2}} \right) - {c_i}4{k_i}\left( {1 - f} \right)\frac{B}{2}{b_i}{h_{{i1}}}\left( {f\frac{{B{N_i}}}{{{N_1} + {N_2}}} + \left( {1 + {r_{{ - i}}}} \right)\left( {1 - f} \right)\frac{B}{2}} \right)} \hfill \\ { = {{\left( {{b_i}{k_i}\left( {f\frac{{B{N_i}}}{{{N_1} + {N_2}}} + \left( {1 + {r_{{ - i}}}} \right)\left( {1 - f} \right)\frac{B}{2}} \right) - {h_{{i1}}}\left( {1 - f} \right)\frac{B}{2}} \right)}^2} + {c_i}^2{k_i}^2{{\left( {f\frac{{B{N_i}}}{{{N_1} + {N_2}}} + \left( {1 + {r_{{ - i}}}} \right)\left( {1 - f} \right)\frac{B}{2}} \right)}^2} + } \hfill \\ {2{c_i}{k_i}\left( {f\frac{{B{N_i}}}{{{N_1} + {N_2}}} + \left( {1 + {r_{{ - i}}}} \right)\left( {1 - f} \right)\frac{B}{2}} \right){h_{{i1}}}\left( {1 - f} \right)\frac{B}{2} + 2{b_i}{c_i}{k_i}^2{{\left( {f\frac{{B{N_i}}}{{{N_1} + {N_2}}} + \left( {1 + {r_{{ - i}}}} \right)\left( {1 - f} \right)\frac{B}{2}} \right)}^2} - } \hfill \\ {{c_i}4{k_i}\left( {1 - f} \right)\frac{B}{2}{b_i}{h_{{i1}}}\left( {f\frac{{B{N_i}}}{{{N_1} + {N_2}}} + \left( {1 + {r_{{ - i}}}} \right)\left( {1 - f} \right)\frac{B}{2}} \right)} \hfill \\ } $$

On solving,

$$ \matrix{{*{20}{c}} { = {{\left( {{b_i}{k_i}\left( {f\frac{{B{N_i}}}{{{N_1} + {N_2}}} + \left( {1 + {r_{{ - i}}}} \right)\left( {1 - f} \right)\frac{B}{2}} \right) - {h_{{i1}}}\left( {1 + {r_{{ - i}}}} \right)\left( {1 - f} \right)\frac{B}{2}} \right)}^2}} \hfill \\ { + {c_i}{k_i}\left( {f\frac{{B{N_i}}}{{{N_1} + {N_2}}} + \left( {1 + {r_{{ - i}}}} \right)\left( {1 - f} \right)\frac{B}{2}} \right)\left( {\left( {{c_i}{k_i} + 2{b_i}{k_i}} \right)\left( {f\frac{{B{N_i}}}{{{N_1} + {N_2}}} + \left( {1 + {r_{{ - i}}}} \right)\left( {1 - f} \right)\frac{B}{2}} \right) + 2{h_{{i1}}}\left( {1 - f} \right)\frac{B}{2}\left( {1 - 2{b_i}} \right)} \right) > 0} \hfill \\ } $$

Thus, Eq. (11) has two positive real roots, $r_i^l$ and $r_i^u$, defined by,

$$ r_i^j = \frac{{ - {b_q}\pm \sqrt {{{b_q}^2 - 4{a_q}{c_q}}} }}{{2{a_q}}},j = u,l,i = 1,2 $$

(12)

Since $b_q^2 > b_q^2 - 4{a_q}{c_q}$, hence, we obtain, $\left| {{b_q}} \right| > \sqrt {{b_q^2 - 4{a_q}{c_q}}}$ Since,

$$ \matrix{{*{20}{c}} {{b_q} < 0 \Rightarrow \left| {{b_q}} \right| = - {b_q}} \hfill \\ { - {b_q} > \sqrt {{b_q^2 - 4{a_q}{c_q}}} } \hfill \\ { - {b_q} - \sqrt {{b_q^2 - 4{a_q}{c_q}}} > 0} \hfill \\ {\frac{{ - {b_q} - \sqrt {{b_q^2 - 4{a_q}{c_q}}} }}{{2{a_q}}} > 0,\,{\text{because}},\,{a_q} > 0} \hfill \\ } $$

Thus, $r_i^l > 0$ and by definition $0 < r_i^l < r_i^u$. Further, $r_i^u > 1$ since $- \frac{{{b_q}}}{{2{a_q}}} > 1$ as shown below.Since h _i1 ≈ 0.001 and k _i  ≈ .0001. Clearly, h _i1 > 2k _i implies that $- \frac{{{b_q}}}{{2{a_q}}} > 1$.Therefore, clearly, $r_i^u > 1$.

We next show that if ${b_i} > \frac{{{c_i}{k_i}}}{{{h_{{i1}}} - {k_i}}},r_i^l > {r_{{t - i}}}$, and f > f _ti then $r_i^l \geqslant 1$, where $0 < {r_{{t - i}}} < 1$.Let $f > {f_{{ti}}} = \frac{{\frac{{\left( {{h_{{i1}}} - {k_i}} \right)\left( {1 - 2{b_i}} \right) + {c_i}{k_i}}}{{{b_i}\left( {{h_{{i1}}} - {k_i}} \right) - {c_i}{k_i}}}}}{{\frac{{2{N_i}}}{{{N_1} + {N_2}}} + \frac{{\left( {{h_{{i1}}} - {k_i}} \right)\left( {1 - 2{b_i}} \right) + {c_i}{k_i}}}{{{b_i}\left( {{h_{{i1}}} - {k_i}} \right) - {c_i}{k_i}}}}}$, where $\left( {\frac{{2{N_i}}}{{{N_1} + {N_2}}} + \frac{{\left( {1 - 2{b_i}} \right)\left( {{h_{{i1}}} - {k_i}} \right) + {c_i}{k_i}}}{{{b_i}\left( {{h_{{i1}}} - {k_i}} \right) - {c_i}{k_i}}}} \right) > 0$ since

$$ \matrix{{*{20}{c}} {{b_i} > \frac{{{c_i}{k_i}}}{{{h_{{i1}}} - {k_i}}}.} \hfill \\ { \Rightarrow f\left( {\frac{{2{N_i}}}{{{N_1} + {N_2}}} + \frac{{\left( {1 - 2{b_i}} \right)\left( {{h_{{i1}}} - {k_i}} \right) + {c_i}{k_i}}}{{{b_i}\left( {{h_{{i1}}} - {k_i}} \right) - {c_i}{k_i}}}} \right) > \frac{{\left( {1 - 2{b_i}} \right)\left( {{h_{{i1}}} - {k_i}} \right) + {c_i}{k_i}}}{{{b_i}\left( {{h_{{i1}}} - {k_i}} \right) - {c_i}{k_i}}}} \hfill \\ { \Rightarrow f\left( {\frac{{2{N_i}}}{{{N_1} + {N_2}}} + \frac{{\left( {{h_{{i1}}} - {k_i}} \right)\left( {1 - {b_i}} \right)}}{{{b_i}\left( {{h_{{i1}}} - {k_i}} \right) - {c_i}{k_i}}} - 1} \right) > \frac{{\left( {{h_{{i1}}} - {k_i}} \right)\left( {1 - {b_i}} \right)}}{{{b_i}\left( {{h_{{i1}}} - {k_i}} \right) - {c_i}{k_i}}} - 1} \hfill \\ { \Rightarrow \frac{{\left( {{h_{{i1}}} - {k_i}} \right)\left( {1 - {b_i}} \right)}}{{{b_i}\left( {{h_{{i1}}} - {k_i}} \right) - {c_i}{k_i}}} < 1 + \frac{{2f{N_i}}}{{\left( {{N_1} + {N_2}} \right)\left( {1 - f} \right)}}} \hfill \\ { \Rightarrow {r_{{t - i}}} < 1.} \hfill \\ } $$

(13)

Since, ${b_i} > \frac{{{c_i}{k_i}}}{{{h_{{i1}}} - {k_i}}}$, clearly, ${r_{{t - i}}} > 0$.Let

$$ r_{{ - i}}^l \geqslant {r_{{t - i}}} = \frac{{\left( {{c_i}{k_i} - {b_i}\left( {{h_{{i1}}} - {k_i}} \right)} \right)\frac{{fB{N_i}}}{{{N_1} + {N_2}}} + \left( {{h_{{i1}}} - {k_i}} \right)\left( {1 - f} \right)\frac{B}{2}\left( {1 - {b_i}} \right)}}{{\left( {1 - f} \right)\frac{B}{2}\left( {{b_i}\left( {{h_{{i1}}} - {k_i}} \right) - {c_i}{k_i}} \right)}} $$

(14)

On rearranging the terms,

$$ \matrix{{*{20}{c}} { \Rightarrow \left( {{h_{{i1}}} + \left( {{b_i} + {c_i}} \right)\left( {1 + {r_{{ - i}}}} \right){k_i}} \right)\left( {1 - f} \right)\frac{B}{2} + {k_i}\left( {1 + {c_i}} \right)\left( {1 - f} \right)\frac{B}{2} + \left( {{b_i} + {c_i}} \right){k_i}f\frac{{B{N_i}}}{{{N_1} + {N_2}}} + {b_i}{h_{{i1}}}\left( {\left( {1 + {r_{{ - i}}}} \right)\left( {1 - f} \right)\frac{B}{2} + f\frac{{B{N_i}}}{{{N_1} + {N_2}}}} \right) \geqslant 0} \hfill \\ { \Rightarrow {a_q} - \left( { - {b_q}} \right) + {c_q} \geqslant 0} \hfill \\ { \Rightarrow - 4a_q^2 + 4{a_q}\left( { - {b_q}} \right) + {{\left( { - {b_q}} \right)}^2} - 4{a_q}{c_q} \geqslant {{\left( { - {b_q}} \right)}^2}} \hfill \\ { \Rightarrow {{\left( { - {b_q} - 2{a_q}} \right)}^2} \geqslant {b_q}^2 - 4{a_q}{c_q}} \hfill \\ } $$

We next show that $- {b_q} - {2}{a_q} > 0$.

Clearly, $2\left( {1 + {c_i}} \right) < \frac{{{h_{{i1}}}}}{{{k_i}}}$ since ${a_i} + {b_i} + {c_i} = {1}$ and h _i1 ≈ 0.001and k _i  ≈ .0001.

$$ \left( {2\left( {1 + {c_i}} \right) - \frac{{{h_{{i1}}}}}{{{k_i}}}} \right)\frac{1}{{\left( {{b_i} + {c_i}} \right)}} - 1 - \frac{{2f{N_i}}}{{{N_1} + {N_2}}} < 0 $$

Clearly, $r_{{ - i}}^l > \left( {2\left( {1 + {c_i}} \right) - \frac{{{h_{{i1}}}}}{{{k_i}}}} \right)\frac{1}{{\left( {{b_i} + {c_i}} \right)}} - 1 - \frac{{2f{N_i}}}{{{N_1} + {N_2}}}$

$$ \matrix{{*{20}{c}} { \Rightarrow \left( {1 + {r_{{ - i}}}} \right)\left( {{b_i} + {c_i}} \right){k_i}\left( {1 - f} \right)\frac{B}{2} + {h_{{i1}}}\left( {1 - f} \right)\frac{B}{2} > {k_i}\left( {1 + {c_i}} \right)\left( {1 - f} \right)B - \left( {{b_i} + {c_i}} \right){k_i}f\frac{{B{N_i}}}{{{N_1} + {N_2}}}} \hfill \\ { \Rightarrow \left( {{b_i} + {c_i}} \right){k_i}f\frac{{B{N_i}}}{{{N_1} + {N_2}}} + \left( {{h_{{i1}}} + \left( {{b_i} + {c_i}} \right)\left( {1 + {r_{{ - i}}}} \right){k_i}} \right)\left( {1 - f} \right)\frac{B}{2} - {k_i}\left( {1 + {c_i}} \right)\left( {1 - f} \right)B > 0} \hfill \\ { \Rightarrow - {b_q} - 2{a_q} > 0.} \hfill \\ } $$

Thus, ${\left( { - {b_q} - 2{a_q}} \right)^2} \geqslant {b_q}^2 - 4{a_q}{c_q}$

$$ \matrix{{*{20}{c}} { \Rightarrow - {b_q} - \sqrt {{{b_q}^2 - 4{a_q}{c_q}}} \geqslant 2{a_q}} \hfill \\ { \Rightarrow r_i^l \geqslant 1} \hfill \\ } $$

(15)

Thus, if ${b_i} > \frac{{{c_i}{k_i}}}{{{h_{{i1}}} - {k_i}}},r_i^l > {r_{{t - i}}}$, and f > f _ti then $r_i^l > = 1$. On reversing the inequality in (13), if ${b_i} > \frac{{{c_i}{k_i}}}{{{h_{{i1}}} - {k_i}}}$, and f ≤ f _ti then $r_i^l < 1$. If ${b_i} \leqslant \frac{{{c_i}{k_i}}}{{{h_{{i1}}} - {k_i}}}$ then r _t−i  < 0, therefore, clearly from (14) and (15), $r_i^l < 1$. Hence, we obtain conditions specified in Table 1.

1.3 A.3. Proposition 2: For problem IA with the three sub-populations, Table 2 specifies the optimal f and IA value for the conditions specified in Table 1

Proof

The upper-level utility function is $IA = \sum\limits_{{i = 1}}^2 {I{A_i}}$

$$ = B\sum\limits_{\begin{subarray} i, - i = 1 \\ i \ne - i \end{subarray} }^2 {\left[ {\frac{{\left( {1 - r_i^l + r_{{ - i}}^l} \right)\left( {1 - f} \right)B}}{2} + \frac{{fB{N_i}}}{{{N_i} + {N_{{ - i}}}}}} \right]\left( {{h_i}_1 - r_i^l{k_i}} \right)} $$

We next solve the upper-level problem.

$$ IA:\mathop{{\max }}\limits_f \sum\limits_{{i = 1}}^2 {I{A_i}\left( {r_i^l*(f)} \right)}, i = 1,2, $$

(16)

$$ {\text{s}}{\text{.t}}{.}\,{0} \leqslant f \leqslant 1 $$

(17)

$$ r_i^l*(f) = \arg \,\max \left( {{L_i}\left( {r_i^l} \right)} \right) $$

(18)

For the Nash equilibrium, (1,1), clearly, IA is a decreasing function in f. Thus, from Table 1, if ${b_i} > \frac{{{c_i}{k_i}}}{{{h_{{i1}}} - {k_i}}},i = 1,2$ then $f* = \max \left\{ {{f_{{t1}}},{f_{{t2}}}} \right\}$ is optimal, resulting in ${r_i}* = 1$ at the lower level and $IA_M^{*}\left( {r_1^{*},r_1^{*}} \right) = \frac{B}{2}\left( {{h_{{11}}} - {k_1} + {h_{{21}}} - {k_2}} \right)$.

For the Nash equilibrium, $\left( {r_1^l,r_2^l} \right)$, first derivative of IA with respect to f is given below:

$$ \frac{{dIA}}{{df}} = \frac{{\partial IA}}{{\partial f}} + \frac{{\partial IA}}{{\partial r_1^l}} \cdot \frac{{\partial r_1^l}}{{\partial f}} + \frac{{\partial IA}}{{\partial r_2^l}} \cdot \frac{{\partial r_2^l}}{{\partial f}} \kern3pt \cdot$$

Clearly, the total number of infections prevented decrease with the increase in the preferences $\left( {r_1^l,r_2^l} \right)$ of the LDs to allocate proportionally plus an increase in the fixed budget (budget to be allocated in proportion to the number of HIV/AIDS infections). That is, IA is decreasing in f, $r_1^l$, and $r_2^l,\frac{{\partial IA}}{{\partial f}} < 0,\frac{{\partial IA}}{{\partial r_1^l}} < 0,\,{\text{and}}\,\frac{{\partial IA}}{{\partial r_2^l}} < 0$. Based on the literature, the preferences $\left( {r_1^l,r_2^l} \right)$ of the LDs to allocate proportionally increase with the increase in the fixed budget (increase in f). That is, $r_1^l,r_2^l$ is increasing in f. Therefore, IA is decreasing in f. Thus, we obtain optimal f * and IA* specified in Table 2, based on conditions of Table 1.