Analysis of a nonlinear crack in a piezoelectric half-space via displacement discontinuity method

Abstract

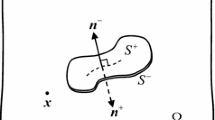
In this paper, we derive the exact closed-form fundamental solutions due to uniform extended displacement discontinuities over a triangular element in a piezoelectric half-space. Using the triangular elements to partition the penny-shaped crack, the triangular element fundamental solutions are verified by comparing with the existing analytical solution associated with the penny-shaped crack. The polarization saturation model is then applied to an elliptical crack in the piezoelectric half-space, and the resulting nonlinear fracture problem is solved by combing the triangular element fundamental solutions and the displacement discontinuity method. The electric yielding zone and the extended field intensity factors are obtained by an iterative approach. The effects of the applied mechanical load and electric displacement, the polarization saturation in the yielding zone, and the aspect ratio of the elliptical crack on the yielding zone size and field intensity factors are discussed through numerical examples.

Appendices

Appendix 1

The coefficients $L_{f} ,\;L_{h} ,\;L_{fk}^{i} ,\;L_{hk}^{ij} ,\;L_{fk\alpha }^{i}$ and $\;L_{hk\alpha }^{ij} (i, j=1, 2, 3, k=1, 2, 3, \alpha =1, 2)$ in Eqs. (3a), (3b), (3c) and (3d) are listed below:

$$\begin{aligned}&L_{f} =c_{44} \omega _{41} s_{4} D_{4} ,\quad L_{h} =c_{44} \omega _{41} s_{4} D_{44} ,\\&L_{f1}^{i} =\omega _{i1} (c_{44} A_{i} +c_{44} D_{i} s_{i} -e_{15} B_{i} ),\\&L_{f2}^{i} =\omega _{i1} (c_{33} A_{i} -e_{33} B_{i} s_{i} -c_{13} D_{i} ), \\&L_{f3}^i =\omega _{i1} (e_{33} A_{i} s_{i} +\varepsilon _{33} B_{i} s_{i} -e_{31} D_{i} ),\\&L_{h1}^{ij} =\omega _{i1} (c_{44} A_{ij} +c_{44} D_{ij} s_{i} -e_{15} B_{ij} ),\\&L_{h2}^{ij} =\omega _{i1} (c_{33} A_{ij} -e_{33} B_{ij} s_{i} -c_{13} D_{ij} ),\\&L_{h3}^{ij} =\omega _{i1} (e_{33} A_{i} s_{i} +\varepsilon _{33} B_{i} s_{i} -e_{31} D_{i} ), \\&L_{f11}^{i} =\vartheta _{i1} (c_{44} A_{i} +c_{44} D_{i} s_{i} -e_{15} B_{i} ),\\&L_{f12}^{i} =\vartheta _{i2} (c_{44} A_{i} +c_{44} D_{i} s_{i} -e_{15} B_{i} ), \\&L_{f21}^{i} =\vartheta _{i1} (c_{33} A_{i} -e_{33} B_{i} s_{i} -c_{13} D_{i} ),\\&L_{f22}^{i} =\vartheta _{i2} (c_{33} A_{i} -e_{33} B_{i} s_{i} -c_{13} D_{i} ), \\&L_{f31}^{i} =\vartheta _{i1} (e_{33} A_{i} s_{i} +\varepsilon _{33} B_{i} s_{i} -e_{31} D_{i} ),\\&L_{f32}^{i} =\vartheta _{i2} (e_{33} A_{i} s_{i} +\varepsilon _{33} B_{i} s_{i} -e_{31} D_{i} ), \\&L_{h11}^{ij} =\vartheta _{i1} (c_{44} A_{ij} +c_{44} D_{ij} s_{i} -e_{15} B_{ij} ),\\&L_{h12}^{ij} =\vartheta _{i2} (c_{44} A_{ij} +c_{44} D_{ij} s_{i} -e_{15} B_{ij} ), \\&L_{h21}^{ij} =\vartheta _{i1} (c_{33} A_{ij} -e_{33} B_{ij} s_{i} -c_{13} D_{ij} ),\\&L_{h22}^{ij} =\vartheta _{i2} (c_{33} A_{ij} -e_{33} B_{ij} s_{i} -c_{13} D_{ij} ),\\&L_{h31}^{ij} =\vartheta _{i1} (e_{33} A_{ij} s_{i} +\varepsilon _{33} B_{ij} s_{i} -e_{31} D_{ij} ),\\&L_{h32}^{ij} =\vartheta _{i2} (e_{33} A_{ij} s_{i} +\varepsilon _{33} B_{ij} s_{i} -e_{31} D_{ij} ), \end{aligned}$$

where $s_{i}$ are the eigenvalues, $c_{ij}, e_{ij}, \varepsilon _{ij}$ are the elastic constants, PE constants and dielectric constants; $A_{i }(A_{ij}), B_{i }(B_{ij}), D_{i }(D_{ij}), \omega _{i1}, \vartheta _{i1}$ and $\vartheta _{i2}$ are the material-related constants listed in Ding et al. (1997). Furthermore, the subscripts f and h indicate the parameters associated with full- and half-spaces respectively.

Appendix 2

The coefficients in Eqs. (6a), (6b), (6c) and (6d) are listed below (for $k=1-5$ and $i=1-3$):

$$\begin{aligned} M_{fk}^{i} =\sum _{l=1}^{6} {N_{kl}^{i} } , \end{aligned}$$

(27)

where the subscript f on the left-hand side of Eq. (27) indicates the full-space parameters, and

$$\begin{aligned} N_{11}^{i}= & {} \frac{(y_{1} -y_{2} ) \left[ {(y-y_{1} )(x(y_{2} -y_{1} )+y(x_{1} -x_{2} )+(x_{2} y_{1} -x_{1} y_{2} ))+(x_{1} -x_{2} ) \bar{{z}}_{i}^{2} } \right] }{G_{1}^{i} },\nonumber \\ N_{12}^{i}= & {} \frac{(y_{1} -y_{3} ) \left[ {(y-y_{1} )(x(y_{1} -y_{3} )+y(x_{3} -x_{1} )+(x_{1} y_{3} -x_{3} y_{1} )) +(x_{3} -x_{1} )\bar{{z}}_{i}^{2} } \right] }{G_{2}^{i} },\nonumber \\ N_{13}^{i}= & {} \frac{(y_{2} -y_{1} )\left[ {(y-y_{2} ) (x(y_{2} -y_{1} )+y(x_{1} -x_{2} )+(x_{2} y_{1} -x_{1} y_{2} )) +(x_{1} -x_{2} )\bar{{z}}_{i}^{2}} \right] }{G_{3}^{i}},\nonumber \\ N_{14}^{i}= & {} \frac{(y_{2} -y_{3} ) \left[ {(y-y_{2} )(x(y_{3} -y_{2} )+y(x_{2} -x_{3} )+(x_{3} y_{2} -x_{2} y_{3} )) +(x_{2} -x_{3} )\bar{{z}}_{i}^{2}} \right] }{G_{4}^{i}},\nonumber \\ N_{15}^{i}= & {} \frac{(y_{3} -y_{1} ) \left[ {(y-y_{3})(x(y_{1} -y_{3} ) +y(x_{3} -x_{1} )+(x_{1} y_{3} -x_{3} y_{1} )) +(x_{3} -x_{1} )\bar{{z}}_{i}^{2} } \right] }{G_{5}^{i} },\nonumber \\ N_{16}^{i}= & {} \frac{(y_{3} -y_{2} ) \left[ {(y-y_{3} )(x(y_{3} -y_{2} )+y(x_{2} -x_{3} )+(x_{3} y_{2} -x_{2} y_{3} )) +(x_{2} -x_{3} )\bar{{z}}_{i}^{2} } \right] }{G_{6}^{i} }, \end{aligned}$$

(28)

$$\begin{aligned} N_{21}^{i}= & {} \frac{(x_1 -x_2 )\left[ {(x-x_1 )(x(y_2 -y_1 )+y(x_1 -x_2 )+(x_2 y_1 -x_1 y_2 ))+(y_1 -y_2 )\bar{{z}}_i^2 } \right] }{G_1^i }, \nonumber \\ N_{22}^{i}= & {} \frac{(x_{1} -x_{3} )\left[ {(x-x_{1} ) (x(y_{1} -y_{3} )+y(x_{3} -x_{1} )+(x_{1} y_{3} -x_{3} y_{1} )) +(y_{1} -y_{3} ) \bar{{z}}_{i}^{2} } \right] }{G_{2}^{i} },\nonumber \\ N_{23}^{i}= & {} \frac{(x_{2} -x_{1}) \left[ {(x-x_{2} )(x(y_{2} -y_{1} )+y(x_{1} -x_{2} )+(x_{2} y_{1} -x_{1} y_{2} ))+(y_{2} -y_{1} )\bar{{z}}_{i}^{2}} \right] }{G_{3}^{i}},\nonumber \\ N_{24}^i= & {} \frac{(x_{2} -x_{3} ) \left[ {(x-x_{2} )(x(y_{3} -y_{2} )+y(x_{2} -x_{3} )+(x_{3} y_{2} -x_{2} y_{3} ))+(y_{2} -y_{3} ) \bar{{z}}_{i}^{2} } \right] }{G_{4}^{i} },\nonumber \\ N_{25}^{i}= & {} \frac{(x_{3} -x_{1} ) \left[ {(x-x_{3} )(x(y_{1} -y_{3} )+y(x_{3} -x_{1} )+(x_{1} y_{3} -x_{3} y_{1} )) +(y_{3} -y_{1} )\bar{{z}}_{i}^{2} } \right] }{G_{5}^{i} },\nonumber \\ N_{26}^{i}= & {} \frac{(x_{3} -x_{2} ) \left[ {(x-x_{3} )(x(y_{3} -y_{2} )+y(x_{2} -x_{3} )+(x_{3} y_{2} -x_{2} y_{3}))+(y_{3} -y_{2} ) \bar{{z}}_{i}^{2}} \right] }{G_{6}^{i}}, \end{aligned}$$

(29)

$$\begin{aligned} N_{31}^{i}= & {} \frac{(x_{1} -x_{2} )\left[ {(y-y_{1} ) (x(y_{2} -y_{1} )+y(x_{1} -x_{2} )+(x_{2} y_{1} -x_{1} y_{2} )) +(x_{1} -x_{2} )\bar{{z}}_{i}^{2}} \right] }{G_{1}^{i} },\nonumber \\ N_{32}^{i}= & {} \frac{(x_{1} -x_{3} )\left[ {(y-y_{1} ) (x(y_{1} -y_{3} )+y(x_{3} -x_{1} )+(x_{1} y_{3} -x_{3} y_{1} ))+(x_{3} -x_{1} )\bar{{z}}_{i}^{2} } \right] }{G_{2}^{i} },\nonumber \\ N_{33}^{i}= & {} \frac{(x_{2} -x_{1} ) \left[ {(y-y_{2} )(x(y_{2} -y_{1} )+y(x_{1} -x_{2} )+(x_{2} y_{1} -x_{1} y_{2} )) +(x_{1} -x_{2} )\bar{{z}}_{i}^{2} } \right] }{G_{3}^{i} },\nonumber \\ N_{34}^{i}= & {} \frac{(x_{2} -x_{3} ) \left[ {(y-y_{2} )(x(y_{3} -y_{2} )+y(x_{2} -x_{3} )+(x_{3} y_{2} -x_{2} y_{3} )) +(x_{2} -x_{3})\bar{{z}}_{i}^{2} } \right] }{G_{4}^{i}},\nonumber \\ N_{35}^{i}= & {} \frac{(x_{3} -x_{1} )\left[ {(y-y_{3} ) (x(y_{1} -y_{3} )+y(x_{3} -x_{1} )+(x_{1} y_{3} -x_{3} y_{1} ))+(x_{3} -x_{1} )\bar{{z}}_{i}^{2} } \right] }{G_5^i },\nonumber \\ N_{36}^{i}= & {} \frac{(x_{3} -x_{2} )\left[ {(y-y_{3} ) (x(y_{3} -y_{2} )+y(x_{2} -x_{3} )+(x_{3} y_{2} -x_{2} y_{3} )) +(x_{2} -x_{3} )\bar{{z}}_{i}^{2} } \right] }{G_{6}^{i}}, \end{aligned}$$

(30)

$$\begin{aligned} N_{41}^{i}= & {} \frac{\bar{{z}}_{i} (y_{1} -y_{2} ) \left[ {(x-x_{1} )(x_{2} -x_{1} )+(y-y_{1} )(y_{2} -y_{1} )} \right] }{G_{1}^{i} }, \nonumber \\ N_{42}^{i}= & {} \frac{\bar{{z}}_{i} (y_{1} -y_{3} ) \left[ {(x-x_{1} )(x_{1} -x_{3} )+(y-y_{1} )(y_{1} -y_{3} )} \right] }{G_{2}^{i} }, \nonumber \\ N_{43}^{i}= & {} \frac{\bar{{z}}_{i} (y_{2} -y_{1}) \left[ {(x-x_{2} )(x_{2} -x_{1} )+(y-y_{2} )(y_{2} -y_{1} )} \right] }{G_{3}^{i} }, \nonumber \\ N_{44}^{i}= & {} \frac{\bar{{z}}_{i} (y_{2} -y_{3} ) \left[ {(x-x_{2} )(x_{3} -x_{2} )+(y-y_{2} )(y_{3} -y_{2} )} \right] }{G_{4}^{i} }, \nonumber \\ N_{45}^{i}= & {} \frac{\bar{{z}}_{i} (y_{3} -y_{1} ) \left[ {(x-x_{3} )(x_{1} -x_{3} )+(y-y_{3} )(y_{1} -y_{3} )} \right] }{G_{5}^{i} }, \nonumber \\ N_{46}^{i}= & {} \frac{\bar{{z}}_{i} (y_{3} -y_{2} ) \left[ {(x-x_{3} )(x_{3} -x_{2} )+(y-y_{3} )(y_{3} -y_{2} )} \right] }{G_{6}^{i}}, \end{aligned}$$

(31)

$$\begin{aligned} N_{51}^{i}= & {} \frac{\bar{{z}}_{i} (x_{1} -x_{2}) \left[ {(x-x_{1} )(x_{2} -x_{1} )+(y-y_{1} )(y_{2} -y_{1} )} \right] }{G_{1}^{i} }, \nonumber \\ N_{52}^{i}= & {} \frac{\bar{{z}}_{i} (x_{1} -x_{3} ) \left[ {(x-x_{1} )(x_{1} -x_{3} )+(y-y_{1} )(y_{1} -y_{3} )} \right] }{G_{2}^{i} }, \nonumber \\ N_{53}^{i}= & {} \frac{\bar{{z}}_{i} (x_{2} -x_{1} ) \left[ {(x-x_{2} )(x_{2} -x_{1} )+(y-y_{2} )(y_{2} -y_{1} )} \right] }{G_{3}^{i} }, \nonumber \\ N_{54}^{i}= & {} \frac{\bar{{z}}_{i} (x_{2} -x_{3} ) \left[ {(x-x_{2} )(x_{3} -x_{2} )+(y-y_{2})(y_{3} -y_{2})} \right] }{G_{4}^{i}}, \nonumber \\ N_{55}^{i}= & {} \frac{\bar{{z}}_{i} (x_{3} -x_{1} ) \left[ {(x-x_{3} )(x_{1} -x_{3} )+(y-y_{3} )(y_{1} -y_{3} )} \right] }{G_{5}^{i} }, \nonumber \\ N_{56}^{i}= & {} \frac{\bar{{z}}_{i} (x_{3} -x_{2} ) \left[ {(x-x_{3} )(x_{3} -x_{2} )+(y-y_{3} )(y_{3} -y_{2} )} \right] }{G_{6}^{i} }, \end{aligned}$$

(32)

In Eqs. (28)–(32),

$$\begin{aligned} G_{1}^{i}= & {} \sqrt{(x-x_{1} )^{2}+(y-y_{1} )^{2}+\bar{{z}}_{i}^{2}}\nonumber \\&\times \left[ {\begin{array}{l} (x(y_{2} -y_{1} )+y(x_{1} -x_{2} )+(x_{2} y_{1} -x_{1} y_{2} ))^{2} \\ \quad +\left( {(x_{1} -x_{2})^{2}+(y_{1} -y_{2} )^{2}} \right) \bar{{z}}_{i}^{2} \\ \end{array}} \right] , \nonumber \\ G_{2}^{i}= & {} \sqrt{(x-x_{1} )^{2}+(y-y_{1} )^{2}+\bar{{z}}_{i}^{2}}\nonumber \\&\times \left[ {\begin{array}{l} \left( {x(y_{1} -y_{3} )+y(x_{3} -x_{1}) +(x_{1} y_{3} -x_{3} y_{1} )} \right) ^{2} \\ \quad +\left( {(x_{1} -x_{3})^{2}+(y_{1} -y_{3})^{2}} \right) \bar{{z}}_{i}^{2}\\ \end{array}} \right] , \nonumber \\ G_{3}^{i}= & {} \sqrt{(x-x_{2} )^{2}+(y-y_{2})^{2}+\bar{{z}}_{i}^{2}}\nonumber \\&\times \left[ {\begin{array}{l} \left( {x(y_{2} -y_{1} )+y(x_{1} -x_{2} ) +(x_{2} y_{1} -x_{1} y_{2} )} \right) ^{2} \\ \quad +\left( {(x_{1} -x_{2} )^{2}+(y_{1} -y_{2})^{2}} \right) \bar{{z}}_{i}^{2} \\ \end{array}} \right] , \nonumber \\ G_{4}^{i}= & {} \sqrt{(x-x_{2} )^{2}+(y-y_{2})^{2}+\bar{{z}}_{i}^{2}}\nonumber \\&\times \left[ {\begin{array}{l} \left( {x(y_{3} -y_{2} )+y(x_{2} -x_{3} ) +(x_{3} y_{2} -x_{2} y_{3} )} \right) ^{2}\\ \quad +\left( {(x_{3} -x_{2} )^{2}+(y_{3} -y_{2} )^{2}} \right) \bar{{z}}_{i}^{2} \\ \end{array}} \right] , \nonumber \\ G_{5}^{i}= & {} \sqrt{(x-x_{3})^{2}+(y-y_{3})^{2}+\bar{{z}}_{i}^{2} }\nonumber \\&\times \left[ {\begin{array}{l} \left( {x(y_{1} -y_{3} )+y(x_{3} -x_{1} )+ (x_{1} y_{3} -x_{3} y_{1} )} \right) ^{2}\\ \quad +\left( {(x_{1} -x_{3} )^{2}+(y_{1} -y_{3} )^{2}} \right) \bar{{z}}_{i}^{2} \\ \end{array}} \right] , \nonumber \\ G_{6}^{i}= & {} \sqrt{(x-x_{3})^{2}+(y-y_{3})^{2}+\bar{{z}}_{i}^{2}}\nonumber \\&\times \left[ {\begin{array}{l} \left( {x(y_{3} -y_{2} )+y(x_{2} -x_{3} ) +(x_{3} y_{2} -x_{2} y_{3} )} \right) ^{2}\\ \quad +\left( {(x_{2} -x_{3} )^{2}+(y_{2} -y_{3} )^{2}} \right) \bar{{z}}_{i}^{2} \\ \end{array}} \right] . \end{aligned}$$

(33)

We point out that by replacing $\bar{{z}}_{i}$ in $M_{fk}^{i}$ by $z_{ij} (i,j=1,2,3$), one obtains the expressions for $M_{hk}^{ij}$ where the subscript h denotes the parameters of the half-space. We further point out that the parameters $\bar{{z}}_{i}$ and $z_{ij}$ are defined in Eq. (4).