Local Supermajorities

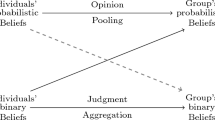
Abstract

This paper explores two non-standard supermajority rules in the context of judgment aggregation over multiple logically connected issues. These rules set the supermajority threshold in a local, context sensitive way—partly as a function of the input profile of opinions. To motivate the interest of these rules, I prove two results. First, I characterize each rule in terms of a condition I call ‘Block Preservation’. Block preservation says that if a majority of group members accept a judgment set, then so should the group. Second, I show that one of these rules is, in a precise sense, a judgment aggregation analogue of a rule for connecting qualitative and quantitative belief that has been recently defended by Hannes Leitgeb. The structural analogy is due to the fact that Leitgeb sets thresholds for qualitative beliefs in a local, context sensitive way—partly as a function of the given credence function.

Appendix: Proofs of theorems

Proof of theorem 1

Suppose ${\mathbf{A}}$ and $\vec {J}$ are respectively an arbitrary agenda and an arbitrary profile. First, one can easily check that ${\mathbf{LS}}^{-}$ satisfies the three characterizing properties. Second, let F be an arbitrary rule that satisfies the three characterizing properties. We prove that $\forall \varphi \in {\mathbf{A}}, \varphi \in {\mathbf{LS}}^{-}(\vec {J})\Leftrightarrow \varphi \in {\mathbf{F}}(\vec {J})$.

$[\Rightarrow ]$: suppose $\varphi \in {\mathbf{LS}}^{-}(\vec {J})$. Then there is a threshold $t > 0.5$ with ${\texttt{cons}}(\vec {J}, t)={\mathbf{LS}}^{-}(\vec {J})$ and so $\varphi \in {\texttt{cons}}(\vec {J},t)$. We show that ${\texttt{cons}}(\vec {J},t)$ is a set of sentences that meets the conditions in the antecedent of strong block preservation, instantiating the quantifiers with profile $\vec {J}$, agenda A and threshold t. Because F satisfies strong block preservation, establishing this implies ${\texttt{cons}}(\vec {J},t)\subseteq {\mathbf{F}}(\vec {J})$ and so $\varphi \in {\mathbf{F}}(\vec {J})$.

Obviously: ${\texttt{cons}}(\vec {J},t) \subseteq {\mathbf{A}}$. For conditions (i) and (ii), it is also obvious that ${\texttt{cons}}(\vec {J},t)$ is consistent (${\texttt{cons}}$-sets must be consistent) and ${\texttt{support}}(\psi , \vec {J})\ge t$ for $\psi \in {\texttt{cons}}(\vec {J},t)$. Finally, to establish (iii), there cannot be any set T inconsistent with ${\texttt{cons}}(\vec {J},t)$ such that for all $\psi \in T, {\texttt{support}}(\psi , \vec {J})\ge t$. If there was, $T \subseteq {\texttt{cons}}(\vec {J}, t)$, which is impossible because ${\texttt{cons}}(\vec {J}, t)$ is consistent. Since the antecedent of strong block preservation is met, ${\texttt{cons}}(\vec {J},t) \subseteq {\mathbf{F}}(\vec {J})$, so $\varphi \in {\mathbf{F}}(\vec {J})$.

$[\Leftarrow ]$: suppose $\varphi \in {\mathbf{F}}(\vec {J})$. Because F is a uniform relaxed quota rule, given $\vec {J}$, there is a threshold $t_{\vec {J}}$ such that

$$ (\#) \forall \psi \,[\psi \in {\mathbf{F}}(\vec {J})\,{\text{iff }}\,{\texttt{support}}(\psi , \vec {J})\ge t_{\vec {J}}] $$

Fix some such $t_{\vec {J}}$. Note that by (#) and the fact that ${\mathbf{F}}(\vec {J})$ is consistent, ${\mathbf{F}}(\vec {J})={\texttt{cons}}(\vec {J},t_{\vec {J}})$. But we know ${\texttt{cons}}(\vec {J},t_{\vec {J}}) \subseteq {\mathbf{LS}}^{-}(\vec {J})$, so ${\mathbf{F}}(\vec {J}) \subseteq {\mathbf{LS}}^{-}(\vec {J})$, and hence $\varphi \in {\mathbf{LS}}^{-}(\vec {J})$.

Proof of theorem 2

First, we note that ${\mathbf{LS}}^{+}$ satisfies all of the characterizing conditions. As before, we prove that for all $\varphi \in {\mathbf{A}}, \varphi \in {\mathbf{LS}}^{+}(\vec {J}) \Leftrightarrow \varphi \in {\mathbf{F}}(\vec {J})$, where ${\mathbf{F}}$ is an arbitrary aggregation function satisfying the characterizing conditions.

$[\Rightarrow ]$: suppose $\varphi \in {\mathbf{LS}}^{+}(\vec {J})$. Then there is a threshold t such that ${\texttt{closed}}(\vec {J}, t)={\mathbf{LS}}^{+}(\vec {J})$ and so $\varphi \in {\texttt{closed}}(\vec {J}, t)$. To prove $\varphi \in {\mathbf{F}}(\vec {J})$, we check that ${\texttt{closed}}(\vec {J}, t)$ satisfies the conditions in the antecedent of intermediate block preservation. First, ${\texttt{closed}}(\vec {J}, t)$ must be a closed and consistent subset of ${\mathbf{A}}$, thus satisfying condition (i). Condition (ii) is satisfied by construction of ${\texttt{closed}}(\vec {J}, t)$. To check condition (iii), suppose there was a $\psi$ such that ${\texttt{closed}}(\vec {J}, t) \nvDash \psi$ and ${\texttt{support}}(\psi , \vec {J})\ge t$. This would imply $\psi \in {\texttt{closed}}(\vec {J}, t)$, which contradicts the claim that $\psi$ is not entailed by ${\texttt{closed}}(\vec {J}, t)$. By intermediate block preservation $\varphi \in {\mathbf{F}}(\vec {J})$.

$[\Leftarrow ]$: suppose $\varphi \in {\mathbf{F}}(\vec {J})$. We know that ${\mathbf{F}}(\vec {J})$ is consistent and closed. Because F is a uniform relaxed quota rule, given $\vec {J}$, there is a threshold $t_{\vec {J}}\,$ such that

$$ \varphi \in {\mathbf{F}}(\vec {J})\,{\text{iff }}\,{\texttt{support}}(\varphi, \vec {J})\ge t_{\vec {J}} $$

Fix some such $t_{\vec {J}}$. Because ${\mathbf{F}}(\vec {J})$ is consistent and closed, ${\mathbf{F}}(\vec {J})={\texttt{closed}}(\vec {J}, t_{\vec {J}}))$. Since, by definition, ${\texttt{closed}}(\vec {J}, t_{\vec {J}})) \subseteq {\mathbf{LS}}^{+}(\vec {J}), \varphi \in {\mathbf{LS}}^{+}(\vec {J})$.

Proof of Fact 1

We assume that that A is a $\sigma$-algebra and $\vec {J} \in {\mathbf{RP}}$. We check that $P^{\vec {J}}(\cdot )$, that is ${\texttt{support}}(\cdot , \vec {J})$, satisfies the Kolmogorov axioms.

Non-negativity:  For $p \in {\mathbf{A}}, P^{\vec {J}}(p)\ge 0$ follows from the definition of ${\texttt{support}}$.

Tautology:  For any tautology, $\top , P^{\vec {J}}(\top )=1$, because $\vec {J}$ is a rational profile, which implies consistent and complete relative to ${\mathbf{A}}$ ($\top \in {\mathbf{A}}$ because ${\mathbf{A}}$ is a $\sigma$-algebra).

Additivity: Suppose p and q are mutually exclusive. Let ${\texttt{support}}(p, \vec {J})=x$ and ${\texttt{support}}(q, \vec {J})=y$. It follows that ${\texttt{support}}(p \cup q, \vec {J})=x+y$, because the fact that $\vec {J}$ is a rational profile entails each of the following: (i) every group member who either accepts p or accepts q must also accept $p \cup q$ (note that $p \cup q$ must be in ${\mathbf{A}}$ because of ${\mathbf{A}}$’s closure properties); (ii) no group member can believe both p and q (as they are mutually exclusive); (iii) no group member other than those who either accept p or q can accept $p \cup q$.

 

Proof of theorem 3

$[\subseteq ]$: suppose $p \in {\mathbf{LS}}^{+}(\vec {J})$. We want to show $p \in {\mathbf{ST}}(P^{\vec {J}})$. Let $q=\bigcap [{\mathbf{LS}}^{+}(\vec {J})]$. Since A is a finite $\sigma$-algebra, $q \in {\mathbf{A}}$. Because ${\mathbf{LS}}^{+}$’s output is closed (relative to A), $q \in {\mathbf{LS}}^{+}(\vec {J})$. We show that q is the strongest $P^{\vec {J}}$-stable proposition (in ${\mathbf{A}}$).

• 1. $q\;{\hbox{is}}\;P^{\vec {J}}{\hbox{-stable:}}$ Suppose it is not, then $P^{\vec {J}}(q)<1$ and there is $w \in q$, s.t. $P^{\vec {J}}(\{w\})\le P^{\vec {J}}(W-q)$. Let $r:=(q-\{w\}) \cup (W-q)$. Given $P^{\vec {J}}(\{w\})\le P^{\vec {J}}(W-q)$, we must have: $P^{\vec {J}}(r)\ge P^{\vec {J}}(q)$. This is equivalent to:

  $$ {\texttt{support}}(r, \vec {J})\ge {\texttt{support}}(q, \vec {J}) $$

  Given this, the fact that ${\mathbf{LS}}^{+}$ is a uniform relaxed quota rule and $q\in {\mathbf{LS}}^{+}(\vec {J}), r\in {\mathbf{LS}}^{+}(\vec {J})$. However, this is incompatible with $q=\bigcap [{\mathbf{LS}}^{+}(\vec {J})]$. Because $w \in q$, and $q=\bigcap [{\mathbf{LS}}^{+}(\vec {J})], w \in r$, but that contradicts r’s definition.

• 2. $q\,{\hbox{is\,strongest\,among\,the}}\,P^{\vec {J}}{\hbox{-stable\,propositions:}}$ suppose there is an r that is $P^{\vec {J}}$-stable and $r\,\subsetneq\,q$. Let w be the world that witnesses $r\,\subsetneq\,q$ (so $w \in q, w\notin r$). Because r is $P^{\vec {J}}$-stable, ${\{s\mathbin { | } P^{\vec {J}}(s)\ge P^{\vec {J}}(r)\}}$ must be non-empty (it includes r), consistent and closed. But then ${\texttt{closed}}(\vec {J}, P^{\vec {J}}(r))$ is non-empty, consistent and closed. Moreover, because $r\,\subsetneq\,q, P^{\vec {J}}(r)\le P^{\vec {J}}(q)$. This, together with the fact that ${\texttt{closed}}(\vec {J}, P^{\vec {J}}(r))$ is non-empty, consistent and closed, implies:

  $$ {\texttt{closed}}(\vec {J}, P^{\vec {J}}(q)) \subseteq {\texttt{closed}}(\vec {J}, P^{\vec {J}}(r)) $$

  Because of the definition of ${\mathbf{LS}}^{+}$, this implies:

  • (i) ${\texttt{closed}}(\vec {J}, P^{\vec {J}}(r)) \subseteq {\mathbf{LS}}^{+}(\vec {J})$

  In addition, we must have all of the following.

  • (ii) $r \in {\texttt{closed}}(\vec {J}, P^{\vec {J}}(r))$;

  • (iii) $q = \bigcap [{\mathbf{LS}}^{+}(\vec {J})]$;

  • (iv) $w \in q, w \notin r$

  (ii) is immediate because $r \in {\{s\mathbin { | } P^{\vec {J}}(s)\ge P^{\vec {J}}(r)\}}$; (iii) and (iv) repeat how we fixed q and w respectively at the beginning of this proof.

  But these four claims are contradictory. By (i) and (ii), $r \in {\mathbf{LS}}^{+}(\vec {J})$. By (iii) and (iv), w is in every member of ${\mathbf{LS}}^{+}(\vec {J})$. But it is not in r, so $r \notin {\mathbf{LS}}^{+}(\vec {J})$.

Putting together (1) and (2) implies that q is the strongest P-stable proposition.

$[\supseteq ]$: suppose $p \in {\mathbf{ST}}(P^{\vec {J}})$. Let $q=\bigcap {\mathbf{ST}}(P^{\vec {J}})$ and $t'=P^{\vec {J}}(q)$. Note that it immediately follows that $P^{\vec {J}}(p)\ge t'$. Furthermore, it follows that if ${\texttt{closed}}({\vec {J}},t')$ is non-empty, $p \in {\texttt{closed}}({\vec {J}},t')$ and so $p \in {\mathbf{LS}}^{+}(\vec {J})$.

All we have to show is that ${\texttt{closed}}(\vec {J}, t')$ is non-empty, which it is iff ${\{p\mathbin { | } {\texttt{support}}(p, \vec {J})\ge t'\}}$ (i.e. ${\{p\mathbin { | } P^{\vec {J}}(p)\ge t'\}}$) is closed and consistent. This follows by Leitgeb’s theorem that for every probability function P, including $P^{\vec {J}}, {\{p\mathbin { | } P(p)\ge P(q)\}}$ is consistent and closed (relative to ${\mathbf{A}}$) when q is P-stable.