Skip to main content
SpringerLink
Log in

Evaluation of Steady State Probabilities of the • / G / ∞ Queuing System for Different Input Flow Models

  • Published:
Cybernetics and Systems Analysis Aims and scope

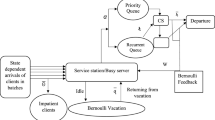
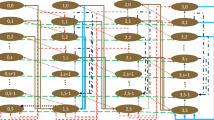
Abstract

Five input flow models are considered whose structures are considerably more complicated than a Poisson structure and in which steady state probabilities of the •/ G / ∞ queuing system can be found in explicit form (the Poisson distribution). For these models, a combination of the Poisson distribution (the analytical part) and statistical simulation (the statistical part) allows one to evaluate steady state probabilities by accelerated simulation. The accuracy of the obtained estimates is illustrated by numerical examples.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. G. P. Klimov, Stochastic Queuing Systems [in Russian], Nauka, Moscow (1966).

    Google Scholar 

  2. L. Takács, “On Erlang’s formula,” Ann. Math. Stat., Vol. 40, No 1, 71–78 (1969).

    Article  MathSciNet  MATH  Google Scholar 

  3. D. König and D. Stoyan, Methoden der Bedienungstheorie, Akademie-Verlag, Berlin (1976).

    MATH  Google Scholar 

  4. T. Saati, Elements of Queuing Theory and Its Applications [Russian translation], Librocom, Moscow (2010).

    Google Scholar 

  5. B. V. Gnedenko and I. N. Kovalenko, Introduction to Queuing Theory [in Russian], LKI, Moscow (2012).

    MATH  Google Scholar 

  6. B. V. Gnedenko, Yu. K. Belyaev, and A. D. Solovyev, Mathematical Methods in Reliability Theory [in Russian], Librocom, Moscow (2013).

    Google Scholar 

  7. B. A. Sevast’yanov, “An ergodic theorem for Markov processes and its application to telephone systems with refusals,” Theory Probab. Appl., Vol. 2, Iss. 1, 106–116 (1957).

    MathSciNet  MATH  Google Scholar 

  8. D. König, K. Mattes, and K. Nawrotzki, Verallgemeinerungen der Erlangschen und Engsetschen Formeln (eine Methode in der Bedienungstheorie), Akademie-Verlag, Berlin (1967).

    MATH  Google Scholar 

  9. P. Franken, D. Koenig, U. Arndt, and V. Schmidt, Queues and Point Processes [Russian translation], Naukova Dumka, Kyiv (1984).

    MATH  Google Scholar 

  10. I. N. Kovalenko, “Rare events in queueing systems — a survey,” Queueing Systems, Vol. 16, No 1, 1–49 (1994).

    Article  MathSciNet  MATH  Google Scholar 

  11. B. Blaszczyszyn, T. Rolski, and V. Schmidt, “Light-traffic approximations in queues and related stochastic models,” in: Advances in Queueing, CRC Press, Boca Raton (1995), pp. 379–406.

  12. I. N. Kovalenko, “Approximation of queues via small-parameter method,” in: Advances in Queueing, CRC Press, Boca Raton (1995), pp. 481–506.

  13. I. N. Kovalenko, “Ergodic and light-traffic properties of a complex repairable system,” Math. Meth. Operat. Res., Vol. 45, No. 2, 387–409 (1997).

    Article  MathSciNet  MATH  Google Scholar 

  14. J. B. Atkinson and I. N. Kovalenko, “On the practical insensitivity of the availability of some redundant repairable systems to the lifetime distribution in light traffic,” in: Proc. Int. Conf. on Probability Analysis of Rare Events, Aviation University, Riga (1999), pp. 83–91.

  15. I. N. Kovalenko, J. B. Atkinson, and K. V. Mikhalevich, “Three cases of light-traffic insensitivity of the loss probability in a GI/G/m/0 loss system to the shape of the service time distribution,” Queueing Systems, Vol. 45, No. 3, 245–271 (2003).

    Article  MathSciNet  MATH  Google Scholar 

  16. V. S. Korolyuk and A. F. Turbin, Mathematical Foundations of the State Lumping of Complex Systems [in Russian], Naukova Dumka, Kyiv (1978).

    MATH  Google Scholar 

  17. V. S. Korolyuk and V. V. Korolyuk, Stochastic Models of Systems, Kluwer, Boston (1999).

    Book  MATH  Google Scholar 

  18. A. Z. Melikov, L. A. Ponomarenko, and V. V. Paladyuk, Teletraffic: Models, Methods, and Optimization [in Russian], Politekhnika, Kyiv (2007).

    Google Scholar 

  19. A. Z. Melikov, L. A. Ponomarenko, and M. I. Fattakhova, Control over Multiservice Communication Networks with Buffer Storage [in Russian], NAU-druk, Kyiv (2008).

    Google Scholar 

  20. L. Ponomarenko, C. S. Kim, and A. Melikov, Performance Analysis and Optimization of Multi-Traffic on Communication Networks, Springer, New York (2010).

    Book  MATH  Google Scholar 

  21. A. V. Livinskaya and E. A. Lebedev, “Limit theorem for multichannel networks in heavy traffic,” Cybernetics and Systems Analysis, Vol. 48, No. 6, 899–905 (2012).

    Article  MathSciNet  MATH  Google Scholar 

  22. E. Lebedev and G. Livinska, “Gaussian approximation of multi-channel networks in heavy traffic,” Communications in Computer and Information Science, No. 356, 122–130 (2013).

    Article  MATH  Google Scholar 

  23. I. N. Kovalenko, N. Yu. Kuznetsov, and Ph. Pegg, Mathematical Theory of Reliability of Time Dependent Systems with Practical Applications, Wiley, Chichester (1997).

    MATH  Google Scholar 

  24. S. M. Ermakov and V. B. Melas, Design and Analysis of Simulation Experiments, Kluwer, Dordrecht (1995).

    MATH  Google Scholar 

  25. P. E. Lassila and J. T. Virtamo, “Efficient importance sampling for Monte Carlo simulation of loss systems,” in: Proc. ITC-16, Teletraffic Engineering in a Competitive World, Elsevier, Edinburgh (1999), pp. 787-796.

  26. P. Glasserman, Ph. Heidelberger, P. Shahabuddin, and T. Zajic, “Multilevel splitting for estimating rare event probabilities,” Operat. Res., Vol. 47, No. 4, 585–600 (1999).

    Article  MathSciNet  MATH  Google Scholar 

  27. A. A. Shumskaya, “Estimation of stationary loss probability in a queuing system with recurrent input flows,” Cybernetics and Systems Analysis, Vol. 40, No. 2, 260–269 (2004).

    Article  MathSciNet  MATH  Google Scholar 

  28. S. M. Yermakov, Monte Carlo Method and Related Matters [in Russian], Nauka, Moscow (1975).

    Google Scholar 

  29. I. N. Kovalenko, N. Yu. Kuznetsov, and V. M. Shurenkov, Random Processes: A Handbook [in Russian], Naukova Dumka, Kyiv (1983).

    MATH  Google Scholar 

  30. A. A. Shumska, “Fast simulation of the steady-state distribution of the number of customers in the queuing system SMBAP |G| ∞,” System Research and Information Technologies, No. 3, 121–129 (2004).

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Physico-Technical Institute of the National Technical University of Ukraine “Igor Sikorsky Kyiv Polytechnic Institute”, Kyiv, Ukraine

    I. N. Kuznetsov & A. A. Shumskaya

Authors
  1. I. N. Kuznetsov
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. A. A. Shumskaya
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to I. N. Kuznetsov.

Additional information

Translated from Kibernetika i Sistemnyi Analiz, No. 2, March–April, 2017, pp. 122–133.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Kuznetsov, I.N., Shumskaya, A.A. Evaluation of Steady State Probabilities of the • / G / ∞ Queuing System for Different Input Flow Models. Cybern Syst Anal 53, 269–279 (2017). https://doi.org/10.1007/s10559-017-9927-5

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10559-017-9927-5

Keywords

Search

Navigation