Endogenous interval games in oligopolies and the cores

Abstract

In this article we study interval games in oligopolies following the $\gamma$-approach. First, we analyze their non-cooperative foundation and show that each coalition is associated with an endogenous real interval. Second, the Hurwicz criterion turns out to be a key concept to provide a necessary and sufficient condition for the non-emptiness of each of the induced core solution concepts: the interval and the standard $\gamma$-cores. The first condition permits to ascertain that even for linear and symmetric industries the interval $\gamma$-core is empty. Moreover, by means of the approximation technique of quadratic Bézier curves we prove that the second condition always holds, hence the standard $\gamma$-core is non-empty, under natural properties of profit and cost functions.

Appendix

1.1 Proofs of propositions in Sect. 2

Proof of Proposition 2.1

$[\Longrightarrow ]$ Take $\hat{x}^{{\mathcal {P}}}\in {\mathbf {X}^{{\mathcal {P}}}}$ and let $\hat{X}=\sum _{S\in {{\mathcal {P}}}}\hat{x}^S$. By (3), for each $S\in {{\mathcal {P}}}$ it holds that:

$$\begin{aligned} \hat{x}^S\in {B^S(\hat{x}^{-S})}&\Longleftrightarrow \pi _S(\hat{x}^S,\hat{x}^{-S})=\max _{y^S\in {X^S}}\pi _S(y^S,\hat{x}^{-S})\\&\Longleftrightarrow p(\hat{X}-\hat{x}^S+\hat{x}^S)\hat{x}^S-C_S(\hat{x}^S)=\max _{y^S\in {X^S}}p(\hat{X}-\hat{x}^S+y^S)y^S-C_S(y^S)\\&\Longleftrightarrow \psi _S(\hat{x}^S,\hat{x}^S,\hat{X})=\max _{y^S\in {X^S}}\psi _S(y^S,\hat{x}^S,\hat{X})\\&\Longleftrightarrow \hat{x}^S\in {R_S(\hat{X})}\text{. } \end{aligned}$$

Hence, we conclude that $\hat{X}\in {R_{{\mathcal {P}}}(\hat{X})}$.

$[\Longleftarrow ]$ Take $\hat{X}\in {R_{{\mathcal {P}}}(\hat{X})}$. By (6), it holds that $\hat{X}=\sum _{S\in {{\mathcal {P}}}}\hat{x}^S$ and for each $S\in {{\mathcal {P}}}$, $\hat{x}^S\in {R_S(\hat{X})}$. By the same argument to the one in the first part of the proof it follows that for each $S\in {{\mathcal {P}}}$ we have $\hat{x}^S\in {B^S(\hat{x}^{-S})}$, and therefore $\hat{x}^{{\mathcal {P}}}\in {\mathbf {X}^{{\mathcal {P}}}}$. $\square$

Proof of Proposition 2.2

First, we show points (i) and (ii). For each $S\in {{\mathcal {P}}}$, $X^S$ is compact and convex. It follows from the continuity, the strict monotonicity and the convexity of any cost function $C_i$, that coalition cost function $C_S$ as defined in (1) is continuous, strictly increasing and convex. Moreover, the inverse demand function p is continuous, strictly decreasing and concave on $[0,\xi ]$. It follows from Theorem 3.3.3 (page 30) in Okuguchi and Szidarovszky (1990) that $\mathbf {X}^{{\mathcal {P}}}$ is a polyhedron and that the equilibrium total output $\bar{X}$ is the same for any Nash equilibrium which proves points (i) and (ii).

Then, we prove point (iii). From Lemma 3.3.1 (page 27) in Okuguchi and Szidarovszky (1990) we deduce for any $S\in {{\mathcal {P}}}$ and all $X\in {X^N}$ that $R_S(X)$ as defined in (5) is a (possibly degenerate) closed and bounded interval which we denote by $[\alpha _S(X),\beta _S(X)]$. By point (ii), we know that there exists a unique equilibrium total output $\bar{X}$. It follows that the polyhedron $\mathbf {X}^{{\mathcal {P}}}$ can be represented as the intersection of the orthotope $\prod _{S\in {{\mathcal {P}}}}R_S(\bar{X})=\prod _{S\in {{\mathcal {P}}}}[\alpha _S(\bar{X}),\beta _S(\bar{X})]$ and the hyperplane $\big \lbrace {x^{{\mathcal {P}}}\in {X^{{\mathcal {P}}}}:\sum _{S\in {{\mathcal {P}}}}x^S=\bar{X}}\big \rbrace$, i.e.,

$$\begin{aligned} \mathbf {X}^{{\mathcal {P}}}=\bigg \lbrace {x^{{\mathcal {P}}}\in {X^{{\mathcal {P}}}}:\forall S\in {{\mathcal {P}}}, x^S\in {\big [\alpha _S(\bar{X}),\beta _S(\bar{X})\big ]} \text{ and } \sum _{S\in {{\mathcal {P}}}}x^S=\bar{X}}\bigg \rbrace \text{. } \end{aligned}$$

The polyhedron $\mathbf {X}^{{\mathcal {P}}}$ is compact and convex as the intersection of two compact and convex sets. Since a convex set is always connected, we deduce that the polyhedron $\mathbf {X}^{{\mathcal {P}}}$ is compact and connected. Moreover, the continuity of the inverse demand function p and of any coalition cost function $C_S$ implies that the coalition profit function $\pi _S$ as in (2) is continuous. It follows that the set $\pi _S(\mathbf {X}^{{\mathcal {P}}})$ is compact and connected as the image of a compact and connected set by a continuous function. Since a subset of ${\mathbb {R}}$ is connected if and only if it is an interval, we conclude that $\pi _S(\mathbf {X}^{{\mathcal {P}}})$ is a compact real interval, which proves point (iii).$\square$

1.2 Approximation of the inverse demand function

Given an inverse demand function p satisfying assumption (a), we construct a sequence of inverse demand functions which, in addition, are differentiable on $]0,\xi [$, denoted by $(p_\epsilon )_{\epsilon >0}$ that uniformly converges to p by means of the approximation technique of Bézier curves Bézier (1976).

A Bézier curve is a parametric curve defined through specific points called control points. A particular class of Bézier curves are quadratic Bézier curves defined with three control points $X_0$, $X_1$ and $X_2$:

Formally, this quadratic Bézier curve is the path traced by the function $B:[0,1]\longrightarrow {\mathbb {R}}^2$ defined as:

$$\begin{aligned} B(t)=(1-t)^2X_0+2(1-t)tX_1+t^2X_2 \end{aligned}$$

(21)

Proposition 5.1

Let p be an inverse demand function satisfying assumption (a). Then, there exists a sequence of inverse demand functions $(p_\epsilon )_{\epsilon >0}$ which, in addition, are differentiable on $]0,\xi [$ that uniformly converges to p.

Proof

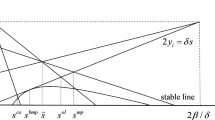
First, for any $X\in {{\mathcal {X}}}$ and each $\epsilon >0$, we define a quadratic Bézier curve. The steps of this construction are illustrated below:

For any $X\in {{\mathcal {X}}}$, define $N_\epsilon (X)$ the neighborhood of X with radius $\epsilon$ as:

$$\begin{aligned} N_\epsilon (X)=\lbrace {Y\in {{\mathbb {R}}_+}:|Y-X |<\epsilon }\rbrace \text{. } \end{aligned}$$

For any $X\in {\mathcal {X}}$, there exists $\bar{\epsilon }>0$ such that for all $\epsilon <\bar{\epsilon }$, it holds that $N_\epsilon (X)\subset ]0,\xi [$. Moreover, since ${\mathcal {X}}$ is at most denumerable, there exists $\bar{\bar{\epsilon }}>0$ such that for all $\epsilon <\bar{\bar{\epsilon }}$ it holds that:

$$\begin{aligned} \forall (X,X')\in {{\mathcal {X}}\times {\mathcal {X}}}, N_\epsilon (X)\cap N_\epsilon (X')=\emptyset \text{. } \end{aligned}$$

In the remainder of the proof, we assume everywhere that $\epsilon <\min \lbrace {\bar{\epsilon },\bar{\bar{\epsilon }}}\rbrace$. Take any $X\in {{\mathcal {X}}}$. For each $\epsilon >0$, in order to construct the quadratic Bézier curve, we consider three control points given by $X_0=(\inf N_\epsilon (X),p(\inf N_\epsilon (X)))$, $X_2=(\sup N_\epsilon (X),p(\sup N_\epsilon (X)))$ and $X_1$ defined as the intersection point between the tangent lines to the curve of p at points $X_0$ and $X_2$ respectively. Given these three control points, the quadratic Bézier curve is the path traced by the function $B_X^\epsilon :[0,1]\longrightarrow {\mathbb {R}}^2$ defined as in (21). It is well-known that the quadratic Bézier curve $B_X^\epsilon$ can be parametrized by a polynomial function denoted by $f_X^\epsilon :\overline{N_\epsilon (X)}\longrightarrow {\mathbb {R}}_+$ where $\overline{N_\epsilon (X)}$ is the closure of $N_\epsilon (X)$.

Then, for each $\epsilon >0$ we define the inverse demand function $p_\epsilon :{\mathbb {R}}_+\longrightarrow {\mathbb {R}}_+$ as:

$$\begin{aligned} p_\epsilon (Y)=\left\{ \begin{array}{cl} \displaystyle f_X^\epsilon (Y) &{} \quad \text{ if } \text{ for } \text{ some } X\in {{\mathcal {X}}}, Y\in {N_\epsilon (X)}\text{, } \\ p(Y) &{}\quad \text{ otherwise. }\end{array}\right. \end{aligned}$$

(22)

By the construction of control points $X_0$, $X_1$ and $X_2$, it follows from the properties of the inverse demand function p and of the quadratic Bézier curves defined above that $p_\epsilon$ as defined in (22) is strictly decreasing, concave on $[0,\xi ]$ and differentiable on $]0,\xi [$.

It remains to show that the sequence $(p_\epsilon )_{\epsilon >0}$ uniformly converges to p. Take $\zeta >0$ and assume that $Y\not \in {{\mathcal {X}}}$. It follows that there exists $\epsilon _1>0$ such that for each $\epsilon <\epsilon _1$ and for any $X\in {{\mathcal {X}}}$ we have $Y\not \in {N_{\epsilon }(X)}$. Hence, by (22) for each $\epsilon <\epsilon _1$ we have $p_{\epsilon }(Y)=p(Y)$, and so $|{p_{\epsilon }(Y)-p(Y)}|=0<\zeta$. Then, assume that $Y\in {{\mathcal {X}}}$. For each $\epsilon >0$ we denote by $G_Y^\epsilon$ the convex hull of the set of control points $\lbrace {X_0,X_1,X_2}\rbrace$:

$$\begin{aligned} G_Y^\epsilon =co\lbrace {X_0,X_1,X_2}\rbrace \text{. } \end{aligned}$$

By the construction of control points $X_0$, $X_1$ and $X_2$ it holds that:

$$\begin{aligned} \lim _{\epsilon \longrightarrow 0}G_Y^\epsilon =\lbrace {(Y,p(Y))}\rbrace \end{aligned}$$

(23)

Moreover, recall that $B_Y^\epsilon$ is defined as a convex combination of control points $X_0$, $X_1$ and $X_2$. Hence, for each $\epsilon >0$ we have $B_Y^\epsilon \subseteq G_Y^\epsilon$, and therefore $(Y,f_Y^\epsilon (Y))\in {G_Y^\epsilon }$. By (23) we deduce that there exists $\epsilon _2>0$ such that for each $\epsilon <\epsilon _2$, we have:

$$\begin{aligned} |{p_{\epsilon }(Y)-p(Y)}|=|{f_Y^{\epsilon }(Y)-p(Y)}|<\zeta \text{. } \end{aligned}$$

Finally, take $\epsilon _3=\min \lbrace {\epsilon _1,\epsilon _2}\rbrace$. It follows for each $\epsilon <\epsilon _3$ that:

$$\begin{aligned} \forall Y\in {{\mathbb {R}}_+}, |{p_{\epsilon }(Y)-p(Y)}|<\zeta \text{, } \end{aligned}$$

which proves that the sequence $(p_\epsilon )_{\epsilon >0}$ uniformly converges to p.$\square$