Unique local determination of convex bodies

Abstract

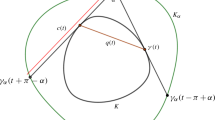
Barker and Larman asked the following. Let ${K' \subset {\mathbb{R}}^d}$ be a convex body, whose interior contains a given convex body ${K \subset {\mathbb{R}}^d}$, and let, for all supporting hyperplanes H of K, the (d − 1)-volumes of the intersections ${K' \cap H}$ be given. Is K′ then uniquely determined? Yaskin and Zhang asked the analogous Question when, for all supporting hyperplanes H of K, the d-volumes of the “caps” cut off from K′ by H are given. We give local positive answers to both of these questions, for small C ²-perturbations of K, provided the boundary of K is C ²₊ . In both cases, (d − 1)-volumes or d-volumes can be replaced by k-dimensional quermassintegrals for ${1 \le k \le d-1}$ or for ${1 \le k \le d}$, respectively. Moreover, in the first case we can admit, rather than hyperplane sections, sections by l-dimensional affine planes, where ${1 \le k \le l \le d-1}$. In fact, here not all l-dimensional affine subspaces are needed, but only a small subset of them (actually, a (d − 1)-manifold), for unique local determination of K′.