A size-dependent constitutive modelling framework for localised failure analysis

Abstract

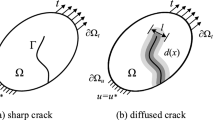
Localised deformation of materials usually takes place in thin bands during the nonlinear phase of the deformation process. The orientation and size of these localisation bands are important properties characterising the post-localisation behaviour of the materials, and hence should be taken into account in constitutive modelling. In this research, a new approach is proposed for the integration of both size and orientation of a localisation band in the constitutive description beyond the onset of localisation. Since a length scale related to the size of the localisation band appears in the model description, its post-localisation response then scales with both the band size and the size of the volume element containing it. Therefore, size effects are intrinsically included and post-localisation behaviour is correctly captured, which helps ensure convergence of numerical solutions upon discretisation refinement in numerical analysis of boundary value problems. The concept together with implementation features of the framework and its performances at constitutive level and in the analysis of boundary value problems are presented in this paper.

Appendices

Appendix 1

In this section, the Von Mises and Drucker–Prager constitutive models are briefly summarised, details of those two models can be found elsewhere in literature [19, 74, 76, 87].

1.1 Yield criterion

The Von Mises criterion is a pressure-independent criterion which is normally used for metals and normally-consolidated clays under relatively rapid loading conditions (undrained behaviour). The yield function following the Von Mises criterion is:

$$\begin{aligned} f\left( {\varvec{\sigma }},\kappa \right) =\sqrt{3J_{2}}-c\left( \kappa \right) \end{aligned}$$

(36)

in which c is the yield strength which is a function of the softening parameter $\kappa$, and

$$\begin{aligned} J_{2}=\frac{1}{2}\left( s_{1}^{2}+s_{2}^{2}+s_{3}^{2} \right) \end{aligned}$$

(37)

is the second invariant of the deviatoric stresses ${\varvec{s}}={\varvec{\sigma }}-p{{\varvec{\delta }} }$ with $p=\frac{1}{3}\left( \sigma _{1}+\sigma _{2}+\sigma _{3} \right)$ being the hydrostatic pressure, and ${{\varvec{\delta }} }$ the Kronecker delta. The softening parameter $\kappa$ is normally dependent on the strain history and in this paper the rate of $\kappa$ is defined as the second invariant of the plastic strain vector $\dot{{{\varvec{e}}}}^{p}$ based on the strain hardening hypothesis:

$$\begin{aligned} \dot{\kappa }=\sqrt{\frac{2}{3}\dot{{{\varvec{e}}}}^{p}:\dot{{{\varvec{e}}}}^{p}} \end{aligned}$$

(38)

and then $\kappa$ can be integrated along the loading path as:

$$\begin{aligned} \kappa =\int {\dot{\kappa }\mathrm {d}t} \end{aligned}$$

(39)

The softening rule for the yield strength c is either linear- or exponential-function of $\kappa$ as following:

$$\begin{aligned} c=c_{0}+b\cdot \kappa \end{aligned}$$

(40)

or:

$$\begin{aligned} c=c_{0}-c_{0}\cdot a\left( 1-e^{-b\,\cdot \, \kappa } \right) \end{aligned}$$

(41)

with $c_{0}$ being the initial yield strength, b the softening modulus and a a parameter governing the residual strength of the material.

The Drucker–Prager criterion includes a dependence on the hydrostatic pressure p which is widely used to describe the inelastic behaviour of granular materials, drained clays, rock and concrete under compression. The Drucker–Prager yield function is defined as:

$$\begin{aligned} f\left( {\varvec{\sigma }},\kappa \right) =\sqrt{3J_{2}}+\alpha \cdot p-\beta \cdot c\left( \kappa \right) \end{aligned}$$

(42)

with $\alpha ,\beta$ constants given by:

$$\begin{aligned} \alpha =\frac{3\tan \varphi }{\sqrt{3+4\tan ^{2}\varphi }}\quad \beta =\frac{3}{\sqrt{3+4\tan ^{2}\varphi }} \end{aligned}$$

(43)

in which $\varphi$ is the internal friction angle and c is the cohesion of a material which is a function of the softening parameter $\kappa$ (Eq. 40). The concept of non-associative flow is applied by defining the plastic potential $g\left( {\varvec{\sigma }},\kappa \right)$ equal to $f\left( {\varvec{\sigma }},\kappa \right)$ but with the dilatancy angle $\psi$ substituted for the friction angle $\varphi$.

1.2 Flow rule

The Von Mises and Drucker–Prager constitutive models are classical elasto-plastic models in which the evolution of the plastic strains can be written as:

$$\begin{aligned} \dot{{\varvec{\varepsilon }} }^{p}=\dot{\lambda }\frac{\partial f}{\partial {\varvec{\sigma }}}=\dot{\lambda }{\varvec{l}} \end{aligned}$$

(44)

with $\dot{\lambda }$ being the non-negative plastic multiplier determining the magnitude of the plastic flow and ${\varvec{l}}$ the plastic flow direction. In case of non-associative plasticity, ${\varvec{l}}$ is replaced by ${\varvec{m}}=\frac{\partial g}{\partial {\varvec{\sigma }}}$.

1.3 Tangent stiffness

The tangent stiffness of elasto-plastic constitutive models can be derived in the same procedure. Firstly, the relation between the stress and the elastic strain is defined:

$$\begin{aligned} {\varvec{\sigma }}={\varvec{D}}^{e}:{\varvec{\varepsilon }}^{e}={\varvec{D}}^{e}:\left( {{\varvec{\varepsilon }}-{\varvec{\varepsilon }}}^{p} \right) \end{aligned}$$

(45)

in which ${\varvec{D}}^{e}$ is the elastic stiffness. Secondly, the consistency condition of the plastic flow can be written as:

$$\begin{aligned} {\varvec{l}}^{T}\dot{{\varvec{\sigma }} }+\left( \frac{\partial f}{\partial \kappa } \right) ^{T}\dot{\kappa }=0 \end{aligned}$$

(46)

From the definitions (38) and (44), the hardening variable can be defined as a function of the plastic multiplier:

$$\begin{aligned} \dot{\kappa }=\dot{\lambda }{\varvec{h}}\left( {\varvec{\sigma }},\kappa \right) \end{aligned}$$

(47)

with ${\varvec{h}}$ being a vector function of the stress and the hardening variable. If we define the hardening modulus h as:

$$\begin{aligned} h={-\left( \frac{\partial f}{\partial \kappa } \right) }^{T}{\varvec{h}}\left( {\varvec{\sigma }},\kappa \right) \end{aligned}$$

(48)

then the consistency condition can be rewritten as:

$$\begin{aligned} {\varvec{l}}^{T}\dot{{\varvec{\sigma }} }-h\dot{\lambda }=0 \end{aligned}$$

(49)

Differentiating Eq. (45) and substituting it in the consistency condition yield an expression for the magnitude of the plastic flow:

$$\begin{aligned} \dot{\lambda }=\frac{{\varvec{l}}^{T}:{\varvec{D}}^{e}:\dot{{\varvec{\varepsilon }} }}{{h+{\varvec{l}}}^{T}:{\varvec{D}}^{e}:{\varvec{m}}} \end{aligned}$$

(50)

Finally, back substitution the expression of $\dot{\lambda }$ to the rate form of Eq. (45) leads to the relationship between the stress rate $\dot{{\varvec{\sigma }} }$ and the strain rate $\dot{{\varvec{\varepsilon }} }$ in the form:

$$\begin{aligned} \dot{{\varvec{\sigma }} }=\left( {\varvec{D}}^{e}-\frac{\left( {\varvec{D}}^{e}:{\varvec{m}} \right) \otimes \left( {\varvec{l}}^{T}:{\varvec{D}}^{e} \right) }{{h+{\varvec{l}}}^{T}:{\varvec{D}}^{e}:{\varvec{m}}} \right) :\dot{{\varvec{\varepsilon }} }={\varvec{D}}:\dot{{\varvec{\varepsilon }} } \end{aligned}$$

(51)

with ${\varvec{D}}$ being the tangent stiffness.

Fig. 24

A one-dimensional linear softening model

Appendix 2

Assuming a linear softening behaviour for the material inside the localization band (Fig. 24), with tangent stiffness $a_{o}>0$ and $a_{i}<0$ in one-dimensional (1D) cases for the behaviour outside and inside the localization band, respectively. The 1D formulation (Eq.  16) in this case involves inverting ${\left( 1-f \right) a}_{i}+{fa}_{o}$ that can be singular. We will investigate when this singularity can happens in this specific case.

Given the material fracture energy $G_{F}$ as the energy released due to cracking and the width h of the localiaation band (termed Fracture Process Zone in concrete/rock cracking), we have the following relationship (see Fig. 24):

$$\begin{aligned} \frac{G_{F}}{h}=\frac{f_{t}^{{\prime 2}}}{2}\left( \frac{1}{a_{o}}-\frac{1}{a_{i}} \right) \end{aligned}$$

(52)

From this equation, we obtain

$$\begin{aligned} a_{i}=\frac{1}{\frac{1}{a_{o}}-\frac{{2G}_{F}}{hf_{t}^{{\prime ^{2}}}}} \end{aligned}$$

(53)

On the other hand, from the singularity of ${\left( 1-f \right) a}_{i}+{fa}_{o}$ we can write:

$$\begin{aligned} a_{i}=-\frac{fa_{o}}{1-f}=-\frac{\frac{h}{H}a_{o}}{1-\frac{h}{H}} \end{aligned}$$

(54)

Equating (53) and (54) we can find the condition that makes ${\left( 1-f \right) a}_{i}+{fa}_{o}$ singular:

$$\begin{aligned} a_{i}=\frac{1}{\frac{1}{a_{o}}-\frac{{2G}_{F}}{hf_{t}^{{\prime ^2}}}}=-\frac{\frac{h}{H}a_{o}}{1-\frac{h}{H}} \end{aligned}$$

(55)

After a few manipulations, the above condition becomes:

$$\begin{aligned} G_{F}=\frac{Hf_{t}^{{\prime ^2}}}{2a_{o}} \end{aligned}$$

(56)

This is in fact the value of fracture energy $G_{F}$ below which there is macro snap back. The above condition (56) therefore corresponds to perfectly brittle behaviour in the macro response, and singularity in this simple 1D case just happens when $G_{F}$ is equal to that limit. Higher or lower values of $G_{F}$ will therefore not affect the inversion of ${\left( 1-f \right) a}_{i}+{fa}_{o}$, and in all these cases it can be straightforwardly proved that the displacement velocity is always a monotonically increasing quantity.

Appendix 3

For quasi-brittle behaviour, a damage model characterising the effects of micro-cracking on the material response can be used. In such cases, the behaviour of the material inside the localisation band is governed by the following constitutive equations:

$$\begin{aligned}&\hbox {Stress-strain-damage:} \qquad \qquad {\varvec{\sigma }}_{i}=\left( 1-D \right) {\varvec{D}}^{e}:{\varvec{\varepsilon }}_{i} \end{aligned}$$

(57)

$$\begin{aligned}&\hbox {Damage (yield) function: }\quad \quad f\left( {\varvec{\sigma }},D \right) \!=\!\frac{{\varvec{\sigma }}_{i}^{+}:{\varvec{D}}^{e}:{\varvec{\sigma }}_{i}^{+}}{2\left( 1-D \right) ^{2}}-F\left( D \right) \!=\!0\nonumber \\ \end{aligned}$$

(58)

where ${\varvec{\sigma }}_{i}^{\mathrm {+}}$ is the “positive” part of the stress inside the localisation band, obtained using the eigenvalue decomposition [88]. The evolution of the scalar damage variable $\mathrm {D}$ is governed by function $F\left( D \right)$ of the form:

$$\begin{aligned} F\left( D \right) =\frac{f_{t}^{{\prime ^{2}}}}{2E}\left[ \frac{E+E_{p}\left( 1-D \right) ^{n}}{E\left( 1-D \right) +E_{p}\left( 1-D \right) ^{n}} \right] ^{2} \end{aligned}$$

(59)

in which $f_{t}^{{\prime }}$ is the uniaxial tensile strength, and two parameters $E_{p}$ and n are determined from the fracture energy of the material. Details on links between these parameters and the fracture energy can be found in [52]. The nonlocal version of the model can be found in [65].