Skip to main content
SpringerLink
Log in

Self-contact modeling on beams experiencing loop formation

  • Original Paper
  • Published:
Computational Mechanics Aims and scope Submit manuscript

Abstract

Many engineering scenarios involve contact between beam structures or, eventually, self-contact. Specifically when dealing with a beam submitted to large torsion loads and considering large displacements and rotations, it is possible to occur self-contact. Beams with low bending stiffness loaded with large torsion can present a loop, followed by self-contact and sometimes a snarl formation. This work presents a numerical model and numerical procedures to solve such a kind of self-contact in beams under loop formation. Numerical examples are presented in the context of initially straight nitinol beams. Numerical tests showed that friction may influence loop and snarling formation. Numerical models could predict self-contact occurrence events with good agreement with experimental results already published in literature. Snarling patterns (when occurred) were also predicted correctly, but with delay when compared to experiments. This delay showed to be friction-dependent.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17

Similar content being viewed by others

Notes

  1. Alternatively one could also write the virtual power theorem, leading to different equations. This was done for instance in [19].

  2. It was considered that the derivatives of matrices \({\varvec{\Psi } }^{\mathbf{i}+\mathbf{1}}\) and \({\varvec{\Upsilon }}^{\mathbf{i}+\mathbf{1}}\) have no important contributions to the tangent operator. The classical Newmark method was applied as described, considering the inertial and damping matrices. However, strictly speaking, the tangent operator should also contain Gateaux derivatives components from damping and inertia matrices, which were not considered. This does not imply in wrong solutions, once the weak form is complete and includes all contributions. The only possible implication would be a non-quadratic asymptotic convergence rate. However, good convergence rate was achieved in numerical examples here developed, even including large nonlinear behavior.

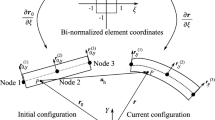
  3. The solution \((\upzeta _{1\mathrm{c}},{\upzeta }_{2\mathrm{c}})\) is not necessarily unique, once it depends on the nature of the curves. For straight non-parallel beams the solution is unique but, for generic spatial curves one can have multiple points obeying criterion for local minimum distance points. The present work assumes, as Zavarise and Wriggers [28], that beams are parameterized as straight lines for contact detection purposes, even after suffering bending. Clearly this is a geometric approximation, which should lead to good results in cases of meshes with small-length beam elements.

  4. This parameterization is used only in the contact evaluation of the here utilized model. It is completely different and independent from the parameterization used in structural model, presented in Sect. 2. For the simulations here presented, e.g., quadratic isoparametric interpolation was assumed for structural model, which is not consistent with the linear interpolation here assumed. This inconsistency, however, does not represent a drawback, once contact detection and structural interpolation are absolutely independent terms in problem weak form and can be with no problems be treated this way. Some tests were performed for frictionless contact considering higher order polynomials. However, increasing the interpolation degree would give a more precise contact detection, but does not ensure smoothness between beam elements, which could be achieved by Hermite interpolation using neighbor elements, such as done in [30]. This technique was not here applied and can be an object of future work.

  5. To shorten notation \(\mathbf{z}_{\mathbf{1}}^{\mathbf{i}+\mathbf{1}} ({\upzeta }_{1\hbox {c}} )\) and \(\mathbf{z}_{\mathbf{2}}^{\mathbf{i}+\mathbf{1}} ({\upzeta }_{2\hbox {c}} )\) are simply written by \(\mathbf{z}_{\mathbf{1}}^{\mathbf{i}+\mathbf{1}} \) and \(\mathbf{z}_{\mathbf{2}}^{\mathbf{i}+\mathbf{1}}\). All calculations involving gap function and its consistent linearization (leading to the tangent operator) for contact computation are evaluated using \({\upzeta }_{1\hbox {c}}\) and \({\upzeta }_{2\hbox {c}}\) values.

  6. One can note that, once linear interpolation is assumed, second derivatives present in Eqs. (49) and (50) are null.

  7. The parameters are related to the initial-touch position on both beams. These values have to be reset and re-set in case of losing and re-establishing contact. Furthermore, the actual beam has non-null cross section dimensions and, even small, strictly speaking will not lead to a line to line interaction, as implicitly modeled by the here used approach. Physically the contact interaction takes place on both beams external surface. Then, a more elaborated tangential gap function would have to consider the actual surface points of contact in both beams, mapping then on surfaces. This kinematics is absolutely more complicated than the proposed by Zavarise and Wriggers [28] approach. The real importance of such an effect, however, may take place only in problems with larger dimensions of cross section (when compared to beam length) or situations in which torsion and rolling may occur. An enhanced kinematic description involving ideas to be generalized to beam to beam contact is presented by Gay Neto et al. [37].

  8. Evaluation of expressions (60) and (61) is purely geometric. It is done by imposing the equality: \({\Delta }\lambda \mathbf{t} \,=\, {\Delta }\lambda _1\mathbf{t}_1 \,+\, {\Delta }\lambda _2\mathbf{t}_2\).

  9. Pinball radius value, once enough for detecting contact properly, does not affect contact forces, gap calculations, etc. It is only a first detection tool to help in efficiency of contact detection.

  10. Initial conditions (displacement and velocity) of point “B” were considered to be null in the beginning of the third load-step. One can note that Fig. 8 imposed displacement is not a ramp loading, but a composition of a null derivative function in origin—actually a polynomial of second order, until the time achieves 1 s, followed by a constant slope function until the time 4.5 s is achieved. This imposed displacement series avoids a jerk in the very beginning of dynamics which could be done in a traditional ramp loading. This time-series showed smoother oscillation results along time than a traditional “ramp”. This was very useful, once dealing with a very low damping rate.

References

  1. Love AEH (1944) A treatise on the mathematical theory of elasticity. Dover publications, New York

    MATH  Google Scholar 

  2. Liu FC (1975) Kink formation and rotational response of single and multi-strand electromechanical cables. Technical Note N-1403, Civil Engineering Lab, Naval Construction Batallion Center

  3. Ross AL (1977) Cable kinking analysis and prevention. J Eng Ind 99:112–115

    Article  Google Scholar 

  4. Yabuta T (1984) Submarine cable kink analysis. Bulletin of JSME 27:1821–1828

    Article  Google Scholar 

  5. Coyne J (1990) Analysis of the formation and elimination of loops in twisted cable. IEEE J Ocean Eng 15:72–83

    Article  Google Scholar 

  6. Thompson JMT, Champneys AR (1996) From helix to localized writhing in the torsional post-buckling of elastic rods. Proc R Soc Lond A 452:117–138 [Royal Society Publishing]

    Article  MathSciNet  MATH  Google Scholar 

  7. Goss VGA, van der Heijden GHM, Thompson JMT, Neukirch S (2005) Experiments on snap buckling, hysteresis and loop formation in twisted rods. Exp Mech 45(2):101–111

    Article  Google Scholar 

  8. van der Heijden GHM, Neukirch S, Goss VGA, Thompson JMT (2003) Instability and self-contact phenomena in the writhing of clamped rods. Int J Mech Sci 45:161–196

    Article  MATH  Google Scholar 

  9. Gay Neto A, Martins CA (2013) Structural stability of flexible lines in catenary configuration under torsion. Mar Struct 34:16–40

    Article  Google Scholar 

  10. Miyazaki Y, Kondo K (1997) Analytical solution of spatial elastica and its application to kinking problem. Int J Solid Struct 27:3619–3636

    Article  MathSciNet  Google Scholar 

  11. Goyal S, Perkins NC, Lee CL (2008) Non-linear dynamic intertwining of rods with self-contact. Int J Nonlinear Mech 43:65–73

    Article  MATH  Google Scholar 

  12. Antman SS (1974) Kirchhoff’s problem for nonlinearly elastic rods. Q Appl Math 32:221–240

    MathSciNet  MATH  Google Scholar 

  13. Simo JC (1985) A finite strain beam formulation. Three-dimensional dynamic problem. Part I. Comput Methods Appl Mech Eng 49:55–70

    Article  MATH  Google Scholar 

  14. Vu-Quoc L, Simo JC (1987) On the dynamics of Earth-orbiting flexible satellites with multibody components. AIAA J Guidance Control Dyn 10(6):549–558 paper G87–088

    Article  MATH  Google Scholar 

  15. Simo JC, Vu-Quoc L (1986) Three-dimensional finite-strain rod model. Part II: Computational aspects. Comput Methods Appl Mech Eng 58(1):79–116

    Article  MATH  Google Scholar 

  16. Simo JC, Vu-Quoc L (1988) On the dynamics in space of rods undergoing large motions-A geometrically exact approach. Comput Methods Appl Mech Eng 66:125–161

    Article  MathSciNet  MATH  Google Scholar 

  17. McRobie FA, Lasenby J (1999) Simo-Vu Quoc rods using Clifford algebra. Int J Numer Methods Eng 45(4):377–398

    Article  MathSciNet  MATH  Google Scholar 

  18. Pimenta PM, Yojo T, Pimenta PM (1993) Geometrically exact analysis of spatial frames. Appl Mech Rev 46:118–128

    Article  Google Scholar 

  19. Pimenta PM, Campello EMB, Wriggers P (2008) An exact conserving algorithm for nonlinear dynamics with rotational DOF’s and general hyperelasticity. part 1: Rods. Comput Mech 42:715–732

    Article  MathSciNet  MATH  Google Scholar 

  20. Gay Neto A, Martins CA, Pimenta PM (2014) Static analysis of offshore risers with a geometrically-exact 3D beam model subjected to unilateral contact. Comput Mech 53:125–145

    Article  MathSciNet  MATH  Google Scholar 

  21. Chamekh M, Mani-Aouadi S, Moakher M (2014) Stability of elastic rods with self-contact. Comput Methods Appl Mech Eng 279:227–246

    Article  MathSciNet  Google Scholar 

  22. Gay Neto A, Pimenta PM, Martins CA (2013) Loop formation in catenary risers on installation conditions: a comparison of statics and dynamics. Proceedings of the \(32^{nd}\) International Conference on Ocean, Offshore and Arctic Engineering

  23. Wriggers P (2008) Nonlinear Finite Element Methods. Springer-Verlag, Berlin Heidelberg

    MATH  Google Scholar 

  24. Pimenta PM, Campello EMB (2001) Geometrically nonlinear analysis of thin-walled space frames. Proceedings of the Second European Conference on Computational Mechanics, II ECCM, Krakow

  25. Campello EMB, Pimenta PM, Wriggers P (2003) A triangular finite shell element based on a fully nonlinear shell formulation. Comput Mech 31:505–518

    Article  MATH  Google Scholar 

  26. Pauletti RM, Pimenta PM (1995) Formulação de um elemento finito de cabo incorporando o efeito do atrito. Revista Internacional de Métodos Numéricos para Cálculo y Diseño en Ingeniería 11(4):565–576

    Google Scholar 

  27. Wriggers P, Zavarise G (1997) On contact between three-dimensional beams undergoing large deflections. Commun Numer Methods Eng 13:429–438

    Article  MathSciNet  MATH  Google Scholar 

  28. Zavarise G, Wriggers P (2000) Contact with friction between beams in 3-D space. Int J Numer Methods Eng 49:977–1006

    Article  MATH  Google Scholar 

  29. Litewka P, Wriggers P (2002) Friction contact between 3D beams. Comput Mech 28:26–39

    Article  MATH  Google Scholar 

  30. Litewka P (2007) Hermite polynomial smoothing in beam-to-beam frictional contact. Comput Mech 40:815–826

    Article  MATH  Google Scholar 

  31. Konyukhov A, Schweizerhof K (2008) On the solvability of closest point projection procedures in contact analysis: analysis and solution strategy for surfaces of arbitrary geometry. Comput Methods Appl Mech Eng 197:3045–3056

    Article  MathSciNet  MATH  Google Scholar 

  32. Konyukhov A, Schweizerhof K (2010) Geometrically exact covariant approach for contact between curves. Comput Methods Appl Mech Eng 199:2510–2531

    Article  MathSciNet  MATH  Google Scholar 

  33. Litewka P (2013) Enhanced multiple-point beam-to-beam frictionless contact finite element. Comput Mech 52:1365–1380

    Article  MathSciNet  MATH  Google Scholar 

  34. Durville D (2012) Contact-friction modeling within elastic beam assemblies: an application to knot tightening. Comput Mech 49:687–707

    Article  MathSciNet  MATH  Google Scholar 

  35. Wriggers P (2002) Computational contact mechanics. Wiley, West Sussex

    Google Scholar 

  36. Belytschko T, Neal MO (1991) Contact-impact by the Pinball algorithm with penalty and Lagrangian methods. Int J Numer Methods Eng 31:547–572

    Article  MATH  Google Scholar 

  37. Gay Neto A, Pimenta PM, Wriggers P (2014) Contact between rolling beams and flat surfaces. Int J Numer Methods Eng 97:683–706

    Article  Google Scholar 

Download references

Acknowledgments

The authors acknowledge FAPESP (Fundação de Amparo à Pesquisa do Estado de São Paulo) for the support under the Grants 2012/09912-0 and 2014/11910-0. The second author acknowledges also the support by CNPq (Conselho Nacional de Desenvolvimento Científico e Tecnológico) under the Grant 303091/2013-4.

Author information

Authors and Affiliations

  1. Polytechnic School at University of São Paulo, São Paulo, Brazil

    Alfredo Gay Neto & Paulo M. Pimenta

  2. Leibniz Universität Hannover, Hannover, Germany

    Peter Wriggers

Authors
  1. Alfredo Gay Neto
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Paulo M. Pimenta
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Peter Wriggers
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Alfredo Gay Neto.

Electronic supplementary material

Below is the link to the electronic supplementary material.

Supplementary material 1 (mp4 2096 KB)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Gay Neto, A., Pimenta, P.M. & Wriggers, P. Self-contact modeling on beams experiencing loop formation. Comput Mech 55, 193–208 (2015). https://doi.org/10.1007/s00466-014-1092-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00466-014-1092-3

Keywords

Search

Navigation