Skip to main content
SpringerLink
Log in

Cohesive-zone-model formulation and implementation using the symmetric Galerkin boundary element method for homogeneous solids

  • Original Paper
  • Published:
Computational Mechanics Aims and scope Submit manuscript

Abstract

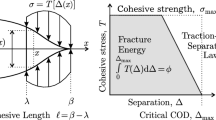
A new symmetric boundary integral formulation for cohesive cracks growing in the interior of homogeneous linear elastic isotropic media with a known crack path is developed and implemented in a numerical code. A crack path can be known due to some symmetry implications or the presence of a weak or bonded surface between two solids. The use of a two-dimensional exponential cohesive law and of a special technique for its inclusion in the symmetric Galerkin boundary element method allows us to develop a simple and efficient formulation and implementation of a cohesive zone model. This formulation is dependent on only one variable in the cohesive zone (relative displacement). The corresponding constitutive cohesive equations present a softening branch which induces to the problem a potential instability. The development and implementation of a suitable solution algorithm capable of following the growth of the cohesive zone and subsequent crack growth becomes an important issue. An arc-length control combined with a Newton–Raphson algorithm for iterative solution of nonlinear equations is developed. The boundary element method is very attractive for modeling cohesive crack problems as all nonlinearities are located along the boundaries (including the crack boundaries) of linear elastic domains. A Galerkin approximation scheme, applied to a suitable symmetric integral formulation, ensures an easy treatment of cracks in homogeneous media and excellent convergence behavior of the numerical solution. Numerical results for the wedge split and mixed-mode flexure tests are presented.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Needleman A (1987) A continuum model for void nucleation by inclusion debonding. J Appl Mech-T ASME 54: 525–532

    Article  MATH  Google Scholar 

  2. Camacho GT, Ortiz M (1996) Computational modelling of impact damage in brittle materials. Int J Solids Struct 33: 2899–2938

    Article  MATH  Google Scholar 

  3. Ortiz M, Pandolfi A (1999) Finite-deformation irreversible cohesive elements for three dimensional crack propagation analysis. Int J Numer Meth Eng 44: 1267–1283

    Article  MATH  Google Scholar 

  4. Xu XP, Needleman A (1993) Void nucleation by inclusion debonding in a crystal matrix. Model Simul Mater Sci 1: 111–132

    Article  Google Scholar 

  5. Chandra N, Shet C (2004) A micromechanistic perspective of cohesive zone approach in modeling fracture. CMES-Comp Model Eng 5(1): 21–34

    MATH  Google Scholar 

  6. Bažant Z, Planas J (1998) Fracture and size effect in concrete and other quasibrittle materials. CRC Press LLC, Boca Raton

    Google Scholar 

  7. Barenblatt GI (1959) The formation of equilibrium cracks during brittle fracture. General ideas and hypotheses. Axially-symmetric cracks. PMM-J Appl Math Mech 23: 622–636

    Article  MathSciNet  MATH  Google Scholar 

  8. Hilleborg A, Modéer M, Petersson PE (1976) Analysis of a crack formation and crack growth in concrete by fracture mechanics and finite elements. Cement Concr Res 6: 773–782

    Article  Google Scholar 

  9. Brebbia CA, Telles JCF, Wrobel LC (1984) Boundary element techniques. Springer, Berlin

    Book  MATH  Google Scholar 

  10. París F, Cañas J (1997) Boundary element method, fundamentals and applications. Oxford University Press, Oxford

    MATH  Google Scholar 

  11. Bonnet M (1999) Boundary integral equation methods for solids and fluids. Wiley, Chichester

    Google Scholar 

  12. Aliabadi MH (2002) The boundary element method vol 2—aplications in solids and structures. Wiley, Chichester

    Google Scholar 

  13. Sutradhar A, Paulino G, Gray L (2008) Symmetric Galerkin boundary element method. Springer, Berlin

    MATH  Google Scholar 

  14. Bonnet M, Maier G, Polizzotto C (1998) Symmetric Galerkin boundary element method. ASME Appl Mech Rev 51(11): 669–704

    Article  Google Scholar 

  15. Wang Z, Wu Q (1991) A new approach treating corners in boundary element method. In: Brebbia CA (ed) Boundary elements XIII. Springer, Berlin, pp 901–911

    Chapter  Google Scholar 

  16. Mitra AK, Ingber MS (1993) A new multiple-node method to resolve the difficulties in the BIEM caused by corners and discontinuous boundary conditions. Int J Numer Meth Eng 36: 1735–1746

    Article  MathSciNet  MATH  Google Scholar 

  17. Rjasanow S, Steinbach O (2007) The fast solution of boundary integral equations. Springer, New York

    MATH  Google Scholar 

  18. Sauter SA, Schwab C (2011) Boundary element methods. Springer, Berlin

    Book  MATH  Google Scholar 

  19. Gray LJ (1998) Evaluation of singular and hypersingular Galerkin boundary integrals: direct limits and symbolic computation. In: Sladek V, Sladek J (eds) Singular integrals in the boundary element method, advances in boundary elements. Computational Mechanics Publications, Southampton, pp 33–84

    Google Scholar 

  20. Kitey R, Phan AV, Tippur HV, Kaplan T (2006) Modeling of crack growth through particulate clusters in brittle matrix by symmetric-Galerkin boundary element method. Int J Fract 141(1): 11–25

    Article  MATH  Google Scholar 

  21. Wriggers P (2002) Computational contact mechanics. Springer, Berlin

    Google Scholar 

  22. Fafard M, Massicotte B (1993) Geometrical interpretation of the arc-length method. Comput Struct 46: 603–615

    Article  MathSciNet  MATH  Google Scholar 

  23. Crisfield MA (1997) Non-linear finite element analysis of solids and structures, vol 2. Wiley, Chichester

    Google Scholar 

  24. Ragon SA, Gürdal Z, Watson LT (2002) A comparison of three algorithms for tracing nonlinear equilibrium paths of structural systems. Int J Solids Struct 39: 689–698

    Article  MATH  Google Scholar 

  25. Mallardo V, Alessandri C (2004) Arc-length procedures with BEM in physically nonlinear problems. Eng Anal Bound Elem 28: 547–559

    Article  MATH  Google Scholar 

  26. Mallardo V (2009) Integral equations and nonlocal damage theory: a numerical implementation using the BDEM. Int J Fract 157: 13–32

    Article  MATH  Google Scholar 

  27. Yang B, Ravi-Chandar K (1998) A single-domain dual-boundary-element formulation incorporating a cohesive zone model for elastostatic cracks. Int J Fract 22: 381–391

    Google Scholar 

  28. Aliabadi MH, Saleh AL (1998) Fracture mechanics analysis of cracking in plain and reinforced concrete using boundary element method. Eng Fract Mech 93: 115–144

    Article  Google Scholar 

  29. Saleh AL, Aliabadi MH (1995) Three-dimensional crack-growth simulation using BEM. Eng Fract Mech 51: 533–545

    Article  Google Scholar 

  30. Maier G, Novati G, Cen Z (1993) Symmetric boundary element method for quasi-brittle fracture and frictional problems. Comput Mech 13: 74–89

    MATH  Google Scholar 

  31. Bolzon G, Fedele R, Maier G (2002) Parameter identification of a cohesive crack model by Kalman filter. Comput Method Appl Meach 13: 74–89

    Google Scholar 

  32. Maier G, Frangi A (1998) Symmetric boundary element method for “discrete” crack modelling of fracture processes. Comput Assist Mech Eng Sci 5: 201–226

    MathSciNet  MATH  Google Scholar 

  33. Salvadori A (2003) A symmetric boundary integral formulation for cohesive interface problems. Comput Mech 22: 381–391

    Article  MathSciNet  Google Scholar 

  34. Távara L, Mantič V, Salvadori A, Gray LJ, París F (2010) SGBEM for cohesive cracks in homogeneous media. Key Eng Mater 454: 1–10

    Article  Google Scholar 

  35. Rizzo FJ (1967) An integral equation approach to boundary value problems of classical elastostatics. Q Appl Math 25: 83–95

    MATH  Google Scholar 

  36. Cruse TA (1988) Boundary element analysis in computational fracture mechanics. Kluwer, Boston

    Book  MATH  Google Scholar 

  37. Mantič V, París F (1997) Symmetry properties of the kernels of the hypersingular integral and the corresponding regularized integral in the 2D Somigliana stress identity for isotropic materials. Eng Anal Bound Elem 20: 163–168

    Article  Google Scholar 

  38. Sirtori S (1979) General stress analysis method by means of integral equations and boundary elements. Meccanica 14: 210–218

    Article  MATH  Google Scholar 

  39. Hartmann F, Katz C, Protopsaltis B (1985) Boundary elements and symmetry. Ingenieur-Archiv 55: 440–449

    Article  MATH  Google Scholar 

  40. Maier G, Polizzotto C (1987) A Galerkin approach to boundary element elastoplastic analysis. Comput Method Appl Meach 60: 175–194

    Article  MATH  Google Scholar 

  41. Maier G, Novati G, Sirtori S (1990) On symmetrization in boundary element elastic and elastoplastic analysis. In: Kuhn G, Mang H (eds) Discretization methods in structural mechanics. Springer, Berlin, pp 191–200

    Chapter  Google Scholar 

  42. Sirtori S, Maier G, Novati G, Miccoli S (1992) A Galerkin symmetric boundary element method in elasticity: formulation and implementation. Int J Numer Meth Eng 35: 255–282

    Article  MathSciNet  MATH  Google Scholar 

  43. Maier G, Miccoli S, Novati G, Sirtori S (1993) A Galerkin symmetric boundary element method in plasticity: formulation and implementation. In: Kane JH, Maier G, Tosaka N, Atluri SN (eds) Advances in boundary element techniques. Springer, Berlin, pp 288–328

    Chapter  Google Scholar 

  44. Salvadori A (2006) Numerical simulations of cohesive interface problems via boundary integral equations. Proceedings of IABEM 2006, Graz

  45. Hölzer SM (1993) How to deal with hypersingular integrals in the symmetric BEM. Commun Numer Meth Eng 9: 219–232

    Article  Google Scholar 

  46. Frangi A, Novati G (1996) Symmetric BE method in two-dimensional elasticity: evaluation of double integrals for curved elements. Comput Mech 19: 58–68

    Article  MathSciNet  MATH  Google Scholar 

  47. Nintcheu Fata S (2009) Explicit expressions for 3D boundary integrals in potential theory. Int J Numer Meth Eng 78(1): 32–47

    Article  MathSciNet  MATH  Google Scholar 

  48. Salvadori A (2010) Analytical integrations in 3D BEM for elliptic problems: evaluation and implementation. Int J Numer Meth Eng 84: 505–542

    MathSciNet  MATH  Google Scholar 

  49. Salvadori A (2002) Analytical integrations in 2D BEM elasticity. Int J Numer Meth Eng 53(7): 1695–1719

    Article  MathSciNet  MATH  Google Scholar 

  50. Salvadori A, Gray L (2007) Analytical integrations and SIFs computation in 2D fracture mechanics. Int J Numer Meth Eng 70: 445–495

    Article  MathSciNet  MATH  Google Scholar 

  51. Phan AV (2012) A non-singular boundary integral formula for frequency domain analysis of the dynamic T-stress. Int J Fract 173(1): 37–48

    Article  Google Scholar 

  52. Ruiz G, Pandolfi A, Ortiz M (2001) Three-dimensional cohesive modeling of dynamic mixed-mode fracture. Int J Numer Meth Eng 52: 97–120

    Article  Google Scholar 

  53. Gray LJ, Paulino GH (1997) Symmetric Galerkin boundary integral fracture analysis for plane orthotropic elasticity. Comput Mech 20: 26–33

    Article  MATH  Google Scholar 

  54. Zienkiewicz OC, Taylor RL (2000) The finite element method: the basis, vol 1, 5th edn. Oxford Press, Oxford

    Google Scholar 

  55. Remmers JC, Borst R, Needleman A (2008) The simulation of dynamic crack propagation using the cohesive segments method. J Mech Phys Solids 56: 70–92

    Article  MathSciNet  MATH  Google Scholar 

  56. Riks E (1979) An incremental approach to the solution of snapping and buckling problems. Int J Solids Struct 15: 529–551

    Article  MathSciNet  MATH  Google Scholar 

  57. Ramm E (1981) Strategies for tracing the non-linear response near limit-points. In: Wunderlich W, Stein E, Bathe KJ (eds) Non-linear finite element analysis in structural mechanics. Procededing European US workshop. Springer, Berlin

    Google Scholar 

  58. Denarié E, Saouma VE, Iocco A, Varelas D (2001) Concrete fracture process zone characterization with fiber optics. J Eng Mech-ASCE 127(5): 494–502

    Article  Google Scholar 

  59. Valaroso N, Champaney L (2006) A damage-mechanics-based approach for modelling decohesion in adhesively bonded assemblies. Eng Fract Mech 73: 2774–2801

    Article  Google Scholar 

  60. Vodička R, Mantič V, París F (2006) Note on the removal of rigid body motions in the solution of elastostatic traction boundary value problems by SGBEM. Eng Anal Bound Elem 30: 790–798

    Article  MATH  Google Scholar 

  61. Blázquez A, Mantič V, París F, Cañas J (1996) On the removal of rigid body motions in the solution of elastostatic problems by direct BEM. Int J Numer Meth Eng 39: 4021–4038

    Article  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Grupo de Elasticidad y Resistencia de Materiales, Universidad de Sevilla, Camino de los Descubrimientos s/n, 41092, Sevilla, Spain

    Luis Távara, Vladislav Mantič & Federico París

  2. Department of Civil Engineering, University of Brescia, Via Branze 38, 25123, Brescia, Italy

    Alberto Salvadori

  3. BSSI A/S, Bergen, Norway

    Leonard J. Gray

Authors
  1. Luis Távara
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Vladislav Mantič
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Alberto Salvadori
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Leonard J. Gray
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Federico París
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Luis Távara.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Távara, L., Mantič, V., Salvadori, A. et al. Cohesive-zone-model formulation and implementation using the symmetric Galerkin boundary element method for homogeneous solids. Comput Mech 51, 535–551 (2013). https://doi.org/10.1007/s00466-012-0808-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00466-012-0808-5

Keywords

Search

Navigation