Random Sequential Adsorption of Discs on Surfaces of Constant Curvature: Plane, Sphere, Hyperboloid, and Projective Plane

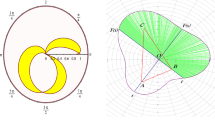
Abstract

We present an algorithm to simulate random sequential adsorption (random “parking”) of discs on constant curvature surfaces: the plane, sphere, hyperboloid, and projective plane, all embedded in three-dimensional space. We simulate complete parkings efficiently by explicitly calculating the boundary of the available area in which discs can park and concentrating new points in this area. We use our algorithm to study the number distribution and density of discs parked in each space, where for the plane and hyperboloid we consider two different periodic tilings each. We make several notable observations: (1) on the sphere, there is a critical disc radius such the number of discs parked is always exactly four: the random parking is deterministic. We prove this statement and also show that random parking on the surface of a d-dimensional sphere would have deterministic behaviour at the same critical radius. (2) The average number of parked discs does not always monotonically increase as the disc radius decreases: on the plane (square with periodic boundary conditions), there is an interval of decreasing radius over which the average decreases. We give a heuristic explanation for this counterintuitive finding. (3) As the disc radius shrinks to zero, the density (average fraction of area covered by parked discs) appears to converge to the same constant for all spaces, though it is always slightly larger for a sphere and slightly smaller for a hyperboloid. Therefore, for parkings on a general curved surface we would expect higher local densities in regions of positive curvature and lower local densities in regions of negative curvature.

Appendix l: Tiles

1.1 Geometry of the Surfaces

The geometric structures of the spaces we consider is given by thinking of them as collections of either «point vectors»or «line vectors»; there is a duality between these spaces. «Point vectors»$\langle x,y,z \rangle$ live in «point space», «line vectors»$\langle u,v,w \rangle$ live in «line space». Sometimes we need to convert between «point vectors»and «line vectors».

1.2 Possible Tilings

The table below gives a subset of the possible tilings for different spaces. Recall a [p, q]-tiling consists of p-polygons, meeting q at each vertex. The patterns below generalize to infinite families of tilings.

1.3 Tiling Representations and Formulae

The central tile (a [p, q]-tile) has face centre $\langle 0,0,1 \rangle$ (the origin), vertices $\varvec{\upsilon }$, edge midpoints $\varvec{\epsilon }$, and neighbour tiles with face centres $\varvec{\digamma }\!$. These objects are all «point vectors»which live in «point space». They have distances $\upsilon$, $\epsilon$, $\digamma \! = 2\epsilon$ (respectively) from the («point space») origin $\langle 0,0,1 \rangle$. The inradius of the tile is $\epsilon$, and the circumradius is $\upsilon$.

The coordinates of these are given by the following, where $\theta$ is any odd multiple of ${\pi \over p}$, and $\vartheta$ is any even multiple of ${\pi \over p}$:

The central tile has edge normals $\varvec{\eta }\!$. These are «line vectors»which live in «line space». They have distance $\eta = \epsilon$ from the («point space») origin $\langle 0,0,1 \rangle$. $\varvec{\eta }$ «line vectors»are the line$\cdot$ normalized cross$\cdot$ product of two adjacent $\varvec{\upsilon }$ «point vectors». Conversely/dually $\varvec{\upsilon }$ «point vectors»are the point$\cdot$ normalized cross$\cdot$ product of two adjacent $\varvec{\eta }$ «line vectors». The edge normal vectors are computed as shown in the table below, where $\vartheta$ runs over all integer multiples of ${2\pi \over p}$.

To calculate the inradius $\epsilon$ and circumradius $\upsilon$, it is helpful to think about triangulating the tile polygons. Let the triangles be $\varvec{\chi }$ with angles $\{\chi _{\varvec{\digamma }}^{},\chi _{\varvec{\epsilon }}^{},\chi _{\varvec{\upsilon }}^{}\} = \{{\pi \over p},{\pi \over 2},{\pi \over q}\}$ at the tile face centre $\langle 0,0,1 \rangle$, vertices $\varvec{\upsilon }\!$, edge midpoints $\varvec{\epsilon }$, and neighbour tile face centres $\varvec{\digamma }\!$. The triangle edge lengths can be found by using basic trigonometry.

Each triangle $\varvec{\chi }$ has area $\chi$, and each tile has area $X = 2p\chi$. For the plane, $\chi$ can scale freely. For the other tilings, $\chi$ is determined by the angles, as follows:

The circumradius $\upsilon \!$ of a tile can be determined by the law of sines, once we know the inradius $\epsilon$. This in turn is determined by the law of cosines, except for the plane, where we can choose it arbitrarily. The formulae are:

For the cases we have implemented, we have

Note that for the plane, $\epsilon$ & $\upsilon$ can scale freely; however, their ratio is always fixed; we choose $\epsilon = 1$.

For a given point in the central tile, we will need to find the corresponding points in the translated tiles. This is done by translating the point along geodesics on the (point space) surface, which is practice is done by multiplying the point by a matrix. The symmetry group is generated by translations $\varvec{\tau }_{\varvec{\digamma }\!}$ (from the origin $\langle 0,0,1 \rangle$ to the neighbour tile face centres $\varvec{\digamma }\!$). A translation $\varvec{\tau }_{\langle \alpha \!,\beta \!,\!\gamma \rangle }$ from the origin $\langle 0,0,1 \rangle$ to the point $\langle \alpha \!,\beta \!,\!\gamma \rangle$ has inverse $\varvec{\sigma }_{\langle \alpha \!,\beta \!,\!\gamma \rangle } = \varvec{\tau }_{\langle {-}\alpha ,{-}\beta ,\gamma \rangle }$, where these are computed as

These three families have several nice properties. At each vertex $\varvec{\upsilon }$, there are no “duplicate” vertices which map to it under the translations or products of translations $\varvec{\sigma }_{\varvec{\digamma }\!}$ (or inverses $\varvec{\tau }_{\varvec{\digamma }\!}$). Exactly 2 tiles share each edge, which are opposite edges. Thus, the p-polygons must have an even number of sides. Since p is even, this means that polygons can be translated between neighbours. Thus, there is no conflict/collision among points parked near edges or vertices. Also, the translations about each vertex $\varvec{\upsilon }$ are compatible: if you perform q translations, then you will end up in the original tile, with the original orientation.

In what follows, for the sphere, when we say “translations”, we really mean $\{\mathrm{identity}\}$; in other words, there is no need to translate points. For the , when we say “translations”, we really mean $\{{\pm }\mathrm{identity}\}$: each parked point on a unit sphere implies a second parked point diametrically opposite. Otherwise, these have the same properties as the other tilings.

1.4 Tile Neighbours

The list below shows the number of translated tiles we used, for small, medium, and large radius (the cases are separated by dots). These numbers were found by trial and error to be sufficient that the number of geometrical errors was small enough. We did not keep track of the exact cut-off radius.

Note that especially for small discs $2\rho < \epsilon$, we need not consider all discs in neighbour tiles. When the neighbour tile shares a vertex, we consider only disc centres located less than $\varsigma$ away from the vertex («point vector»). When the neighbour tile shares an edge, we consider only disc centres located less than $\varsigma$ away from the edge («line vector»).

For each tiling, we list the nearest vertex vectors $\varvec{\upsilon }$

For each tiling, we list the nearest edge midpoint vectors $\varvec{\epsilon }$

For each tiling, we list the neighbour face centre vectors $\varvec{\digamma }\!$

For each tiling, we list the nearest edge normal vectors $\varvec{\eta }$

For each tiling, we list the neighbour translation matrices $\varvec{\sigma }_{\varvec{\digamma }\!}$