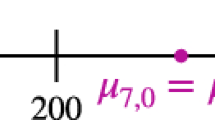Abstract
The long-time behavior of an initial step resulting in a dispersive shock wave (DSW) for the one-dimensional isentropic Euler equations regularized by generic, third-order dispersion is considered by use of Whitham averaging. Under modest assumptions, the jump conditions (DSW locus and speeds) for admissible, weak DSWs are characterized and found to depend only upon the sign of dispersion (convexity or concavity) and a general pressure law. Two mechanisms leading to the breakdown of this simple wave DSW theory for sufficiently large jumps are identified: a change in the sign of dispersion, leading to gradient catastrophe in the modulation equations, and the loss of genuine nonlinearity in the modulation equations. Large amplitude DSWs are constructed for several particular dispersive fluids with differing pressure laws modeled by the generalized nonlinear Schrödinger equation. These include superfluids (Bose–Einstein condensates and ultracold fermions) and “optical fluids.” Estimates of breaking times for smooth initial data and the long-time behavior of the shock tube problem are presented. Detailed numerical simulations compare favorably with the asymptotic results in the weak to moderate amplitude regimes. Deviations in the large amplitude regime are identified with breakdown of the simple wave DSW theory.













Similar content being viewed by others
References
Ablowitz, M.J., Baldwin, D.E., Hoefer, M.A.: Soliton generation and multiple phases in dispersive shock and rarefaction wave interaction. Phys. Rev. E 80(1), 016603 (2009)
Barsi, C., Wan, W., Sun, C., Fleischer, J.W.: Dispersive shock waves with nonlocal nonlinearity. Opt. Lett. 32(20), 2930–2932 (2007)
Barsi, C., Wan, W., Jia, S., Fleischer, J.: Spatially dispersive shock waves in nonlinear optics. In: Chen, Z., Morandotti, R. (eds.) Nonlinear Photonics and Novel Optical Phenomena. Springer Series in Optical Sciences, vol. 170, pp. 231–257. Springer, Berlin/Heidelberg (2012)
Biondini, G., Kodama, Y.: On the Whitham equations for the defocusing nonlinear Schroödinger equation with step initial data. J. Nonlinear Sci. 16, 435–481 (2006)
Chang, J.J., Engels, P., Hoefer, M.A.: Formation of dispersive shock waves by merging and splitting Bose-Einstein condensates. Phys. Rev. Lett. 101, 170404 (2008)
Chanson, H.: Current knowledge in hydraulic jumps and related phenomena: a survey of experimental results. Eur. J. Mech. B 28(2), 191–210 (2009)
Chen, T., Pavlovic, N.: The quintic NLS as the mean field limit of a boson gas with three-body interactions. J. Funct. Anal. 260(4), 959–997 (2011)
Christie, D.R.: The morning glory of the gulf of Carpentaria: a paradigm for non-linear waves in the lower atmosphere. Austral. Met. Mag. 41, 21–60 (1992)
Christodoulides, D.N., Carvalho, M.I.: Bright, dark, and gray spatial soliton states in photorefractive media. J. Opt. Soc. Am. B 12(9), 1628–1633 (1995)
Conforti, M., Baronio, F., Trillo, S.: Dispersive shock waves in phase-mismatched second-harmonic generation. Opt. Lett. 37(6), 1082–1084 (2012)
Conti, C., Fratalocchi, A., Peccianti, M., Ruocco, G., Trillo, S.: Observation of a gradient catastrophe generating solitons. Phys. Rev. Lett. 102(8), 083902 (2009)
Courant, R., Friedrichs, K.O.: Supersonic Flow and Shock Waves. Springer, New York (1948)
Crosta, M., Fratalocchi, A., Trillo, S.: Bistability and instability of dark-antidark solitons in the cubic-quintic nonlinear Schroödinger equation. Phys. Rev. A 84(6), 011604 (2011)
Crosta, M., Trillo, S., Fratalocchi, A.: Crossover dynamics of dispersive shocks in Bose-Einstein condensates characterized by two- and three-body interactions. Phys. Rev. A 85(4), 011604 (2012)
Crosta, M., Trillo, S., Fratalocchi, A.: The Whitham approach to dispersive shocks in systems with cubicquintic nonlinearities. New J. Phys. 14(9), 093019 (2012)
Csordás, A., Almásy, O., Szépfalusy, P.: Gradient corrections to the local-density approximation for trapped superfluid Fermi gases. Phys. Rev. A 82(6), 063609 (2010)
Dafermos, C.M.: Hyperbolic Conservation Laws in Continuum Physics, 3rd edn. Springer, New York (2009)
Dias, F., Milewski, P.: On the fully-nonlinear shallow-water generalized Serre equations. Phys. Lett. A 374(8), 1049–1053 (2010)
Dutton, Z., Budde, M., Slowe, C., Hau, L.V.: Observation of quantum shock waves created with ultra-compressed slow light pulses in a Bose-Einstein condensate. Science 293, 663 (2001)
El, G.A., Krylov, A.L.: General solution of the Cauchy problem for the defocusing NLS equation in the Whitham limit. Phys. Lett. A 203, 77–82 (1995)
El, G.A., Geogjaev, V.V., Gurevich, A.V., Krylov, A.L.: Decay of an initial discontinuity in the defocusing NLS hydrodynamics. Phys. D 87, 186–192 (1995)
El, G.A.: Generating function of the Whitham-KdV hierarchy and effective solution of the Cauchy problem. Phys. Lett. A 222, 393–399 (1996)
El, G.A.: Resolution of a shock in hyperbolic systems modified by weak dispersion. Chaos 15, 037103 (2005)
El, G.A., Grimshaw, R.H.J., Smyth, N.F.: Unsteady undular bores in fully nonlinear shallow-water theory. Phys. Fluids 18(2), 027104–027117 (2006)
El, G.A., Gammal, A., Khamis, E.G., Kraenkel, R.A., Kamchatnov, A.M.: Theory of optical dispersive shock waves in photorefractive media. Phys. Rev. A 76(5), 053813 (2007)
Esler, J.G., Pearce, J.D.: Dispersive dam-break and lock-exchange flows in a two-layer fluid. J. Fluid Mech. 667, 555–585 (2011)
Forest, M.G., McLaughlin, K.T.-R.: Onset of oscillations in nonsoliton pulses in nonlinear dispersive fibers. J. Nonlinear Sci. 8(1), 43–62 (1998)
Gavrilyuk, S.L.: Large amplitude oscillations and their ’thermodynamics’ for continua with ’memory’. Eur. J. Mech. B 13, 753–764 (1994)
Gavrilyuk, S.L., Teshukov, V.M.: Generalized vorticity for bubbly liquid and dispersive shallow water equations. Contin. Mech. Therm. 13, 365–382 (2001)
Ghofraniha, N., Conti, C., Ruocco, G., Trillo, S.: Shocks in nonlocal media. Phys. Rev. Lett. 99, 043903 (2007)
Ghofraniha, N.: Amato Santamaria, L., Folli, V., Trillo, S., DelRe, E., Conti, C.: Measurement of scaling laws for shock waves in thermal nonlocal media. Opt. Lett. 37(12), 2325–2327 (2012)
Giorgini, S., Pitaevskii, L.P., Stringari, S.: Theory of ultracold atomic Fermi gases. Rev. Mod. Phys. 80(4), 1215 (2008)
Grava, T., Tian, F.-R.: The generation, propagation, and extinction of multiphases in the KdV zero-dispersion limit. Commun. Pure Appl. Math. 55(12), 1569–1639 (2002)
Grava, T., Pierce, V.U., Tian, F.-R.: Initial value problem of the Whitham equations for the Camassa-Holm equation. Phys. D 238(1), 55–66 (2009)
Green, A.E., Naghdi, P.M.: A derivation of equations for wave propagation in water of variable depth. J. Fluid Mech. 78(02), 237–246 (1976)
Gurevich, A.V., Pitaevskii, L.P.: Nonstationary structure of a collisionless shock wave. Sov. Phys. JETP 38(2), 291–297 (1974). (Translation from Russian of A. V. Gurevich and L. P. Pitaevskii. Zh. Eksp. Teor. Fiz. 65, 590–604 (1973)
Gurevich, A.V., Meshcherkin, A.P.: Expanding self-similar discontinuities and shock waves in dispersive hydrodynamics. Sov. Phys. JETP 60(4), 732–740 (1984)
Gurevich, A.V., Krylov, A.L.: Dissipationless shock waves in media with positive dispersion. Sov. Phys. JETP 65(5), 944–953 (1987)
Gurevich, A.V., Krylov, A.L., El, G.A.: Nonlinear modulated waves in dispersive hydrodynamics. Sov. Phys. JETP 71, 899–910 (1990)
Hoefer, M.A., Ablowitz, M.J., Coddington, I., Cornell, E.A., Engels, P., Schweikhard, V.: Dispersive and classical shock waves in Bose-Einstein condensates and gas dynamics. Phys. Rev. A 74, 023623 (2006)
Hoefer, M.A., Ablowitz, M.J.: Interactions of dispersive shock waves. Phys. D 236, 44–64 (2007)
Hoefer, M.A., Ablowitz, M.J.: Dispersive shock waves. Scholarpedia 4(11), 5562 (2009)
Holloway, P., Pelinovsky, E., Talipova, T.: Internal tide transformation and oceanic internal solitary waves. In: Grimshaw, R.H.J. (ed.) Environmental Stratified Flows, pp. 29–60. Kluwer, Boston (2001)
Jia, S., Wan, W., Fleischer, J.W.: Dispersive shock waves in nonlinear arrays. Phys. Rev. Lett. 99(22), 223901–223904 (2007)
Jia, S., Haataja, M., Fleischer, J.W.: Rayleigh-Taylor instability in nonlinear Schroödinger flow. New J. Phys. 14(7), 075009 (2012)
Jin, S., Levermore, C.D., McLaughlin, D.W.: The semiclassical limit of the defocusing NLS hierarchy. Commun. Pure Appl. Math. 52(5), 613–654 (1999)
Joseph, J.A., Thomas, J.E., Kulkarni, M., Abanov, A.G.: Observation of shock waves in a strongly interacting Fermi gas. Phys. Rev. Lett. 106(15), 150401 (2011)
Kamchatnov, A.M., Kuo, Y.-H., Lin, T.-C., Horng, T.-L., Gou, S.-C., Clift, R., El, G.A., Grimshaw, R.H.J.: Undular bore theory for the Gardner equation. Phys. Rev. E 86, 036605 (2012)
Karpman, V.I.: Non-linear Waves in Dispersive Media. Pergamon Press, Oxford (1974)
Ketterle, W., Zwierlein, M.W.: Making, probing and understanding ultracold Fermi gases. Riv. Nuovo Cimento 31(5), 247–422 (2008)
Kevrekidis, P.G., Frantzeskakis, D.J., Carretero-Gonzlez, R.: Emergent Nonlinear Phenomena in Bose-Einstein Condensates : Theory and Experiment. Springer Series on Atomic, Optical, and Plasma Physics, vol. 45, pp. 1615–5653. Springer, Berlin (2008).
Khamis, E.G., Gammal, A., El, G.A., Yu, G.G., Kamchatnov, A.M.: Nonlinear diffraction of light beams propagating in hotorefractive media with embedded reflecting wire. Phys. Rev. A 78(1), 013829 (2008)
Kodama, Y., Pierce, V.U., Tian, F.-R.: On the Whitham equations for the defocusing complex modified KdV equation. SIAM J. Math. Anal. 41(1), 26–58 (2008)
Lax, P.D.: Development of singularities of solutions of nonlinear hyperbolic partial differential equations. J. Math. Phys. 5(5), 611–613 (1964)
Lax, P.D.: Hyperbolic Systems of Conservation Laws and the Mathematical Theory of Shock Waves. SIAM, Philadelphia (1973)
Lax, P.D., Levermore, C.D.: The small dispersion limit of the Korteweg-de Vries equation: 1. Commun. Pure Appl. Math. 36(3), 253–290 (1983)
Lax, P.D., Levermore, C.D.: The small dispersion limit of the Korteweg-de Vries equation: 2. Commun. Pure Appl. Math. 36(5), 571–593 (1983)
Lax, P.D., Levermore, C.D.: The small dispersion limit of the Korteweg-de Vries equation: 3. Commun. Pure Appl. Math. 36(6), 809–830 (1983)
Leach, J.A., Needham, D.J.: The large-time development of the solution to an initial-value problem for the Korteweg-de Vries equation: I. initial data has a discontinuous expansive step. Nonlinearity 21(10), 2391–2408 (2008)
Liepmann, H.W., Roshko, A.: Elements of Gasdynamics. Wiley, New York (1957)
Lowman, N.K., Hoefer, M.A.: Dispersive shock waves in viscously deformable media. J. Fluid Mech. 718, 524–557 (2013)
Madelung, E.: Quantentheorie in hydrodynamischer form. Zeitschrift fur Physik 40(3–4), 322–326 (1927)
Marchant, T., Smyth, N.: Undular bore solution of the Camassa-Holm equation. Phys. Rev. E 73(5), 057602 (2006)
Mateo, Muaz: A.: Effective mean-field equations for cigar-shaped and disk-shaped Bose-Einstein condensates. Phys. Rev. A 77(1), 013617 (2008).
Meppelink, R., Koller, S.B., Vogels, J.M., van der Straten, P., van Ooijen, E.D., Heckenberg, N.R., Rubinsztein-Dunlop, H., Haine, S.A., Davis, M.J.: Observation of shock waves in a large Bose-Einstein condensate. Phys. Rev. A 80(4), 043606–043607 (2009)
Novikov, S., Manakov, S.V., Pitaevskii, L.P., Zakharov, V.E.: Theory of Solitons. Consultants Bureau, New York (1984)
Pierce, V.U., Tian, F.-R.: Self-similar solutions of the non-strictly hyperbolic Whitham equations. Commun. Math. Sci. 4, 799–822 (2006)
Pierce, V.U., Tian, F.-R.: Large time behavior of the zero dispersion limit of the fifth order KdV equation. Dyn. PDE 4(1), 87–109 (2007)
Pierce, V.U., Tian, F.-R.: Self-similar solutions of the non-strictly hyperbolic Whitham equations for the KdV hierarchy. Dyn. PDE 4(3), 263–282 (2007)
Rottman, J.W., Grimshaw, R.H.J.: Atmospheric internal solitary waves. In: Grimshaw, R.H.J. (ed.) Environmental Stratified Flows, pp. 61–88. Kluwer, Boston (2001)
Salasnich, L., Parola, A., Reatto, L.: Effective wave equations for the dynamics of cigar-shaped and disk-shaped Bose condensates. Phys. Rev. A 65(4), 043614 (2002)
Salasnich, L.: Dynamical properties of the unitary Fermi gas: collective modes and shock waves. Few-Body Syst. (2012). doi:10.1007/s00601-012-0442-y
Segev, M., Crosignani, B., Yariv, A., Fischer, B.: Spatial solitons in photorefractive media. Phys. Rev. Lett. 68(7), 923 (1992)
Serre, F.: Contribution a l’etude des ecoulements permanents et variables dans les canaux. La Houille Blanche 3, 374–388 (1953)
Simula, T.P., Engels, P., Coddington, I., Schweikhard, V., Cornell, E.A., Ballagh, R.J.: Observations on sound propagation in rapidly rotating Bose-Einstein condensates. Phys. Rev. Lett. 94, 080404 (2005)
Smith, R.K.: Waves and bores in the lower atmosphere: the “morning glory” and related phenomena. Earth-Sci. Rev. 25, 1501–1518 (1988)
Smoller, J.: Shock Waves and Reaction-Diffusion Equations. Springer, New York (1994)
Strang, G.: On the construction and comparison of difference schemes. SIAM J. Numer. Anal. 5(3), 506–517 (1968)
Su, C.H., Gardner, C.S.: Korteweg-deVries equation and generalizations. III. Derivation of the Korteweg-deVries equation and Burgers equation. J. Math. Phys. 10(3), 536–539 (1969)
Sulem, C., Sulem, P.-L.: The Nonlinear Schrödinger Equation. Springer, New York (1999)
Taylor, R.J., Baker, D.R., Ikezi, H.: Observation of collisionless electrostatic shocks. Phys. Rev. Lett. 24, 206–209 (1970)
Temple, B.: Systems of conservation laws with coinciding shock and rarefaction curves. Contemp. Math 17(143), 151 (1983)
Tsarev, S.P.: Poisson brackets and one-dimensional Hamiltonian systems of hydrodynamic type. Sov. Math. Dokl. 31, 488–491 (1985)
Venakides, S.: The zero-dispersion limit of the Korteweg-de Vries equation with non-trivial reflection coefficient. Commun. Pure Appl. Math. 38, 125–155 (1985)
Wagner, D.H.: Equivalence of the Euler and Lagrangian equations of gas dynamics for weak solutions. J. Diff. Equ. 68(1), 118–136 (1987)
Wan, W., Jia, S., Fleischer, J.W.: Dispersive superfluid-like shock waves in nonlinear optics. Nat. Phys. 3(1), 46–51 (2007)
Wan, W., Muenzel, S., Fleischer, J.W.: Wave tunneling and hysteresis in nonlinear junctions. Phys. Rev. Lett. 104(7), 073903 (2010)
Whitham, G.B.: Non-linear dispersive waves. Proc. Roy. Soc. Ser. A 283, 238–261 (1965)
Whitham, G.B.: Linear and Nonlinear Waves. Wiley, New York (1974)
Wijngaarden, L.V.: One-dimensional flow of liquids containing small gas bubbles. Ann. Rev. Fluid Mech. 4, 369–396 (1972)
Acknowledgments
The author gratefully acknowledges financial support from the National Science Foundation via DMS-1008973.
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by M. I. Weinstein.
Appendix: Numerical Methods
Appendix: Numerical Methods
The numerical solution of the gNLS equation (3.1) for the shock tube problem, the initial step in density
is briefly described here. A pseudospectral, time-splitting method is implemented for the accurate solution of long-time evolution for \(x\in (0,L)\). The initial data (11.1) are smoothed by use of the hyperbolic tangent initial condition
where \(x_0 = L/2\). Time stepping proceeds by use of second-order Strang splitting Strang (1968) where the linear PDE
is advanced half a time step \(\Delta t/2\) exactly followed by a full time step of the nonlinear ODE
The linear PDE is then advanced half a time step with the initial data \(\psi _\mathrm {L}(x,t+\Delta t/2) = \psi _{\mathrm {NL}}(x,t+\Delta t)\), giving the second-order accurate approximation of \(\psi (x,t+\Delta t) \approx \psi _\mathrm {L}(x, \Delta t)\). Equation (11.2) is projected onto a truncated cosine basis of \(N\) terms that maintains Neumann (\(\psi _x = 0\)) boundary conditions, computed efficiently via the fast Fourier transform (FFT), and integrated explicitly in time. The nonlinear ODE (11.3) conserves \(|\psi _{\mathrm {NL}}|^2\) so is also integrated explicitly in time. The parameter \(\Delta x = L/N\) is the spatial grid spacing of the grid points \(x_j = \Delta x (j - 1/2)\), \(j = 1,2,\ldots ,N\). The accuracy of the solution is monitored by computing the relative deviation in the conserved \(L^2\) norm \(E(t) = \int _{\mathbb {R}} | \psi (x,t) |^2 \mathrm{d} x\), \(E_{\mathrm {rel}} = |E(t_\mathrm {f})-E(0)|/E(0)\) where \(t_\mathrm {f}\) is the final time. All computations presented here exhibit \(E_{\mathrm {rel}} < 10^{-8}\). Also, the accurate spatial resolution of the oscillatory structures is supported by the fact that the coefficient of the largest wavenumber in the cosine series is less than \(5 \cdot 10^{-10}\) (oftentimes much less). The numerical parameters \(L\), \(N\), \(\Delta t\), and \(t_\mathrm {f}\) vary depending upon the nonlinearity strength and jump height; For example, for power-law gNLS with \(p = 2\) and \(\rho _2 \ge 11\), \(N = 2^{16}\), \(L = 1,200\), \(\Delta t = 0.0002\), and \(t_\mathrm {f} = 30\), whereas for \(p = 2/3\) with \(\rho _2 = 2\), \(N = 3 \cdot 2^{14}\), \(L = 3,000\), \(\Delta t = 0.002\), and \(t_\mathrm {f} = 500\).
The extraction of the DSW speeds \(v_+\), \(s_+\), and minimum density \(\rho _\mathrm {min}\) is performed as follows: The precise location of the DSW soliton trailing edge is computed by creating a local cubic spline interpolant through the computed grid points in the neighborhood of the dark soliton minimum. A root finder is applied to the derivative of this interpolant in order to extract the off-grid location of the soliton edge \(x_s(t)\) and \(\rho _\mathrm {min}\equiv |\psi (x_s(t_\mathrm {f}),t_\mathrm {f})|^2\). The slope of a linear least-squares fit through \(x_s(t_j)\) for \(j = 1, \ldots , 100\) equispaced \(t_j \in [t_\mathrm {f}-1,t_\mathrm {f}]\) determines \(s_+\). For the leading, linear wave edge, an envelope function is determined by least-squares fitting two lines, each through about 30 local maxima and minima, respectively, of the DSW density in the vicinity of the trailing edge. The extrema are computed the same as for the soliton minimum. The point of intersection of these two lines is the location of the linear wave edge \(x_v(t)\). The same fitting procedure as was used to determine \(s_+\) from \(x_s(t)\) is used to extract \(v_+\) from \(x_v(t)\).
Rights and permissions
About this article
Cite this article
Hoefer, M.A. Shock Waves in Dispersive Eulerian Fluids. J Nonlinear Sci 24, 525–577 (2014). https://doi.org/10.1007/s00332-014-9199-4
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00332-014-9199-4