Abstract
In this paper we develop a general, systematic, microlocal framework for the Fredholm analysis of non-elliptic problems, including high energy (or semiclassical) estimates, which is stable under perturbations. This framework, described in Sect. 2, resides on a compact manifold without boundary, hence in the standard setting of microlocal analysis.
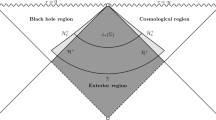
Many natural applications arise in the setting of non-Riemannian b-metrics in the context of Melrose’s b-structures. These include asymptotically de Sitter-type metrics on a blow-up of the natural compactification, Kerr-de Sitter-type metrics, as well as asymptotically Minkowski metrics.
The simplest application is a new approach to analysis on Riemannian or Lorentzian (or indeed, possibly of other signature) conformally compact spaces (such as asymptotically hyperbolic or de Sitter spaces), including a new construction of the meromorphic extension of the resolvent of the Laplacian in the Riemannian case, as well as high energy estimates for the spectral parameter in strips of the complex plane. These results are also available in a follow-up paper which is more expository in nature (Vasy in Uhlmann, G. (ed.) Inverse Problems and Applications. Inside Out II, 2012).
The appendix written by Dyatlov relates his analysis of resonances on exact Kerr-de Sitter space (which then was used to analyze the wave equation in that setting) to the more general method described here.








Similar content being viewed by others
Notes
General asymptotically hyperbolic spaces do not arise from a similar blow-up of a de Sitter-type space, rather could be thought of as a generalization of the blown-up n-dimensional de Sitter space, i.e. the generalization is after the blow-up. A different perspective, via an asymptotically Minkowski space, is briefly discussed in Sect. 5, and in more detail in [55].
This adjoint analysis also shows up for Minkowski space-time as the ‘original’ problem.
Though not the parametrix construction for the Poisson operator, or for the forward fundamental solution of Baskin [1]; for these we would need a parametrix construction in the present compact boundaryless, but analytically non-trivial (for this purpose), setting.
It does affect the location of the poles and corresponding resonant states of (P σ −ıQ σ )−1, hence the constant in Theorem 1.4 has to be replaced by the appropriate resonant state and exponential growth/decay, as in the second part of that theorem.
This means that we require the stronger of \(\operatorname {Im}\sigma>\beta_{\pm}^{-1}(1/2-s)\) to hold in (1.2). If we perturb Kerr-de Sitter space time, we need to increase the requirement on \(\operatorname {Im}\sigma\) slightly, i.e. the size of the half space has to be slightly reduced.
Since we are not making a statement for almost real σ, semiclassical trapping, discussed in the previous paragraph, does not matter.
This has been completed after the original version of this manuscript, see [21].
Certain kinds of perturbations conormal to the boundary, in particular polyhomogeneous ones, would only change the analysis and the conclusions slightly.
Another possibility would be to require uniform estimates on compact subsets; this makes no difference here.
In fact, even in classical microlocal analysis it is better to keep at least a ‘shadow’ of the interior of S ∗ X by working with T ∗ X∖o considered as a half-line bundle over S ∗ X with homogeneous objects on it; this keeps the action of the Hamilton vector field on the fiber-radial variable, i.e. the defining function of S ∗ X in \(\overline{T}^{*}X\), non-trivial, which is important at radial points, which in turn play a central role below.
This depends on choices unless k=0; they are naturally sections of a line bundle that encodes the differential of the boundary defining function at S ∗ X. However, the only relevant notion here is ellipticity, and later the Hamilton vector field up to multiplication by a positive function, which is independent of choices. In fact, we emphasize that all the requirements in Sect. 2.2 listed for p, q and later p ħ,z and q ħ,z , except possibly (2.5)–(2.6), are also fulfilled if P σ −ıQ σ is replaced by any smooth positive multiple, so one may factor out positive factors at will. This is useful in the Kerr-de Sitter space discussion. For (2.5)–(2.6), see footnote 19.
We adopt the convention that ħ denotes semiclassical objects, while h is the actual semiclassical parameter.
If X is a manifold with corners and Z is a product-type, or p-, submanifold, i.e. there are local coordinates x 1,…,x l , y 1,…,y n−l near p∈Z in which X is given by x 1≥0,…,x l ≥0, and Z is given by the vanishing of some of the x j and some of the y i , then one can blow up Z in X to obtain a new manifold with corners, [X;Z]. This is a space that is identical to X away from Z and in which Z is replaced by the front face ff of the blow up, namely the inward pointing spherical normal bundle, S + NZ, of Z in X. The space comes with a \(\mathcal {C}^{\infty}\) blow-down map β:[X;Z]→X which is thus a diffeomorphism away from ff. Roughly, the blow-up amounts to introducing cylindrical coordinates around Z: directions tangent to Z are unaffected, but those normal to Z are, and one is distinguishing directions of approach to Z modulo TZ; recall that N p Z=T p X/T p Z. One can easily write down projective local coordinates on this space. We refer to the Appendix of [39] for a concise but more detailed description, and to [43, Sect. 4] for a more leasurely discussion in a special case.
Unfortunately the sign convention here is the opposite of that adopted in the more expository paper [54].
Thus, they are connected components in the extended sense that they may be empty.
An extreme example would be Λ ±=Σ ±. Another extreme is if one or both are empty.
The precise behavior of \(\mp\tilde{\rho}^{k-1}\mathsf {H}_{p}\rho_{0}\), or of linear defining functions, is irrelevant, because we only need a relatively weak estimate. It would be relevant if one wanted to prove Lagrangian regularity.
If H p is radial at L ±, this is independent of the choice of the density ν. Indeed, with respect to fν, the adjoint of P σ is \(f^{-1}P_{\sigma}^{*} f\), with \(P_{\sigma}^{*}\) denoting the adjoint with respect to ν. This is \(P_{\sigma}^{*}+f^{-1}[P_{\sigma}^{*},f]\), and the principal symbol of \(f^{-1}[P_{\sigma}^{*},f]\in\varPsi_{\operatorname {cl}}^{k-1}(X)\) vanishes at L ± as H p f=0. In general, we can only change the density by factors f with \(\mathsf {H}_{p} f|_{L_{\pm}}=0\), which in Kerr-de Sitter space-times would mean factors independent of ϕ at the event horizon. A similar argument shows the independence of the condition from the choice of f when one replaces P σ by fP σ , under the same conditions: either radiality, or just \(\mathsf {H}_{p} f|_{L_{\pm}}=0\).
Our convention in estimates such as (2.9) and (2.10) is that if one assumes that all the quantities on the right hand side are in the function spaces indicated by the norms then so is the quantity on the left hand side, and the estimate holds. As we see below, at Λ ± not all relevant function space statements appear in the estimate, so we need to be more explicit there.
These estimates follow immediately from the microlocal elliptic parametrix construction. Alternatively, they follow from microlocal elliptic regularity plus the closed graph theorem, as used below in the real principal type setting.
See e.g. [32, Theorem 26.1.4] for the standard statement, and see the proof of [32, Theorem 26.1.6] for turning this into an estimate. Concretely, with the notation below, we may assume −N<s, and one has by the standard form of the theorem that if u∈H −N, Au∈H s, GP σ u∈H s−k+1, then Bu∈H s. Let \(\mathcal {Z}\) be the Hilbert space of distributions u∈H −N with Au∈H s, GP σ u∈H s−k+1 with norm \(\|u\|_{\mathcal {Z}}^{2}=\|u\|_{H^{-N}}^{2}+\|Au\|^{2}_{H^{s}}+\|GP_{\sigma}u\|^{2}\). Then \(B:\mathcal {Z}\to H^{-N}\) is continuous, since it is already continuous H −N→H −N, and it takes values in H s by the standard version of the propagation of singularities. Thus, if u j →u in \(\mathcal {Z}\) and Bu j →v in H s, then Bu j →Bu in H −N, so v=Bu and thus \(v\in \operatorname {Ran}B\), so the graph of \(B:\mathcal {Z}\to H^{s}\) is closed, so it is continuous, giving (2.10) below. However, this is a rather round about argument, since propagation of singularities is typically proved by positive commutator estimates (cf. the proofs of Propositions 2.3–2.4 below); these are microlocal so would need to pieced together to prove (2.10); using the standard propagation of singularities avoids the explicit ‘piecing together’ at the cost of invoking, somewhat superfluously, the closed graph theorem.
Note that this is consistent with (2.7).
Note the switch compared to Proposition 2.3! Also, β does not matter when \(\operatorname {Im}\sigma=0\); we define it here so that the two propositions are consistent via dualization, which reverses the sign of the imaginary part.
In particular that C ϵ is not a continuous family with values in classical operators in Ψ s−(k−1)/2(X), so principal symbols for the family should be considered as representatives of equivalence classes modulo lower order symbols, S s−(k−1)/2−1. The same applies to the commutators computed below.
Strictly speaking, with the definitions we have adopted, one should multiply c by 1−ψ, where ψ has compact support, identically 1 near the zero section. As this does not affect the behavior of c near fiber-infinity we do not do this explicitly here.
Reality is needed to ensure that (2.15) holds.
If one assumes that q is microlocally the square of a symbol, one need not use the sharp Gårding inequality. Since q is a tool we use in the problems we study, not given to us by the problem, one may make this choice if one wishes to do so.
Since the original version of this paper, the work of Haber and Vasy [29] showed that elements of this kernel are in fact Lagrangian distributions, i.e. they possess iterative regularity under the module of first order pseudodifferential operators with principal symbol vanishing on the Lagrangian, under additional assumptions on the structure at Λ ±, namely that Λ ± consists of radial points for H p . The latter holds, for instance, in de Sitter and de Sitter-Schwarzschild spaces, as well as on Minkowski space, but not on Kerr-de Sitter space.
Recall that Ω is the domain of σ for which Q σ is defined.
If we do not restrict \(|\!\operatorname {Im}z|<C'h\), we would only assume this when z is real. We discuss this in Sect. 7.
As an example, the standard semiclassical propagation of singularities result is that \(\mathrm {WF}_{h}^{s,r}(u)\), u={u h } h∈(0,1) a polynomially bounded family, is a union of maximally extended bicharacteristics of p ħ,z in \(\varSigma_{\hbar ,z}\setminus \mathrm {WF}_{h}^{s-1,r+1}(P_{\hbar ,z}u)\); this is the Sobolev version of [63, Theorem 12.5]. Note that the choice of r=−1 is most usual, but h commutes with P ħ,z , so the orders may be adjusted easily. This can be translated into a uniform estimate as h→0 using the uniform boundedness principle; see [54, Sect. 4.4], though as in the case of the classical estimates and the application of the closed graph theorem there, this is somewhat round about: see footnote 22. Concretely, in this case, one has the estimate
$$ \|Bu\|_{H^s_h}\leq C\bigl(h^{-1}\|GP_{h,z} u \|_{H^{s-k+1}_h}+\|Au\|_{H^s_h}+h\|u\|_{H^{-N}_h}\bigr), $$in the notation corresponding to (2.10), with bicharacteristics understood as integral curves of \(\mathsf {H}_{p_{h,z}}\) both at h=0 and as at fiber-infinity, S ∗ X.
Here and below the error term \(h\|u\|_{H^{-N}_{h}}\) can be changed to \(h^{N}\|u\|_{H^{-N}_{h}}\) by iterating the estimates, as required anyway for the proof of the \(\|u\|_{H^{-N}_{h}}\)-type error.
For ϵ>0, such a function F provides an escape function, \(\tilde{F}=e^{-C F}\mathsf {H}_{p_{\hbar ,z}}F\) on the set where 1+ϵ≤F≤2−ϵ. Namely, by taking C>0 sufficiently large, \(\mathsf {H}_{p_{\hbar ,z}}\tilde{F}<0\) there; thus, every bicharacteristic must leave the compact set F −1([1+ϵ,2−ϵ]) in finite time. However, the existence of such an F is a stronger statement than that of an escape function: a bicharacteristic segment cannot leave F −1([1+ϵ,2−ϵ]) via the boundary F=2−ϵ in both directions since F cannot have a local minimum. This is exactly the way this condition is used in [15].
Condition (i) follows by letting \(\tilde{F}=\varphi_{+}^{2\kappa} +\varphi_{-}^{2\kappa}\) with the notation of [61, Lemma 4.1]; so
$$\mathsf {H}_p^2\tilde{F}=4\kappa^2 \bigl(c_+^4-\kappa^{-1}c_+\mathsf {H}_p c_+\bigr) \varphi_+^{2\kappa} +4\kappa^2 \bigl(c_-^4+ \kappa^{-1}c_-\mathsf {H}_p c_-\bigr)\varphi_-^{2\kappa} $$near the trapped set, φ +=0=φ −. Thus, for sufficiently large κ, \(\mathsf {H}_{p}\tilde{F}>0\) outside \(\tilde{F}=0\). Since \(\tilde{F}=0\) defines the trapped set, in order to satisfy Definition 2.16, writing K and O instead of K ± and O ±, one lets \(K=\{\tilde{F}\leq\alpha\}\), \(O=\{\tilde{F}<\beta\}\) for suitable (small) α and β, α<β, and takes \(F=G\circ\tilde{F}\) with G strictly decreasing, G|[0,α]>2, G|[β,∞)<1.
In fact, \(\mathcal {P}\in \varPsi _{{\mathrm{b}}}^{k}(\bar{M})\) works similarly.
Standard up to equivalence, such as \((\sum_{|\alpha|\leq s}\int_{\operatorname {Im}\sigma=-\alpha}\langle|\sigma |\rangle^{2(s-|\alpha|)} \|D_{y}^{\alpha}v\|^{2}_{L^{2}(X;\nu)}\,d\sigma )^{1/2}\).
See the discussion after (2.21). Also note that while for \(\mathcal {P}-\imath \mathcal {Q}\) the coefficients a jκ are smooth, the operator mapping f to a jκ is not smoothing exactly because of the non-smoothness of the expansion for the adjoint operator: it maps sufficiently regular f to smooth functions, but cannot be applied to all distributional f.
See Remark 3.3.
For now z real being the only case, but in Sect. 7 z complex is allowed in the same expression.
This actually does not matter for the discussion below, but due to Sect. 3.3 it ensures that the choice of the extension is irrelevant.
We could also work with T ∗ℝ and standard dual variables via a logarithmic change of variables, changing dilations to translations, but in view of the previous section, the b-setup is particularly convenient.
Operating with −logτ in place of τ one would have translation invariance, no changes required into the b-notation, and one would use the Fourier transform.
In particular, this shows that the support of the Schwartz kernel of the inverse, with the first (left) factor giving the ‘outgoing’ and the second (right) factor the ‘incoming’ (i.e. the one in which the integral is taken) variables, satisfies
$$ \begin{aligned} \operatorname {supp}K_{(P_\sigma-\imath Q_\sigma)^{-1}}&\subset \bigl(X\times \mathfrak {t}^{-1}\bigl((-\infty,\mathfrak {t}_0]\big) \bigr)\cup \bigl( \mathfrak {t}^{-1}\bigl([\mathfrak {t}_1,+\infty)\bigr)\times X \bigr) \\ &\quad {}\cup (\mathfrak {t}\times \mathfrak {t})^{-1}\bigl\{\bigl( \mathfrak {t}',\mathfrak {t}''\bigr)\in( \mathfrak {t}_0,\mathfrak {t}_1)^2:\ \mathfrak {t}'\geq \mathfrak {t}''\bigr\}; \end{aligned} $$for \(K_{(P_{\sigma}^{*}+\imath Q_{\sigma}^{*})^{-1}}\) the two factors are reversed. This also gives that the Laurent coefficients have similar support properties at any pole. In summary, there is a ‘block lower triangular’ structure (with the first variable being on the vertical axis, increasing downwards, as in matrix notation) to \(\operatorname {supp}K_{(P_{\sigma}-\imath Q_{\sigma})^{-1}}\), with the middle piece, \((\mathfrak {t}_{0},\mathfrak {t}_{1})^{2}\), itself being lower triangular.
Again, as discussed in Remark 7.4, the large \(\operatorname {Im}\sigma\) assumptions only affect the existence part below, and do so relatively mildly.
As remarked above, these are independent of the choice of j for σ∈ℂ as long as σ∈Ω j .
Recall that this operator, when considered as a product, refers to \(\psi(P_{\sigma}-\imath Q^{(j)}_{\sigma})^{-1}\), with j appropriately chosen.
Here the asymptotic behavior as x→0 is the interesting statement.
See Sect. 3 for a quick introduction to b-geometry and further references.
If we had worked with e −2t instead of e −t above, we would obtain x 2 as the defining function of the temporal face, rather than x.
This needs the analogous statement for full subprincipal symbol, not only its imaginary part.
There is in fact a not too complicated global escape function, e.g.
$$f=\frac{2Y\cdot\zeta-z}{2\sqrt{1+|Y|^2}(Y\cdot\zeta-z)} =\frac{2Y\cdot\hat{\zeta}-z|\zeta|^{-1}}{ 2\sqrt{1+|Y|^2}(Y\cdot\hat{\zeta}-z|\zeta|^{-1})}, $$which is a smooth function on the characteristic set in T ∗ℝn−1 as Y⋅ζ≠z there; further, it extends smoothly to the characteristic set in \(\overline{T}^{*}\mathbb {R}^{n-1}\) away from L ± since \(\sqrt {1+|Y|^{2}}(Y\cdot\hat{\zeta}-z|\zeta|^{-1})\) vanishes only there near S ∗ℝn−1 (where these are valid coordinates), at which it has conic points. This function arises in a straightforward manner when one reduces Minkowski space, \(\mathbb {R}^{n}=\mathbb {R}^{n-1}_{z'}\times \mathbb {R}_{t}\) with metric g 0, to the boundary of its radial compactification, as described in Sect. 5, and uses the natural escape function
$$ \tilde{f}=\frac{t t^*-z' (z')^*}{t^*\sqrt{t^2+|z'|^2}} $$(4.22)there; here t ∗ is the dual variable of t and (z′)∗ of z′, outside the origin.
In fact, in the de Sitter context, this essentially means moving to the boundary of n-dimensional Minkowski space, where our (n−1)-dimensional model is the ‘upper hemisphere’, see Sect. 5. Thus, doubling over means working with the whole boundary, but putting an absorbing operator near the equator, corresponding to the usual Cauchy hypersurface in Minkowski space, and solving from the radial points at both the future and past light cones towards the equator—this would be impossible without the complex absorption.
Note that our methods work equally well for asymptotically de Sitter spaces in the sense of [53]; after the blow up, the boundary metric is ‘frozen’ at the point that is blown up, hence the induced problem at the front face is the same as for the de Sitter metric with asymptotics given by this ‘frozen’ metric.
The standard Poincaré ball model is the metric \(4\frac{dw^{2}}{(1-|w|^{2})^{2}}\) on \(\mathbb {B}^{n-1}\). Introducing polar coordinates, \(w=\tilde{r}\omega\), the present form arises by letting \(\nu=\frac{1-\tilde{r}^{2}}{1+\tilde{r}^{2}}\), i.e. \(\tilde{r}^{2}=\frac{1-\nu}{1+\nu}\) with \(\nu=\sqrt{1-r^{2}}\); recall that μ=1−r 2. Thus, ν and \(1-\tilde{r}^{2}\) are equivalent boundary defining functions.
In fact, a stronger statement can be made: a calculation completely analogous to what we just performed, see Remark 4.6, shows that in μ<0, P σ is a conjugate (times a power of μ) of a Klein-Gordon-type operator on (n−1)-dimensional de Sitter space with μ=0 being the boundary (i.e. where time goes to infinity). Thus, if σ is not a pole of \(\mathcal {R}(\sigma)\) and \((P_{\sigma}-\imath Q_{\sigma})\tilde{u}=0\) then one would have a solution u of this Klein-Gordon-type equation near μ=0, i.e. infinity, that rapidly vanishes at infinity. It is shown in [53, Proposition 5.3] by a Carleman-type estimate that this cannot happen; although there σ 2∈ℝ is assumed, the argument given there goes through almost verbatim in general. Thus, if Q σ is supported in μ<c, c<0, i.e. the Schwartz kernel is supported in (−∞,c)×(−∞,c) in terms of μ, then \(\tilde{u}\) is also supported in μ<c. This argument can be applied to the highest order Laurent term which has support intersecting (c,∞)×(c,∞) non-trivially (which need not be the overall highest order term), so if σ is a pole of (P σ −ıQ σ )−1 with a Laurent coefficient with support intersecting (c,∞)×(c,∞) non-trivially, but σ is not a pole of \(\mathcal {R}(\sigma)\), then the corresponding resonant state is supported in μ<c, unless the dual state is supported in μ≤0. Applying the argument to the highest order terms with support intersecting (c,∞)×(0,∞) non-trivially (with the first factor corresponding to the resonant state, the second to the dual state), we see that all poles of (P σ −ıQ σ )−1 with Laurent coefficients with support intersecting (c,∞)×(0,∞) non-trivially are given by poles of \(\mathcal {R}(\sigma)\).
Though of course the resonances vary with the perturbation, in the same manner as they would vary when perturbing any other Fredholm problem.
For ‘classical’ results, the interior is automatically irrelevant.
Note that μ=x 2.
There can be no support in μ −1((−∞,c]) in view of footnote 44.
In view of the block lower triangular structure of the Schwartz kernel of (P σ −ıQ σ )−1, as explained in footnote 44, at least for σ near a fixed σ 0, one can change Q σ by a holomorphic finite rank operator family, keeping its support in μ<c in both factors, so that for the new Q σ the poles of (P σ −ıQ σ )−1 near σ 0 are exactly those of ψ(P σ −ıQ σ )−1 ϕ, with multiplicities. This in particular implies that in the perturbation framework of Sect. 2.7, for perturbations P σ (w)−ıQ σ (w), w close to w 0, the poles of ψ(P σ (w)−ıQ σ (w))−1 ϕ are necessarily close to the poles of ψ(P σ (w 0)−ıQ σ (w 0))−1 ϕ, with multiplicities. In the Kerr-de Sitter setting for small angular momentum, a, as in Theorem 1.4, this justifies the simplicity and one dimensionality of the zero resonance: while for a=0, (P σ (a)−ıQ σ (a))−1 may have other resonant states at σ=0, only 1 contributes to ψ(P σ (a)−ıQ σ (a))−1 ϕ with a=0, with a resulting simple resonance, hence for small a the same holds.
Only when \(\operatorname {Im}\sigma\to\infty\) can such a change matter.
Since replacing t>0 by t<0 in the region we consider reverses the sign when relating D ρ and D t , the signs would agree with those from the discussion after [53, Eq. (7.4)] at the backward light cone.
As pointed out to the author by Gunther Uhlmann, this means that the Klein model of hyperbolic space is the one induced by the Minkowski boundary reduction.
Recall footnote 57 for the connection to the standard Poincaré model.
The latter is only done to avoid combining for the same operator the estimates we state below for an operator L σ and its adjoint; as follows from the remark above regarding the sign of σ, for the operator here, the microlocal picture near the backward light cone is like that for the L σ considered in Sect. 2, and near the forward light cone like that for \(L_{\sigma}^{*}\). It is thus fine to include both the backward and the forward light cones; we just end up with a combination of the problem we study here and its adjoint, and with function spaces much like in [39, 57].
This could be relaxed: quadratic behavior with small leading term would be fine as well; quadratic behavior follows from H p being tangent to Λ ±; smallness is needed so that H p |ξ|−1 can be used to dominate this in terms of homogeneous dynamics, so that the dynamical character of L ± (sink/source) is as desired.
Or far from, in \({\tilde {\mu }}>0\).
By compactness considerations, \(e^{C\tilde{F}}\mathsf {H}_{p_{\hbar ,z}}\tilde{F}\) is an escape function outside Γ z for C>0 large, cf. footnote 34, i.e. its \(\mathsf {H}_{p_{\hbar ,z}}\) is bounded below by a positive constant on compact subsets of T ∗ X 0∖Γ z . Correspondingly, in either the future or the past direction, bicharacteristics must either reach r=r ± in finite time, or have a sequence of points tending to L ± or Γ z . In the latter two cases the whole bicharacteristic is easily seen to be tending to these in view of the local dynamics at L ± (source/sink), and in case of Γ z since \(\tilde{F}\circ\gamma\) cannot have local maxima. Indeed, if there is a sequence, say, t n →+∞ with γ(t n )→Γ z then \(\tilde{F}(\gamma(t))\to0\) as t→+∞; if γ does not tend to Γ z , then there is a sequence s n →+∞ with γ(s n ) bounded away from Γ z , one can take a subsequence along which γ(s n ) converges to a limit α, which is thus a point at which \(\tilde{F}=0\); then \(\gamma|_{[s_{n},s_{n}+1]}\) converges to the bicharacteristic through this point (along the subsequence), which is impossible since \(\tilde{F}\) would have to be zero along this segment, but it can only have strict local minima away from Γ z .
A different way of phrasing the argument is to regard a compact interval I in \((r_{-},\frac{3}{2} r_{s})\cup(\frac{3}{2} r_{s},r_{+})\) as the gluing region, for sufficiently small a, for r∈I, \(\mathsf {H}_{p_{\hbar ,z}}r=0\) still implies \(\pm \mathsf {H}_{p_{\hbar ,z}}^{2}r>0\), and [15] is applicable. This concretely means that one uses Theorem 1 of [61] with an absorbing potential in the de Sitter-Schwarzschild setting with K=Γ z in the notation there, where it applies equally well given our observations regarding the dynamics, including normal hyperbolicity. If instead one works with compact subsets of \(\{{\tilde {\mu }}>0\}\setminus\varGamma_{z}\), one has non-trapping dynamics for a small, and the results still apply.
Note that one of the roots tends to −∞ if az>0 and \({\tilde {\mu }}\searrow a^{2}\kappa\sin^{2}\theta\), and to +∞ if az<0 and \({\tilde {\mu }}\searrow a^{2}\kappa \sin^{2}\theta\).
The other component, \(\varSigma_{\hbar ,-\operatorname {sgn}z}\) does intersect \({\tilde {\mu }}= a^{2}\kappa\sin^{2}\theta\), but only does so at fiber infinity which was already analyzed for the classical dynamics. This corresponds to the root of the quadratic polynomial in ζ that escaped to ∓∞ and reemerges from ±∞ at \({\tilde {\mu }}= a^{2}\kappa\sin^{2}\theta\), depending on the sign of az.
We already remarked this outside the ergoregion, but here we need to consider the ergoregion.
By (6.13) there are at least two zeros; in view of \({\tilde {\mu }}\) having a single critical point between (r −,r +), there are exactly two zeros.
Since in the ergoregions \(r^{-4}({\tilde {\mu }}-a^{2})<0\).
There is a slight complication if the projection of Γ z enters the ergoregion as the operator ceases to be elliptic, though the latter is assumed by Wunsch and Zworski; in this case one needs a pseudodifferential absorber, which however barely affects their arguments.
We need to assume this since p ħ,z ,q ħ,z are not real, so the ellipticity of p ħ,z does not imply this. Arranging this in the Lorentzian setting is the reason for an extended argument starting with the paragraph of (3.17).
Recall that we are not assuming semiclassical non-trapping here, which is the reason we cannot simply quote the relevant part of Theorem 2.14 for the meromorphy.
This is possible for \(\frac{d\tau}{\tau}\) time-like. Note further that typically γ is not in the ‘spatial’ slice \(T^{*}_{x} X\); the latter need even not be space-like.
References
Baskin, D.: A parametrix for the fundamental solution of the Klein-Gordon equation on asymptotically de Sitter spaces. J. Funct. Anal. 259(7), 1673–1719 (2010)
Bieri, L.: Part I: Solutions of the Einstein vacuum equations. In: Extensions of the Stability Theorem of the Minkowski Space in General Relativity. AMS/IP Studies in Advanced Mathematics, vol. 45, pp. 1–295. Am. Math. Soc., Providence (2009)
Bieri, L., Zipser, N.: Extensions of the Stability Theorem of the Minkowski Space in General Relativity. AMS/IP Studies in Advanced Mathematics, vol. 45. Am. Math. Soc., Providence (2009)
Blue, P., Soffer, A.: Phase space analysis on some black hole manifolds. J. Funct. Anal. 256(1), 1–90 (2009)
Bony, J.-F., Häfner, D.: Decay and non-decay of the local energy for the wave equation on the de Sitter-Schwarzschild metric. Commun. Math. Phys. 282(3), 697–719 (2008)
Borthwick, D., Perry, P.: Scattering poles for asymptotically hyperbolic manifolds. Trans. Am. Math. Soc. 354(3), 1215–1231 (2002) (electronic)
Cardoso, F., Vodev, G.: Uniform estimates of the resolvent of the Laplace-Beltrami operator on infinite volume Riemannian manifolds. II. Ann. Henri Poincaré 3(4), 673–691 (2002)
Carter, B.: Global structure of the Kerr family of gravitational fields. Phys. Rev. 174, 1559–1571 (1968)
Christodoulou, D., Klainerman, S.: The Global Nonlinear Stability of the Minkowski Space. Princeton Mathematical Series, vol. 41. Princeton University Press, Princeton (1993)
Dafermos, M., Rodnianski, I.: A proof of Price’s law for the collapse of a self-gravitating scalar field. Invent. Math. 162(2), 381–457 (2005)
Dafermos, M., Rodnianski, I.: The wave equation on Schwarzschild-de Sitter space times. arXiv:0709.2766 (2007)
Dafermos, M., Rodnianski, I.: The red-shift effect and radiation decay on black hole spacetimes. Commun. Pure Appl. Math. 62, 859–919 (2009)
Dafermos, M., Rodnianski, I.: The black hole stability problem for linear scalar perturbations. arXiv:1010.5137 (2010)
Dafermos, M., Rodnianski, I.: Decay of solutions of the wave equation on Kerr exterior space-times I–II: The cases of |a|≪m or axisymmetry. arXiv:1010.5132 (2010)
Datchev, K., Vasy, A.: Gluing semiclassical resolvent estimates via propagation of singularities. Int. Math. Res. Not. 2012, 5409–5443 (2012)
Datchev, K., Vasy, A.: Propagation through trapped sets and semiclassical resolvent estimates. Ann. Inst. Fourier, to appear. arXiv:1010.2190
Dimassi, M., Sjöstrand, J.: Spectral Asymptotics in the Semi-classical Limit. London Mathematical Society Lecture Note Series, vol. 268. Cambridge University Press, Cambridge (1999)
Donninger, R., Schlag, W., Soffer, A.: A proof of Price’s law on Schwarzschild black hole manifolds for all angular momenta. Adv. Math. 226(1), 484–540 (2011)
Dyatlov, S.: Exponential energy decay for Kerr–de Sitter black holes beyond event horizons. Math. Res. Lett. 18(5), 1023–1035 (2011)
Dyatlov, S.: Quasi-normal modes and exponential energy decay for the Kerr-de Sitter black hole. Commun. Math. Phys. 306(1), 119–163 (2011)
Dyatlov, S.: Asymptotic distribution of quasi-normal modes for Kerr-de Sitter black holes. Ann. Henri Poincaré 13, 1101–1166 (2012)
Fefferman, C., Graham, C.R.: Conformal invariants. In: The Mathematical Heritage of Élie Cartan, Lyon, 1984. Astérisque Numero Hors Serie, pp. 95–116 (1985)
Finster, F., Kamran, N., Smoller, J., Yau, S.-T.: Decay of solutions of the wave equation in the Kerr geometry. Commun. Math. Phys. 264(2), 465–503 (2006)
Finster, F., Kamran, N., Smoller, J., Yau, S.-T.: Linear waves in the Kerr geometry: a mathematical voyage to black hole physics. Bull., New Ser., Am. Math. Soc. 46(4), 635–659 (2009)
Friedlander, F.G.: Radiation fields and hyperbolic scattering theory. Math. Proc. Camb. Philos. Soc. 88(3), 483–515 (1980)
Graham, C.R., Zworski, M.: Scattering matrix in conformal geometry. Invent. Math. 152(1), 89–118 (2003)
Guillarmou, C., Hassell, A., Sikora, A.: Resolvent at low energy III: The spectral measure. arXiv:1009.3084 (2010)
Guillarmou, C.: Meromorphic properties of the resolvent on asymptotically hyperbolic manifolds. Duke Math. J. 129(1), 1–37 (2005)
Haber, N., Vasy, A.: Propagation of singularities around a Lagrangian submanifold of radial points. arXiv:1110.1419 (2011)
Hassell, A., Melrose, R.B., Vasy, A.: Spectral and scattering theory for symbolic potentials of order zero. Adv. Math. 181, 1–87 (2004)
Hassell, A., Melrose, R.B., Vasy, A.: Microlocal propagation near radial points and scattering for symbolic potentials of order zero. Anal. Partial Differ. Equ. 1, 127–196 (2008)
Hörmander, L.: The Analysis of Linear Partial Differential Operators, vols. 1–4. Springer, Berlin (1983)
Kay, B.S., Wald, R.M.: Linear stability of Schwarzschild under perturbations which are nonvanishing on the bifurcation 2-sphere. Class. Quantum Gravity 4(4), 893–898 (1987)
Lindblad, H., Rodnianski, I.: Global existence for the Einstein vacuum equations in wave coordinates. Commun. Math. Phys. 256(1), 43–110 (2005)
Lindblad, H., Rodnianski, I.: The global stability of Minkowski space-time in harmonic gauge. Ann. Math. 171(3), 1401–1477 (2010)
Marzuola, J., Metcalfe, J., Tataru, D., Tohaneanu, M.: Strichartz estimates on Schwarzschild black hole backgrounds. Commun. Math. Phys. 293(1), 37–83 (2010)
Mazzeo, R., Melrose, R.B.: Meromorphic extension of the resolvent on complete spaces with asymptotically constant negative curvature. J. Funct. Anal. 75, 260–310 (1987)
Mazzeo, R.: Elliptic theory of differential edge operators. I. Commun. Partial Differ. Equ. 16(10), 1615–1664 (1991)
Melrose, R.B.: Spectral and Scattering Theory for the Laplacian on Asymptotically Euclidean Spaces. Dekker, New York (1994)
Melrose, R.B., Sá Barreto, A., Vasy, A.: Asymptotics of solutions of the wave equation on de Sitter-Schwarzschild space. arXiv:0811.2229 (2008)
Melrose, R.B., Sá Barreto, A., Vasy, A.: Analytic continuation and semiclassical resolvent estimates on asymptotically hyperbolic spaces. arXiv:1103.3507 (2011)
Melrose, R.B., Vasy, A., Wunsch, J.: Diffraction of singularities for the wave equation on manifolds with corners. Astérisque, to appear. arXiv:0903.3208 (2009)
Melrose, R.B.: The Atiyah-Patodi-Singer Index Theorem. Research Notes in Mathematics, vol. 4. AK Peters, Wellesley (1993)
Nonnenmacher, S., Zworski, M.: Quantum decay rates in chaotic scattering. Acta Math. 203(2), 149–233 (2009)
Polarski, D.: On the Hawking effect in de Sitter space. Class. Quantum Gravity 6(5), 717–722 (1989)
Sá Barreto, A., Wunsch, J.: The radiation field is a Fourier integral operator. Ann. Inst. Fourier (Grenoble) 55(1), 213–227 (2005)
Sá Barreto, A., Zworski, M.: Distribution of resonances for spherical black holes. Math. Res. Lett. 4(1), 103–121 (1997)
Shubin, M.A.: Pseudodifferential Operators and Spectral Theory. Springer, Berlin (1987)
Tataru, D.: Local decay of waves on asymptotically flat stationary spacetimes. arXiv:0910.5290 (2009)
Tataru, D., Tohaneanu, M.: A local energy estimate on Kerr black hole backgrounds. Int. Math. Res. Not. 2011(2), 248–292 (2011)
Taylor, M.E.: Partial Differential Equations. Basic Theory. Texts in Applied Mathematics, vol. 23. Springer, New York (1996)
Vasy, A.: Propagation of singularities in three-body scattering. Astérisque 262 (2000)
Vasy, A.: The wave equation on asymptotically de Sitter-like spaces. Adv. Math. 223, 49–97 (2010)
Vasy, A.: Microlocal analysis of asymptotically hyperbolic spaces and high energy resolvent estimates. In: Uhlmann, G. (ed.) Inverse Problems and Applications. Inside Out II. MSRI Publications, vol. 60. Cambridge University Press, Cambridge (2012)
Vasy, A.: Analytic continuation and high energy estimates for the resolvent of the Laplacian on forms on asymptotically hyperbolic spaces. arXiv:1206.5454 (2012)
Vasy, A.: The wave equation on asymptotically Anti-de Sitter spaces. Anal. Partial Differ. Equ. 5, 81–144 (2012)
Vasy, A., Zworski, M.: Semiclassical estimates in asymptotically Euclidean scattering. Commun. Math. Phys. 212, 205–217 (2000)
Vodev, G.: Local energy decay of solutions to the wave equation for nontrapping metrics. Ark. Mat. 42(2), 379–397 (2004)
Wald, R.M.: Note on the stability of the Schwarzschild metric. J. Math. Phys. 20(6), 1056–1058 (1979)
Wang, F.: Radiation field for vacuum Einstein equation. PhD thesis, Massachusetts Institute of Technology (2010)
Wunsch, J., Zworski, M.: Resolvent estimates for normally hyperbolic trapped sets. Ann. Henri Poincaré 12(7), 1349–1385 (2011)
Yagdjian, K., Galstian, A.: Fundamental solutions for the Klein-Gordon equation in de Sitter spacetime. Commun. Math. Phys. 285(1), 293–344 (2009)
Zworski, M.: Lectures on Semiclassical Analysis. Am. Math. Soc., Providence (2012)
Acknowledgements
A.V. is very grateful to Maciej Zworski, Richard Melrose, Semyon Dyatlov, Mihalis Dafermos, Gunther Uhlmann, Jared Wunsch, Rafe Mazzeo, Kiril Datchev, Colin Guillarmou, Andrew Hassell, Dean Baskin and Peter Hintz for very helpful discussions, for their enthusiasm for this project and for carefully reading parts of this manuscript. Special thanks are due to Semyon Dyatlov in this regard who noticed an incomplete argument in an earlier version of this paper in holomorphy considerations, and to Mihalis Dafermos, who urged the author to supply details to the argument at the end of Sect. 6, which resulted in the addition of Sect. 3.3, as well as the part of Sect. 3.2 and Sect. 7 covering the complex absorption, to the main body of the argument, and that of Sect. 2.7 for stability considerations. A.V. is also very grateful to the three anonymous referees whose detailed reports improved the manuscript significantly.
A.V. gratefully acknowledges partial support from the National Science Foundation under grants number DMS-0801226 and DMS-1068742 and from a Chambers Fellowship at Stanford University, as well as the hospitality of Mathematical Sciences Research Institute in Berkeley. S.D. is grateful for partial support from the National Science Foundation under grant number DMS-0654436.
Author information
Authors and Affiliations
Corresponding author
Appendix A: Comparison with cutoff resolvent constructions (by Semyon Dyatlov)
S.D.’s address is Department of Mathematics, University of California, Berkeley, CA 94720-3840, USA, and e-mail address is dyatlov@math.berkeley.edu.
Appendix A: Comparison with cutoff resolvent constructions (by Semyon Dyatlov)
In this appendix, we will first examine the relation of the resolvent considered in the present paper to the cutoff resolvent for slowly rotating Kerr–de Sitter metric constructed in [20] using separation of variables and complex contour deformation near the event horizons. Then, we will show how to extract information on the resolvent beyond event horizons from information about the cutoff resolvent.
First of all, let us list some notation of [20] along with its analogues in the present paper:
The difference between P g (ω) and P σ is due to the fact that P g (ω) was defined using Fourier transform in the \(\tilde{t}\) variable and P σ is defined using Fourier transform in the variable \(t=\tilde{t}+h(r)\). We will henceforth use the notation of the present paper.
We assume that δ>0 is small and fixed, and α is small depending on δ. Define
Then [20, Theorem 2] gives a family of operators
meromorphic in σ∈ℂ and such that P g (σ)R g (σ)f=f on K δ for each f∈L 2(K δ ).
Proposition A.1
Assume that the complex absorbing operator Q σ satisfies the assumptions of Sect. 6.5 in the ‘classical’ case and furthermore, its Schwartz kernel is supported in (X∖X +)2. Let R g (σ) be the operator constructed in [20] and R(σ)=(P σ −iQ σ )−1 be the operator defined in Theorem 1.2 of the present paper. Then for each \(f\in C_{0}^{\infty}(K_{\delta})\),
Proof
The proof follows [20, Proposition 1.2]. Denote by u 1 the left-hand side of (A.1) and by u 2 the right-hand side. Without loss of generality, we may assume that f lies in the kernel \(\mathcal{D}'_{k}\) of the operator D ϕ −k, for some k∈ℤ; in this case, by [20, Theorem 1], u 1 can be extended to the whole X + and solves the equation P σ u 1=f there. Moreover, by [20, Theorem 3], u 1 is smooth up to the event horizons {r=r ±}. Same is true for u 2; therefore, the difference u=u 1−u 2 solves the equation P σ (u)=0 and is smooth up to the event horizons.
Since both sides of (A.1) are meromorphic, we may further assume that \(\mathop {\operatorname {Im}}\sigma>C_{e}\), where C e is a large constant. Now, the function \(\tilde{u}(t,\cdot)=e^{-it\sigma}u(\cdot)\) solves the wave equation \({\square }_{g} \tilde{u}=0\) and is smooth up to the event horizons in the coordinate system (t,r,θ,ϕ); therefore, if C e is large enough, by [20, Proposition 1.1] \(\tilde{u}\) cannot grow faster than exp(C e t). Therefore, u=0 as required. □
Now, we show how to express the resolvent R(σ) on the whole space in terms of the cutoff resolvent R g (σ) and the nontrapping construction in the present paper. Let Q σ be as above, but with the additional assumption of semiclassical ellipticity near ∂X δ , and \(Q'_{\sigma}\in \varPsi _{\hbar }^{-\infty}\) be an operator satisfying the assumptions of Sect. 6.5 in the ‘semiclassical’ case on the trapped set. Moreover, we require that the semiclassical wavefront set of \(|\sigma|^{-2}Q'_{\sigma}\) be compact and \(Q'_{\sigma}=\chi Q'_{\sigma}=Q'_{\sigma}\chi\), where \(\chi\in C_{0}^{\infty}(K_{\delta})\). Such operators exist for α small enough, as the trapped set is compact and located O(α) close to the photon sphere {r=3r s /2} and thus is far from the event horizons. Denote \(R'(\sigma)=(P_{\sigma}-iQ_{\sigma}-iQ'_{\sigma})^{-1}\); by Theorem 2.14 applied in the case of Sect. 6.5, for each C 0 there exists a constant σ 0 such that for s large enough, \(\mathop {\operatorname {Im}}\sigma>-C_{0}\), and \(|\!\mathop {\operatorname {Re}}\sigma|>\sigma_{0}\),
We now use the identity
(To verify it, multiply both sides of the equation by \(P_{\sigma}-iQ_{\sigma}-iQ'_{\sigma}\) on the left and on the right.) Combining (A.2) with the fact that for each N, \(Q'_{\sigma}\) is bounded \(H^{-N}_{|\sigma|^{-1}}\to H^{N}_{|\sigma|^{-1}}\) with norm O(|σ|2), we get for σ not a pole of χR(σ)χ,
Also, if σ 0 is a pole of R(σ) of algebraic multiplicity j, then we can multiply the identity (A.2) by (σ−σ 0)j to get an estimate similar to (A.3) on the function (σ−σ 0)j R(σ), holomorphic at σ=σ 0.
The discussion above in particular implies that the cutoff resolvent estimates of [5] also hold for the resolvent R(σ). Using the Mellin transform, we see that the resonance expansion of [5] is valid for any solution u to the forward time Cauchy problem for the wave equation on the whole M δ , with initial data in a high enough Sobolev class; the terms of the expansion are defined and the remainder is estimated on the whole M δ as well.
Rights and permissions
About this article
Cite this article
Vasy, A. Microlocal analysis of asymptotically hyperbolic and Kerr-de Sitter spaces (with an appendix by Semyon Dyatlov). Invent. math. 194, 381–513 (2013). https://doi.org/10.1007/s00222-012-0446-8
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00222-012-0446-8