Crossover to the Stochastic Burgers Equation for the WASEP with a Slow Bond

Abstract

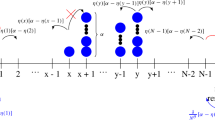
We consider the weakly asymmetric simple exclusion process in the presence of a slow bond and starting from the invariant state, namely the Bernoulli product measure of parameter ${\rho \in (0,1)}$. The rate of passage of particles to the right (resp. left) is ${\frac{1}{2} + \frac{a}{2n^{\gamma}}}$ (resp. ${\frac{1}{2} - \frac{a}{2n^{\gamma}}}$) except at the bond of vertices ${\{-1,0\}}$ where the rate to the right (resp. left) is given by ${\frac{\alpha}{2n^\beta} + \frac{a}{2n^{\gamma}}}$ (resp. ${\frac{\alpha}{2n^\beta}-\frac{a}{2n^{\gamma}}}$). Above, ${\alpha > 0}$, ${\gamma \geq \beta \geq 0}$, ${a\geq 0}$. For ${\beta < 1}$, we show that the limit density fluctuation field is an Ornstein–Uhlenbeck process defined on the Schwartz space if ${\gamma > \frac{1}{2}}$, while for ${\gamma = \frac{1}{2}}$ it is an energy solution of the stochastic Burgers equation. For ${\gamma \geq \beta =1}$, it is an Ornstein–Uhlenbeck process associated to the heat equation with Robin’s boundary conditions. For ${\gamma \geq \beta > 1}$, the limit density fluctuation field is an Ornstein–Uhlenbeck process associated to the heat equation with Neumann’s boundary conditions.