Skip to main content
SpringerLink
Log in

Minimal gain marching schemes: searching for unstable steady-states with unsteady solvers

  • Original Article
  • Published:
Theoretical and Computational Fluid Dynamics Aims and scope Submit manuscript

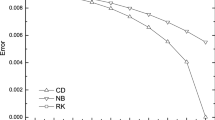
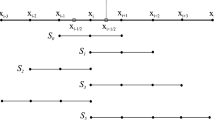
Abstract

Reference solutions are important in several applications. They are used as base states in linear stability analyses as well as initial conditions and reference states for sponge zones in numerical simulations, just to name a few examples. Their accuracy is also paramount in both fields, leading to more reliable analyses and efficient simulations, respectively. Hence, steady-states usually make the best reference solutions. Unfortunately, standard marching schemes utilized for accurate unsteady simulations almost never reach steady-states of unstable flows. Steady governing equations could be solved instead, by employing Newton-type methods often coupled with continuation techniques. However, such iterative approaches do require large computational resources and very good initial guesses to converge. These difficulties motivated the development of a technique known as selective frequency damping (SFD) (Åkervik et al. in Phys Fluids 18(6):068102, 2006). It adds a source term to the unsteady governing equations that filters out the unstable frequencies, allowing a steady-state to be reached. This approach does not require a good initial condition and works well for self-excited flows, where a single nonzero excitation frequency is selected by either absolute or global instability mechanisms. On the other hand, it seems unable to damp stationary disturbances. Furthermore, flows with a broad unstable frequency spectrum might require the use of multiple filters, which delays convergence significantly. Both scenarios appear in convectively, absolutely or globally unstable flows. An alternative approach is proposed in the present paper. It modifies the coefficients of a marching scheme in such a way that makes the absolute value of its linear gain smaller than one within the required unstable frequency spectra, allowing the respective disturbance amplitudes to decay given enough time. These ideas are applied here to implicit multi-step schemes. A few chosen test cases shows that they enable convergence toward solutions that are unstable to stationary and oscillatory disturbances, with either a single or multiple frequency content. Finally, comparisons with SFD are also performed, showing significant reduction in computer cost for complex flows by using the implicit multi-step MGM schemes.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Similar content being viewed by others

References

  1. Åkervik, E., Brandt, L., Henningson, D.S., Hoepffner, J., Marxen, O., Schlatter, P.: Steady solutions of the Navier-Stokes equations by selective frequency damping. Phys. Fluids 18(6), 068102 (2006)

    Article  Google Scholar 

  2. Åkervik, E., Hœpffner, J., Ehrenstein, U., Henningson, D.S.: Optimal growth, model reduction and control in a separated boundary-layer flow using global eigenmodes. J. Fluid Mech. 579, 305–314 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  3. Alves, L.S.B.: Preconditioned implicit Runge–Kutta schemes for unsteady simulations of low mach number compressible flows. In: Idelsohn, S., Sonzogni, V., Coutinho, A., Cruchaga, M., Lew, A., Cerrolaza M. (eds.) 1st Pan-American Congress on Computational Mechanics (2015)

  4. Alves, L.S.B., Kelly, R.E., Karagozian, A.R.: Transverse jet shear layer instabilities. Part II: linear analysis for large jet-to-crossflow velocity ratios. J. Fluid Mech. 602, 383–401 (2008)

    MATH  MathSciNet  Google Scholar 

  5. Bagheri, S., Schlatter, P., Schmid, P.J., Henningson, D.S.: Global stability of a jet in crossflow. J. Fluid Mech. 624, 33–44 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  6. Barletta, A., Alves, L.S.B.: Transition to absolute instability for (not so) dummies (2014). arXiv:1403.5794

  7. Barone, M.F., Lele, S.K.: Receptivity of the compressible mixing layer. J. Fluid Mech. 540, 301–335 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  8. Bijl, H., Carpenter, M.H., Vatsa, V.N., Kennedy, C.A.: Implicit time integration schemes for the unsteady compressible Navier–Stokes equations: laminar flow. J. Comput. Phys. 179, 313–329 (2002)

    Article  MATH  Google Scholar 

  9. Blaschak, J.G., Kriegsmann, G.A.: A comparative study of absorbing boundary conditions. J. Comput. Phys. 77, 109–139 (1988)

    Article  MATH  Google Scholar 

  10. Bodony, D.J.: An analysis of sponge zones for computational fluid mechanics. J. Comput. Phys. 212(2), 681–702 (2006)

    Article  MATH  MathSciNet  Google Scholar 

  11. Brevdo, L., Laure, P., Dias, F., Bridges, T.J.: Linear pulse structure and signalling in a film flow on an inclined plane. J. Fluid Mech. 396, 37–71 (1999)

    Article  MATH  MathSciNet  Google Scholar 

  12. Chomaz, J.M.: Global instabilities in spatially developing flows: non-normality and nonlinearity. Annu. Rev. Fluid Mech. 37, 357–392 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  13. Collis, S.S., Lele, S.K.: Receptivity to surface roughness near a swept leading edge. J. Fluid Mech. 380, 141–168 (1999)

    Article  MATH  Google Scholar 

  14. Colonius, T.: Modelling artificial boundary conditions for compressible flow. Annu. Rev. Fluid Mech. 136, 315–345 (2004)

    Article  MATH  Google Scholar 

  15. Colonius, T., Lele, S.K.: Computational aeroacoustics: progress on nonlinear problems of sound generation. Prog. Aerosp. Sci. 40, 345–416 (2004)

    Article  Google Scholar 

  16. Cunha, G., Passaggia, P.Y., Lazareff, M.: Optimization of the selective frequency damping parameters using model reduction. Phys. Fluids 27(094103), 1–22 (2015)

    Google Scholar 

  17. Dahlquist, G.: A special stability problem for linear multistep methods. BIT Numer. Math. 3, 27–43 (1963)

    Article  MATH  MathSciNet  Google Scholar 

  18. Falcao, C.E.G., Medeiros, F.E.L., Alves, L.S.B.: Implicit Runge–Kutta physical-time marching in low mach preconditioned density-based methods. In: 7th AIAA Theoretical Fluid Mechanics Conference, AIAA 2014-3085. AIAA Aviation (2014)

  19. Germanos, R.A.C., de Souza, L.F., de Medeiros, M.A.F.: Numerical investigation of the three-dimensional secondary instabilities in the time-developing compressible mixing layer. J. Braz. Soc. Mech. Sci. Eng. 31(2), 125–136 (2009)

    Article  Google Scholar 

  20. Jones, L.E., Sandberg, R.D., Sandham, N.D.: Stability and receptivity characteristics of a laminar separation bubble on an aerofoil. J. Fluid Mech. 648, 257–296 (2010)

    Article  MATH  Google Scholar 

  21. Jordi, B.E., Cotter, C.J., Sherwin, S.J.: Encapsulated formulation of the selective frequency damping method. Phys. Fluids 26(034101), 1–10 (2014)

    Google Scholar 

  22. Jordi, B.E., Cotter, C.J., Sherwin, S.J.: An adaptive selective frequency damping method. Phys. Fluids 27(094104), 1–8 (2015)

    Google Scholar 

  23. Kelly, R.E., Alves, L.S.B.: A uniformly valid asymptotic solution for the transverse jet and its linear stability analysis. Philos. Trans. R. Soc. Lond. Ser. A Math. Phys. Sci. 366, 2729–2744 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  24. Knoll, D.A., Keyes, D.E.: Jacobian-free Newton–Krylov method: a survey of approaches and applications. J. Comput. Phys. 193, 357–397 (2004)

    Article  MATH  MathSciNet  Google Scholar 

  25. Lardjane, N., Fedioun, I., Gokalp, I.: Accurate initial conditions for the direct numerical simulation of temporal compressible binary shear layers with high density ratio. Comput. Fluids 33, 549–576 (2004)

    Article  MATH  Google Scholar 

  26. Lax, P.D., Richtmyer, R.D.: Survey of the stability of linear finite difference equations. Commun. Pure Appl. Math. IX, 267–293 (1956)

    Article  MATH  MathSciNet  Google Scholar 

  27. Loiseau, J.C., Robinet, J.C., Cherubini, S., Leriche, E.: Investigation of the roughness-induced transition: global stability analyses and direct numerical simulations. J. Fluid Mech. 760, 175–211 (2014)

    Article  MathSciNet  Google Scholar 

  28. Lomax, H., Pulliam, T.H., Zingg, D.W.: Fundamentals of Computational Fluid Dynamics. Scientific Computation. Springer, Berlin (2001)

    Book  MATH  Google Scholar 

  29. Megerian, S., Davitian, J., Alves, L.S.B., Karagozian, A.R.: Transverse jet shear layer instabilities. Part I: experimental studies. J. Fluid Mech. 593, 93–129 (2007)

    Article  MATH  Google Scholar 

  30. Michalke, A.: Survey on jet instability theory. Prog. Aerosp. Sci. 21, 159–199 (1984)

    Article  Google Scholar 

  31. Pier, B.: Local and global instabilities in the wake of a sphere. J. Fluid Mech. 603, 39–61 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  32. Pulliam, T.H., Steger, J.L.: Implicit finite-difference simulations of three-dimensional compressible flow. AIAA J. 18(2), 159–167 (1980)

    Article  MATH  Google Scholar 

  33. Saric, W.S., Reed, H.L., White, E.B.: Stability and transition of three-dimensional boundary-layers. Annu. Rev. Fluid Dyn. 35, 413–440 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  34. Schmid, P.J.: Nonmodal stability theory. Annu. Rev. Fluid Mech. 39, 129–162 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  35. Sparrow, C.: The Lorenz Equations: Bifurcations, Chaos and Strange Attractors, Applied Mathematical Sciences, vol. 41. Springer, New York (1982)

    Book  MATH  Google Scholar 

  36. Steinberg, S., Roache, P.J.: Symbolic manipulation and computational fluid dynamics. J. Comput. Phys. 57, 251–284 (1985)

    Article  MATH  MathSciNet  Google Scholar 

  37. Teixeira, R.S., Alves, L.S.B.: Modeling far field entrainment in compressible flows. Int. J. Comput. Fluid Dyn. 26, 67–78 (2012)

    Article  MathSciNet  Google Scholar 

  38. Theofilis, V.: Global linear instability. Annu. Rev. Fluid Mech. 43, 319–352 (2011)

    Article  MATH  MathSciNet  Google Scholar 

  39. Wang, L., Mavriplis, D.J.: Implicit solution of the unsteady Euler equations for high-order accurate discontinuous Galerkin discretizations. J. Comput. Phys. 225(2), 1994–2015 (2007)

    Article  MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Programa de Pós-Graduação em Engenharia de Defesa, Instituto Militar de Engenharia, Rio de Janeiro, RJ, 22290-270, Brazil

    Renan de S. Teixeira

  2. Laboratório de Mecânica Teórica e Aplicada, Departamento de Engenharia Mecânica, Universidade Federal Fluminense, Niterói, RJ, 24210-240, Brazil

    Leonardo S. de B. Alves

Authors
  1. Renan de S. Teixeira
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Leonardo S. de B. Alves
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Leonardo S. de B. Alves.

Additional information

Communicated by Vassilios Theofilis.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

de S. Teixeira, R., S. de B. Alves, L. Minimal gain marching schemes: searching for unstable steady-states with unsteady solvers. Theor. Comput. Fluid Dyn. 31, 607–621 (2017). https://doi.org/10.1007/s00162-017-0426-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00162-017-0426-0

Keywords

Search

Navigation