Abstract
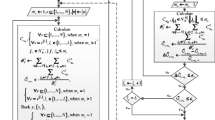
This paper introduces four new methods for robust design optimization (RDO) of complex engineering systems. The methods involve polynomial dimensional decomposition (PDD) of a high-dimensional stochastic response for statistical moment analysis, a novel integration of PDD and score functions for calculating the second-moment sensitivities with respect to the design variables, and standard gradient-based optimization algorithms. New closed-form formulae are presented for the design sensitivities that are simultaneously determined along with the moments. The methods depend on how statistical moment and sensitivity analyses are dovetailed with an optimization algorithm, encompassing direct, single-step, sequential, and multi-point single-step design processes. Numerical results indicate that the proposed methods provide accurate and computationally efficient optimal solutions of RDO problems, including an industrial-scale lever arm design.









Similar content being viewed by others
References
Browder A (1996) Mathematical analysis: an introduction. Undergraduate texts in mathematics. Springer, New York
Busbridge I (1948) Some integrals involving hermite polynomials. J Lond Math Soc 23:135–141
Chen W, Allen J, Tsui K, Mistree F (1996) Procedure for robust design: minimizing variations caused by noise factors and control factors. J Mech Des, Trans ASME 118(4):478–485
DOT (2001) Dot—designoptimization tools, user’s manual. Vanderplaats Research and Development, Inc., Colorado Springs, CO
Du XP, Chen W (2000) Towards a better understanding of modeling feasibility robustness in engineering design. J Mech Des 122(4):385–394
Efron B, Stein C (1981) The jackknife estimate of variance. Ann Stat 9(3):586–596
Gautschi W (2004) Orthogonal polynomials: computation and approximation. Numerical mathematics and scientific computation. Oxford University Press, Oxford
Huang B, Du X (2007) Analytical robustness assessment for robust design. Struct Multidisc Optim 34(2):123–137
Lee I, Choi KK, Du L, Gorsich D (2008) Dimension reduction method for reliability-based robust design optimization. Comput Struct 86(13–14):1550–1562
Lee S, Chen W, Kwak B (2009) Robust design with arbitrary distributions using gauss-type quadrature formula. Struct Multidisc Optim 39(3):227–243
Mourelatos Z, Liang J (2006) A methodology for trading-off performance and robustness under uncertainty. J Mech Des 128(4):856–863
Park G, Lee T, Kwon H, Hwang K (2006) Robust design: an overview. AIAA J 44(1):181–191
Rahman S (2008) A polynomial dimensional decomposition for stochastic computing. Int J Numer Methods Eng 76(13):2091–2116
Rahman S (2009a) Extended polynomial dimensional decomposition for arbitrary probability distributions. J Eng Mech ASCE 135(12):1439–1451
Rahman S (2009b) Stochastic sensitivity analysis by dimensional decomposition and score functions. Probab Eng Mech 24(3):278–287
Rahman S (2010) Statistical moments of polynomial dimensional decomposition. J Eng Mech ASCE 136(7):923–927
Rahman S (2011) Decomposition methods for structural reliability analysis revisited. Probab Eng Mech 26(2):357–363
Rahman S (2012) Approximation errors in truncated dimensional decompositions. Math Comput, submitted
Rahman S, Xu H (2004) A univariate dimension-reduction method for multi-dimensional integration in stochastic mechanics. Probab Eng Mech 19(4):393–408
Ramakrishnan B, Rao S (1996) A general loss function based optimization procedure for robust design. Eng Optim 25(4):255–276
Rubinstein R, Shapiro A (1993) Discrete event systems: sensitivity analysis and stochastic optimization by the score function method. Wiley series in probability and mathematical statistics. Wiley, New York
Sobol I (2003) Theorems and examples on high dimensional model representation. Reliab Eng Syst Saf 79(2):187–193
Stephens R, Fuchs H (2001) Metal fatigue in engineering. Wiley-Interscience, New York
Taguchi G (1993) Taguchi on robust technology development: bringing quality engineering upstream. ASME Press series on international advances in design productivity. ASME Press, New York
Toropov V, Filatov A, Polynkin A (1993) Multiparameter structural optimization using FEM and multipoint explicit approximations. Struct Multidisc Optim 6(1):7–14
Wang H, Kim N (2006) Robust design using stochastic response surface and sensitivities. In: 11th AIAA/ISSMO multidisciplinary analysis and optimization conference
Xu H, Rahman S (2004) A generalized dimension-reduction method for multidimensional integration in stochastic mechanics. Int J Numer Methods Eng 61(12):1992–2019
Youn B, Xi Z, Wang P (2008) Eigenvector dimension reduction (EDR) method for sensitivity-free probability analysis. Struct Multidisc Optim 37(1):13–28
Zaman K, McDonald M, Mahadevan S, Green L (2011) Robustness-based design optimization under data uncertainty. Struct Multidisc Optim 44(2):183–197
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Ren, X., Rahman, S. Robust design optimization by polynomial dimensional decomposition. Struct Multidisc Optim 48, 127–148 (2013). https://doi.org/10.1007/s00158-013-0883-z
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00158-013-0883-z