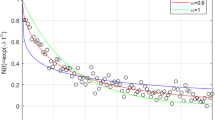
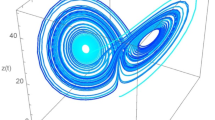
Summary
We consider a fractional-order differential equation involving fractal activity time to represent the stochastic behaviour of a log-price process of an underlying asset. The log-price process is defined in terms of fractional integration of the fractional derivative of Brownian motion on fractal time. A stable solution to the extrapolation and filtering problems associated is obtained in terms of covariance vaguelette functions (Angulo and Ruiz-Medina 1999). A simulation study is carried out to illustrate the methodology presented.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Adler, R.J. (1981), The Geometry of Random Fields, John Wiley, New York.
Angulo, J.M. and Ruiz-Medina, M.D. (1999), Multiresolution approximation to the stochastic inverse problem. Advances in Applied Probability, 31, 1039–1057.
Anh V.V., Angulo, J.M. and Ruiz-Medina, M.D. (1999), Possible long-range dependence in fractional random fields. Journal of Statistical Planning and Inference, 80, 95–110.
Benassi, A., Jaffard, S. and Roux, D. (1997), Elliptic Gaussian random processes. Revista Maternática Iberoamericana, 13, 19–90.
Calvet, L. and Fisher, A. (2001), Forecasting multifractal volatility. Journal of Econometrics, 105, 27–58.
Daubechies, I. (1992), Ten Lectures on Wavelets, SIAM, Philadelphia.
Donoho, D.L. (1995), Nonlinear solution of linear inverse problems by wavelet-vaguelette decomposition. Applied and Computational Harmonic Analysis, 2, 101–126.
Fisher, A., Calvet, L. and Mandelbrot, B. (1997), Multifractality of the Deutschmark/US Dollar exchange rates. Cowles Foundation Discussion Paper, 1165.
Hartvig, N.V., Jensen, J.L. and Pedersen, J. (2001), A class of risk neutral densities with heavy tails. Finance and Stochastics, 5, 115–128.
Heyde, C.C. (1999), A risky asset model with strong dependence through fractal activity time. Journal of Applied Probability, 36, 1234–1239.
Kato, T. (1995), Perturbation Theory of Linear Operators, Springer-Verlag, Berlin.
Mandelbrot, B., Fisher, A. and Calvet, L. (1997), A multifractal model of asset returns. Cowles Foundation Discussion Paper, 1164.
Meyer, Y. (1992), Wavelets and Operators, Cambridge University Press, Cambridge.
Podlubny, I. (1999), Fractional Differential Equations, Academic Press, Inc., San Diego.
Rozanov, Y.A. (1996), Random Fields and Stochastic Partial Differential Equations, Kluwer Academic Publishers, Dordrecht.
Ruiz-Medina, M.D., Angulo, J.M. and Anh, V.V. (2002), Stochastic fractional-order differential models on fractals. Theory of Probability and Mathematical Statistics, 67, 130–146.
Ruiz-Medina, M.D., Anh, V.V. and Angulo, J.M. (2001), Stochastic fractional-order differential models with fractal boundary conditions. Statistics and Probability Letters, 54, 47–60.
Samorodnitsky, G. and Taqqu, M.S. (1994), Stable Non-Gaussian Random Processes. Stochastic Models with Infinite Variance, Chapman & Hall, New York.
Triebel, H. (1997), Fractals and Spectra, Birkhäuser, Basel.
Wong, E. and Hajek, B. (1985), Stochastic Processes in Engineering Systems, Springer-Verlag, New York.
Zariphopoulou, T. (1997), A solution to valuation with unhedgeable risks. Finance and Stochastics, 5, 61–82.
Acknowledgments
This work has been supported in part by projects BFM2000-1465 and BFM2002-01836 of the DGI, Spain.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Fernández-Pascual, R., Ruiz-Medina, M.D. & Angulo, J.M. Multiscale estimation of processes related to the fractional Black-Scholes equation. Computational Statistics 18, 401–415 (2003). https://doi.org/10.1007/BF03354606
Published:
Issue Date:
DOI: https://doi.org/10.1007/BF03354606