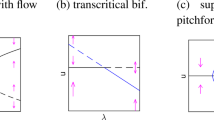
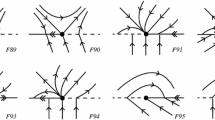
Summary
We explore the relation between the classical continuum model of Euler buckling and an iterated mapping which is not only a mathematical discretization of the former but also has an exact, discrete mechanical analogue. We show that the latter possesses great numbers of “parasitic” solutions in addition to the natural discretizations of classical buckling modes. We investigate this rich bifurcational structure using both mechanical analysis of the boundary value problem and dynamical studies of the initial value problem, which is the familiar standard map. We use this example to explore the links between discrete initial and boundary value problems and, more generally, to illustrate the complex relations among physical systems, continuum and discrete models and the analytical and numerical methods for their study.
Similar content being viewed by others
References
Amick, C., Ching, E. S. C., Kadanoff, L. P., and Rom-Kedar, V. (1992) Beyond All Orders: Singular Perturbations in a Mapping.J. of Nonlinear Science 2:9–67.
Antman, S. S., and Adler, C. L. (1987) Design of Material Properties that Yield a Prescribed Global Buckling Response.J. Applied Mech 109: 263–268.
Arnold, V. I. (1983) Geometrical Methods in the Theory of Ordinary Differential Equations. New York, Berlin, Heidelberg: Springer. (Grundlehren der Math. Wiss.250).
Aubry, S. (1983) The Twist Map, the Extended Frenkel-Kontorova Model and the Devil's Staircase.Physica D 7: 240–258.
Babuška, I. (1990) The Problem of Modeling the Elastomechanics in Engineering.Computer Methods in Mechanics and Engineering 82: 155–182.
Channel, P. J., and Scovel, C. (1990) Symplectic Integration of Hamiltonian Systems.Nonlinearity 3: 231–259.
Chenciner, A. (1983) Bifurcations de difféomorphismes deR 2 au voinsinage d'un point fixe élliptique. Les Houches Summer School Proceedings, ed. R. Helleman, G. Iooss, North Holland.
Chirikov, B. V. (1979) A Universal Instability of Many-Dimensional Oscillator Systems.Phys. Reports 52:263–379.
Coxeter, H. S. M. (1969)Introduction to Geometry. New York, NY, Chichester, England: John Wiley and Sons.
Crandall, M. G., and Rabinowitz, P. H. (1970) Nonlinear Sturm-Liouville Eigenvalue Problems and Topological Degree.J. Math. Mech. 19:1083–1102.
De Vogelaére, R. (1956) Methods of Integration Which Preserve the Contact Transformation Property of the Hamiltonian Equations. Department of Mathematics, University of Notre Dame, report4.
Devaney, R. L. (1986)An Introduction to Chaotic Dynamical Systems. Menlo Park, CA: The Benjamin/Cummings Publishing Co., Inc.
Domokos G. (1991) Computer Experiments with Elastic Chains.Newsletter of the Technical University of Budapest 9(1): 14–26.
Domokos G. (1992) Secondary Bifurcations in the Euler Problem.Newsletter of the Technical University of Budapest 10(1): 4–11.
El Naschie, M. S. (1990) On the Suspectibility of Local Elastic Buckling to Chaos.ZAMM 70(12): 535–542.
Euler, L. (1744)Methodus inveniendi lineas curvas maximi minimive proprietate gaudentes. Lausanne:Genf (German edition: Ostwald's Klassiker der Exakten Wiss.75 Leipzig: W. Engelmann).
Fontich, E. and Simo, C. (1990) The Splitting of Separatrices for Analytic Diffeomorphisms.J. Ergod Theory and Dynamical Systems 10: 295–318.
Gáspár Zs., and Domokos, G. (1989) Global Investigation of Discrete Models of the Euler Buckling Problem.Acta Technica Acad. Sci. Hung. 102(3–4): 227–238.
Greene, J. M. (1979) Method for Determining Stochastic Transition.J. Math. Phys. 20(6): 1183–1201.
Guckenheimer, J., and Holmes, P. (1983)Nonlinear Oscillations, Dynamical Systems and Bifurcation of Vector Fields. New York, Berlin, Heidelberg (Appl. Math. Sci. 42.)
Guckenheimer, J., Myers, M. R., Wicklin, F. J., and Worfolk, P. A. (1991) dstool: A Dynamical System Toolkit with an Interactive Graphical Interface.Center for Applied Mathematics, Cornell University.
Hegedüs I. (1986) Analysis of lattice single layer cylindrical structures.J. of Space Structures 2: 87–89.
Holmes, P. (1982) The Dynamics of Repeated Impact with a Sinusoidally Vibrating Table.J. of Sound and Vibration 84(2): 173–189.
Holmes, P., and Williams, R. F. (1985) Knotted Periodic Orbits in Suspensions of Smale's Horseshoe: Torus Knots and Bifurcation Sequences.Arch. Rat. Mech. Anal. 90(2): 115–194.
Kirchhoff, G. (1859) Über das Gleichgewicht und die Bewegung eines unendlich dünnen elastischen Stabes.J. für Math. (Crelle) 56: 285–313.
Lazutkin, V. F., Schachmannski, I. G., and Tabanov, M. B. (1989) Splitting of Separatrices for Standard and Semistandard Mappings.Physica D 40: 235–248.
Lichtenberg, A. J., and Lieberman, M. A. (1982)Regular and Stochastic Motion. New York, Berlin, Heidelberg: Springer. (Appl. Math. Sci.38).
Love, A. E. H. (1927)A Treatise on the Mathematical Theory of Elasticity. Dover Publications, N.Y.
Maddocks, J. H. (1984) Stability of Nonlinearly Elastic Rods.Arch. Rat. Mech. Anal. 85(4): 311–354.
Maddocks, J. H. (1987) Stability and Folds.Arch. Rat. Mech. 99(4): 301–328.
Marsden, J. E., O'Reilly, O., Wicklin, F. J., and Zombro, B. W. (1991) Symmetry, Stability, Geometric Phases and Mechanical Integrators.Nonlinear Sci. Today 1(1): 4–11,1(2): 14–21.
Melnikov, V. K. (1963) On the Stability of the Center for Time Periodic Perturbations.Trans. Moscow Math. Soc. 12: 1–57.
Meyer, K. (1970) Generic Bifurcation of Periodic Points.Trans. Ann. Math. Soc. 149: 95–107.
Meyer, K. (1971) Generic Stability Properties of Periodic Points.Trans. Ann. Math. Soc. 154: 273–277.
Mielke, A., and Holmes, P. (1988) Spatially Complex Equilibria of Buckled Rods.Arch. Rat. Mech. 101(4): 319–348.
Peitgen, H. O., Saupe, D., and Schmitt, K. (1981) Nonlinear Elliptic Boundary Value Problems Versus Finite Difference Approximations: Numerically Irrelevant Solutions.J. Reine u. Angew. Math. (Crelle) 322: 74–117.
Reinhall, P. G., Caughey, T. K., and Sorti, D. W. (1989) Order and Chaos in a Discrete Duffing Oscillator: Implications on Numerical Integration.J. Appl. Mech. 56(1): 162–167.
Rózsa P. (1974)Linear Algebra and Applications. (In Hungarian:Lineáris algebra és alkalmazásai) Budapest: Müszaki Könyvkiadó.
Thompson, J. M. T., and Virgin, L. N. (1988) Spatial Chaos and Localization Phenomena.Physics Letters A 126(8–9): 491–496.
Weinberger, H.F. (1974)Variational Methods for Eigenvalue Approximation. CBMS Conference Series 15,SIAM, Philadelphia.
Author information
Authors and Affiliations
Additional information
Communicated by Jerrold Marsden
Rights and permissions
About this article
Cite this article
Domokos, G., Holmes, P. Euler's problem, Euler's method, and the standard map; or, the discrete charm of buckling. J Nonlinear Sci 3, 109–151 (1993). https://doi.org/10.1007/BF02429861
Received:
Accepted:
Issue Date:
DOI: https://doi.org/10.1007/BF02429861