Skip to main content
SpringerLink
Log in

Numerical solution of Cauchy type singular integral equations with logarithmic weight, based on arbitrary collocation points

  • Published:
Computational Mechanics Aims and scope Submit manuscript

Abstract

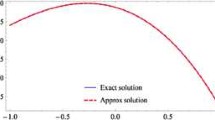
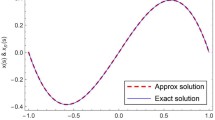
Singular integral equations with a Cauchy type kernel and a logarithmic weight function can be solved numerically by integrating them by a Gauss-type quadrature rule and, further, by reducing the resulting equation to a linear system by applying this equation at an appropriate number of collocation points x k. Until now these x k have been chosen as roots of special functions. In this paper, an appropriate modification of the original method permits the arbitrary choice of x k without any loss in the accuracy. The performance of the method is examined by applying it to a numerical example and a plane crack problem.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  • Danloy, B. (1973): Numerical construction of Gaussian quadrature formulas for 29-1. Math. Comput. 27, 861–869

    Google Scholar 

  • Erdogan, F.; Gupta, G. D.; Cook, T. S. (1973): Numerical solution of singular integral equations. In: Sih, G. C. (ed): Mechanics of fracture, vol. 1, Leyden: Noordhoff

    Google Scholar 

  • Erdogan, F.; Biricikoglu, V. (1973): Two bonded half planes with a crack going through the interface. Int. J. Eng. Sci. 11, 745–766

    Google Scholar 

  • Erdogan, F.; Cook, T. S. (1974): Antiplane shear crack terminating at and going through a bimaterial interface. Int. J. Fract. 10, 227–240

    Google Scholar 

  • Junghanns, P.; Silbermann, B. (1981): Zur Theorie der Näherung verfahren für singuläre Integralgleichungen auf Intervallen. Math. Nachr. 103, 199–244

    Google Scholar 

  • Ioakimidis, N. I. (1976): General methods for the solution of crack problems in the theory of plane elasticity. Doctoral Thesis at the National Technical University of Athens, Greece. Univ. Microfilms order no. 76-21, 056

  • Ioakimidis, N. I. (1980): The numerical solution of crack problems in plane elasticity in the case of loading discontinuities. Eng. Fract. Mech. 13, 709–716

    Google Scholar 

  • Ioakimidis, N. I. (1981): On the natural interpolation formula for Cauchy type integral equations of the first kind. Computing 26, 73–77

    Google Scholar 

  • Ioakimidis, N. I. (1984): Application of interpolation formulas to the numerical solution of singular integral equations. Serdica 10, 78–87

    Google Scholar 

  • Ioakimidis, N. I.; Theocaris, P. S. (1980): On the selection of collocation points for the numerical solution of singular integral equations with generalized kernels appearing in elasticity problems. Comput. Struct. 11, 289–295

    Google Scholar 

  • Kulich, N. V. (1974): The computation of certain Cauchy-type integrals and singular integrals with logarithmic singularities. Versi Akademii Navuk BSSR-Seriya Fizika-Matematychnykh 1, 90–94

    Google Scholar 

  • Muskhelishvili, N. I. (1975): Some basic problems of the mathematical theory of elasticity. Leyden: Noordhoff

    Google Scholar 

  • Stroud, A. H.; Secrest D. (1966): Gaussian quadrature formulas. Englewood, Cliffs, NJ: Prentice-Hall

    Google Scholar 

  • Theocaris, P. S.; Chrysakis, A. C.; Ioakimidis, N. I. (1979): Cauchy-type integrals and integral equations with logarithmic singularities. J. Eng. Math. 13, 63–74

    Google Scholar 

  • Tsamasphyros, G.; Theocaris, P. S. (1976): Sur une méthode génerale de quadrature des integrales du type Cauchy. Balkan Conference of Applied Mathematics in Salonica.

  • Tsamasphyros, G.; Theocaris, P. S. (1979): Numerical solution of systems of singular equations with variable coefficients. Appl. Anal. 7, 37–52

    Google Scholar 

  • Tsamasphyros, G.; Theocaris, P. S. (1981a); Are special collocation points necessary for the numerical solution of singular integral equations? Int. J. Fract. 17, R21-R24.

    Google Scholar 

  • Tsamasphyros, G.; Theocaris, P. S. (1981b): Equivalence and convergence of direct and indirect methods for the numerical solution of singular integral equations. Computing 27, 71–80

    Google Scholar 

  • Tsamasphyros, G. (1986): A study of factors influencing the solution of singular integral equations. Eng. Fract. Mech. 24, 567–578

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Section of Mechanics, Department of Engineering Science, The National Technical University of Athens, 5, Heroes of Polytechnion Avenue, GR-15773, Athens, Greece

    A. C. Chrysakis & G. Tsamasphyros

Authors
  1. A. C. Chrysakis
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. G. Tsamasphyros
    View author publications

    You can also search for this author in PubMed Google Scholar

Additional information

Communicated by D. E. Beskos, March 8, 1990

Rights and permissions

Reprints and permissions

About this article

Cite this article

Chrysakis, A.C., Tsamasphyros, G. Numerical solution of Cauchy type singular integral equations with logarithmic weight, based on arbitrary collocation points. Computational Mechanics 7, 21–29 (1990). https://doi.org/10.1007/BF00370054

Download citation

  • Issue Date:

  • DOI: https://doi.org/10.1007/BF00370054

Keywords

Search

Navigation