Abstract
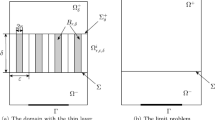
We show that the spectrum of the Dirichlet problem for the Laplace operator in a layer with a doubly periodic structure has gaps and determine several characteristics of their location. The result is obtained by asymptotic analysis of a model spectral problem on the periodicity cell.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
M. Sh. Birman and M. Z. Solomjak, Spectral Theory of Selfadjoint Operators in Hilbert Space [in Russian], Leningrad State Univ. Press, Leningrad (1980); English transl., D. Reidel, Dordrecht (1987).
P. A. Kuchment, Russian Math. Surveys, 37, 1–60 (1982).
M. M. Skriganov, Geometric and Arithmetical Methods in the Spectral Theory of Multidimensional Periodic Operators [in Russian] (Trudy Mat. Inst. Akad. Nauk, Vol. 171), Nauka, Leningrad (1985).
P. Kuchment, Floquet Theory for Partial Differential Equations (Operator Theory Adv. Appl., Vol. 60), Birchäuser, Basel (1993).
I. M. Gel’fand, Dokl. Akad. Nauk SSSR, 73, 1117–1120 (1950).
O. A. Ladyzhenskaya, Boundary Value Problems of Mathematical Physics [in Russian], Nauka, Moscow (1973); English transl. (Appl. Math. Sci., Vol. 49), Springer, New York (1985).
I. V. Kamotskii and S. A. Nazarov, “On eigenfunctions localized near the edge of a thin domain [in Russian],” in: Problemy Mat. Analiza, No. 19, Nauchnaya Kniga, Novosibirsk (1999), pp. 105–148.
S. A. Nazarov, Math. Bohem., 127, 283–292 (2002).
G. Cardone, T. Durante, and S. A. Nazarov, SIAM J. Math. Anal., 42, 2581–2609 (2010).
L. Friedlander and M. Solomyak, Russ. J. Math. Phys., 15, 238–242 (2008).
L. Friedlander and M. Solomyak, Israel J. Math., 170, 337–354 (2009).
K. Yoshitomi, J. Differ. Equations, 142, 123–166 (1998).
V. Kozlov, J. Differ. Equations, 230, 532–555 (2006).
J. Hadamard, “Mémoire sur le problème d’analyse relatif à l’équilibre des plaques èlastiques encastrés,” in: OEuvres, Vol. 2, Editions du CNRS, Paris (1968), pp. 515–631.
L. A. Ivanov, L. A. Kotko, and S. G. Krein, “Boundary value problems in variable domains [in Russian],” in: Differents. uravn. i ikh primen., No. 19, Izdat. Akad. Nauk Lit.SSR, Vilnius (1977).
M. I. Vishik and L. A. Lyusternik, Amer. Math. Soc. Transl. (2), 20, 239–364 (1962).
S. A. Nazarov, Asymptotic Theory of Thin Plates and Rods: Dimensionality Reduction and Integral Estimates [in Russian], Nauchnaya Kniga, Novosibirsk (2002).
W. G. Mazja, S. A. Nazarov, and B. A. Plamenewski, Asymptotische Theorie elliptischer Randwertaufgaben in singulär gestörten Gebieten (Math. Lehrbücher Monogr., Vol. 82), Vol. 1, Akademie, Berlin (1991).
S. A. Nazarov and M. V. Olyushin, St. Petersburg Math. J., 5, 371–387 (1994).
S. A. Nazarov, “On optimization of a patch [in Russian],” in: Problemy Mat. Analiza, No. 42, Nauchnaya Kniga, Novosibirsk (2009), pp. 65–82.
S. A. Nazarov, K. Ruotsalainen, and Ya. Taskinen, “Spectral gaps in Dirichlet and Neumann problems on a plane perforated by a doubly periodic family of circular holes [in Russian],” in: Problemy Mat. Analiza, No. 62, Nauchnaya Kniga, Novosibirsk (2011), pp. 51–100.
Author information
Authors and Affiliations
Corresponding author
Additional information
__________
Translated from Teoreticheskaya i Matematicheskaya Fizika, Vol. 174, No. 3, pp. 398–415, March, 2013.
Rights and permissions
About this article
Cite this article
Nazarov, S.A. Spectral properties of a thin layer with a doubly periodic family of thinning regions. Theor Math Phys 174, 343–359 (2013). https://doi.org/10.1007/s11232-013-0031-3
Received:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11232-013-0031-3