Skip to main content
SpringerLink
Log in

Changes in tendon stiffness and running economy in highly trained distance runners

  • Original Article
  • Published:
European Journal of Applied Physiology Aims and scope Submit manuscript

Abstract

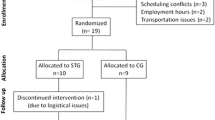
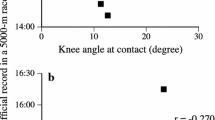
The purpose of this study was to determine if changes in triceps-surae tendon stiffness (TST K) could affect running economy (RE) in highly trained distance runners. The intent was to induce increased TST K in a subgroup of runners by an added isometric training program. If TST K is a primary determinant of RE, then the energy cost of running (EC) should decrease in the trained subjects. EC was measured via open-circuit spirometry in 12 highly trained male distance runners, and TST K was measured using ultrasonography and dynamometry. Runners were randomly assigned to either a training or control group. The training group performed 4 × 20 s isometric contractions at 80% of maximum voluntary plantarflexion moment three times per week for 8 weeks. All subjects (mean \( \dot{V}{\text{O}}_{ 2} { \max } \) = 67.4 ± 4.6 ml kg−1 min−1) continued their usual training for running. TST K was measured every 2 weeks. EC was measured in both training and control groups before and after the 8 weeks at three submaximal velocities, corresponding to 75, 85 and 95% of the speed at lactate threshold (sLT). Isometric training did neither result in a mean increase in TST K (0.9 ± 25.8%) nor a mean improvement in RE (0.1 ± 3.6%); however, there was a significant relationship (r 2 = 0.43, p = 0.02) between the change in TST K and change in EC, regardless of the assigned group. It was concluded that TST K and EC are somewhat labile and change together.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  • Alexander RM (1974) The mechanics of jumping by a dog (Canis familiaris). J Zool 173:549–573

    Article  Google Scholar 

  • An KN, Takahashi K, Harrigan TP, Chao EY (1984) Determination of muscle orientations and moment arms. J Biomech Eng 106:280–282

    Article  CAS  PubMed  Google Scholar 

  • Arampatzis A, Morey-Klapsing G, Karamanidis K, DeMonte G, Stafilidis S, Bruggemann GP (2005) Differences between measured and resultant joint moments during isometric contractions at the ankle joint. J Biomech 38:885–892

    Google Scholar 

  • Arampatzis A, De Monte G, Karamanidis K, Morey-Klapsing G, Stafilidis S, Bruggemann GP (2006) Influence of the muscle-tendon unit’s mechanical and morphological properties on running economy. J Exp Biol 209:3345–3357

    Article  PubMed  Google Scholar 

  • Arampatzis A, Karamanidis K, Albracht K (2007) Adaptational responses of the human Achilles tendon by modulation of the applied cyclic strain magnitude. J Exp Biol 210:2743–2753

    Article  PubMed  Google Scholar 

  • Biewener AA, Roberts TJ (2000) Muscle and tendon contributions to force, work, and elastic energy savings: a comparative perspective. Exerc Sport Sci Rev 28:99–107

    CAS  PubMed  Google Scholar 

  • Chow JW, Darling WG (1999) The maximum shortening velocity of muscle should be scaled with activation. J Appl Physiol 86:1025–1031

    CAS  PubMed  Google Scholar 

  • Fletcher JR, Esau SP, MacIntosh BR (2009) Economy of running: beyond the measurement of oxygen uptake. J Appl Physiol 107:1918–1922

    Article  PubMed  Google Scholar 

  • Hof AL, Van Zandwijk JP, Bobbert MF (2002) Mechanics of human triceps surae muscle in walking, running and jumping. Acta Physiol Scand 174:17–30

    Article  CAS  PubMed  Google Scholar 

  • Hunter I, Smith GA (2007) Preferred and optimal stride frequency, stiffness and economy: changes with fatigue during a 1-h high-intensity run. Eur J Appl Physiol 100:653–661

    Article  PubMed  Google Scholar 

  • Ishikawa M, Komi PV (2008) Muscle fascicle and tendon behavior during human locomotion revisited. Exerc Sport Sci Rev 36:193–199

    Article  PubMed  Google Scholar 

  • Ishikawa M, Pakaslahti J, Komi PV (2007) Medial gastrocnemius muscle behavior during human running and walking. Gait Posture 25:380–384

    Article  CAS  PubMed  Google Scholar 

  • Ito M, Akima H, Fukunaga T (2000) In vivo moment arm determination using B-mode ultrasonography. J Biomech 33:215–218

    Article  CAS  PubMed  Google Scholar 

  • Jones AM, Carter H (2000) The effect of endurance training on parameters of aerobic fitness. Sports Med 29:373–386

    Article  CAS  PubMed  Google Scholar 

  • Kay AD, Blazevich AJ (2009) Isometric contractions reduce plantar flexor moment, Achilles tendon stiffness, and neuromuscular activity but remove the subsequent effects of stretch. J Appl Physiol 107:1181–1189

    Article  PubMed  Google Scholar 

  • Kubo K, Kanehisa H, Kawakami Y, Fukunaga T (2000a) Elasticity of tendon structures of the lower limbs in sprinters. Acta Physiol Scand 168:327–335

    Article  CAS  PubMed  Google Scholar 

  • Kubo K, Kanehisa H, Kawakami Y, Fukunaga T (2000b) Elastic properties of muscle-tendon complex in long-distance runners. Eur J Appl Physiol 81:181–187

    Article  CAS  PubMed  Google Scholar 

  • Kubo K, Kanehisa H, Fukunaga T (2001a) Effects of different duration isometric contractions on tendon elasticity in human quadriceps muscles. J Physiol 536:649–655

    Article  CAS  PubMed  Google Scholar 

  • Kubo K, Kanehisa H, Ito M, Fukunaga T (2001b) Effects of isometric training on the elasticity of human tendon structures in vivo. J Appl Physiol 91:26–32

    CAS  PubMed  Google Scholar 

  • Kubo K, Kanehisa H, Kawakami Y, Fukunaga T (2001c) Effects of repeated muscle contractions on the tendon structures in humans. Eur J Appl Physiol 84:162–166

    Article  CAS  PubMed  Google Scholar 

  • Kubo K, Kanehisa H, Kawakami Y, Fukunaga T (2001d) Influences of repetitive muscle contractions with different modes on tendon elasticity in vivo. J Appl Physiol 91:277–282

    CAS  PubMed  Google Scholar 

  • Kubo K, Kanehisa H, Fukunaga T (2002) Effects of resistance and stretching training programmes on the viscoelastic properties of human tendon structures in vivo. J Physiol 538:219–226

    Article  CAS  PubMed  Google Scholar 

  • Kubo K, Yata H, Kanehisa H, Fukunaga T (2006) Effects of isometric squat training on the tendon stiffness and jump performance. Eur J Appl Physiol 96:305–314

    Article  PubMed  Google Scholar 

  • Lichtwark GA, Barclay CJ (2010) The influence of tendon compliance on muscle power output and efficiency during cyclic contractions. J Exp Biol 213:707–714

    Article  CAS  PubMed  Google Scholar 

  • Lichtwark GA, Wilson AM (2006) Interactions between the human gastrocnemius muscle and the Achilles tendon during incline, level and decline locomotion. J Exp Biol 209:4379–4388

    Article  CAS  PubMed  Google Scholar 

  • Lichtwark GA, Wilson AM (2007) Is Achilles tendon compliance optimised for maximum muscle efficiency during locomotion? J Biomech 40:1768–1775

    Article  CAS  PubMed  Google Scholar 

  • Lichtwark GA, Wilson AM (2008) Optimal muscle fascicle length and tendon stiffness for maximising gastrocnemius efficiency during human walking and running. J Theor Biol 252:662–673

    Article  CAS  PubMed  Google Scholar 

  • Lichtwark GA, Bougoulias K, Wilson AM (2007) Muscle fascicle and series elastic element length changes along the length of the human gastrocnemius during walking and running. J Biomech 40:157–164

    Article  CAS  PubMed  Google Scholar 

  • Mademli L, Arampatzis A (2008) Mechanical and morphological properties of the triceps surae muscle-tendon unit in old and young adults and their interaction with a submaximal fatiguing contraction. J Electromyogr Kinesiol 18:89–98

    Article  PubMed  Google Scholar 

  • Mademli L, Arampatzis A, Walsh M (2006) Effect of muscle fatigue on the compliance of the gastrocnemius medialis tendon and aponeurosis. J Biomech 39:426–434

    Article  PubMed  Google Scholar 

  • Maganaris CN (2000) In vivo measurement-based estimations of the moment arm in the human tibialis anterior muscle-tendon unit. J Biomech 33:375–379

    Article  CAS  PubMed  Google Scholar 

  • Maganaris CN, Paul JP (1999) In vivo human tendon mechanical properties. J Physiol 521(Pt 1):307–313

    Article  CAS  PubMed  Google Scholar 

  • Maxwell SE, Delaney HD (2004) Designing experiments and analyzing data: a model comparison perspective. Routledge, New York

    Google Scholar 

  • Muramatsu T, Muraoka T, Takeshita D, Kawakami Y, Hirano Y, Fukunaga T (2001) Mechanical properties of tendon and aponeurosis of human gastrocnemius muscle in vivo. J Appl Physiol 90:1671–1678

    CAS  PubMed  Google Scholar 

  • Nader GA (2006) Concurrent strength and endurance training: from molecules to man. Med Sci Sports Exerc 38:1965–1970

    Article  PubMed  Google Scholar 

  • Nicol C, Komi PV, Marconnet P (1991) Fatigue effects of marathon running on neuromuscular performance. Scand J Med Sci Sports 1:10–17

    Article  Google Scholar 

  • Pollock ML (1977) Submaximal and maximal working capacity of elite distance runners. Part I: Cardiorespiratory aspects. Ann N Y Acad Sci 301:310–322

    Article  CAS  PubMed  Google Scholar 

  • Roberts TJ (2002) The integrated function of muscles and tendons during locomotion. Comp Biochem Physiol A Mol Integr Physiol 133:1087–1099

    Article  PubMed  Google Scholar 

  • Rosager S, Aagaard P, Dyhre-Poulsen P, Neergaard K, Kjaer M, Magnusson SP (2002) Load-displacement properties of the human triceps surae aponeurosis and tendon in runners and non-runners. Scand J Med Sci Sports 12:90–98

    Article  CAS  PubMed  Google Scholar 

  • Saunders PU, Pyne DB, Telford RD, Hawley JA (2004) Factors affecting running economy in trained distance runners. Sports Med 34:465–485

    Article  PubMed  Google Scholar 

  • Spoor CW, van Leeuwen JL, Meskers CG, Titulaer AF, Huson A (1990) Estimation of instantaneous moment arms of lower-leg muscles. J Biomech 23:1247–1259

    Article  CAS  PubMed  Google Scholar 

  • Spurrs RW, Murphy AJ, Watsford ML (2003) The effect of plyometric training on distance running performance. Eur J Appl Physiol 89:1–7

    Article  PubMed  Google Scholar 

  • Stafilidis S, Arampatzis A (2007) Muscle–tendon unit mechanical and morphological properties and sprint performance. J Sports Sci 25:1035–1046

    Article  PubMed  Google Scholar 

  • Stainsby WN, Lambert CR (1979) Determination of oxygen uptake in skeletal muscle. Exerc Sport Sci Rev 7:125–151

    Article  CAS  PubMed  Google Scholar 

  • Storen O, Helgerud J, Stoa EM, Hoff J (2008) Maximal strength training improves running economy in distance runners. Med Sci Sports Exerc 40:1087–1092

    Article  PubMed  Google Scholar 

  • Svedahl K, MacIntosh BR (2003) Anaerobic threshold: the concept and methods of measurement. Can J Appl Physiol 28:299–323

    CAS  PubMed  Google Scholar 

  • Wang XT, Ker RF, Alexander RM (1995) Fatigue rupture of wallaby tail tendons. J Exp Biol 198:847–852

    CAS  PubMed  Google Scholar 

  • Xu F, Montgomery DL (1995) Effect of prolonged exercise at 65 and 80% of VO2max on running economy. Int J Sports Med 16:309–313

    Article  CAS  PubMed  Google Scholar 

Download references

Acknowledgments

The authors would like to thank NSERC Canada and the National Aboriginal Achievement Foundation of Canada for financial support. They also thank the subjects participating in this study for their dedication and cooperation. Adamantios Arampatzis and his students are also acknowledged for spending the time to demonstrate the use of ultrasound imaging for quantitative evaluation of muscle fiber orientation and tendon translation.

Author information

Authors and Affiliations

  1. Human Performance Laboratory, Faculty of Kinesiology, University of Calgary, Calgary, AB, T2N 1N4, Canada

    Jared R. Fletcher, Shane P. Esau & Brian R. MacIntosh

Authors
  1. Jared R. Fletcher
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Shane P. Esau
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Brian R. MacIntosh
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Brian R. MacIntosh.

Additional information

Communicated by Susan Ward.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Fletcher, J.R., Esau, S.P. & MacIntosh, B.R. Changes in tendon stiffness and running economy in highly trained distance runners. Eur J Appl Physiol 110, 1037–1046 (2010). https://doi.org/10.1007/s00421-010-1582-8

Download citation

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00421-010-1582-8

Keywords

Search

Navigation