Skip to main content
SpringerLink
Log in

Convergence analysis of multilevel Monte Carlo variance estimators and application for random obstacle problems

  • Published:
Numerische Mathematik Aims and scope Submit manuscript

Abstract

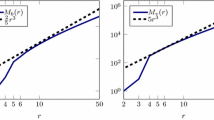
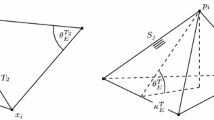
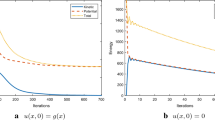
We develop a novel convergence theory for the multilevel sample variance estimators in the framework of the multilevel Monte Carlo methods. We prove that, dependent on the regularity of the quantity of interest, the multilevel sample variance estimator may achieve the same asymptotic cost/error relation as the multilevel sample mean, which is superior to the standard Monte Carlo method. Weaker regularity assumptions result in reduced convergence rates, quantified in our analysis. The general convergence theory is applied to a class of scalar elliptic obstacle problems with rough random obstacle profiles, which is a simple model of contact between a deformable body with a rough uncertain substrate. Numerical experiments confirm theoretical convergence proofs.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16

Similar content being viewed by others

References

  1. Adams, R.A.: Sobolev Spaces, vol. 65. Academic Press [A subsidiary of Harcourt Brace Jovanovich, Publishers], New York, London (1975)

    MATH  Google Scholar 

  2. Babuška, I., Nobile, F., Tempone, R.: A stochastic collocation method for elliptic partial differential equations with random input data. SIAM J. Numer. Anal. 45(3), 1005–1034 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  3. Barth, A., Schwab, C., Zollinger, N.: Multi-level Monte Carlo finite element method for elliptic PDEs with stochastic coefficients. Numer. Math. 119(1), 123–161 (2011)

    Article  MATH  MathSciNet  Google Scholar 

  4. Brezis, H.: Nouveaux théorèmes de régularité pour les problèmes unilatéraux. Rencontre entre physiciens théoriciens et mathématiciens 12, pp. 14. Strasbourg,(1971)

  5. Brezzi, F., Hager, W.W., Raviart, P.-A.: Error estimates for the finite element solution of variational inequalities. Numer. Math. 28(4), 431–443 (1977)

    Article  MATH  MathSciNet  Google Scholar 

  6. Bungartz, H.-J., Griebel, M.: Sparse grids. Acta Numer. 13, 147–269 (2004)

    Article  MathSciNet  Google Scholar 

  7. Chernov, A., Schwab, C.: First order \(k\)-th moment finite element analysis of nonlinear operator equations with stochastic data. Math. Comput. 82(284), 1859–1888 (2013)

    Article  MATH  MathSciNet  Google Scholar 

  8. Ciarlet, P.G.: The Finite Element Method for Elliptic Problems. Studies in Mathematics and its Applications, vol. 4. North-Holland Publishing Co., Amsterdam (1978)

    Google Scholar 

  9. Cliffe, K.A., Giles, M.B., Scheichl, R., Teckentrup, A.L.: Multilevel Monte Carlo methods and applications to elliptic PDEs with random coefficients. Comput. Vis. Sci. 14(1), 3–15 (2011)

    Article  MATH  MathSciNet  Google Scholar 

  10. Da Prato, G.: An introduction to infinite-dimensional analysis. Universitext, Springer, Berlin (2006), (Revised and extended from the 2001 original by Da Prato)

  11. Forster, R., Kornhuber, R.: A polynomial chaos approach to stochastic variational inequalities. J. Numer. Math. 18(4), 235–255 (2010)

  12. Giles, M.: Improved multilevel Monte Carlo convergence using the Milstein scheme. In: Monte Carlo and quasi-Monte Carlo methods 2006, pp. 343–358. Springer, Berlin, Heidelberg (2008)

  13. Giles, M.B.: Multilevel Monte Carlo path simulation. Oper. Res. 56(3), 607–617 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  14. Glowinski, R.: Numerical Methods for Nonlinear Variational Problems. Springer Series in Computational Physics, Springer, New York (1984)

    Book  MATH  Google Scholar 

  15. Gräser, C., Kornhuber, R.: Multigrid methods for obstacle problems. J. Comput. Math. 27(1), 1–44 (2009)

    MATH  MathSciNet  Google Scholar 

  16. Hackbusch, W.: Multigrid Methods and Applications, Volume 4 of Springer Series in Computational Mathematics. Springer, Berlin (1985)

    Google Scholar 

  17. Kornhuber, R.: Monotone multigrid methods for elliptic variational inequalities. I. Numer. Math. 69(2), 167–184 (1994)

    Article  MATH  MathSciNet  Google Scholar 

  18. Kornhuber, R., Schwab, C., Wolf, M.-W.: Multi-level Monte-Carlo finite element methods for stochastic elliptic variational inequalities. SIAM J. Numer. Anal. 52(3), 1243–1268 (2014)

    Article  MathSciNet  Google Scholar 

  19. Ledoux, M., Talagrand, M.: Probability in Banach Spaces, Volume 23 of Ergebnisse der Mathematik und ihrer Grenzgebiete (3) [Results in Mathematics and Related Areas (3)]. Springer, Berlin (1991). (Isoperimetry and processes)

  20. Light, W.A., Cheney, E.W.: Approximation Theory in Tensor Product Spaces. Lecture Notes in Mathematics, vol. 1169. Springer, Berlin (1985)

    Google Scholar 

  21. Mishra, S., Schwab, C., Šukys, J.: Multi-level Monte Carlo finite volume methods for nonlinear systems of conservation laws in multi-dimensions. J. Comput. Phys. 231(8), 3365–3388 (2012)

    Article  MATH  MathSciNet  Google Scholar 

  22. Pébay, P.: Formulas for robust, one-pass parallel computation of covariances and arbitrary-order statistical moments. Sandia Report SAND2008-6212, Sandia National Laboratories (2008)

  23. Persson, B.N.J., Albohr, O., Tartaglino, U., Volokitin, A.I., Tosatti, E.: On the nature of surface roughness with application to contact mechanics, sealing, rubber friction and adhesion. J. Phys. Condens. Matter 17, R1–R62 (2005)

    Article  Google Scholar 

  24. Prévôt, C., Röckner, M.: A Concise Course on Stochastic Partial Differential Equations. Lecture Notes in Mathematics, vol. 1905. Springer, Berlin (2007)

  25. Schwab, C., Gittelson, C.J.: Sparse tensor discretizations of high-dimensional parametric and stochastic PDEs. Acta Numer. 20, 291–467 (2011)

    Article  MATH  MathSciNet  Google Scholar 

  26. Tai, X.-C.: Rate of convergence for some constraint decomposition methods for nonlinear variational inequalities. Numer. Math. 93(4), 755–786 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  27. Welford, B.P.: Note on a method for calculating corrected sums of squares and products. Technometrics 4, 419–420 (1962)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgments

The authors thank Prof. Christoph Schwab, Dr. Annika Lang and Jonas Šukys (ETH Zürich) for the discussion on unbiased variance estimators, and Prof. Rolf Krause (USI, Lugano) for mentioning the reference [23].

Author information

Authors and Affiliations

  1. Department of Mathematics and Statistics, University of Reading, Whiteknights Campus, PO Box 220, Reading, RG6 6AX, UK

    Claudio Bierig & Alexey Chernov

Authors
  1. Claudio Bierig
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Alexey Chernov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Alexey Chernov.

Additional information

The major part of the research presented in this paper has been carried out at the Hausdorff Center of Mathematics, University of Bonn, Germany. The authors acknowledge support by HCM and by the University of Reading.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Bierig, C., Chernov, A. Convergence analysis of multilevel Monte Carlo variance estimators and application for random obstacle problems. Numer. Math. 130, 579–613 (2015). https://doi.org/10.1007/s00211-014-0676-3

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00211-014-0676-3

Mathematics Subject Classification

Search

Navigation