Catalyst Layer Performance in PEM Fuel Cell: Analytical Solutions

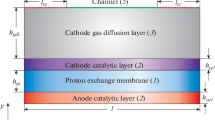
Abstract

We report the most general analytical solutions to a macrohomogeneous model for performance of the cathode catalyst layer (CCL) in PEM fuel cell. Equations for the shapes of local parameters through the CCL thickness and for the CCL polarization curve are derived for the regime of mixed oxygen- and proton-transport limitations. In the limit of ideal oxygen transport, an equation for the CCL polarization curve valid in the whole range of currents is obtained. Good agreement between analytical and exact numerical results is demonstrated.

Appendices

Appendix

Here, we report the details of the derivation of Eq. 15. Integrating Eq. 13 once, we get

$$ \dfrac{\partial {\tilde{j}}}{\partial {\tilde{x}}} + \dfrac{\tilde{j}^2}{2} - \gamma\tilde{j} = \left.\dfrac{\partial {\tilde{j}}}{\partial {\tilde{x}}} \right|_1 \equiv -\dfrac{\beta^2}{2} $$

(30)

where β is constant. Equation 30 can, in principle, further be integrated; however, this leads to rather cumbersome expressions. Fortunately, smallness of γ allows us to employ asymptotic technique. We write

$$ \tilde{j}\simeq \tilde{j}^0 + \gamma\tilde{j}^1 $$

(31)

where $\tilde{j}^0$ is the leading-order solution and $\tilde{j}^1$ is the first-order correction. Substituting Eq. 31 into Eq. 30, collecting the terms with like powers of γ and neglecting the terms with γ ², we get equations for $\tilde{j}^0$ and $\tilde{j}^1$:

$$ \dfrac{\partial {\tilde{j}^0}}{\partial {\tilde{x}}} + \dfrac{\left(\tilde{j}^0\right)^2}{2} = -\dfrac{\beta^2}{2}, \quad \tilde{j}^0(1) = 0 \\ $$

(32)

$$ \dfrac{\partial {\tilde{j}^1}}{\partial {\tilde{x}}} + \tilde{j}^0\tilde{j}^1 = \tilde{j}^0, \quad \tilde{j}^1(1)=0 $$

(33)

The solution to Eq. 32 is

$$ \tilde{j}^0 = \beta\tan\left(\dfrac{\beta}{2}(1-\tilde{x}) \right) \ \mbox{.} $$

(34)

With this $\tilde{j}^0$, the solution to Eq. 33 reads

$$ \tilde{j}^1 = - \dfrac{1}{2} \left( 1 + \tan^2\left(\dfrac{\beta}{2}\bigl( 1-\tilde{x}\bigr) \right)\right) \bigl( 1 - \cos\left(\beta(1-\tilde{x})\right)\bigr) $$

(35)

The oxygen concentration and parameter γ can now be calculated using the leading-order solution $\tilde{j}^0$ in equation for $\tilde{c}$. Substituting $\tilde{j}=\tilde{j}^0$ into Eq. 8, we get

$$ \tilde{D}\dfrac{\partial {\tilde{c}}}{\partial {\tilde{x}}} = \tilde{j}_0 - \beta\tan\left(\dfrac{\beta}{2}(1-\tilde{x}) \right), \quad \tilde{c}(1)= \tilde{c}_1 $$

(36)

where $\tilde{c}_1$ is the oxygen concentration at the CCL/GDL interface. Solving this equation, we find

$$ \tilde{c} = \tilde{c}_1 - \dfrac{\tilde{j}_0}{\tilde{D}}\left( 1 - \tilde{x} \right) + \dfrac{1}{\tilde{D}} \ln\left( 1 + \tan^2\left(\dfrac{\beta}{2}\bigl( 1-\tilde{x}\bigr) \right)\right) $$

(37)

Calculating ${\partial {\ln\tilde{c}}}/{\partial {\tilde{x}}}$ and setting $\tilde{x}=1$ in the resulting expression, we find

$$ \gamma = \dfrac{\tilde{j}_0}{\tilde{D}\tilde{c}_1} $$

(38)

Thus, the analysis above is valid provided that $\tilde{j}_0/(\tilde{D}\tilde{c}_1) \ll 1$.

The approximate solution to Eq. 13 is given by Eq. 31 with $\tilde{j}^0$, $\tilde{j}^1$, and γ given by Eqs. 34, 35, and 38, respectively. Parameter β can be found from the condition $\tilde{j}(0) = \tilde{j}_0$; with Eq. 31, this leads to the following equation:

$$ \beta\tan\left(\dfrac{\beta}{2}\right) - \dfrac{\tilde{j}_0\bigl( 1 - \cos\beta \bigr)}{2\tilde{D}\tilde{c}_1} \left( 1 + \tan^2\left(\dfrac{\beta}{2}\right)\right)= \tilde{j}_0 $$

(39)

The shape of local overpotential can now be calculated from Eq. 6:

$$ \tilde{\eta} = {\rm{arcsinh}}\left( - \dfrac{\varepsilon^2}{\tilde{c}}\dfrac{\partial {\tilde{j}}}{\partial {\tilde{x}}} \right) \mbox{.} $$

(40)

Using here Eqs. 31, 34, 35, and 37, we come up with Eq. 15.

Nomenclature

$\tilde{~}$ :

Marks dimensionless variables

b :

Tafel slope (V)

c :

Oxygen molar concentration (mol cm^− 3)

c _ref :

Reference oxygen molar concentration (mol cm^− 3)

D :

Oxygen diffusion coefficient in the CCL (cm² s^− 1)

D _* :

Characteristic oxygen diffusivity (cm² s^− 1), Eq. 5

D _b :

Oxygen diffusion coefficient in the GDL (cm² s^− 1)

F :

Faraday constant

j ₀ :

Cell current density (A cm^− 2)

j :

Local proton current density in the CCL (A cm^− 2)

j _* :

Characteristic current density (A cm^− 2), Eq. 5

i _* :

Volumetric exchange current density (A cm^− 3)

l _b :

GDL thickness (cm)

l _t :

Catalyst layer thickness (cm)

n :

Number of electrons transferred in the half-cell reaction

x :

Coordinate across the CCL (cm)

Subscripts

0:

Membrane/CCL interface

1:

CCL/GDL interface

b :

GDL

t :

Catalyst layer

*:

Characteristic value

Greek

β :

Dimensionless parameter, solution to Eq. 39, or Eq. 19

γ :

Small parameter, Eq. 14

η :

Overpotential (V)

η ₀ :

Overpotential at x = 0, total voltage loss in the CCL (V)

ε :

Dimensionless Newman’s reaction penetration depth, Eq. 9

σ _t :

CCL ionic conductivity (Ω ^− 1 cm^− 1)