1 Introduction

Consider the differential equations with several variable deviating arguments of either delayed

$$ x^{\prime}(t)+\sum_{i=1}^{m}p_{i}(t)x \bigl( \tau_{i}(t) \bigr) =0\quad\text{for all }t\geq t_{0}, $$
(E)

or advanced type

figure a

where \(p_{i}\), \(q_{i}\), \(1\leq i\leq m\), are functions of nonnegative real numbers, and \(\tau_{i}\), \(\sigma_{i}\), \(1\leq i\leq m\), are functions of positive real numbers such that

$$ \tau_{i}(t)< t, \quad t\geq t_{0}\quad\text{and}\quad \lim _{t\rightarrow\infty}\tau_{i}(t)=\infty, \quad 1\leq i \leq m, $$
(1.1)

and

figure b

respectively.

In addition, we consider the initial condition for (E)

$$ x(t)=\varphi(t), \quad t\leq t_{0}, $$
(1.2)

where \(\varphi:(-\infty,t_{0}]\rightarrow{\mathbb{R}}\) is a bounded Borel measurable function.

A solution of (E), (1.2) is an absolutely continuous on \([t_{0},\infty)\) function satisfying (E) for almost all \(t\geq t_{0}\) and (1.2) for all \(t\leq t_{0}\). By a solution of (\(\mathrm {E}^{\prime }\)) we mean an absolutely continuous on \([t_{0},\infty)\) function satisfying (\(\mathrm {E}^{\prime }\)) for almost all \(t\geq t_{0}\).

A solution of (E) or (\(\mathrm {E}^{\prime }\)) is oscillatory if it is neither eventually positive nor eventually negative. If there exists an eventually positive or an eventually negative solution, the equation is nonoscillatory. An equation is oscillatory if all its solutions oscillate.

The problem of establishing sufficient conditions for the oscillation of all solutions of equations (E) or (\(\mathrm {E}^{\prime }\)) has been the subject of many investigations. The reader is referred to [1–23] and the references cited therein. Most of these papers concern the special case where the arguments are nondecreasing, while a small number of these papers are concerned with the general case where the arguments are not necessarily monotone. See, for example, [1–4, 12] and the references cited therein.

In the present paper, we establish new oscillation criteria for the oscillation of all solutions of (E) and (\(\mathrm {E}^{\prime }\)) when the arguments are not necessarily monotone. Our results essentially improve several known criteria existing in the literature.

Throughout this paper, we are going to use the following notation:

$$\begin{aligned}& \alpha:=\liminf_{t\rightarrow\infty} \int _{\tau(t)}^{t} \sum_{i=1}^{m}p_{i}(s)\,ds, \end{aligned}$$
(1.3)
$$\begin{aligned}& \beta:=\liminf_{t\rightarrow\infty} \int _{t}^{\sigma(t)} \sum_{i=1}^{m}q_{i}(s)\,ds, \end{aligned}$$
(1.4)
$$\begin{aligned}& D(\omega):= \textstyle\begin{cases} 0, & \text{if }\omega>1/e, \\ \frac{1-\omega-\sqrt{1-2\omega-\omega^{2}}}{2}, & \text{if } \omega\in [ 0,1/e ], \end{cases}\displaystyle \end{aligned}$$
(1.5)
$$\begin{aligned}& \mathit {MD}:=\limsup_{t\rightarrow\infty} \int _{\tau(t)}^{t}\sum_{i=1}^{m}p_{i}(s)\,ds, \end{aligned}$$
(1.6)
$$\begin{aligned}& \mathit {MA}:=\limsup_{t\rightarrow\infty} \int _{t}^{\sigma(t)} \sum_{i=1}^{m}q_{i}(s)\,ds, \end{aligned}$$
(1.7)

where \(\tau(t)=\max_{1\leq i\leq m}\tau_{i}(t)\), \(\sigma(t)= \min_{1\leq i\leq m}\sigma_{i}(t)\) and \(\tau_{i}(t)\), \(\sigma_{i}(t)\) (in (1.6) and (1.7)) are nondecreasing, \(i=1,2,\ldots,m\).

1.1 DDEs

By Remark 2.7.3 in [18], it is clear that if \(\tau_{i}(t)\), \(1\leq i\leq m\), are nondecreasing and

$$ \mathit {MD}>1, $$
(1.8)

then all solutions of (E) are oscillatory. This result is similar to Theorem 2.1.3 [18] which is a special case of Ladas, Lakshmikantham and Papadakis’s result [15].

In 1978 Ladde [17] and in 1982 Ladas and Stavroulakis [16] proved that if

$$ \alpha>\frac{1}{e}, $$
(1.9)

then all solutions of (E) are oscillatory.

In 1984, Hunt and Yorke [8] proved that if \(\tau_{i}(t)\) are nondecreasing, \(t-\tau_{i}(t)\leq\tau_{0}\), \(1\leq i\leq m\), and

$$ \liminf_{t\rightarrow\infty}\sum_{i=1}^{m}p_{i}(t) \bigl( t- \tau_{i}(t) \bigr) >\frac{1}{e}, $$
(1.10)

then all solutions of (E) are oscillatory.

Assume that \(\tau_{i}(t)\), \(1\leq i\leq m\), are not necessarily monotone. Set

$$ h_{i}(t):=\sup_{t_{0}\leq s\leq t}\tau_{i}(s) \quad \text{and}\quad h(t):=\max_{1\leq i\leq m}h_{i}(t), \quad i=1,2, \ldots,m, $$
(1.11)

for \(t\geq t_{0}\), and

$$ \begin{gathered} a_{1}(t,s):=\exp \Biggl\{ \int_{s}^{t}\sum_{i=1}^{m}p_{i}( \zeta)\,d\zeta \Biggr\} , \\ a_{r+1}(t,s):=\exp \Biggl\{ \int_{s}^{t}\sum_{i=1}^{m}p_{i}( \zeta)a_{r}\bigl(\zeta,\tau_{i}(\zeta)\bigr)\,d\zeta \Biggr\} , \quad r \in\mathbb{N} . \end{gathered} $$
(1.12)

Clearly, \(h_{i}(t)\), \(h(t)\) are nondecreasing and \(\tau_{i}(t)\leq h _{i}(t)\leq h(t)< t\) for all \(t\geq t_{0}\).

In 2016, Braverman et al. [1] proved that if, for some \(r\in \mathbb{N}\),

$$ \limsup_{t\rightarrow\infty} \int_{h(t)}^{t}\sum_{i=1}^{m}p _{i}(\zeta)a_{r}\bigl(h(t),\tau_{i}(\zeta) \bigr)\,d\zeta>1, $$
(1.13)

or

$$ \limsup_{t\rightarrow\infty} \int_{h(t)}^{t}\sum_{i=1}^{m}p _{i}(\zeta)a_{r}\bigl(h(t),\tau_{i}(\zeta) \bigr)\,d\zeta>1-D(\alpha), $$
(1.14)

or

$$ \liminf_{t\rightarrow\infty} \int_{h(t)}^{t}\sum_{i=1}^{m}p _{i}(\zeta)a_{r}\bigl(h(t),\tau_{i}(\zeta) \bigr)\,d\zeta>\frac{1}{e}, $$
(1.15)

then all solutions of (E) oscillate.

In 2017, Chatzarakis and Péics [4] proved that if

$$ \limsup_{t\rightarrow\infty} \int_{h(t)}^{t}\sum_{i=1}^{m}p _{i}(\zeta)a_{r}\bigl(h(\zeta),\tau_{i}(\zeta) \bigr)\,d\zeta>\frac{1+\ln \lambda_{0}}{\lambda_{0}}-D(\alpha), $$
(1.16)

where \(\lambda_{0}\) is the smaller root of the transcendental equation \(e^{\alpha\lambda}=\lambda\), then all solutions of (E) are oscillatory.

Very recently, Chatzarakis [3] proved that if, for some \(j\in \mathbb{N}\),

$$ \limsup_{t\rightarrow\infty} \int_{h(t)}^{t}\overline{P}(s)\exp \biggl( \int_{\tau(s)}^{h(t)}\overline{P}_{j}(u)\,du \biggr) \,ds>1 , $$
(1.17)

or

$$ \limsup_{t\rightarrow\infty} \int_{h(t)}^{t}\overline{P}(s)\exp \biggl( \int_{\tau(s)}^{h(t)}\overline{P}_{j}(u)\,du \biggr) \,ds>1-D( \alpha), $$
(1.18)

or

$$ \limsup_{t\rightarrow\infty} \int_{h(t)}^{t}\overline{P}(s)\exp \biggl( \int_{\tau(s)}^{t}\overline{P}_{j}(u)\,du \biggr) \,ds>\frac{1}{D( \alpha)}, $$
(1.19)

or

$$ \limsup_{t\rightarrow\infty} \int_{h(t)}^{t}\overline{P}(s)\exp \biggl( \int_{\tau(s)}^{h(s)}\overline{P}_{j}(u)\,du \biggr) \,ds>\frac{1+ \ln\lambda_{0}}{\lambda_{0}}-D(\alpha), $$
(1.20)

or

$$ \liminf_{t\rightarrow\infty} \int_{h(t)}^{t}\overline{P}(s)\exp \biggl( \int_{\tau(s)}^{h(s)}\overline{P}_{j}(u)\,du \biggr) \,ds> \frac{1}{e}, $$
(1.21)

where

$$ \overline{P}_{j}(t)=\overline{P}(t) \biggl[ 1+ \int_{\tau(t)}^{t} \overline{P}(s)\exp \biggl( \int_{\tau(s)}^{t}\overline{P}(u)\exp \biggl( \int_{\tau(u)}^{u}\overline{P}_{j-1}(\xi)\,d\xi \biggr) \,du \biggr) \,ds \biggr] , $$
(1.22)

with \(\overline{P}_{0}(t)=\overline{P}(t)=\sum_{i=1}^{m}p_{i}(t)\), then all solutions of (E) are oscillatory.

1.2 ADEs

For equation (\(\mathrm {E}^{\prime }\)), the dual condition of (1.8) is

$$ \mathit {MA}>1 $$
(1.23)

(see [18], paragraph 2.7).

In 1978 Ladde [17] and in 1982 Ladas and Stavroulakis [16] proved that if

$$ \beta>\frac{1}{e}, $$
(1.24)

then all solutions of (\(\mathrm {E}^{\prime }\)) are oscillatory.

In 1990, Zhou [23] proved that if \(\sigma_{i}(t)\) are nondecreasing, \(\sigma_{i}(t)-t\leq\sigma_{0}\), \(1\leq i\leq m\), and

$$ \liminf_{t\rightarrow\infty}\sum_{i=1}^{m}q_{i}(t) \bigl( \sigma_{i}(t)-t \bigr) >\frac{1}{e}, $$
(1.25)

then all solutions of (\(\mathrm {E}^{\prime }\)) are oscillatory. (See also [5], Corollary 2.6.12.)

Assume that \(\sigma_{i}(t)\), \(1\leq i\leq m\), are not necessarily monotone. Set

$$ \rho_{i}(t):=\inf_{s\geq t}\sigma_{i}(s), \quad t \geq t_{0} \quad\text{and}\quad\rho(t):=\min_{1\leq i\leq m} \rho_{i}(t), \quad t\geq t_{0} $$
(1.26)

and

$$ \begin{gathered} b_{1}(t,s):=\exp \Biggl\{ \int_{t}^{s}\sum_{i=1}^{m}q_{i}( \zeta)\,d\zeta \Biggr\} , \\ b_{r+1}(t,s):=\exp \Biggl\{ \int_{t}^{s}\sum_{i=1}^{m}q_{i}( \zeta)b_{r}\bigl(t,\sigma_{i}(\zeta)\bigr)\,d\zeta \Biggr\} , \quad r \in\mathbb{N} . \end{gathered} $$
(1.27)

Clearly, \(\rho_{i}(t)\), \(\rho(t)\) are nondecreasing and \(\sigma_{i}(t) \geq\rho_{i}(t)\geq\rho(t)>t\) for all \(t\geq t_{0}\).

In 2016, Braverman et al. [1] proved that if, for some \(r\in \mathbb{N}\),

$$ \limsup_{t\rightarrow\infty} \int_{t}^{\rho(t)}\sum_{i=1} ^{m}q_{i}(\zeta)b_{r}\bigl(\rho(t), \sigma_{i}(\zeta)\bigr) \,d\zeta>1, $$
(1.28)

or

$$ \limsup_{t\rightarrow\infty} \int_{t}^{\rho(t)}\sum_{i=1} ^{m}q_{i}(\zeta)b_{r}\bigl(\rho(t), \sigma_{i}(\zeta)\bigr) \,d\zeta>1-D( \beta), $$
(1.29)

or

$$ \liminf_{t\rightarrow\infty} \int_{t}^{\rho(t)}\sum_{i=1} ^{m}q_{i}(\zeta)b_{r}\bigl(\rho(t), \sigma_{i}(\zeta)\bigr) \,d\zeta> \frac{1}{e}, $$
(1.30)

then all solutions of (\(\mathrm {E}^{\prime }\)) are oscillatory.

Very recently, Chatzarakis [3] proved that if, for some \(j\in \mathbb{N}\),

$$ \limsup_{t\rightarrow\infty} \int_{t}^{\rho(t)}\overline{Q}(s) \exp \biggl( \int_{\rho(t)}^{\sigma(s)}\overline{Q}_{j}(u)\,du \biggr) \,ds>1 , $$
(1.31)

or

$$ \limsup_{t\rightarrow\infty} \int_{t}^{\rho(t)}\overline{Q}(s) \exp \biggl( \int_{\rho(t)}^{\sigma(s)}\overline{Q}_{j}(u)\,du \biggr) \,ds>1-D( \beta), $$
(1.32)

or

$$ \limsup_{t\rightarrow\infty} \int_{t}^{\rho(t)}\overline{Q}(s) \exp \biggl( \int_{t}^{\sigma(s)}\overline{Q}_{j}(u)\,du \biggr) \,ds>\frac{1}{D( \beta)}, $$
(1.33)

or

$$ \limsup_{t\rightarrow\infty} \int_{t}^{\rho(t)}\overline{Q}(s) \exp \biggl( \int_{\rho(s)}^{\sigma(s)}\overline{Q}_{j}(u)\,du \biggr) \,ds>\frac{1+ \ln\lambda_{0}}{\lambda_{0}}-D(\beta), $$
(1.34)

or

$$ \liminf_{t\rightarrow\infty} \int_{t}^{\rho(t)}\overline{Q}(s) \exp \biggl( \int_{\rho(s)}^{\sigma(s)}\overline{Q}_{j}(u)\,du \biggr) \,ds> \frac{1}{e}, $$
(1.35)

where

$$ \overline{Q}_{j}(t)=\overline{Q}(t) \biggl[ 1+ \int_{t}^{\sigma(t)} \overline{Q}(s)\exp \biggl( \int_{t}^{\sigma(s)}\overline{Q}(u)\exp \biggl( \int_{u}^{\sigma(u)}\overline{Q}_{j-1}(\xi)\,d\xi \biggr) \,du \biggr) \,ds \biggr] , $$
(1.36)

with \(\overline{Q}_{0}(t)=\overline{Q}(t)=\sum_{i=1}^{m}q _{i}(t)\), then all solutions of (\(\mathrm {E}^{\prime }\)) are oscillatory.

2 Main results

2.1 DDEs

We further study (E) and derive new sufficient oscillation conditions, involving limsup and liminf, which essentially improve all known results in the literature. For this purpose, we will use the following three lemmas. The proofs of them are similar to the proofs of Lemmas 2.1.1, 2.1.3 and 2.1.2 in [5], respectively.

Lemma 1

Assume that \(h(t)\) is defined by (1.11). Then

$$ \liminf_{t\rightarrow\infty} \int_{\tau(t)}^{t}\sum_{i=1} ^{m}p_{i}(s)\,ds=\liminf_{t\rightarrow\infty} \int_{h(t)}^{t}\sum_{i=1}^{m}p_{i}(s)\,ds. $$
(2.1)

Lemma 2

Assume that x is an eventually positive solution of (E), \(h(t)\) is defined by (1.11) and α by (1.3) with \(0<\alpha\leq1/e\). Then

$$ \liminf_{t\rightarrow\infty}\frac{x(t)}{x(h(t))}\geq D(\alpha) . $$
(2.2)

Lemma 3

Assume that x is an eventually positive solution of (E), \(h(t)\) is defined by (1.11) and α by (1.3) with \(0<\alpha\leq1/e\). Then

$$ \liminf_{t\rightarrow\infty}\frac{x(h(t))}{x(t)}\geq\lambda_{0} , $$
(2.3)

where \(\lambda_{0}\) is the smaller root of the transcendental equation \(\lambda=e^{\alpha\lambda}\).

Based on the above lemmas, we establish the following theorems.

Theorem 1

Assume that \(h(t)\) is defined by (1.11) and, for some \(j\in \mathbb{N}\),

$$ \limsup_{t\rightarrow\infty} \int_{h(t)}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{h(t)}P(u)\exp \biggl( \int_{\tau(u)} ^{u}\overline{R}_{j}(\xi)\,d\xi \biggr) \,du \biggr) \,ds>1, $$
(2.4)

where

$$ \overline{R}_{j}(t)=P(t) \biggl[ 1+ \int_{\tau(t)}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{t}P(u)\exp \biggl( \int_{\tau(u)}^{u} \overline{R}_{j-1}(\xi)\,d\xi \biggr) \,du \biggr) \,ds \biggr] , $$
(2.5)

with \(P(t)=\sum_{i=1}^{m}p_{i}(t)\), \(\overline{R}_{0}(t)=\lambda_{0}P(t)\), and \(\lambda_{0}\) is the smaller root of the transcendental equation \(\lambda=e^{\alpha\lambda}\). Then all solutions of (E) are oscillatory.

Proof

Assume, for the sake of contradiction, that there exists a nonoscillatory solution \(x(t)\) of (E). Since \(-x(t)\) is also a solution of (E), we can confine our discussion only to the case where the solution \(x(t)\) is eventually positive. Then there exists a \(t_{1}>t _{0}\) such that \(x(t)>0\) and \(x ( \tau_{i}(t) ) >0\), \(1\leq i\leq m\), for all \(t\geq t_{1}\). Thus, from (E) we have

$$ x^{\prime}(t)=-\sum_{i=1}^{m}p_{i}(t)x \bigl( \tau_{i}(t) \bigr) \leq0\quad \text{for all }t\geq t_{1}, $$

which means that \(x(t)\) is an eventually nonincreasing function of positive numbers. Taking into account that \(\tau_{i}(t)\leq h(t)\), (E) implies that

$$ x^{\prime}(t)+ \Biggl( \sum_{i=1}^{m}p_{i}(t) \Biggr) x \bigl( h(t) \bigr) \leq x^{\prime}(t)+\sum _{i=1}^{m}p_{i}(t)x \bigl( \tau_{i}(t) \bigr) =0\quad \text{for all }t\geq t_{1}, $$

or

$$ x^{\prime}(t)+P(t)x \bigl( h(t) \bigr) \leq0\quad \text{for all }t \geq t_{1}. $$
(2.6)

Observe that (2.3) implies that, for each \(\epsilon>0\), there exists a \(t_{\epsilon}\) such that

$$ \frac{x(h(t))}{x(t)}>\lambda_{0}-\epsilon\quad\text{for all }t \geq t_{\epsilon}\geq t_{1}. $$
(2.7)

Combining inequalities (2.6) and (2.7), we obtain

$$ x^{\prime}(t)+ ( \lambda_{0}-\epsilon ) P(t)x(t)\leq0 ,\quad t \geq t_{\epsilon}, $$

or

$$ x^{\prime}(t)+\overline{R}_{0}(t,\epsilon)x(t)\leq0 , \quad t \geq t_{\epsilon}, $$
(2.8)

where

$$ \overline{R}_{0}(t,\epsilon)= ( \lambda_{0}-\epsilon ) P(t). $$
(2.9)

Applying the Grönwall inequality in (2.8), we conclude that

$$ x(s)\geq x(t)\exp \biggl( \int_{s}^{t}\overline{R}_{0}(\xi, \epsilon)\,d\xi \biggr) , \quad t\geq s\geq t_{\epsilon}. $$
(2.10)

Now we divide (E) by \(x ( t ) >0\) and integrate on \([ s,t ] \), so

$$\begin{aligned} - \int_{s}^{t}\frac{x^{\prime}(u)}{x(u)}\,du =& \int_{s}^{t}\sum_{i=1}^{m}p_{i}(u) \frac{x ( \tau_{i}(u) ) }{x(u)}\,du \\ \geq& \int_{s}^{t} \Biggl( \sum _{i=1}^{m}p_{i}(u) \Biggr) \frac{x ( \tau(u) ) }{x(u)}\,du \\ =& \int_{s}^{t}P(u)\frac{x ( \tau(u) ) }{x(u)}\,du \end{aligned}$$

or

$$ \ln\frac{x(s)}{x(t)}\geq \int_{s}^{t}P(u)\frac{x ( \tau(u) ) }{x(u)}\,du, \quad t\geq s \geq t_{\epsilon}. $$
(2.11)

Since \(\tau(u)< u\), setting \(u=t\), \(s=\tau ( u ) \) in (2.10), we take

$$ x \bigl( \tau(u) \bigr) \geq x(u)\exp \biggl( \int_{\tau(u)}^{u} \overline{R}_{0}(\xi, \epsilon)\,d\xi \biggr) . $$
(2.12)

Combining (2.11) and (2.12), we obtain, for sufficiently large t,

$$ \ln\frac{x(s)}{x(t)}\geq \int_{s}^{t}P(u)\exp \biggl( \int_{\tau(u)} ^{u}\overline{R}_{0}(\xi, \epsilon)\,d\xi \biggr) \,du $$

or

$$ x(s)\geq x(t)\exp \biggl( \int_{s}^{t}P(u)\exp \biggl( \int_{\tau(u)} ^{u}\overline{R}_{0}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) . $$
(2.13)

Hence,

$$ x\bigl(\tau ( s ) \bigr)\geq x(t)\exp \biggl( \int_{\tau ( s ) }^{t}P(u)\exp \biggl( \int_{\tau(u)}^{u} \overline{R}_{0}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) . $$
(2.14)

Integrating (E) from \(\tau(t)\) to t, we have

$$ x(t)-x\bigl(\tau(t)\bigr)+ \int_{\tau(t)}^{t}\sum_{i=1}^{m}p_{i}(s)x \bigl( \tau_{i}(s) \bigr) \,ds=0, $$

or

$$ x(t)-x\bigl(\tau(t)\bigr)+ \int_{\tau(t)}^{t} \Biggl( \sum _{i=1}^{m}p_{i}(s) \Biggr) x \bigl( \tau(s) \bigr) \,ds\leq0 , $$

i.e.,

$$ x(t)-x\bigl(\tau(t)\bigr)+ \int_{\tau(t)}^{t}P(s)x \bigl( \tau(s) \bigr) \,ds \leq0. $$
(2.15)

It follows from (2.14) and (2.15) that

$$ x(t)-x\bigl(\tau(t)\bigr)+x(t) \int_{\tau(t)}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{t}P(u)\exp \biggl( \int_{\tau(u)}^{u} \overline{R}_{0}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds\leq0 . $$

Multiplying the last inequality by \(P(t)\), we find

$$\begin{aligned}& P(t)x(t)-P(t)x\bigl(\tau(t)\bigr) \\& \quad{} +P(t)x(t) \int_{\tau(t)}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{t}P(u)\exp \biggl( \int_{\tau(u)}^{u} \overline{R}_{0}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds\leq0 . \end{aligned}$$
(2.16)

Furthermore,

$$ x^{\prime}(t)=-\sum_{i=1}^{m}p_{i}(t)x \bigl( \tau_{i}(t) \bigr) \leq-x \bigl( \tau(t) \bigr) \sum _{i=1}^{m}p_{i}(t)=-P(t)x \bigl( \tau(t) \bigr) . $$
(2.17)

Combining inequalities (2.16) and (2.17), we have

$$ \begin{aligned} &x^{\prime}(t) +P(t)x(t) \\ &\quad{} +P(t)x(t) \int_{\tau(t)}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{t}P(u)\exp \biggl( \int_{\tau(u)}^{u} \overline{R}_{0}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds\leq0 . \end{aligned} $$

Hence,

$$ x^{\prime}(t) +P(t) \biggl[ 1+ \int_{\tau(t)}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{t}P(u)\exp \biggl( \int_{\tau(u)}^{u} \overline{R}_{0}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds \biggr] x(t) \leq0, $$

or

$$ x^{\prime}(t)+\overline{R}_{1}(t,\epsilon)x(t)\leq0, $$
(2.18)

where

$$ \overline{R}_{1}(t,\epsilon)=P(t) \biggl[ 1+ \int_{\tau(t)}^{t}P(s) \exp \biggl( \int_{\tau ( s ) }^{t}P(u)\exp \biggl( \int_{\tau(u)}^{u}\overline{R}_{0}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds \biggr] . $$

Clearly, (2.18) resembles (2.8) with \(\overline{R}_{0}\) replaced by \(\overline{R}_{1}\), so an integration of (2.18) on \([ s,t ] \) leads to

$$ x(s)\geq x(t)\exp \biggl( \int_{s}^{t}\overline{R}_{1}(\xi, \epsilon)\,d\xi \biggr) . $$
(2.19)

Taking the steps starting from (2.8) to (2.14), we may see that x satisfies the inequality

$$ x \bigl( \tau(u) \bigr) \geq x(u)\exp \biggl( \int_{\tau(u)}^{u} \overline{R}_{1}(\xi, \epsilon)\,d\xi \biggr) . $$
(2.20)

Combining now (2.11) and (2.20), we obtain

$$ x(s)\geq x(t)\exp \biggl( \int_{s}^{t}P(u)\exp \biggl( \int_{\tau(u)} ^{u}\overline{R}_{1}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) , $$

from which we take

$$ x\bigl(\tau(s)\bigr)\geq x(t)\exp \biggl( \int_{\tau(s)}^{t}P(u)\exp \biggl( \int_{\tau(u)}^{u}\overline{R}_{1}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) . $$
(2.21)

By (2.15) and (2.21) we have

$$ x(t)-x\bigl(\tau(t)\bigr)+x(t) \int_{\tau(t)}^{t}P(s)\exp \biggl( \int_{\tau(s)} ^{t}P(u)\exp \biggl( \int_{\tau(u)}^{u}\overline{R}_{1}(\xi, \epsilon )\,d\xi \biggr) \,du \biggr) \,ds\leq0. $$

Multiplying the last inequality by \(P(t)\), as before, we find

$$ x^{\prime}(t)+P(t) \biggl[ 1+ \int_{\tau(t)}^{t}P(s)\exp \biggl( \int_{\tau(s)}^{t}P(u)\exp \biggl( \int_{\tau(u)}^{u}\overline{R} _{1}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds \biggr] x(t)\leq0 . $$

Therefore, for sufficiently large t,

$$ x^{\prime}(t)+\overline{R}_{2}(t,\epsilon)x(t)\leq0, $$
(2.22)

where

$$ \overline{R}_{2}(t,\epsilon)=P(t) \biggl[ 1+ \int_{\tau(t)}^{t}P(s) \exp \biggl( \int_{\tau(s)}^{t}P(u)\exp \biggl( \int_{\tau(u)}^{u} \overline{R}_{1}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds \biggr] . $$

Repeating the above procedure, it follows by induction that for sufficiently large t

$$ x^{\prime}(t)+\overline{R}_{j}(t,\epsilon)x(t)\leq0, \quad j \in\mathbb{N} , $$

where

$$ \overline{R}_{j}(t)=P(t) \biggl[ 1+ \int_{\tau(t)}^{t}P(s)\exp \biggl( \int_{\tau(s)}^{t}P(u)\exp \biggl( \int_{\tau(u)}^{u}\overline{R} _{j-1}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds \biggr] . $$

Moreover, since \(\tau ( s ) \leq h ( s ) \leq h ( t ) \), we have

$$ x\bigl(\tau(s)\bigr)\geq x\bigl(h(t)\bigr)\exp \biggl( \int_{\tau(s)}^{h(t)}P(u)\exp \biggl( \int_{\tau(u)}^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) . $$
(2.23)

Integrating (E) from \(h(t)\) to t and using (2.23), we obtain

$$\begin{aligned} 0 =&x(t)-x\bigl(h(t)\bigr)+ \int_{h(t)}^{t}\sum_{i=1}^{m}p_{i}(s)x \bigl( \tau_{i}(s) \bigr) \,ds \\ \geq&x(t)-x\bigl(h(t)\bigr)+ \int_{h(t)}^{t} \Biggl( \sum _{i=1}^{m}p_{i}(s) \Biggr) x \bigl( \tau(s) \bigr) \,ds \\ =&x(t)-x\bigl(h(t)\bigr)+ \int_{h(t)}^{t}P(s)x \bigl( \tau(s) \bigr) \,ds \\ \geq&x(t)-x\bigl(h(t)\bigr)+x \bigl( h ( t ) \bigr) \int_{h(t)}^{t}P(s) \exp \biggl( \int_{\tau(s)}^{h(t)}P(u)\exp \biggl( \int_{\tau(u)}^{u} \overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds, \end{aligned}$$

i.e.,

$$ \begin{aligned}[b] &x(t)-x\bigl(h(t)\bigr) \\ &\quad{} +x\bigl(h(t)\bigr) \int_{h(t)}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{h(t)}P(u)\exp \biggl( \int_{\tau(u)} ^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds\leq0 . \end{aligned} $$
(2.24)

The strict inequality is valid if we omit \(x(t)>0\) on the left-hand side. Therefore,

$$ x\bigl(h(t)\bigr) \biggl[ \int_{h(t)}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{h(t)}P(u)\exp \biggl( \int_{\tau(u)} ^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds-1 \biggr] < 0, $$

or

$$ \int_{h(t)}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{h(t)}P(u) \exp \biggl( \int_{\tau(u)}^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds-1< 0. $$

Taking the limit as \(t\rightarrow\infty\), we have

$$ \limsup_{t\rightarrow\infty} \int_{h(t)}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{h(t)}P(u)\exp \biggl( \int_{\tau(u)} ^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds\leq1 . $$

Since ϵ may be taken arbitrarily small, this inequality contradicts (2.4).

The proof of the theorem is complete. □

Theorem 2

Assume that α is defined by (1.3) with \(0<\alpha\leq 1/e\) and \(h(t)\) by (1.11). If for some \(j\in\mathbb{N} \)

$$ \limsup_{t\rightarrow\infty} \int_{h(t)}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{h(t)}P(u)\exp \biggl( \int_{\tau(u)} ^{u}\overline{R}_{j}(\xi)\,d\xi \biggr) \,du \biggr) \,ds>1-D(\alpha) , $$
(2.25)

where \(\overline{R}_{j}\) is defined by (2.5), then all solutions of (E) are oscillatory.

Proof

Let x be an eventually positive solution of (E). Then, as in the proof of Theorem 1, (2.24) is satisfied, i.e.,

$$ x(t)-x\bigl(h(t)\bigr)+x\bigl(h(t)\bigr) \int_{h(t)}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{h(t)}P(u)\exp \biggl( \int_{\tau(u)} ^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds\leq0 . $$

That is,

$$ \int_{h(t)}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{h(t)}P(u) \exp \biggl( \int_{\tau(u)}^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds\leq1-\frac{x(t)}{x(h(t))}, $$

which gives

$$ \begin{aligned}[b] &\limsup_{t\rightarrow\infty} \int_{h(t)}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{h(t)}P(u)\exp \biggl( \int_{\tau(u)} ^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds \\ &\quad \leq 1 - \liminf_{t\rightarrow\infty} \frac{x(t)}{x(h(t))}. \end{aligned} $$
(2.26)

By combining Lemmas 1 and 2, it becomes obvious that inequality (2.2) is fulfilled. So, (2.26) leads to

$$ \limsup_{t\rightarrow\infty} \int_{h(t)}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{h(t)}P(u)\exp \biggl( \int_{\tau(u)} ^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds\leq1-D( \alpha). $$

Since ϵ may be taken arbitrarily small, this inequality contradicts (2.25).

The proof of the theorem is complete. □

Remark 1

It is clear that the left-hand sides of both conditions (2.4) and (2.25) are identical, also the right-hand side of condition (2.25) reduces to (2.4) in case that \(\alpha=0\). So it seems that Theorem 2 is the same as Theorem 1 when \(\alpha =0\). However, one may notice that the condition \(0<\alpha\leq1/e\) is required in Theorem 2 but not in Theorem 1.

Theorem 3

Assume that α is defined by (1.3) with \(0<\alpha\leq 1/e\) and \(h(t)\) by (1.11). If for some \(j\in\mathbb{N} \)

$$ \limsup_{t\rightarrow\infty} \int_{h(t)}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{t}P(u)\exp \biggl( \int_{\tau(u)}^{u} \overline{R}_{j}(\xi)\,d\xi \biggr) \,du \biggr) \,ds>\frac{1}{D(\alpha)}-1 , $$
(2.27)

where \(\overline{R}_{j}\) is defined by (2.5), then all solutions of (E) are oscillatory.

Proof

Assume, for the sake of contradiction, that there exists a nonoscillatory solution x of (E) and that x is eventually positive. Then, as in the proof of Theorem 1, (2.23) is satisfied, which yields

$$ x\bigl(\tau(s)\bigr)\geq x(t)\exp \biggl( \int_{\tau(s)}^{t}P(u)\exp \biggl( \int_{\tau(u)}^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) . $$

Integrating (E) from \(h(t)\) to t, we have

$$ x(t)-x\bigl(h(t)\bigr)+ \int_{h(t)}^{t}\sum_{i=1}^{m}p_{i}(s)x \bigl(\tau_{i}(s)\bigr)\,ds=0 , $$

or

$$ x(t)-x\bigl(h(t)\bigr)+ \int_{h(t)}^{t} \Biggl( \sum _{i=1}^{m}p_{i}(s) \Biggr) x\bigl(\tau(s) \bigr)\,ds\leq0. $$

Thus

$$ x(t)-x\bigl(h(t)\bigr)+ \int_{h(t)}^{t}P(s)x\bigl(\tau(s)\bigr)\,ds\leq0. $$

By virtue of (2.23), the last inequality gives

$$ x(t)-x\bigl(h(t)\bigr)+ \int_{h(t)}^{t}P(s)x(t)\exp \biggl( \int_{\tau(s)}^{t}P(u) \exp \biggl( \int_{\tau(u)}^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds\leq0, $$

or

$$ x(t)-x\bigl(h(t)\bigr)+x(t) \int_{h(t)}^{t}P(s)\exp \biggl( \int_{\tau(s)}^{t}P(u) \exp \biggl( \int_{\tau(u)}^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds\leq0. $$

Thus, for all sufficiently large t, it holds

$$ \int_{h(t)}^{t}P(s)\exp \biggl( \int_{\tau(s)}^{t}P(u)\exp \biggl( \int_{\tau(u)}^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds\leq\frac{x(h(t))}{x(t)}-1. $$

Letting \(t\rightarrow\infty\), we take

$$ \limsup_{t\rightarrow\infty} \int_{h(t)}^{t}P(s)\exp \biggl( \int_{\tau(s)}^{t}P(u)\exp \biggl( \int_{\tau(u)}^{u}\overline{R} _{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds\leq \limsup_{t\rightarrow\infty} \frac{x(h(t))}{x(t)}-1, $$

which, in view of (2.2), gives

$$ \limsup_{t\rightarrow\infty} \int_{h(t)}^{t}P(s)\exp \biggl( \int_{\tau(s)}^{t}P(u)\exp \biggl( \int_{\tau(u)}^{u}\overline{R} _{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds\leq \frac{1}{D(\alpha)}-1. $$

Since ϵ may be taken arbitrarily small, this inequality contradicts (2.27).

The proof of the theorem is complete. □

Theorem 4

Assume that α is defined by (1.3) with \(0<\alpha\leq 1/e\) and \(h(t)\) by (1.11). If for some \(j\in\mathbb{N} \)

$$ \limsup_{t\rightarrow\infty} \int_{h(t)}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{h(s)}P(u)\exp \biggl( \int_{\tau(u)} ^{u}\overline{R}_{j}(\xi)\,d\xi \biggr) \,du \biggr) \,ds>\frac{1+\ln \lambda_{0}}{\lambda_{0}}-D(\alpha), $$
(2.28)

where \(\overline{R}_{j}\) is defined by (2.5) and \(\lambda_{0}\) is the smaller root of the transcendental equation \(\lambda=e^{\alpha \lambda}\), then all solutions of (E) are oscillatory.

Proof

Assume, for the sake of contradiction, that there exists a nonoscillatory solution x of (E) and that x is eventually positive. Then, as in Theorem 1, (2.23) holds.

Observe that (2.3) implies that, for each \(\epsilon>0\), there exists a \(t_{\epsilon}\) such that

$$ \lambda_{0}-\epsilon< \frac{x(h(t))}{x(t)}\quad\text{for all }t \geq t_{\epsilon}. $$
(2.29)

Noting that by nonincreasingness of the function \(x(h(t))/x(s)\) in s it holds

$$ 1=\frac{x(h(t))}{x(h(t))}\leq\frac{x(h(t))}{x(s)}\leq \frac{x(h(t))}{x(t)}, \quad t_{\epsilon}\leq h(t)\leq s \leq t, $$

in particular for \(\epsilon\in ( 0,\lambda_{0}-1 ) \), by continuity we see that there exists a \(t^{\ast}\in(h(t),t]\) such that

$$ 1< \lambda_{0}-\epsilon=\frac{x(h(t))}{x(t^{\ast})}. $$
(2.30)

By (2.23), it is obvious that

$$ x\bigl(\tau(s)\bigr)\geq x\bigl(h(s)\bigr)\exp \biggl( \int_{\tau(s)}^{h(s)}P(u)\exp \biggl( \int_{\tau(u)}^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) . $$
(2.31)

Integrating (E) from \(t^{\ast}\) to t, we have

$$ x(t)-x\bigl(t^{\ast}\bigr)+ \int_{t^{\ast}}^{t}\sum_{i=1}^{m}p_{i}(s)x \bigl( \tau_{i}(s)\bigr)\,ds=0, $$

or

$$ x(t)-x\bigl(t^{\ast}\bigr)+ \int_{t^{\ast}}^{t} \Biggl( \sum _{i=1}^{m}p _{i}(s) \Biggr) x\bigl(\tau(s) \bigr)\,ds\leq0, $$

i.e.,

$$ x(t)-x\bigl(t^{\ast}\bigr)+ \int_{t^{\ast}}^{t}P(s)x\bigl(\tau(s)\bigr)\,ds\leq0. $$

By using (2.31) along with \(h(s)\leq h(t)\) in combination with the nonincreasingness of x, we have

$$ x(t)-x\bigl(t^{\ast}\bigr)+x\bigl(h(t)\bigr) \int_{t^{\ast}}^{t}P(s)\exp \biggl( \int_{\tau(s)}^{h(s)}P(u)\exp \biggl( \int_{\tau(u)}^{u}\overline{R} _{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds\leq0, $$

or

$$ \int_{t^{\ast}}^{t}P(s)\exp \biggl( \int_{\tau(s)}^{h(s)}P(u)\exp \biggl( \int_{\tau(u)}^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds \leq\frac{x(t^{\ast})}{x(h(t))}-\frac{x(t)}{x(h(t))} . $$

In view of (2.30) and Lemma 2, for the ϵ considered, there exists a \(t_{\epsilon}^{\prime}\geq t_{\epsilon}\) such that

$$ \int_{t^{\ast}}^{t}P(s)\exp \biggl( \int_{\tau(s)}^{h(s)}P(u)\exp \biggl( \int_{\tau(u)}^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds< \frac{1}{\lambda_{0}-\epsilon}-D(\alpha)+\epsilon $$
(2.32)

for \(t\geq t_{\epsilon}^{\prime}\).

Dividing (E) by \(x(t)\) and integrating from \(h(t)\) to \(t^{\ast }\), we find

$$ \int_{h(t)}^{t^{\ast}}\sum_{i=1}^{m}p_{i}(s) \frac{x(\tau_{i} ( s ) )}{x(s)}\,ds=- \int_{h(t)}^{t^{\ast}} \frac{x^{\prime}(s)}{x(s)}\,ds, $$

or

$$ \int_{h(t)}^{t^{\ast}} \Biggl( \sum _{i=1}^{m}p_{i}(s) \Biggr) \frac{x( \tau ( s ) )}{x(s)}\,ds\leq- \int_{h(t)}^{t^{\ast}}\frac{x ^{\prime}(s)}{x(s)}\,ds, $$

i.e.,

$$ \int_{h(t)}^{t^{\ast}}P(s)\frac{x(\tau ( s ) )}{x(s)}\,ds \leq- \int_{h(t)}^{t^{\ast}}\frac{x^{\prime}(s)}{x(s)}\,ds, $$

and using (2.31), we find

$$ \int_{h(t)}^{t^{\ast}}P(s)\frac{x(h(s))}{x(s)}\exp \biggl( \int_{ \tau(s)}^{h(s)}P(u)\exp \biggl( \int_{\tau(u)}^{u}\overline{R}_{j}( \xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds\leq- \int_{h(t)}^{t^{\ast }}\frac{x ^{\prime}(s)}{x(s)}\,ds. $$
(2.33)

By (2.29), for \(s\geq h(t)\geq t_{\epsilon}^{\prime}\), we have \(x(h(s))/x(s)>\lambda_{0}-\epsilon\), so from (2.33) we get

$$ (\lambda_{0}-\epsilon) \int_{h(t)}^{t^{\ast}}P(s)\exp \biggl( \int_{\tau(s)}^{h(s)}P(u)\exp \biggl( \int_{\tau(u)}^{u}\overline{R} _{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds< - \int_{h(t)}^{t^{\ast }}\frac{x ^{\prime}(s)}{x(s)}\,ds. $$

Hence, for all sufficiently large t, we have

$$ \begin{gathered} \int_{h(t)}^{t^{\ast}}P(s)\exp \biggl( \int_{\tau(s)}^{h(s)}P(u) \exp \biggl( \int_{\tau(u)}^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds \\ \quad < -\frac{1}{\lambda_{0}-\epsilon} \int_{h(t)}^{t^{\ast}}\frac{x^{ \prime}(s)}{x(s)}\,ds=\frac{1}{\lambda_{0}-\epsilon} \ln\frac{x(h(t))}{x(t ^{\ast})}=\frac{\ln ( \lambda_{0}-\epsilon ) }{\lambda _{0}-\epsilon}, \end{gathered} $$

i.e.,

$$ \int_{h(t)}^{t^{\ast}}P(s)\exp \biggl( \int_{\tau(s)}^{h(s)}P(u) \exp \biggl( \int_{\tau(u)}^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds< \frac{\ln ( \lambda_{0}-\epsilon ) }{\lambda_{0}-\epsilon}. $$
(2.34)

Adding (2.32) and (2.34), and then taking the limit as \(t\rightarrow \infty\), we have

$$\begin{aligned}& \limsup_{t\rightarrow\infty} \int_{h(t)}^{t}P(s)\exp \biggl( \int_{\tau(s)}^{h(s)}P(u)\exp \biggl( \int_{\tau(u)}^{u}\overline{R} _{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds \\& \quad \leq\frac{1+\ln(\lambda_{0}-\epsilon)}{\lambda_{0}-\epsilon}-D( \alpha)+\epsilon. \end{aligned}$$

Since ϵ may be taken arbitrarily small, this inequality contradicts (2.28).

The proof of the theorem is complete. □

Theorem 5

Assume that \(h(t)\) is defined by (1.11) and for some \(j\in \mathbb{N} \)

$$ \liminf_{t\rightarrow\infty} \int_{h(t)}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{h(s)}P(u)\exp \biggl( \int_{\tau(u)} ^{u}\overline{R}_{j}(\xi)\,d\xi \biggr) \,du \biggr) \,ds>\frac{1}{e} , $$
(2.35)

where \(\overline{R}_{j}\) is defined by (2.5). Then all solutions of (E) are oscillatory.

Proof

Assume, for the sake of contradiction, that there exists a nonoscillatory solution \(x(t)\) of (E). Since \(-x(t)\) is also a solution of (E), we can confine our discussion only to the case where the solution \(x(t)\) is eventually positive. Then there exists a \(t_{1}>t _{0}\) such that \(x(t)>0\) and \(x ( \tau_{i}(t) ) >0\), \(1\leq i\leq m\) for all \(t\geq t_{1}\). Thus, from (E) we have

$$ x^{\prime}(t)=-\sum_{i=1}^{m}p_{i}(t)x \bigl( \tau_{i}(t) \bigr) \leq0\quad \text{for all }t\geq t_{1}, $$

which means that \(x(t)\) is an eventually nonincreasing function of positive numbers. Furthermore, as in previous theorem, (2.31) is satisfied.

Dividing (E) by \(x(t)\) and integrating from \(h(t)\) to t, for some \(t_{2}\geq t_{1}\), we get

$$ \begin{aligned}[b] \ln \biggl( \frac{x(h(t))}{x(t)} \biggr) ={} & \int _{h(t)}^{t} \sum_{i=1}^{m}p_{i}(s) \frac{x ( \tau_{i}(s) ) }{x ( s ) }\,ds \\ \geq{} & \int _{h(t)}^{t} \Biggl( \sum _{i=1}^{m}p_{i}(s) \Biggr) \frac{x ( \tau(s) ) }{x ( s ) }\,ds \\ = {}& \int _{h(t)}^{t}P(s)\frac{x ( \tau(s) ) }{x ( s ) }\,ds. \end{aligned} $$
(2.36)

Combining inequalities (2.31) and (2.36), we obtain

$$ \ln \biggl( \frac{x(h(t))}{x(t)} \biggr) \geq \int_{h(t)}^{t}P(s)\frac{x(h(s))}{x ( s ) }\exp \biggl( \int_{\tau(s)}^{h(s)}P(u)\exp \biggl( \int_{\tau(u)}^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds. $$

Taking into account that x is nonincreasing and \(h(s)< s\), the last inequality becomes

$$ \ln \biggl( \frac{x(h(t))}{x(t)} \biggr) \geq \int_{h(t)}^{t}P(s)\exp \biggl( \int_{\tau(s)}^{h(s)}P(u)\exp \biggl( \int_{\tau(u)}^{u} \overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds. $$
(2.37)

From (2.35), it follows that there exists a constant \(c>0\) such that for sufficiently large t

$$ \int_{h(t)}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{h(s)}P(u) \exp \biggl( \int_{\tau(u)}^{u}\overline{R}_{j}(\xi)\,d\xi \biggr) \,du \biggr) \,ds\geq c>\frac{1}{e}. $$

Choose \(c^{\prime}\) such that \(c>c^{\prime}>1/e\). For every \(\epsilon>0\) such that \(c-\epsilon>c^{\prime}\), we have

$$ \int_{h(t)}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{h(s)}P(u) \exp \biggl( \int_{\tau(u)}^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds>c-\epsilon>c^{\prime}>\frac{1}{e}. $$
(2.38)

Combining inequalities (2.37) and (2.38), we obtain

$$ \ln \biggl( \frac{x(h(t))}{x(t)} \biggr) \geq c^{\prime},\quad t\geq t _{3}. $$

Thus

$$ \frac{x(h(t))}{x(t)}\geq e^{c^{\prime}}\geq ec^{\prime}>1, $$

which yields, for some \(t\geq t_{4}\geq t_{3}\),

$$ x\bigl(h(t)\bigr)\geq\bigl(ec^{\prime}\bigr)x(t). $$

Repeating the above procedure, it follows by induction that for any positive integer k,

$$ \frac{x(h(t))}{x(t)}\geq\bigl(ec^{\prime}\bigr)^{k}\quad \text{for sufficiently large }t. $$

Since \(ec^{\prime}>1\), there is a \(k\in{\mathbb{N}}\) satisfying \(k>2(\ln(2)-\ln(c^{\prime}))/(1+\ln(c^{\prime}))\) such that for t sufficiently large

$$ \frac{x(h(t))}{x(t)}\geq\bigl(ec^{\prime}\bigr)^{k}> \biggl( \frac{2}{c^{\prime }} \biggr) ^{2}. $$
(2.39)

Next we split the integral in (2.38) into two integrals, each integral being no less than \(c^{\prime}/2\):

$$ \begin{aligned} \int_{h(t)}^{t_{m}}P(s)\exp \biggl( \int_{\tau ( s ) }^{h(s)}P(u) \exp \biggl( \int_{\tau(u)}^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds\geq\frac{c^{\prime}}{2}, \\ \int_{t_{m}}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{h(s)}P(u) \exp \biggl( \int_{\tau(u)}^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds\geq\frac{c^{\prime}}{2}. \end{aligned} $$
(2.40)

Integrating (E) from \(t_{m}\) to t, we deduce that

$$ x(t)-x(t_{m})+ \int_{t_{m}}^{t}\sum_{i=1}^{m}p_{i}(s)x \bigl(\tau_{i}(s)\bigr)\,ds=0 , $$

or

$$ x(t)-x(t_{m})+ \int_{t_{m}}^{t} \Biggl( \sum _{i=1}^{m}p_{i}(s) \Biggr) x\bigl(\tau(s) \bigr)\,ds\leq0. $$

Thus

$$ x(t)-x(t_{m})+ \int_{t_{m}}^{t}P(s)x\bigl(\tau(s)\bigr)\,ds\leq0, $$

which, in view of (2.31), gives

$$ x(t)-x(t_{m})+x\bigl(h(t)\bigr) \int_{t_{m}}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{h(s)}P(u)\exp \biggl( \int_{\tau(u)} ^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds\leq0. $$

The strict inequality is valid if we omit \(x(t)>0\) on the left-hand side:

$$ -x(t_{m})+x\bigl(h(t)\bigr) \int_{t_{m}}^{t}P(s)\exp \biggl( \int_{\tau ( s ) }^{h(s)}P(u)\exp \biggl( \int_{\tau(u)} ^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds< 0 . $$

Using the second inequality in (2.40), we conclude that

$$ x(t_{m})>\frac{c^{\prime}}{2}x\bigl(h(t)\bigr). $$
(2.41)

Similarly, integration of (E) from \(h(t)\) to \(t_{m}\) with a later application of (2.31) leads to

$$ x(t_{m})-x\bigl(h(t)\bigr)+x\bigl(h(t_{m})\bigr) \int_{h(t)}^{t_{m}}P(s)\exp \biggl( \int_{\tau ( s ) }^{h(s)}P(u)\exp \biggl( \int_{\tau(u)} ^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds\leq0. $$

The strict inequality is valid if we omit \(x(t_{m})>0\) on the left-hand side:

$$ -x\bigl(h(t)\bigr)+x\bigl(h(t_{m})\bigr) \int_{h(t)}^{t_{m}}\exp \biggl( \int_{\tau ( s ) }^{h(s)}P(u)\exp \biggl( \int_{\tau(u)} ^{u}\overline{R}_{j}(\xi, \epsilon)\,d\xi \biggr) \,du \biggr) \,ds< 0. $$

Using the first inequality in (2.40) implies that

$$ x\bigl(h(t)\bigr)>\frac{c^{\prime}}{2}x\bigl(h(t_{m})\bigr). $$
(2.42)

Combining inequalities (2.41) and (2.42), we obtain

$$ x\bigl(h(t_{m})\bigr)< \frac{2}{c^{\prime}}x\bigl(h(t)\bigr)< \biggl( \frac{2}{c^{\prime}} \biggr) ^{2}x(t_{m}), $$

which contradicts (2.39).

The proof of the theorem is complete. □

2.2 ADEs

Similar oscillation conditions for the (dual) advanced differential equation (\(\mathrm {E}^{\prime }\)) can be derived easily. The proofs are omitted since they are quite similar to the delay equation.

Theorem 6

Assume that \(\rho(t)\) is defined by (1.26), and for some \(j\in \mathbb{N} \)

$$ \limsup_{t\rightarrow\infty} \int_{t}^{\rho(t)}Q(s)\exp \biggl( \int_{\rho(t)}^{\sigma(s)}Q(u)\exp \biggl( \int_{u}^{\sigma(u)} \overline{L}_{j}(\xi)\,d\xi \biggr) \,du \biggr) \,ds>1, $$
(2.43)

where

$$ \overline{L}_{j}(t)=Q(t) \biggl[ 1+ \int_{t}^{\sigma(t)}Q(s)\exp \biggl( \int_{t}^{\sigma(s)}Q(u)\exp \biggl( \int_{u}^{\sigma(u)} \overline{L}_{j-1}(\xi)\,d\xi \biggr) \,du \biggr) \,ds \biggr] , $$
(2.44)

with \(Q(t)=\sum_{i=1}^{m}q_{i}(t)\), \(\overline{L}_{0}(t)=\lambda_{0}Q(t)\) and \(\lambda_{0}\) is the smaller root of the transcendental equation \(\lambda=e^{\beta\lambda}\). Then all solutions of (\(\mathrm {E}^{\prime }\)) are oscillatory.

Theorem 7

Assume that β is defined by (1.4) with \(0<\beta\leq 1/e\) and \(\rho(t)\) by (1.26). If for some \(j\in\mathbb{N} \)

$$ \limsup_{t\rightarrow\infty} \int_{t}^{\rho(t)}Q(s)\exp \biggl( \int_{\rho(t)}^{\sigma(s)}Q(u)\exp \biggl( \int_{u}^{\sigma(u)} \overline{L}_{j}(\xi)\,d\xi \biggr) \,du \biggr) \,ds>1-D(\beta), $$
(2.45)

where \(\overline{L}_{j}\) is defined by (2.44), then all solutions of (\(\mathrm {E}^{\prime }\)) are oscillatory.

Remark 2

It is clear that the left-hand sides of both conditions (2.43) and (2.45) are identical, also the right-hand side of condition (2.45) reduces to (2.43) in case that \(\beta=0\). So it seems that Theorem 7 is the same as Theorem 6 when \(\beta =0\). However, one may notice that the condition \(0<\beta\leq1/e\) is required in Theorem 7 but not in Theorem 6.

Theorem 8

Assume that β is defined by (1.4) with \(0<\beta\leq 1/e\) and \(\rho(t)\) by (1.26). If for some \(j\in\mathbb{N} \)

$$ \limsup_{t\rightarrow\infty} \int_{t}^{\rho(t)}Q(s)\exp \biggl( \int _{t}^{\sigma(s)}Q(u)\exp \biggl( \int_{u}^{\sigma(u)}\overline{L} _{j}(\xi)\,d\xi \biggr) \,du \biggr) \,ds>\frac{1}{D(\beta)}-1, $$
(2.46)

where \(\overline{L}_{j}\) is defined by (2.44), then all solutions of (\(\mathrm {E}^{\prime }\)) are oscillatory.

Theorem 9

Assume that β is defined by (1.4) with \(0<\beta\leq 1/e\) and \(\rho(t)\) by (1.26). If for some \(j\in\mathbb{N} \)

$$ \begin{aligned}[b] & \limsup_{t\rightarrow\infty} \int_{t}^{\rho(t)}Q(s)\exp \biggl( \int_{\rho(s)}^{\sigma(s)}Q(u)\exp \biggl( \int_{u}^{\sigma(u)} \overline{L}_{j}(\xi)\,d\xi \biggr) \,du \biggr) \,ds \\ &\quad >\frac{1+\ln\lambda _{0}}{\lambda_{0}}-D(\beta), \end{aligned} $$
(2.47)

where \(\overline{L}_{j}\) is defined by (2.44) and \(\lambda_{0}\) is the smaller root of the transcendental equation \(\lambda=e^{\beta\lambda }\), then all solutions of (\(\mathrm {E}^{\prime }\)) are oscillatory.

Theorem 10

Assume that \(\rho(t)\) is defined by (1.26) and for some \(j\in \mathbb{N} \)

$$ \liminf_{t\rightarrow\infty} \int_{t}^{\rho(t)}Q(s)\exp \biggl( \int_{\rho(s)}^{\sigma(s)}Q(u)\exp \biggl( \int_{u}^{\sigma(u)} \overline{L}_{j}(\xi)\,d\xi \biggr) \,du \biggr) \,ds>\frac{1}{e}, $$
(2.48)

where \(\overline{Q}_{j}\) is defined by (2.44). Then all solutions of (\(\mathrm {E}^{\prime }\)) are oscillatory.

2.3 Differential inequalities

A slight modification in the proofs of Theorems 1-10 leads to the following results about differential inequalities.

Theorem 11

Assume that all the conditions of Theorem  1 [6] or 2 [7] or 3 [8] or 4 [9] or 5 [10] hold. Then

  1. (i)

    the delay [advanced] differential inequality

    $$ x^{\prime}(t)+\sum_{i=1}^{m}p_{i}(t)x \bigl( \tau_{i}(t) \bigr) \leq0 \quad\quad \Biggl[ x^{\prime}(t)-\sum _{i=1}^{m}q_{i}(t)x \bigl( \sigma_{i}(t) \bigr) \geq0 \Biggr] , \quad \forall t\geq t _{0}, $$

    has no eventually positive solutions;

  2. (ii)

    the delay [advanced] differential inequality

    $$ x^{\prime}(t)+\sum_{i=1}^{m}p_{i}(t)x \bigl( \tau_{i}(t) \bigr) \geq0 \quad\quad \Biggl[ x^{\prime}(t)-\sum _{i=1}^{m}q_{i}(t)x \bigl( \sigma_{i}(t) \bigr) \leq0 \Biggr] ,\quad \forall t\geq t _{0}, $$

    has no eventually negative solutions.

2.4 An example

We give an example that illustrates a case when Theorem 1 of the present paper yields oscillation, while previously known results fail. The calculations were made by the use of MATLAB software.

Example 1

Consider the delay differential equation

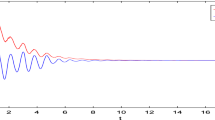
$$ x^{\prime}(t)+\frac{39}{500}x\bigl(\tau_{1}(t)\bigr)+ \frac{19}{500}x\bigl(\tau_{2}(t)\bigr)+ \frac{9}{500}x\bigl( \tau_{3}(t)\bigr)=0, \quad t\geq0, $$
(2.49)

with (see Figure 1, (a))

$$\begin{aligned}& \tau_{1}(t)= \textstyle\begin{cases} -t+12k-2, & \text{if }t\in [ 6k,6k+1 ], \\ 4t-18k-7, & \text{if }t\in [ 6k+1,6k+2 ], \\ -t+12k+3, & \text{if }t\in [ 6k+2,6k+3 ], \\ t-3, & \text{if }t\in [ 6k+3,6k+4 ], \\ -2t+18k+9, & \text{if }t\in [ 6k+4,6k+5 ], \\ 5t-24k-26, & \text{if }t\in [ 6k+5,6k+6 ], \end{cases}\displaystyle \quad\text{and} \quad \begin{gathered} \tau_{2}(t)=\tau_{1}(t)-0.1, \\ \tau_{3}(t)=\tau_{1}(t)-0.2, \end{gathered} \end{aligned}$$

where \(k\in\mathbb{N} _{0}\) and \(\mathbb{N} _{0}\) is the set of nonnegative integers.

Figure 1
figure 1

The graphs of \(\pmb{\tau_{1}(t)}\) and \(\pmb{h_{1}(t)}\) .

Full size image

By (1.11), we see (Figure 1, (b)) that

$$\begin{aligned}& h_{1}(t)= \textstyle\begin{cases} 6k-2, & \text{if }t\in [ 6k,6k+1.25 ], \\ 4t-18k-7, & \text{if }t\in [ 6k+1.25,6k+2 ], \\ 6k+1, & \text{if }t\in [ 6k+2,6k+5.4 ], \\ 5t-24k-26, & \text{if }t\in [ 6k+5.4,6k+6 ], \end{cases}\displaystyle \quad \text{and}\quad \begin{gathered} h_{2}(t)=h_{1}(t)-0.1, \\ h_{3}(t)=h_{1}(t)-0.2, \end{gathered} \end{aligned}$$

and consequently,

$$ h(t)=\max_{1\leq i\leq3} \bigl\{ h_{i}(t) \bigr\} =h_{1}(t) \quad\text{and}\quad\tau(t)=\max_{1\leq i\leq3} \bigl\{ \tau _{i}(t) \bigr\} =\tau_{1}(t). $$

It is easy to verify that

$$ \alpha=\liminf_{t\rightarrow\infty} \int _{\tau(t)}^{t} \sum_{i=1}^{3}p_{i}(s)\,ds=0.134 \cdot\liminf_{k\rightarrow \infty} \int_{6k+1}^{6k+2}\,ds=0.134, $$

and therefore, the smaller root of \(e^{0.134\lambda}=\lambda\) is \(\lambda_{0}=1.16969\).

Observe that the function \(F_{j}:[0,\infty)\rightarrow\mathbb{R} _{+}\) defined as

$$ F_{j}(t)= \int_{h(t)}^{t}P(s)\exp \biggl( \int_{\tau ( s ) } ^{h(t)}P(u)\exp \biggl( \int_{\tau(u)}^{u}\overline{R}_{j}(\xi)\,d\xi \biggr) \,du \biggr) \,ds $$

attains its maximum at \(t=6k+5.4\), \(k\in\mathbb{N} _{0}\), for every \(j\geq1\). Specifically,

$$ F_{1}(t=6k+5.4)= \int_{6k+1}^{6k+5.4}P(s)\exp \biggl( \int_{\tau ( s ) }^{6k+1}P(u)\exp \biggl( \int_{\tau(u)} ^{u}\overline{R}_{1}(\xi)\,d\xi \biggr) \,du \biggr) \,ds $$

with

$$ \overline{R}_{1}(\xi)=P(\xi) \biggl[ 1+ \int_{\tau(\xi)}^{\xi}P(v) \exp \biggl( \int_{\tau ( v ) }^{\xi}P(w)\exp \biggl( \int_{\tau(w)}^{w}\lambda_{0}P(z)\,dz \biggr) \,dw \biggr) \,dv \biggr] . $$

By using an algorithm on MATLAB software, we obtain

$$ F_{1}(t=6k+5.4)\simeq1.0071, $$

and so

$$ \limsup_{t\rightarrow\infty}F_{1}(t)\simeq1.0071>1. $$

That is, condition (2.4) of Theorem 1 is satisfied for \(j=1\), and therefore all solutions of (2.49) are oscillatory.

Observe, however, that

$$\begin{aligned}& \mathit {MD}=\limsup_{k\rightarrow\infty} \int _{6k+1}^{6k+5.4}\sum_{i=1}^{3}p_{i}(s)\,ds=0.5896< 1, \\& \alpha=0.134< \frac{1}{e}, \end{aligned}$$

and

$$\begin{aligned}& \liminf_{t\rightarrow\infty}\sum_{i=1}^{3}p_{i}(t) \bigl( t- \tau_{i}(t) \bigr) \\& \quad = \liminf_{t\rightarrow\infty} \biggl[ \frac{39}{500} \bigl( t- \tau_{1}(t) \bigr) +\frac{19}{500} \bigl( t- \bigl( \tau_{1}(t)-0.1 \bigr) \bigr) +\frac{9}{500} \bigl( t- \bigl( \tau_{1}(t)-0.2 \bigr) \bigr) \biggr] \\& \quad = \liminf_{t\rightarrow\infty} \bigl[ 0.134 \bigl( t-\tau_{1}(t) \bigr) +0.0074 \bigr] =\liminf_{t\rightarrow\infty} \bigl[ 0.134 \bigl( t- \tau_{1}(t) \bigr) \bigr] +0.0074 \\& \quad = 0.134\cdot\liminf_{t\rightarrow\infty} \bigl( t-\tau_{1}(t) \bigr) +0.0074=0.134\cdot1+0.0074=0.1414< \frac{1}{e}. \end{aligned}$$

Also, observe that the function \(G_{r}:[0,\infty)\rightarrow \mathbb{R} _{+}\) defined as

$$ G_{r}(t)= \int_{h(t)}^{t}\sum_{i=1}^{m}p_{i}( \zeta)a_{r}\bigl(h(t), \tau_{i}(\zeta)\bigr)\,d\zeta $$

attains its maximum at \(t=6k+5.4\) and its minimum at \(t=6k+2\), \(k \in\mathbb{N} _{0}\), for every \(r\in\mathbb{N} \). Specifically,

$$\begin{aligned} G_{1}(t =6k+5.4) & = \int_{6k+1}^{6k+5.4}\sum_{i=1}^{3}p_{i}( \zeta)a_{1}\bigl(6k+1,\tau_{i}(\zeta)\bigr)\,d\zeta \\ & = \int_{6k+1}^{6k+2} \bigl[ p_{1}( \zeta)a_{1}\bigl(6k+1,\tau_{1}(\zeta)\bigr)+p _{2}(\zeta)a_{1}\bigl(6k+1,\tau_{2}(\zeta) \bigr) \\ & \quad {}+p_{3}(\zeta)a_{1}\bigl(6k+1,\tau_{3}( \zeta)\bigr) \bigr] \,d\zeta \\ &\quad {}+ \int_{6k+2}^{6k+3} \bigl[ p_{1}( \zeta)a_{1}\bigl(6k+1,\tau_{1}(\zeta)\bigr)+p _{2}(\zeta)a_{1}\bigl(6k+1,\tau_{2}(\zeta) \bigr) \\ & \quad {}+p_{3}(\zeta)a_{1}\bigl(6k+1,\tau_{3}( \zeta)\bigr) \bigr] \,d\zeta \\ &\quad {}+ \int_{6k+3}^{6k+4} \bigl[ p_{1}( \zeta)a_{1}\bigl(6k+1,\tau_{1}(\zeta)\bigr)+p _{2}(\zeta)a_{1}\bigl(6k+1,\tau_{2}(\zeta) \bigr) \\ & \quad {}+p_{3}(\zeta)a_{1}\bigl(6k+1,\tau_{3}( \zeta)\bigr) \bigr] \,d\zeta \\ & \quad {}+ \int_{6k+4}^{6k+5} \bigl[ p_{1}( \zeta)a_{1}\bigl(6k+1,\tau_{1}(\zeta)\bigr)+p _{2}(\zeta)a_{1}\bigl(6k+1,\tau_{2}(\zeta) \bigr) \\ &\quad {}+p_{3}(\zeta)a_{1}\bigl(6k+1,\tau_{3}( \zeta)\bigr) \bigr] \,d\zeta \\ & \quad {}+ \int_{6k+5}^{6k+5.4} \bigl[ p_{1}( \zeta)a_{1}\bigl(6k+1,\tau_{1}(\zeta )\bigr)+p_{2}( \zeta)a_{1}\bigl(6k+1,\tau_{2}(\zeta)\bigr) \\ & \quad {}+p_{3}( \zeta)a_{1}\bigl(6k+1, \tau_{3}(\zeta)\bigr) \bigr] \,d\zeta \\ & \simeq 0.6843 \end{aligned}$$

and

$$\begin{aligned} G_{1}(t =6k+2) =& \int_{6k+1}^{6k+2}\sum_{i=1}^{3}p_{i}( \zeta)a _{1}\bigl(6k+1,\tau_{i}(\zeta)\bigr)\,d\zeta \\ =& \int_{6k+1}^{6k+2} \bigl[ p_{1}( \zeta)a_{1}\bigl(6k+1,\tau_{1}(\zeta)\bigr)+p _{2}(\zeta)a_{1}\bigl(6k+1,\tau_{2}(\zeta) \bigr) \\ &{} +p_{3}(\zeta)a_{1}\bigl(6k+1,\tau_{3}( \zeta)\bigr) \bigr] \,d\zeta \\ \simeq&0.1786. \end{aligned}$$

Thus

$$\begin{aligned}& \limsup_{t\rightarrow\infty}G_{1}(t)\simeq0.6843< 1, \\& \liminf_{t\rightarrow\infty}G_{1}(t)\simeq0.1786< 1/e, \end{aligned}$$

and

$$ 0.6843< 1-D(\alpha)\simeq0.9895. $$

Also

$$ \int_{6k+1}^{6k+5.4}\sum_{i=1}^{3}p_{i}( \zeta)a_{1}\bigl(h(\zeta), \tau_{i}(\zeta)\bigr)\,d\zeta\leq G_{1}(t=6k+5.4)\simeq0.6843. $$

Thus

$$\begin{aligned} \limsup_{k\rightarrow\infty} \int_{6k+1}^{6k+5.4}\sum_{i=1} ^{3}p_{i}(\zeta)a_{1}\bigl(h(\zeta), \tau_{i}(\zeta)\bigr)\,d\zeta \leq&0.6843 < \frac{1+\ln\lambda_{0}}{\lambda_{0}}-D(\alpha)\simeq0.9784 . \end{aligned}$$

Also

$$\begin{aligned}& \limsup_{t\rightarrow\infty} \int_{h(t)}^{t}\overline{P}(s)\exp \biggl( \int_{\tau(s)}^{h(t)}\overline{P}_{1}(u)\,du \biggr) \,ds\simeq0.8639< 1, \\& 0.8639< 1-D(\alpha)\simeq0.9895, \\& \limsup_{t\rightarrow\infty} \int_{h(t)}^{t}\overline{P}(s)\exp \biggl( \int_{\tau(s)}^{t}\overline{P}_{1}(u)\,du \biggr) \,ds \\& \quad = \limsup_{k\rightarrow\infty} \int_{6k+1}^{6k+5.4}\overline{P}(s) \exp \biggl( \int_{\tau(s)}^{6k+5.4}\overline{P}_{1}(u)\,du \biggr) \,ds \simeq3.1806 \\& \quad < \frac{1}{D(\alpha)}\simeq95.2891, \\& \limsup_{t\rightarrow\infty} \int_{h(t)}^{t}\overline{P}(s)\exp \biggl( \int_{\tau(s)}^{h(s)}\overline{P}_{1}(u)\,du \biggr) \,ds \\& \quad \leq \limsup_{t\rightarrow\infty} \int_{h(t)}^{t}\overline{P}(s) \exp \biggl( \int_{\tau(s)}^{h(t)}\overline{P}_{1}(u)\,du \biggr) \,ds \\& \quad \simeq 0.8639< \frac{1+\ln\lambda_{0}}{\lambda_{0}}-D(\alpha) \simeq0.9784, \\& \liminf_{t\rightarrow\infty} \int_{h(t)}^{t}\overline{P}(s)\exp \biggl( \int_{\tau(s)}^{h(t)}\overline{P}_{1}(u)\,du \biggr) \,ds\simeq0.2852< \frac{1}{e}. \end{aligned}$$

That is, none of the conditions (1.8)-(1.10), (1.13)-(1.16) (for \(r=1\)) and (1.17)-(1.21) (for \(j=1\)) is satisfied.

Comments

It is worth noting that the improvement of condition (2.4) to the corresponding condition (1.8) is significant, approximately 70.81%, if we compare the values on the left-hand side of these conditions. Also, the improvement compared to conditions (1.13) and (1.17) is very satisfactory, around 47.17% and 16.58%, respectively.

Finally, observe that conditions (1.13)-(1.21) do not lead to oscillation for the first iteration. On the contrary, condition (2.4) is satisfied from the first iteration. This means that our condition is better and much faster than (1.13)-(1.21).

Remark 3

Similarly, one can construct examples to illustrate the other main results.