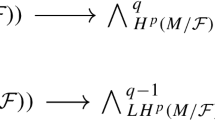Abstract
We prove that the de Rham \( L^{\phi} \)-cohomology of a Riemannian manifold \( M \) admitting a convenient triangulation \( X \) is isomorphic to the simplicial \( \ell^{\phi} \)-cohomology of \( X \) under some assumptions on the Young function \( \phi \). This result implies the quasi-isometry invariance of the first cohomology.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Carrasco Piaggio M., “Orlicz spaces and the large scale geometry of Heintze groups,” Math. Ann., vol. 368, no. 1, 433–481 (2017).
Gol’dshtein V. and Kopylov Ya., “Some calculations of Orlicz cohomology and Poincaré–Sobolev–Orlicz inequalities,” Sib. Electr. Math. Reports, vol. 16, 1079–1090 (2019).
Kopylov Ya., “Orlicz spaces of differential forms on Riemannian manifolds: duality and cohomology,” Problemy Analiza, vol. 6, no. 2, 57–80 (2017).
Kopylov Ya., “On the Orlicz cohomology of star-bounded simplicial complexes,” Sib. Electr. Math. Reports, vol. 18, 710–719 (2021).
Kopylov Ya. A. and Panenko R. A., “\( \Phi \)-Harmonic functions on discrete groups and the first \( \ell^{\Phi} \)-cohomology,” Sib. Math. J., vol. 55, no. 5, 904–914 (2014).
Kopylov Ya. A. and Panenko R. A., “De Rham regularization operators in Orlicz spaces of differential forms on Riemannian manifolds,” Sib. Electr. Math. Reports, vol. 12, 361–371 (2015).
Pansu P., “Cohomologie \( L^{p} \) et piencement,” Comment. Math. Helv., vol. 83, no. 2, 327–357 (2008).
Sequeira E., Relative \( L^{p} \)-Cohomology and Applications to Heintze Groups. PhD Thesis, Universidad de la República (Uruguay) and Université de Lille (France), Lille (2020).
Gol’dshtein V. M. and Troyanov M., “Sobolev inequalities for differential forms and \( L_{q,p} \)-cohomology,” J. Geom. Anal., vol. 16, no. 4, 597–631 (2016).
Puls M., “The first \( L^{p} \)-cohomology of some finitely generated groups and \( p \)-harmonic functions,” J. Funct. Anal., vol. 237, no. 2, 391–401 (2006).
Bourdon M. and Rémy B., “Quasi-isometric invariance of continuous group \( L^{p} \)-cohomology, and first applications to vanishings,” Ann. Henri Lebesgue, vol. 3, 1291–1326 (2020).
Genton L., Scaled Alexander–Spanier Cohomology and \( L_{qp} \)-Cohomology for Metric Spaces. PhD Thesis no. 6330, EPFL, Lausanne (2014).
Gol’dshtein V. M., Kuz’minov V. I., and Shvedov I. A., “The de Rham isomorphism of \( l_{p} \)-cohomology of noncompact Riemannian manifold,” Sib. Math. J., vol. 29, no. 2, 190–197 (1988).
Pansu P., Cohomologie \( L^{p} \): Invariance sour quasiisométries [Preprint] (1995). https://www.math.u-psud.fr/pansu/liste-prepub.html
Attie O., “Quasi-isometry classification of some manifolds of bounded geometry,” Math. Z., vol. 216, no. 4, 501–527 (1994).
Bott R. and Tu L. W., Differential Forms in Algebraic Topology, Springer, New York (1982) (Graduate Text Math.; Vol. 82).
Rao M. M. and Ren Z. D., Theory of Orlicz Spaces, Marcel Dekker, New York (1991) (Monogr. Textbooks Pure Appl. Math.; Vol. 146).
Whitney H., Geometric Integration Theory, Princeton University, Princeton (1957).
Acknowledgments
The author is deeply grateful to Marc Bourdon and Matías Carrasco for their guidance and valuable suggestions, and to Yaroslav Kopylov for his helpful ideas and discussions. The author thanks the anonymous referee for important corrections.
Funding
The work is supported by the Mathematical Center in Akademgorodok under Agreement 075–15–2019–1675 with the Ministry of Science and Higher Education of the Russian Federation.
Author information
Authors and Affiliations
Corresponding author
Additional information
Translated from Sibirskii Matematicheskii Zhurnal, 2022, Vol. 63, No. 4, pp. 935–948. https://doi.org/10.33048/smzh.2022.63.418
Rights and permissions
About this article
Cite this article
Sequeira, E. De Rham’s Theorem for Orlicz Cohomology. Sib Math J 63, 777–788 (2022). https://doi.org/10.1134/S0037446622040188
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1134/S0037446622040188