Surface Effect on Vibration of Timoshenko Nanobeam Based on Generalized Differential Quadrature Method and Molecular Dynamics Simulation

Abstract

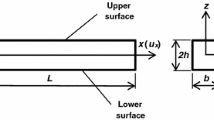
Nanobeams have promising applications in areas such as sensors, actuators, and resonators in nanoelectromechanical systems (NEMS). Considering the effects of gyration inertia, surface layer mass, surface residual stress, and surface Young’s modulus, this study develops the vibration equations of the Timoshenko nanobeam. The generalized differential quadrature (GDQ) method and molecular dynamics (MD) simulation are used to study the surface effect on vibration. For a rectangular cross section, surface residual stress and surface Young’s modulus are all affected by the height of the cross section rather than by the length–height ratio. If surface layer mass is considered, then the first three natural frequencies all decrease relative to their counterparts in the case in which surface layer mass is ignored. Results show that the effect of gyration inertia on resonance frequency is negligible. Longitudinal vibration does not easily occur relative to the bending and rotation vibrations of nanobeams. In addition, the results obtained by the GDQ method fit those obtained by MD simulation for beams with length–height ratios of 4–8. This study provides insights into the mechanism of the vibration of short and deep nanobeams and sheds light on the quantitative design of the elements in NEMSs.

Appendices

Appendix A

In Eqs. (23–25) of Sect. 2, parameters $a_{11}$, $a_{12}$, $a_{21}$, $a_{22}$, $a_{23}$, $a_{24}$, $a_{31}$,${ }a_{32}$, $a_{33}$, $a_{34}$, $a_{35}$, $a_{36}$, and $a_{37}$ are provided as

$$ a_{11} = \frac{{\left[ {Ebh/\left( {1 - \nu^{2} } \right) + 2b\left( {2\mu^{\text{s}} + \lambda^{\text{s}} } \right)} \right]}}{{\left( {3b\tau^{\text{s}} + k_{\text{s}} Gbh} \right)}};a_{12} = \frac{{\left[ {Ebh/\left( {1 - \nu^{2} } \right) + 2b\left( {2\mu^{\text{s}} + \lambda^{\text{s}} - \tau^{\text{s}} } \right)} \right]}}{{\left( {3b\tau^{\text{s}} + k_{\text{s}} Gbh} \right)}};a_{21} = 1; $$

$$ a_{22} = \frac{{ - k_{\text{s}} Gbh}}{{\left( {3b\tau^{\text{s}} + k_{\text{s}} Gbh} \right)}} ;a_{31} = \frac{{ - k_{\text{s}} Gbh}}{{\left( {\rho I_{y} + \frac{1}{2}\rho_{\text{s}} bh^{2} + \frac{{\rho_{\text{s}} }}{6}h^{3} } \right)}}\frac{{L^{2} \left( {\rho bh + 2\rho_{\text{s}} b + 2\rho_{\text{s}} h} \right)}}{{\left( {3b\tau^{\text{s}} + k_{\text{s}} Gbh} \right)}}; $$

$$ a_{32} = \frac{{\left[ {\frac{{Ebh^{3} }}{{12\left( {1 - \nu^{2} } \right)}} + \frac{{bh^{2} }}{2}\left( {2\mu^{\text{s}} + \lambda^{\text{s}}} \right) - k_{\text{s}} k_{m} Gbh} \right]}}{{\left( {\rho I_{y} + \frac{1}{2}\rho_{\text{s}} bh^{2} + \frac{{\rho_{\text{s}} }}{6}h^{3} } \right)}}\frac{{\left( {\rho bh + 2\rho_{\text{s}} b + 2\rho_{\text{s}} h} \right)}}{{\left( {3b\tau^{\text{s}} + k_{\text{s}} Gbh} \right)}}; $$

$$ a_{33} = \frac{{\left( {k_{\text{s}} Gbh + \tau^{\text{s}} b} \right)}}{{\left( {\rho I_{y} + \frac{1}{2}\rho_{\text{s}} bh^{2} + \frac{{\rho_{\text{s}} }}{6}h^{3} } \right)}}\frac{{L^{2} \left( {\rho bh + 2\rho_{\text{s}} b + 2\rho_{\text{s}} h} \right)}}{{\left( {3b\tau^{\text{s}} + k_{\text{s}} Gbh} \right)}}; $$

$$ a_{34} = - \frac{{bh^{2} \nu \tau^{\text{s}} }}{{6\left( {\rho I_{y} + \frac{1}{2}\rho_{\text{s}} bh^{2} + \frac{{\rho_{\text{s}} }}{6}h^{3} } \right)\left( {1 - \nu } \right)}}\frac{{L\left( {\rho bh + 2\rho_{\text{s}} b + 2\rho_{\text{s}} h} \right)}}{{\left( {3b\tau^{\text{s}} + k_{\text{s}} Gbh} \right)}};\quad a_{35} = k_{m} \frac{{\left( {\rho bh + 2\rho_{\text{s}} b + 2\rho_{\text{s}} h} \right)}}{{\left( {\rho I_{y} + \frac{1}{2}\rho_{\text{s}} bh^{2} + \frac{{\rho_{\text{s}} }}{6}h^{3} } \right)}}; $$

$$ a_{36} = \frac{{3k_{m} }}{{2\left( {\rho I_{y} + \frac{1}{2}\rho_{\text{s}} bh^{2} + \frac{{\rho_{\text{s}} }}{6}h^{3} } \right)}}\left[ {Ebh/\left( {1 - \nu^{2} } \right) + 2b\left( {2\mu^{\text{s}} + \lambda^{\text{s}} - \tau^{\text{s}} } \right)} \right]\frac{{\left( {\rho bh + 2\rho_{\text{s}} b + 2\rho_{\text{s}} h} \right)}}{{\left( {3b\tau^{\text{s}} + k_{\text{s}} Gbh} \right)}}; $$

$$ a_{37} = \frac{{k_{m} }}{{\left( {\rho I_{y} + \frac{1}{2}\rho_{\text{s}} bh^{2} + \frac{{\rho_{\text{s}} }}{6}h^{3} } \right)}}\left[ {Ebh/\left( {1 - \nu^{2} } \right) + 2b\left( {2\mu^{\text{s}} + \lambda^{\text{s}} } \right)} \right]\frac{{\left( {\rho bh + 2\rho_{\text{s}} b + 2\rho_{\text{s}} h} \right)}}{{\left( {3b\tau^{\text{s}} + k_{\text{s}} Gbh} \right)}}. $$

Appendix B

In Sect. 3, the procedure to compute the weighting coefficients of the first and higher-order derivatives based on the GDQ method are written as

$$ p\left( {\xi_{i} } \right) = \mathop \prod \limits_{j = 1,j \ne i}^{j = N} \left( {\xi_{i} - \xi_{j} } \right); $$

$$ p\left( \xi \right) = \mathop \prod \limits_{j = 1}^{j = N} \left( {\xi - \xi_{j} } \right); $$

$$ \overline{w}\left( \xi \right) = \mathop \sum \limits_{i = 1}^{N} l_{i} \left( \xi \right)\overline{w}\left( {\xi_{i} } \right); $$

$$ \phi \left( \xi \right) = \mathop \sum \limits_{i = 1}^{N} l_{i} \left( \xi \right)\phi \left( {\xi_{i} } \right); $$

$$ l_{i} \left( \xi \right) = \mathop \prod \limits_{j = 1,j \ne i}^{N} \frac{{\xi - \xi_{k} }}{{\xi_{j} - \xi_{k} }}; $$

$$ l_{j} \left( {\xi_{i} } \right) = \left\{ {\begin{array}{*{20}c} {1, \left( {i = j} \right)} \\ {0,\left( {i \ne j} \right)} \\ \end{array} } \right.; $$

$$ {\varvec{D}}_{ij}^{\left( 1 \right)} = l_{j}^{^{\prime}} \left( {\xi_{i} } \right) = \frac{{p\left( {\xi_{i} } \right)}}{{\left( {\xi_{i} - \xi_{j} } \right)p\left( {\xi_{j} } \right)}}, \left( {i \ne j} \right); $$

$$ {\varvec{D}}_{ii}^{\left( 1 \right)} = l_{i}^{^{\prime}} \left( {\xi_{i} } \right) = \mathop \sum \limits_{j = 1, j \ne i}^{N} \frac{1}{{\xi_{i} - \xi_{j} }} = - \mathop \sum \limits_{j = 1, j \ne i}^{N} B_{ij}^{\left( 1 \right)} ; $$

$$ {\varvec{D}}_{ij}^{\left( r \right)} = n\left( {B_{ii}^{{\left( {r - 1} \right)}} B_{ij}^{\left( 1 \right)} - \frac{{B_{ij}^{{\left( {r - 1} \right)}} }}{{\xi_{i} - \xi_{j} }}} \right),\,\left( {i \ne j} \right); $$

$$ {\varvec{D}}_{ii}^{\left( r \right)} = \mathop \sum \limits_{j = 1, j \ne i}^{N} B_{ij}^{\left( r \right)} ,\,\left( {i = j} \right); $$

Appendix C

In Sect. 3, the process to compute the matrices M, K, and N using the GDQ method can be described as

$$ {\varvec{M}} = \left[ {\begin{array}{*{20}c} {{\varvec{M}}_{{\overline{u}}} } & 0 & 0 \\ 0 & {{\varvec{M}}_{{\overline{w}}} } & 0 \\ 0 & 0 & {{\varvec{M}}_{\phi } } \\ \end{array} } \right]_{3N \times 3N} ; $$

$$ {\varvec{K}} = \left[ {\begin{array}{*{20}c} { - a_{11} {\varvec{D}}^{\left( 2 \right)} } & 0 & 0 \\ 0 & { - a_{21} {\varvec{D}}^{\left( 2 \right)} } & { - a_{22} {\varvec{D}}^{\left( 1 \right)} } \\ 0 & { - a_{33} {\varvec{D}}^{\left( 1 \right)} - a_{34} {\varvec{D}}^{\left( 2 \right)} - a_{35} {\varvec{D}}^{\left( 3 \right)} } & { - a_{31} {\mathbf{I}} - a_{32} {\varvec{D}}^{\left( 2 \right)} } \\ \end{array} } \right]. $$

$$ {\varvec{N}}\left( {\varvec{Z}} \right) = \left[ {\begin{array}{*{20}c} {{\varvec{N}}_{{\overline{u}}} } \\ {{\varvec{N}}_{{\overline{w}}} } \\ {{\varvec{N}}_{\phi } } \\ \end{array} } \right] $$

where

$$ {\varvec{M}}_{{\overline{u}}} = {\varvec{M}}_{{\overline{w}}} = {\varvec{M}}_{\phi } = {\mathbf{I}} = \left[ {\begin{array}{*{20}c} 1 & \cdots & 0 \\ \vdots & \ddots & \vdots \\ 0 & \cdots & 1 \\ \end{array} } \right]_{N \times N} ; $$

$$ {\varvec{N}}_{{\overline{\varvec{u}}}} = - a_{12} \left( {{\varvec{D}}^{\left( 1 \right)} \overline{\varvec{w}}} \right) \circ \left( {{\varvec{D}}^{\left( 2 \right)} \overline{\varvec{w}}} \right); $$

$$ {\varvec{N}}_{{\overline{w}}} = - a_{23} \left( {{\varvec{D}}^{\left( 1 \right)} \overline{\varvec{w}}} \right) \circ \left( {{\varvec{D}}^{\left( 1 \right)} \overline{\varvec{w}}} \right) \circ \left( {{\varvec{D}}^{\left( 2 \right)} \overline{\varvec{w}}} \right) - a_{24} \left[ {\left( {{\varvec{D}}^{\left( 1 \right)} \overline{\varvec{w}}} \right) \circ \left( {{\varvec{D}}^{\left( 2 \right)} \overline{\varvec{u}}} \right) + \left( {{\varvec{D}}^{\left( 1 \right)} \overline{\varvec{u}}} \right) \circ \left( {{\varvec{D}}^{\left( 2 \right)} \overline{\varvec{w}}} \right)} \right]; $$

$$ \begin{aligned} {\varvec{N}}_{\phi } & = - a_{36} \left[ {2\left( {{\varvec{D}}^{\left( 2 \right)} \overline{\varvec{w}}} \right) \circ \left( {{\varvec{D}}^{\left( 2 \right)} \overline{\varvec{w}}} \right) \circ \left( {{\varvec{D}}^{\left( 1 \right)} \overline{\varvec{w}}} \right) + \left( {{\varvec{D}}^{\left( 1 \right)} \overline{\varvec{w}}} \right) \circ \left( {{\varvec{D}}^{\left( 1 \right)} \overline{\varvec{w}}} \right) \circ \left( {{\varvec{D}}^{\left( 3 \right)} \overline{\varvec{w}}} \right)} \right] \\ & \quad - a_{37} \left[ {2\left( {{\varvec{D}}^{\left( 2 \right)} \overline{\varvec{w}}} \right) \circ \left( {{\varvec{D}}^{\left( 2 \right)} \overline{\varvec{u}}} \right) + \left( {{\varvec{D}}^{\left( 1 \right)} \overline{\varvec{w}}} \right) \circ \left( {{\varvec{D}}^{\left( 3 \right)} \overline{\varvec{u}}} \right) + \left( {{\varvec{D}}^{\left( 1 \right)} \overline{\varvec{u}}} \right) \circ \left( {{\varvec{D}}^{\left( 3 \right)} \overline{\varvec{w}}} \right)} \right]; \\ \end{aligned} $$

The operation symbol $\circ$ represents the Hadamard product.

The Hadamard and Kronecker Products are as follows.

Definition 1

Hadamard product.

Let

$$ {\varvec{A}} = \left[ {A_{ij} } \right]_{N \times M} ,{\varvec{B}} = \left[ {B_{ij} } \right]_{N \times M} $$

The Hadamard product expressed in matrix form is

$$ {\varvec{A}} \circ {\varvec{B}} = \left[ {A_{ij} B_{ij} } \right]_{{{\varvec{N}} \times {\varvec{M}}}} . $$

Based on the GDQ method, the computation of the weighting coefficients of the first- and higher-order derivatives is

$$ \left[ {\begin{array}{*{20}c} { - a_{11} {\varvec{D}}^{\left( 2 \right)} - \omega^{2} {\varvec{M}}_{{\overline{u}}} } & 0 & 0 \\ 0 & { - a_{21} {\varvec{D}}^{\left( 2 \right)} - \omega^{2} {\varvec{M}}_{{\overline{w}}} } & { - a_{22} {\varvec{D}}^{\left( 1 \right)} } \\ 0 & { - a_{33} {\varvec{D}}^{\left( 1 \right)} - a_{34} {\varvec{D}}^{\left( 2 \right)} - a_{35} {\varvec{D}}^{\left( 3 \right)} } & { - a_{31} {\mathbf{I}} - a_{32} {\varvec{D}}^{\left( 2 \right)} - \omega^{2} {\varvec{M}}_{\phi } } \\ \end{array} } \right]\left[ {\begin{array}{*{20}c} {\overline{\varvec{u}}} \\ {\overline{\varvec{w}}} \\ \phi \\ \end{array} } \right] + \left[ {\begin{array}{*{20}c} {{\varvec{N}}_{{\overline{u}}} } \\ {{\varvec{N}}_{{\overline{w}}} } \\ {{\varvec{N}}_{\phi } } \\ \end{array} } \right] = \left[ {\begin{array}{*{20}c} 0 \\ 0 \\ 0 \\ \end{array} } \right]. $$

$$ {\varvec{W}} = \left[ {W_{ij} } \right]_{N \times N} $$

$$ W_{ij} = \left\{ {\begin{array}{*{20}l} {1, i - j = 1,\,\,or\,\, i = j = N} \\ { - 1, i - j = - 1,\,\,or\,\, i = j = 1} \\ {0, i - j \ne \pm 1,i = j \ne N ,i = j \ne 1 } \\ \end{array} } \right.. $$

Definition 2

Based on the trapezoidal rule, the integral matrix operators are.

$$ \mathop \int \limits_{a}^{b} f\left( x \right){\text{d}}x = \frac{1}{2}{\varvec{XWF}} = {\varvec{S}}_{{\varvec{x}}} \varvec{F}, $$

where ${\varvec{X}} = \left\{ {x_{1} ,x_{2} , \ldots ,x_{N} } \right\}$, ${\varvec{F}} = \left\{ {f\left( {x_{1} } \right),f\left( {x_{2} } \right), \ldots ,f\left( {x_{N} } \right)} \right\}^{{\text{T}}}$,$\varvec{S}_{{\varvec{x}}} = \left\{ {{\varvec{S}}_{{\varvec{x}}} } \right\}_{1 \times N} = \frac{1}{2}{\varvec{XW}}$