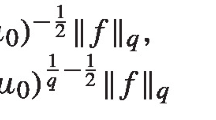Abstract
Let the vector fields \(X_1, \ldots , X_{6}\) form an orthonormal basis of \(\mathcal {H}\), the orthogonal complement of a Cartan subalgebra (of dimension 2) in \({{\,\mathrm{SU}\,}}(3)\). We prove that weak solutions u to the degenerate subelliptic p-Laplacian
have Hölder continuous horizontal derivatives \(\nabla _{\!{\mathcal {H}}}u=(X_1u, \ldots , X_{6}u)\) for \(p\ge 2\). We also prove that a similar result holds for all compact connected semisimple Lie groups.
Similar content being viewed by others
References
Arvanitoyeorgos, A.: An Introduction to Lie Groups and the Geometry of Homogeneous Spaces, Volume 22 of Student Mathematical Library. American Mathematical Society, Providence, RI, 2003. Translated from the 1999 Greek original and revised by the author
Capogna, L.: Regularity of quasi-linear equations in the Heisenberg group. Commun. Pure Appl. Math. 50(9), 867–889 (1997)
Capogna, L.: Regularity for quasilinear equations and \(1\)-quasiconformal maps in Carnot groups. Math. Ann. 313(2), 263–295 (1999)
Capogna, L., Citti, G.: Regularity for subelliptic PDE through uniform estimates in multi-scale geometries. Bull. Math. Sci. 6(2), 173–230 (2016)
Capogna, L., Citti, G., Le Donne, E., Ottazzi, A.: Conformality and Q-harmonicity in sub-Riemannian manifolds. J. Math. Pures Appl. 122, 67–124 (2019)
Domokos, A., Manfredi, J.J.: Nonlinear subelliptic equations. Manuscripta Math. 130(2), 251–271 (2009)
Giusti, E.: Direct Methods in the Calculus of Variations. World Scientific Publishing Co., Inc., River Edge (2003)
Hoffmann, K.H., Morris, S.A.: The Structure of Compact Groups. de Gruyter Studies in Mathematics, vol. 25. Walter de Gruyter Gmbh, Berlin (2006)
Hörmander, L.: Hypoelliptic second order differential equations. Acta Math. 119, 147–171 (1967)
Kinnunen, J., Marola, N., Miranda Jr., M., Paronetto, F.: Harnack’s inequality for parabolic De Giorgi classes in metric spaces. Adv. Differ. Equ. 17(9–10), 801–832 (2012)
Kinnunen, J., Shanmugalingam, N.: Regularity of quasi-minimizers on metric spaces. Manuscripta Math. 105(3), 401–423 (2001)
Ladyzhenskaya, O.A., Ural’tseva, N.N.: Linear and quasilinear elliptic equations. Translated from the Russian by Scripta Technica, Inc. Translation editor: Leon Ehrenpreis. Academic Press, New York-London (1968)
Manfredi, J.J., Mingione, G.: Regularity results for quasilinear elliptic equations in the Heisenberg group. Math. Ann. 339(3), 485–544 (2007)
Mukherjee, S., Zhong, X.: \({C}^{1,\alpha }\)-regularity for variational problems in the Heisenberg group. arXiv:1711.04671 (2017)
Mingione, G., Zatorska-Goldstein, A., Zhong, X.: Gradient regularity for elliptic equations in the Heisenberg group. Adv. Math. 222(1), 62–129 (2009)
Ricciotti, D.: \(p\)-Laplace equation in the Heisenberg group. SpringerBriefs in Mathematics. Springer, Cham; BCAM Basque Center for Applied Mathematics, Bilbao. Regularity of solutions, BCAM SpringerBriefs (2015)
Ricciotti, D.: On the \(C^{1,\alpha }\) regularity of \(p\)-harmonic functions in the Heisenberg group. Proc. Am. Math. Soc. 146(7), 2937–2952 (2018)
Rothschild, L.P., Stein, E.M.: Hypoelliptic differential operators and nilpotent groups. Acta Math. 137(3–4), 247–320 (1976)
Varopoulos, N., Saloff-Coste, L., Coulhon, T.: Analysis and Geometry on Groups. Cambridge Tracts in Mathematics, vol. 100. Cambridge University Press, Cambridge (1992)
Zhong, X.: Regularity for variational problems in the Heisenberg group. arXiv:1711.03284v1 (2017)
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
The authors declare that they have no conflict of interest.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Domokos, A., Manfredi, J.J. \(C^{1,\alpha }\)-subelliptic regularity on \({{\,\mathrm{SU}\,}}(3)\) and compact, semi-simple Lie groups. Anal.Math.Phys. 10, 4 (2020). https://doi.org/10.1007/s13324-019-00350-6
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s13324-019-00350-6