Interface Stability of Compressible Fluid Displacements in Porous Media

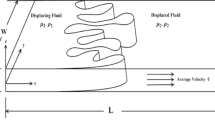
Abstract

We use linear stability theory to investigate the effect of fluid compressibility on interface stability during a dissipative displacement (Darcy flow). We find that compressibility changes the perturbation growth rate as a function of perturbation wavenumber. Our results indicate that both favorable (less than unity) and unfavorable (greater than unity) mobility ratios will always lead to positive maximum growth rate, which traditionally is recognized as the criterion for instability. We conclude, however, that in the case of favorable mobility ratio, the maximum perturbation growth rate is always smaller than the unperturbed growth rate naturally existing in compressible displacements. The interface will still be stable because the perturbation will never exceed the background flow. Therefore, compressibility does not change the stability of displacements, which is ultimately determined by mobility ratio.

Appendix: Long-Wave Asymptotes

We will take ${n}_{y}=0$ and look at Eqs. 53 and 54 under long-wave asymptotic condition (${n}_{y}\to 0$).

$$1-M=-{\sigma }_{D}\left[\frac{1}{{n}_{xD}^{I}+\frac{\tau }{M}}+\frac{M}{{n}_{xD}^{II}-1}\right],$$

(57)

$${\sigma }_{D0}=\frac{M}{\tau }{{n}_{xD}^{I}}^{2}={{n}_{xD}^{II}}^{2}.$$

(58)

After substitution, Eqs. 57 and 58 become

$$\left(M-1\right)=\frac{\frac{M}{\tau }{\sigma }_{D0}}{\sqrt{\frac{M}{\tau }{\sigma }_{D0}}+1}+\frac{M{\sigma }_{D0}}{\sqrt{{\sigma }_{D0}}-1}.$$

(59)

Upon some algebra we arrive at polynomial.

$$\frac{\left(\frac{M}{\tau }+\tau {\left(\frac{M}{\tau }\right)}^\frac{3}{2}\right)}{M-1}{{n}_{xD}^{I}}^{3}+\left[\frac{M-\frac{M}{\tau }}{M-1}-\sqrt{\frac{M}{\tau }}\right]{{n}_{xD}^{I}}^{2}+\left(\frac{M}{\tau }-1\right){n}_{xD}^{I}+1=0.$$

(60)

This is a third-order polynomial in terms of ${n}_{xD}^{I}$, which can be easily solved analytically. Among the three roots to this equation, only one is chosen both ${n}_{xD}^{I}$ and ${n}_{xD}^{II}$ having positive real parts in order to satisfy the requirement set by Eq. 37.

Considering its nonlinearity, roots to Eq. 60 serve well as proper initial guesses for us to solve Eqs. 53 and 54. We are now fully equipped to solve Eqs. 53 and 54. The behavior of decay rate at this limit is shown in Fig. 4. 

Fig. 4

Solution space as ${N}_{yD}\to 0$. Growth (decay) rate is shown to be globally below 1. For cases where this plot matters the most (mobility ratio smaller than 1), this growth (decay) rate corresponds to the maximum rate at all values of ${N}_{yD}$