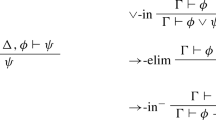Abstract
A lot has been written on solutions to the semantic paradoxes, but very little on the topic of general theories of paradoxicality. The reason for this, we believe, is that it is not easy to disentangle a solution to the paradoxes from a specific conception of what those paradoxes consist in. This paper goes some way towards remedying this situation. We first address the question of what one should expect from an account of paradoxicality. We then present one conception of paradoxicality that has been offered in the literature: the fixed-point conception. According to this conception, a statement is paradoxical if it cannot obtain a classical truth-value at any fixed-point model. In order to assess this proposal rigorously we provide a non-metalinguistic characterization of paradoxicality and we evaluate whether the resulting account satisfies a number of reasonable desiderata.
Similar content being viewed by others
Notes
Just to give the reader an idea, we can identify (i) the naive conception of paradoxicality (see Cook (2011) and Hsiung (2021)); (ii) the conception of paradoxicality as non-normalizability (cf. Prawitz (1965) and Tennant (1982)); (iii) the revision-theoretic conception (cf. Gupta (1982)); (iv) the inclosure-based conception (see Priest (1994)); and (v) the graph-theoretic conception (cf. Walicky (2017) and Rossi (2019), for a couple of recent examples). Some of these conceptions intend to cover all sorts of paradoxes. For the purposes of this paper we are only interested in semantic paradoxicality, and we are thus ignoring paradoxes that affect non-semantic concepts.
Cf. Kripke (1975). Although probably Kripke would not subscribe to some of the ideas that we will put forward below—specially to the view that paradoxicality can behave non-classically in certain circumstances. More recently, the fixed-point conception has been discussed in Cook and Tourville (2020), Cook and Tourville (2016), Cook (2020), Castaldo (2021), Rosenblatt (2021) and Gallovich and Rosenblatt (2022).
Our use of the definite description ‘the fixed-point conception of paradoxicality’ should be taken with some caution. There is a sense in which the use of fixed-points is pervasive. For example, most (if not all) of the conceptions mentioned in Footnote 1 can probably be defined in terms of (the non-existence of) fixed-points. Kripke’s construction is only a very specific example of the general applicability of fixed-points. However, the Kripke-inspired conception we will discuss explicitly relies on special semantic structures called “fixed-point models” (on which more shortly), and it does so in a very direct and blatant way. Thus, our more restrictive use of the term ‘fixed-point’ should not mislead. Thanks to an anonymous reviewer for urging us to clarify this.
Traditionally, paradoxicality is thought to be a property of arguments, and a statement is said to be paradoxical only in a derivative sense—a statement is paradoxical because it contributes to the generation of paradoxical arguments. However, given that in the fixed-point conception paradoxicality is typically attached to statements, we will assume that it is statements that are the (primary) bearers of paradoxicality. This may be contentious, but a discussion would be beyond the scope of the paper. We take it up in ongoing work.
We think that ultimately our account of paradoxicality should apply to natural languages, so we talk about paradoxical statements. A statement, as we are understanding it, is a declarative meaningful (non-ambiguous) type sentence together with a possible context of utterance. Of course, since in this paper our goal will be to characterize paradoxicality for a formal language, this will not be too important, and in fact it will be harmless to use ‘statement’ and ‘sentence’ (or even ‘formula’) interchangeably. The only exception to this occurs in Sect. 3.5, where we consider sentences that fail to express a proposition.
The list is not meant to be exhaustive. We are only suggesting that in evaluating and comparing different accounts of paradoxicality one should bear these desiderata in mind. For one thing, there are other general desiderata that play a role in theory-choice in science and that we have not even mentioned, such as predictive power, unificatory power, fertility, etc. For another, we could expand the list—following Hanness Leitgeb (2007)—by importing some criteria that play a role in the case of theories of truth. For example, we think that it is reasonable to require a theory of paradoxicality to be couched in a language that is rich enough to code facts about its own syntax. We also think that the paradoxicality predicate ought to be untyped.
Cf. Kripke (1975, p. 696) for the original phrase and for his well-known diagnosis of the Nixon example.
Thanks are due to Luca Castaldo for discussion of this point.
Cf. Rosenblatt and Szmuc (2014) for a similar kind of model-theoretic construction.
It would be possible to emulate the paradoxicality predicate Par(x) using Tr(x) together with a paradoxicality operator, \(\mathcal {O^{P}}\). That is, Par(x) can be explicitly defined as \(\mathcal {O^{P}}Tr(x)\). So our choice of employing a predicate rather than an operator for paradoxicality is purely conventional.
We are focusing on this schema just for definiteness, but we are not committing ourselves to it. In fact, part of the appeal of the fixed-point conception is that it is compatible with other schemata as well. We will come back to this below.
Of course, other logical expressions, such as \(\vee \) and \(\exists \), can be defined in terms of these. Also, min stands for the minimum operation and an x-variant of a model \({\mathcal {M}}\) is a model that is exactly like \({\mathcal {M}}\) except perhaps in what it assigns to the variable x. To simplify things, we leave the assignment function (which assigns objects in |\(\mathbb {N}\)| to the variables of \({\mathcal {L}}_{+}\)) implicit in the presentation of the models.
Cf. Rosenblatt (2021) for a more detailed presentation.
Of course, the account will not be consistent if the underlying logic is paraconsistent. But paraconsistent logicians will suggest, first, that what is crucial is non-triviality rather than consistency, and, second, that consistency is just one among various other virtues that a theory may possess. Inconsistency can be viewed as a theoretical cost that can be trumped by other virtues. As Priest (2016, p. 351) puts it: “(...) it is only one criterion amongst many. How to weight it is, I am sure, itself the subject of some dispute. But whatever the weight, an inconsistent theory can be rationally preferable to a consistent one, if the performance of the inconsistent theory outweighs the consistent one on the other criteria.”
There are other general theoretical virtues (see Footnote 6) that we are not taking into account. Some of them may not apply to theories of paradoxicality. We do not think this is a problem. It is not our intention to argue in favor of any form of anti-exceptionalism, so we are happy to admit that there may be some theoretical virtues that play an important role in the assessment of other types of scientific theories but that are of no significance for theories of paradoxicality.
The verification of these claims is left to the reader. We employ the usual convention of writing for example \(Par\ulcorner \lnot \phi \urcorner \) instead of the more cumbersome \(Par\ulcorner neg(\phi )\urcorner \) (where neg represents the corresponding function operating on codes of statements). Also, we write \(\forall x Par\ulcorner \phi (x)\urcorner \) instead of \(\forall x Par\ulcorner \phi \urcorner ({\dot{x}}/\ulcorner x\urcorner )\) (where \({\dot{x}}\) represents the function that maps each number to its numeral and \(Par\ulcorner \phi \urcorner ({\dot{x}}/\ulcorner x\urcorner )\) is the claim that the result of substituting the numeral of x for the variable x in the statement \(\phi \) is paradoxical).
Disjunction, \(\vee \), can be defined using conjunction and negation in the usual way: \(\phi \vee \psi := \lnot (\lnot \phi \wedge \lnot \psi )\). We assume that the falsity constant, \(\bot \), is such that for every model \({\mathcal {M}}\), \(v_{{\mathcal {M}}}(\bot ) = 0\).
The paradox also requires a number of logical rules and also ‘recapture’ rules, that is, rules establishing that one can reason classically in certain contexts. But these are rules that paracomplete theorists typically accept. (With one exception: the argument requires the rule of disjunction-introduction, so it does not obviously carry over to a paracomplete theory based on the weak Kleene schema.)
The conditional, \(\rightarrow \), can be defined in the following way: \(\phi \rightarrow \psi := \lnot (\phi \wedge \lnot \psi )\). It would be interesting to see how this paradox plays out with an intensional conditional, of the sort that Field, Priest and others have advocated, but we leave a careful study of this possibility for another occasion.
There is a different way in which the definition can be generalized. One could consider models \({\mathcal {M}}\) for the base language \({\mathcal {L}_{PA}}\) other than the standard model of PA, and then quantify over every fixed-point extending each of the minimal fixed-points for Par(x) that can be reached from each of those ‘base’ models. This is useful if, for example, one wishes to give a diagnosis of contingent paradoxes. However, since we are limiting ourselves to the standard model of PA, we will not consider this possibility here. Needless to say, nothing important hangs on this (cf. Gallovich and Rosenblatt 2022 for the details).
One potential cost of relying on the notion of \({\mathcal {M}}_{FP}^{ext}\)-consequence is that one looses some of the facts alluded to earlier pertaining to the interaction of the paradoxicality predicate with the logical connectives, the quantifiers and the truth predicate. For example, it is easy to see that \(\rho \) yields a counterexample to \(Tr\ulcorner \phi \urcorner \models ^{*}\lnot Par\ulcorner \phi \urcorner \).
We are indebted to Luca Incurvati, Julien Murzi, Lorenzo Rossi and Giorgio Sbardolini for discussion on these ideas.
This is roughly the way in which Rosenblatt (2021) justifies the imposition of a restriction on Par-intro.
If \(\rho \) is not really a paradox, then one may ask if there are any new paradoxical statements involving the notion of paradoxicality. As one can infer from Sect. 3.2, the answer to this question is positive, although these new paradoxes are not very interesting. For example, the statement \(\lnot Par\ulcorner 0=0\urcorner \wedge \lambda \) is a paradox of this kind and it is diagnosed as such by our account.
At this point, it is important to stress that it is not our aim to establish that the approach we are offering is revenge-free in general. Considering the discussion given by Murzi and Rossi (2020), there is an important distinction one can draw between object-linguistic revenge paradoxes and meta-theoretic revenge paradoxes. They say that object-linguistic revenge paradoxes point to the inexpressibility in a theory of some notion that plays an explanatory or expressive role in that theory, while meta-theoretic revenge paradoxes involve notions that can be defined in the (classical) meta-theory. Given that the idea of ‘playing an explanatory or expressive role’ is one that does not admit of a formal characterization, we think that revenge-freedom is not something that can be formally or conclusively established. Whether a theory is revenge-free in the relevant sense will crucially depend on whether the notions that are inexpressible in the theory are notions that play an explanatory or expressive role in it. In the case of paradoxicality, it seems hard to deny that the notion plays an explanatory role in various non-classical theories. What we have shown is that one can consistently represent that notion in the object-language of a paracomplete theory as long as one is willing to slightly weaken one of the rules Par-intro and Par-elim. To be sure, that only yields revenge-freedom if (i) the resulting paradoxicality predicate is sufficient to play the explanatory role the non-classical theorist expects it to play, and (ii) there are no other inexpressible notions that play an explanatory or expressive role in the non-classical theorist’s overall picture.
One should be careful, though. Since \(\frac{1}{2}\) is now designated, the theory will be such that \(\models Par\ulcorner \phi \urcorner \) in some cases where \(\phi \) is not paradoxical in Kripke’s account. At any rate, it is still true that (i) \(\phi \) is paradoxical in Kripke’s account only if \(Par\ulcorner \phi \urcorner \) is strictly true and (ii) \(\phi \) is not paradoxical in Kripke’s account only if \(\models \lnot Par\ulcorner \phi \urcorner \).
As he puts it: “conventions for handling sentences that do not express propositions are not in any philosophically significant sense "changes in logic." The term ‘three-valued logic’, occasionally used here, should not mislead.” Cf. Kripke (1975, fn. 18).
There may be another sense in which the fixed-point account is compatible with classical logic. It is well known that one can obtain a classical theory of truth from Kripke models if one takes the ‘close-off’ of these models. That is, one takes whatever statements lie outside the extension of the truth predicate at some fixed-point and then one stipulates that Tr(x) is false of those statements. Kripke himself notes this possibility in the Outline. It would be interesting to explore if the same can be done with the construction we have offered for Par(x).
Gupta (1982) offers a number of other objections to the fixed-point approach. Since they are not specifically related to paradoxicality, we have decided to omit them. But, to be sure, a full defense of the fixed-point approach to truth and paradox would require an answer to these other points as well.
The existential quantifier, \(\exists \), is not part of the official language, but it can be defined in the following way: \(\exists x \phi (x):= \lnot \forall x \lnot \phi (x)\).
In speaking of ‘hypodoxes’ we are following Peter Eldrige-Smith’s terminology (Eldridge-Smith 2007).
Cook and Tourville’s idea is that an operator or a predicate is monotonic if and only if it is intensionally monotonic, i.e. monotone relative to the order of the different intensional semantic statuses that a statement can have (which is an order defined by them). Cf. Cook (2020) for the details.
The characterization we are about to offer is due to Cook and Tourville. Since Sophisticated Classification differs from Simple Classification in its treatment of unparadoxical statements, it can be used to offer a different specification of the falsity conditions for paradoxicality claims.
There is another difference between the definitions that is worth highlighting. Consider a statement \(\pi \) saying of itself that it is not paradoxical, \(\lnot Par\ulcorner \pi \urcorner \). It is easy to check that \(v_{{\mathcal {M}}_{FP}}(\pi ) = \frac{1}{2}\), but \(v_{\mathcal {M^{\star }}_{FP}}(\pi ) = 1\). Thus, the amended definition seems committed to the idea that there are statements that are true at a model purely in virtue of how they themselves semantically behave across the different fixed-points extending that model. This idea is incompatible with an intuition about truth that many would find plausible, namely, that the truth-value of a statement should ultimately depend on whether some non-semantic state of affairs obtains. For a discussion of the supervenience of semantics in fixed-point models, cf. Kremer (1988) and Gallovich (2022).
Of course, Cook and Tourville are aware of this limitation.
Unless, of course, one is willing to treat paradoxicality as a typed predicate. Indeed, it would be reasonable to expect a bivalent predicate if the goal were to extensionally capture Kripke’s notion. But we have already noted that our goal is different, and so is Cook and Tourville’s goal.
One promising possibility is to offer a characterization of ‘implicit occurrence’ along the lines of Lavinia Picollo’s account of alethic reference (Picollo 2015). However, pursuing this idea is beyond the scope of this paper.
References
Castaldo, L. (2021). Fixe-point Models for Paradoxical Predicates. Australasian Journal of Logic, 18(7), 688–723.
Cook, R. T. (2011). The No-No Paradox is a Paradox. Australasian Journal of Philosophy, 89(3), 467–482.
Cook, R. T. (2020). An intensional theory of truth: an informal report. The Philosophical Forum, 51(2), 115126.
Cook, R. T., & Tourville, N. (2016). Embracing the technicalities: expressive completeness and revenge. The Review of Symbolic Logic, 9(2), 325–358.
Cook, R. T., & Tourville, N. (2020). Embracing intensionality: paradoxicality and semi-truth operators in fixed point models. Logic Journal of the IGPL, 28(5), 747–770.
Eldridge-Smith, P. (2007). Paradoxes and hypodoxes of time travel. In P. W. Jan Lloyd Jones (Ed.), Art and time (pp. 172–189). Australia: Australian Scholarly Publishing.
Gallovich, C. (2022). Semantic supervenience in fixed-point models. Typescript.
Gallovich, C., & Rosenblatt, L. (2022). Paradoxes, hypodoxes, and more. Typescript.
Gilmore, P. C. (1974). The consistency of partial set theory without extensionality, axiomatic set theory. Proceedings of Symposia in Pure Mathematics: American Math Society, 13, 147–153.
Gupta, A. (1982). Truth and paradox. Journal of Philosophical Logic, 11(1), 1–60.
Gupta, A. (2005). Do the paradoxes pose a special problem for deflationism? In J. Beall & B. Armour-Garb (Eds.), Deflationism and paradox (pp. 133–147). Oxford: Oxford University Press.
Hsiung, M. (2021). In what sense is the no-no paradox a paradox? Philosophical studies, forth- coming.
Kremer, M. (1988). Kripke and the logic of truth. Journal of Philosophical Logic, 17(3), 225–278.
Kripke, S. (1975). Outline of a theory of truth. Journal of Philosophy, 72(19), 690–716.
Lawvere, W. (1969). Diagonal arguments and cartesian closed categories. Reprints in Theory and Applications of Categories, 15, 1–13.
Leitgeb, H. (2007). What theories of truth should be like (but cannot be). Philosophy Compass, 2(2), 276–290.
Martin, R., & Woodruff, P. (1975). On representing ‘True-in-L’ in L. Philosophia, 5(3), 213–217.
Murzi, J., & Rossi, L. (2020). Generalized revenge. Australasian Journal of Philosophy, 98(1), 153–177.
Murzi, J., & Rossi, L. (2022). Non-reflexivity and revenge. Journal of Philosophical Logic, 51, 201–218.
Picollo, L. (2015). Minimalism, reference and paradoxes. In P. Arazim & M. Dancak (Eds.), The logica yearbook 2015 (pp. 163–178). College Publications.
Prawitz, D. (1965). Natural deduction: a proof-theoretical study. New York: Dover.
Priest, G. (1994). The structure of the paradoxes of self-reference. Mind, 103(409), 25–34.
Priest, G. (2016). Logical disputes and the a priori. Logique et Analyse, 236, 347–366.
Rosenblatt, L. (2021). Paradoxicality without Paradox. Erkenntnis.
Rosenblatt, L., & Szmuc, D. (2014). On pathological truths. The Review of Symbolic Logic, 7(4), 601–617.
Rossi, L. (2019). A unified theory of truth and paradox. The Review of Symbolic Logic, 12(2), 209–254.
Tennant, N. (1982). Proof and paradox. Dialectica, 36, 265–296.
Walicky, M. (2017). Resolving infinitary paradoxes. The Journal of Symbolic Logic, 82(2), 709–723.
Acknowledgements
Talks based on earlier versions of this paper were given (online) at the Work in Progress Seminar of the Buenos Aires Logic Group (Buenos Aires, 2021), at the workshop Truth in Expressively Rich Languages (Bristol, 2021), at the EXPRESS-PHILMAT Seminar (Amsterdam-Paris, 2021), and at the workshop The Philosophy of Metamathematical Results (Munich Center for Mathematical Philosophy, 2022). We wish to thank the organizers and participants of all these events. We are especially grateful to Eduardo Barrio, Aylén Bavosa Castro, Lwenn Bussiére-Caraes, Catrin Campbell-Moore, Bruno Da Ré, Hartry Field, Camillo Fiore, Daniel Grimaldi, Volker Halbach, Luca Incurvati, Hannes Leitgeb, Julien Murzi, Federico Pailos, Francesca Poggiolesi, Graham Priest, Ricardo Rodriguez, Ariel Roffé, Lorenzo Rossi, Mariela Rubín, Giorgio Sbardolini, Johannes Stern, Damián Szmuc, Paula Teijeiro, Omar Vasquez and Albert Visser. Last but not least, special thanks go to Luca Castaldo and two anonymous reviewers of Synthese for comments on previous drafts that led to significant improvements. Financial support for this work was provided by the project “Logic and Substructurality” (FFI2017-84805-P), funded by the Spanish MINECO (Ministerio de Economía, Industria y Competitividad).
Author information
Authors and Affiliations
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
T.C. : Non-Classical Approaches to Paradox, Lead Guest Editor : Julien Murzi, Rossi Lorenzo, Topey Brett.
Rights and permissions
About this article
Cite this article
Rosenblatt, L., Gallovich, C. Paradoxicality in Kripke’s theory of truth. Synthese 200, 71 (2022). https://doi.org/10.1007/s11229-022-03625-x
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s11229-022-03625-x