Skip to main content
SpringerLink
Log in

Categories of Locally Hypercompact Spaces and Quasicontinuous Posets

  • Published:
Applied Categorical Structures Aims and scope Submit manuscript

Abstract

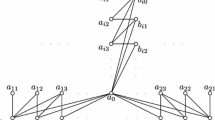
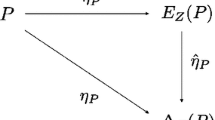
A subset of a topological space is hypercompact if its saturation (the intersection of its neighborhoods) is generated by a finite set. Locally hypercompact spaces are defined by the existence of hypercompact neighborhood bases at each point. We exhibit many useful properties of such spaces, often based on Rudin’s Lemma, which is equivalent to the Ultrafilter Principle and ensures that the Scott spaces of quasicontinuous domains are exactly the locally hypercompact sober spaces. We characterize their patch spaces (the Lawson spaces) as hyperconvex and hyperregular pospaces in which every monotone net has a supremum to which it converges. Moreover, we find extensions to the non-sober case by replacing suprema with cuts, and we provide topological generalizations of known facts for quasicontinuous posets. Similar results are obtained for hypercompactly based spaces and quasialgebraic posets. Furthermore, locally hypercompact spaces are described by certain relations between finite sets and points, providing a quasiuniform approach to such spaces. Our results lead to diverse old and new equivalences and dualities for categories of locally hypercompact spaces or quasicontinuous posets.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Banaschewski, B.: Über den Ultrafilterraum. Math. Nachr. 13, 273–281 (1955)

    Article  MathSciNet  Google Scholar 

  2. Banaschewski, B.: Essential extensions of T\(_0\)-spaces. Gen. Top. Appl. 7, 233–246 (1977)

    MATH  Google Scholar 

  3. Banaschewski, B.: The power of the ultrafilter theorem. J. Lond. Math. Soc. (2) 27, 193–202 (1983)

    Article  MathSciNet  Google Scholar 

  4. Banaschewski, B., Brümmer, G.C.L.: Stably continuous frames. Math. Proc. Camb. Philos. Soc. 104, 7–19 (1988)

    Article  MathSciNet  Google Scholar 

  5. Banaschewski, B., Erné, M.: On Krull’s separation lemma. Order 10, 253–260 (1993)

    Article  MathSciNet  Google Scholar 

  6. Erné, M.: Scott convergence and Scott topology in partially ordered sets II. In: Banaschewski, B., Hoffmann R.-E.(eds.) Continuous Lattices, Proc. Bremen, 1979, Lecture Notes in Math. vol. 871, pp. 61–96. Springer, Berlin–Heidelberg–New York (1981)

    Google Scholar 

  7. Erné, M.: Convergence and distributivity: a survey. In: Hoffmann, R.-E. (ed.) Continuous Lattices and Related Topics. Mathematik Arbeitspapiere Bremen vol. 27, pp. 39–50 (1982)

  8. Erné, M.: Compact generation in partially ordered sets. J. Aust. Math. Soc. (Ser. A) 42, 69–83 (1987)

    Article  MathSciNet  Google Scholar 

  9. Erné, M.: The ABC of order and topology. In: Herrlich, H., Porst, H.-E. (eds.) Category Theory at Work, pp. 57–83. Heldermann, Berlin (1991)

    Google Scholar 

  10. Erné, M.: Prime ideal theorems and systems of finite character. Comment. Math. Univ. Carol. 38, 513–536 (1997)

    MathSciNet  MATH  Google Scholar 

  11. Erné, M.: Minimal bases, ideal extensions, and basic dualities. Topol. Proc. 29, 445–489 (2005)

    MathSciNet  MATH  Google Scholar 

  12. Erné, M.: Choiceless, pointless, but not useless: dualities for preframes. Appl. Categ. Struct. 15, 541–572 (2007)

    Article  MathSciNet  Google Scholar 

  13. Erné, M.: Infinite distributive laws versus local connectedness and compactness properties. Topol. Appl. 156, 2054–2069 (2009)

    Article  MathSciNet  Google Scholar 

  14. Erné, M.: The strength of prime ideal separation, sobriety, and compactness theorems. Topol. Appl. 241, 263–290 (2018)

    Article  Google Scholar 

  15. Erné, M.: Web spaces and worldwide web spaces: topological aspects of domain theory. arXiv:1802.08170 [pdf]

  16. Erné, M.: Choice principles and lift lemmas. Categ. Gen. Algebraic Struct. Appl. 6, 121–146 (2017)

    MathSciNet  MATH  Google Scholar 

  17. Erné, M.: Patchwork and web spaces. Topol. Proc. 52, 1–29 (2018)

    MathSciNet  MATH  Google Scholar 

  18. Fletcher, P., Lindgren, W.F.: Quasi-uniform Spaces. Lecture Notes Pure Appl. Math. vol. 77, Marcel Dekker, New York (1982)

  19. Gierz, G., Hofmann, K.H., Keimel, K., Lawson, J.D., Mislove, M., Scott, D.S.: A Compendium of Continuous Lattices. Springer, Berlin-Heidelberg-New York (1980)

    Book  Google Scholar 

  20. Gierz, G., Hofmann, K.H., Keimel, K., Lawson, J.D., Mislove, M., Scott, D.S.: Continuous Lattices and Domains. Oxford University Press, Oxford (2003)

    Book  Google Scholar 

  21. Gierz, G., Lawson, J.D., Stralka, A.R.: Quasicontinuous posets. Houst. J. Math. 9, 191–208 (1983)

    MathSciNet  MATH  Google Scholar 

  22. Heckmann, R.: An upper power domain construction in terms of strongly compact sets, MFPS ’91. Lecture Notes in Comp. Science, vol. 598, 272–293. Springer, Berlin–Heidelberg–New York (1992)

    Google Scholar 

  23. Heckmann, R., Keimel, K.: Quasicontinuous domains and the Smyth powerdomain. Electron. Notes Theor. Comput. Sci. 298, 215–232 (2013)

    Article  MathSciNet  Google Scholar 

  24. Herrlich, H.: Axiom of Choice. Springer, Berlin - Heidelberg (2006)

    MATH  Google Scholar 

  25. Hofmann, K.H., Mislove, M.: Local compactness and continuous lattices. In: Banaschewski, B., Hoffmann R.-E. (eds.) Continuous Lattices, Lecture Notes in Math., vol. 871, Springer, Berlin–Heidelberg–New York (1981)

    Google Scholar 

  26. Howard, P., Rubin, J.E.: Consequences of the Axiom of Choice, vol. 59. AMS Mathematical Surveys and Monographs, Providence (1998)

    MATH  Google Scholar 

  27. Isbell, J.R.: Meet-continuous lattices. Symp. Math. 16, 41–54 (1975)

    MathSciNet  MATH  Google Scholar 

  28. Johnstone, P.T.: Stone Spaces. Cambridge University Press, Cambridge (1982)

    MATH  Google Scholar 

  29. Künzi, H.P.A., Brümmer, G.C.L.: Sobrification and bicompletion of totally bounded quasi-uniform spaces. Math. Proc. Camb. Philos. Soc. 101, 237–247 (1987)

    Article  MathSciNet  Google Scholar 

  30. Künzi, H.P.: Completely regular ordered spaces. Order 7, 283–293 (1990)

    Article  MathSciNet  Google Scholar 

  31. Lawson, J.D.: T\(_0\)-spaces and pointwise convergence. Topol. Appl. 21, 73–76 (1985)

    Article  Google Scholar 

  32. Lawson, J.D.: Order and strongly sober compactifications. In: Reed, G.M., Roscoe, A.W., Wachter, R.F. (eds.) Topology and Category Theory in Computer Science, pp. 179–205. Clarendon Press, Oxford (1991)

    Google Scholar 

  33. Mao, X., Xu, L.: Quasicontinuity of posets via sobrification. Order 23, 359–369 (2006)

    Article  MathSciNet  Google Scholar 

  34. Mycielski, J.: Two remarks on Tychonoff’s product theorem. Bull. Acad. Polon. Sci. Sér. Sci. Math. Astron. Phys. 8, 439–441 (1964)

    MathSciNet  MATH  Google Scholar 

  35. Nachbin, L.: Topology and Order. D. van Nostrand, Princeton (1965)

    MATH  Google Scholar 

  36. Priestley, H.A.: Intrinsic spectral topologies. In: Papers on General Topology and Applications. Papers from the 8th Summer Conference at Queens College, New York, NY, USA, 18–20 June 1992. The New York Academy of Sciences. Ann. N.Y. Acad. Sci., vol. 728, pp. 78–95 (1994)

  37. Rudin, M.: Directed sets which converge. In: McAuley, L.F., Rao, M.M. (eds.) General Topology and Modern Analysis, pp. 305–307. University of California, Riverside, 1980, Academic Press, (1981)

  38. Schwarz, F., Weck, S.: Scott topology, Isbell topology, and continuous convergence. In: Hoffmann, R.-E., Hofmann, K.H. (eds.) Continuous Lattices and their Applications, pp. 251–271. Marcel Dekker, New York (1985)

    Google Scholar 

  39. Scott, D.S.: Continuous lattices. In: Lawvere, F.W. (ed.) Toposes, Algebraic Geometry and Logic. Lecture Notes in Math, vol. 274, pp. 97–136. Springer, Berlin (1972)

    Google Scholar 

  40. Wyler, O.: Dedekind complete posets and Scott topologies. In: Banaschewski, B., Hoffmann, R.-E. (eds.) Continuous Lattices. Lecture Notes in Math, vol. 871, pp. 384–389. Springer, Berlin (1981)

    Google Scholar 

  41. Yokoyama, T.: A poset with spectral Scott topology is a quasialgebraic domain. Order 26, 331–335 (2009)

    Article  MathSciNet  Google Scholar 

  42. Zhang, W., Xu, X.: \(s_2\)-quasicontinuous posets. Theor. Comput. Sci. 574, 78–85 (2015)

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Faculty for Mathematics and Physics, Leibniz University Hannover, Hannover, Germany

    Marcel Erné

Authors
  1. Marcel Erné
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Marcel Erné.

Additional information

Communicated by Eva Colebunders.

Dedicated to my dear colleague and friend Bob Lowen.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Erné, M. Categories of Locally Hypercompact Spaces and Quasicontinuous Posets. Appl Categor Struct 26, 823–854 (2018). https://doi.org/10.1007/s10485-018-9536-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10485-018-9536-0

Keywords

Mathematics Subject Classification

Search

Navigation