Stochastic models of infectious diseases in a periodic environment with application to cholera epidemics

Abstract

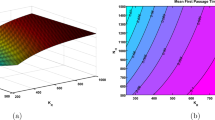
Seasonal variation affects the dynamics of many infectious diseases including influenza, cholera and malaria. The time when infectious individuals are first introduced into a population is crucial in predicting whether a major disease outbreak occurs. In this investigation, we apply a time-nonhomogeneous stochastic process for a cholera epidemic with seasonal periodicity and a multitype branching process approximation to obtain an analytical estimate for the probability of an outbreak. In particular, an analytic estimate of the probability of disease extinction is shown to satisfy a system of ordinary differential equations which follows from the backward Kolmogorov differential equation. An explicit expression for the mean (resp. variance) of the first extinction time given an extinction occurs is derived based on the analytic estimate for the extinction probability. Our results indicate that the probability of a disease outbreak, and mean and standard derivation of the first time to disease extinction are periodic in time and depend on the time when the infectious individuals or free-living pathogens are introduced. Numerical simulations are then carried out to validate the analytical predictions using two examples of the general cholera model. At the end, the developed theoretical results are extended to more general models of infectious diseases.

Appendices

Appendix

A ODE cholera model

The following assumptions about model (1) were made by Posny and Wang (2014b):

• (H1) $f(t,0,0)=h(t,0,0)=0$;

• (H2) $f(t, I, B)\ge 0$ for $(t, I, B)\in \mathbb {R}^3_{+}$;

• (H3) $\frac{\partial f}{\partial I}\ge 0$, $\frac{\partial f}{\partial B}\ge 0$, $\frac{\partial h}{\partial I}\ge 0$ and $\frac{\partial h}{\partial B}< 0$ for $(t, I, B)\in \mathbb {R}^3_{+}$;

• (H4) f and h are both concave functions in terms of I and B, for all $t\ge 0$; i.e.,

  $$\begin{aligned} D^2f = \begin{pmatrix} \frac{\partial ^2 f}{\partial I^2} &{} \frac{\partial ^2 f}{\partial I\partial B}\\ \frac{\partial ^2 f}{\partial B\partial I} &{} \frac{\partial ^2 f}{\partial B^2} \end{pmatrix}, \quad D^2h = \begin{pmatrix} \frac{\partial ^2 h}{\partial I^2} &{} \frac{\partial ^2 h}{\partial I\partial B}\\ \frac{\partial ^2 h}{\partial B\partial I} &{} \frac{\partial ^2 h}{\partial B^2} \end{pmatrix} \end{aligned}$$

  are negative semidefinite for $(t, I, B)\in \mathbb {R}^3_{+}$.

• (H5) $f(t, 0, B)>0$ if $B>0$; $h(t, I, 0)>0$ if $I>0$.

• (H6) For simplicity, write $f=f(t, x_1, x_2)$ and $h=h(t, x_1,x_2)$. There exists $\epsilon >0$, such that for $0<x_1,x_2<\epsilon$, we have

  $$\begin{aligned} u(t, x_1,x_2)\ge \left. \left[ u+\sum _{i=1}^2\frac{\partial u}{\partial x_i}+\frac{1}{2}\sum _{i, j=1}^2\frac{\partial ^2 u}{\partial x_i\partial x_j}\right] \right| _{(t,x_1,x_2)=(t,0,0),}\quad {\text {for }} u=f, h. \end{aligned}$$

The global stability of the DFE when ${\mathcal {R}}_0<1$ and uniform persistence of the solution of model (1) when ${\mathcal {R}}_0>1$ were verified in (Posny and Wang 2014b, Theorems 3, 5) and they are summarized as follows:

Theorem 1

• (i) If ${\mathcal {R}}_0<1$, the DFE of model (1) is globally asymptotically stable, and for any solution x(t) of model (1),

  $$\begin{aligned} \lim _{t\rightarrow \infty }x(t)=(N,0,0,0)^T. \end{aligned}$$

• (ii) Suppose that assumptions (H1)-(H6) hold. If $R_0>1$, then the solutions of system (1) are uniformly persistent and the system admits at least one positive $\omega$-periodic solution.

B general epidemic model

For the more general linearized ODE model in (23),

the conditions on F and V in Bacaër and Ait Dads (2014) for all $t\ge 0$ are

• (H1) F(t) is nonnegative with at least one entry strictly positive;

• (H2) $F(t) - V(t)$ is irreducible;

• (H3) F(t) and V(t) are piecewise continuous and $\omega$-periodic in t;

• (H4) $-V(t)$ is cooperative (i.e., off-diagonal coefficients are nonnegative) and there exists $c>0$ such that $V_{ii}(t)\ge c$ for $t\ge 0$.

C multiple infections

The branching process approximation of the probability of an outbreak in (13) shows good agreement with the time-nonhomogeneous process of the stochastic cholera Models 1 and 2 when initial conditions are either $(I(\tau ),B(\tau ))=(2,2)$ or (0, 6) Fig. 9.

Fig. 9

Probabilities of an outbreak, ${\mathbb {P}}_{outbreak}(2,2,\tau )$ and ${\mathbb {P}}_{outbreak}(0,6,\tau )$, Eq. (13), are graphed for the stochastic cholera Models 1 (solid black curve) and 2 (dashed red curve). The proportion of $2\times 10^4$ sample paths that result in an outbreak ($I+0.25 B\ge 200)$ for the time-nonhomogeneous process at $\tau =0,365/12, 365/6,\ldots ,365$ are marked by blue circles. Parameter values are the same as in Figs. 3, 6