Analysis of a two-strain malaria transmission model with spatial heterogeneity and vector-bias

Abstract

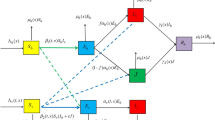
In this paper, we introduce a reaction–diffusion malaria model which incorporates vector-bias, spatial heterogeneity, sensitive and resistant strains. The main question that we study is the threshold dynamics of the model, in particular, whether the existence of spatial structure would allow two strains to coexist. In order to achieve this goal, we define the basic reproduction number $R_{i}$ and introduce the invasion reproduction number ${\hat{R}}_{i}$ for strain $i (i=1,2)$. A quantitative analysis shows that if $R_{i}<1$, then disease-free steady state is globally asymptotically stable, while competitive exclusion, where strain i persists and strain j dies out, is a possible outcome when $R_{i}>1>R_{j}$ $(i\ne j, i,j=1,2)$, and a unique solution with two strains coexist to the model is globally asymptotically stable if $R_{i}>1$, ${\hat{R}}_{i}>1$. Numerical simulations reinforce these analytical results and demonstrate epidemiological interaction between two strains, discuss the influence of resistant strains and study the effects of vector-bias on the transmission of malaria.

Appendix

1.1 The details of assumption $N(t,x)\equiv N(x)$

Here, based on the research of Bai et al. (2018), we will take $b_{h}$ as a typical birth rate function, i.e., $b_{h}=b_{h}(N_{h})=b_{0}e^{-N_{h}/K(x)}$, where $b_{0}>0$ is the maximal individual birth rate of human, and K(x), standing for the local carrying capacity, is supposed to be a positive function of location x.

We prove this result in several steps.

Step 1. It is easy to see that for any $\phi \in C({\bar{\varOmega }},{\mathbb {R}}_{+})$, system (1)–(2) has a unique positive solution $N(t,x,{\phi })$ on $[0,\infty )\times {\bar{\varOmega }}$ (see, Martin and Smith 1990, Corollary 5), with $N(0,x,\phi )=\phi$. Define $P_{t}:C({\bar{\varOmega }},{\mathbb {R}}_{+})\rightarrow C({\bar{\varOmega }},{\mathbb {R}}_{+})$ by

$$\begin{aligned} \begin{aligned} P_{t}[\phi ](x)=N(t,x;\phi ). \end{aligned} \end{aligned}$$

It then follows that $\{P_{t}\}_{t\ge 0}$ is a semifolw on $C({\bar{\varOmega }},{\mathbb {R}}_{+})$.

For any $\phi \in C({\bar{\varOmega }},{\mathbb {R}}_{+})$ with $\phi \gg 0$ one easily finds that $N(t,x;\phi )>0$ for $t\ge 0$ and $x\in \varOmega$. Fix $k\in (0,1)$ and let $w(t,x)=N(t,x;k\phi )-kN(t,x;\phi )$. Then $w(0,x)=0$ for $x\in \varOmega$. For $t>0$, w(t, x) satisfies

$$\begin{aligned} \begin{aligned} \frac{\partial w(t,x)}{\partial t}&=\frac{\partial N(t,x;k\phi )}{\partial t}-k\frac{\partial N(t,x;\phi )}{\partial t}\\&=D_{h}\varDelta w(t,x)-dw(t,x)+b_{h}(N(t,x;k\phi ))N(t,x;k\phi )\\&\quad -kb_{h}(N(t,x;\phi ))N(t,x;\phi ). \end{aligned} \end{aligned}$$

(39)

Let $f(t,N(t,x;\phi ))=b_{h}(N(t,x;\phi ))N(t,x;\phi )$, then (39) can be written as

$$\begin{aligned} \begin{aligned} \frac{\partial w(t,x)}{\partial t}&=D_{h}\varDelta w(t,x)-dw(t,x)+f(t,N(t,x;k\phi ))-f(t,k(N(t,x;\phi )))\\&\quad +f(t,k(N(t,x;\phi )))-kf(t,N(t,x;\phi ))\\&=D_{h}\varDelta w(t,x)-(d-H(t,x))w(t,x)+h(t,x), \end{aligned} \end{aligned}$$

where

$$\begin{aligned} \begin{aligned} H(t,x)&=\int _{0}^{1}\partial _{2}f(t,kN(t,x;\phi )+(1-s)kN(t,x;\phi ))ds,~~~0\le s\le 1,\\ h(t,x)&=f(t,k(N(t,x;\phi )))-kf(t,N(t,x;\phi )). \end{aligned} \end{aligned}$$

Let U(t, s), $t\ge s\ge 0$, be the evolution operator of the linear parabolic equation

$$\begin{aligned} \begin{aligned} \frac{\partial w(t,x)}{\partial t}=D_{h}\varDelta w(t,x)-(d_{h}-H(t,x))w(t,x), ~~~t>0,~~x\in \varOmega . \end{aligned} \end{aligned}$$

Following Smith (1995, Theorem 7.4.1), one easily sees that U(t, s), $t> s\ge 0$, is strongly positive, i.e., for any $\varphi >0$, $U(t,s)\varphi \gg 0$. By the formula of variation of constants, we have

$$\begin{aligned} \begin{aligned} w(t,x)=\int _{0}^{t}U(t,s)h(s,\cdot )(x)ds, ~~~t>0,~~x\in \varOmega . \end{aligned} \end{aligned}$$

Since $N(t,\cdot ;\phi )>0$, $t\ge 0$, when $\phi \gg 0$ and f(t, N) is strictly subhomogeneous in N, we have $h(s,\cdot )>0$ and hence $w(t,\cdot )>0$ for $t>0$. Therefore, $P_{t}(k\phi )>kP_{t}(\phi )$ for each $t>0$, so $P_{t}$ is strictly subhomogeneous.

Step 2. For each $t\ge 0$, the Frechet derivative $DP_{t}(t)=\varPhi (t)$, where $\varPhi (t)$ is the semigroup generated by the linear equation $\frac{\partial N_{h}(t,x)}{\partial t}=d\varDelta N_{h}(t,x)+g(x,0)N_{h}(t,x)$ with $\frac{\partial N_{h}(t,x)}{\partial \nu }=0$, where $g(x,N_{h}(t,x))=b_{h}(N_{h}(t,x))-d_{h}$. It then follows that $r(D(P_{t}(0)))=e^{\lambda _{0}(d,g(x,0))t}$, where $\lambda _{0}(d,g(x,0))$ is the principle eigenvalue of $d\varDelta \phi +g(x,0)\phi =\lambda \phi$ with $\frac{\partial \phi }{\partial \nu }=0$.

Step 3. In the case, where $r(D(P_{t}(0)))>1$, i.e., $\lambda _{0}(d,g(0,x))>0$, apply the Zhao (2017, Theorem 2.3.4) to the time-one map $P_{1}$. There exists a unique positive fixed point of $P_{1}$, which is assumed to be N(x).

Step 4. Since system (1)–(2) is autonomous, then $P_{1}(P_{t}(N(x)))=P_{t+1}(N(x))=P_{t}(P_{1}(N(x)))=P_{t}(N(x))$. The uniqueness of positive fixed point of $P_{1}$ implies that $P_{t}(N(x))=N(x)$, $\forall t\ge 0$, that is, N(x) is a steady state of (1).

Therefore, it is reasonable to assume that $N(t,x)\equiv N(x)$.

1.2 The derivation of P(A|B)

$$\begin{aligned} \begin{aligned} P(A|B)&=\frac{P(B|A)P(A)}{P(B)}=\frac{P(B|A)P(A)}{P(B|A)P(A)+P(B|A^{c})P(A^{c})}\\&=\frac{p\frac{I_{1}(t,x)+I_{2}(t,x)}{N(x)}}{p\frac{I_{1}(t,x)+I_{2}(t,x)}{N(x)}+l\left[ 1-\frac{I_{1}(t,x)+I_{2}(t,x)}{N(x)}\right] }\\&=\frac{p\left[ I_{1}(t,x)+I_{2}(t,x)\right] }{p\left[ I_{1}(t,x)+I_{2}(t,x)\right] +l\left[ N(x)-I_{1}(t,x)-I_{2}(t,x)\right] }. \end{aligned} \end{aligned}$$