Skip to main content
SpringerLink
Log in

SCHUR–WEYL DUALITY FOR HEISENBERG COSETS

  • Published:
Transformation Groups Aims and scope Submit manuscript

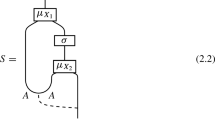
Abstract

Let V be a simple vertex operator algebra containing a rank n Heisenberg vertex algebra H and let C = Com(H;V) be the coset of H in V. Assuming that the module categories of interest are vertex tensor categories in the sense of Huang, Lepowsky and Zhang, a Schur-Weyl type duality for both simple and indecomposable but reducible modules is proven. Families of vertex algebra extensions of C are found and every simple C-module is shown to be contained in at least one V-module. A corollary of this is that if V is rational, C2-cofinite and CFT-type, and Com(C;V) is a rational lattice vertex operator algebra, then C is likewise rational. These results are illustrated with many examples and the C1-cofiniteness of certain interesting classes of modules is established.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. T. Abe, A ℤ2-orbifold model of the symplectic fermionic vertex operator superal-gebra, Math. Z. 255 (2007), no. 4, 755-792.

    Article  MathSciNet  Google Scholar 

  2. T. Abe, C. Dong, H. Li, Fusion rules for the vertex operator algebra M(1) and \( {V}_L^{+}, \) Comm. Math. Phys. 253 (2005), no. 1, 171-219.

  3. D. Adamović, Representations of the N = 2 superconformal vertex algebra, Int. Math. Res. Not. 1999, no. 2, 61-79.

    Article  MATH  MathSciNet  Google Scholar 

  4. D. Adamović, Vertex algebra approach to fusion rules for N = 2 superconformal minimal models, J. Algebra 239 (2001), no. (2), 549-572.

    Article  MATH  MathSciNet  Google Scholar 

  5. D. Adamović, A construction of admissible \( {A}_1^{(1)} \)-modules of level \( -\frac{4}{3}, \) J. Pure Appl. Algebra 196 (2005), no. 2-3, 119-134.

  6. D. Adamović, A. Milas, Vertex operator algebras associated to modular invariant representations for \( {A}_1^{(1)} \), Math. Res. Lett. 2 (1995), no. 5, 563-575.

  7. D. Adamović, A. Milas, On the triplet vertex algebra W(p), Adv. Math. 217 (2008), no. 6, 2664-2699.

    Article  MATH  MathSciNet  Google Scholar 

  8. D. Adamović, A. Milas, Lattice construction of logarithmic modules for certain vertex algebras, Selecta Math. (N.S.) 15 (2009), no. 4, 535-561.

    Article  MATH  MathSciNet  Google Scholar 

  9. D. Adamović, A. Milas, Some applications and constructions of intertwining opera-tors in LCFT, Contemp. Math. 695 (2017), 15-27.

    Article  MATH  Google Scholar 

  10. D. Adamović, O. Perše, Fusion rules and complete reducibility of certain modules for affine Lie algebras, J. Algebra Appl. 13 (2014), no. 1, 1350062.

    Article  MATH  MathSciNet  Google Scholar 

  11. C. Alfes, T. Creutzig, The mock modular data of a family of superalgebras, Proc. Amer. Math. Soc. 142 (2014), no. 7, 2265-2280.

    Article  MATH  MathSciNet  Google Scholar 

  12. T. Arakawa, Rationality of Bershadsky-Polyakov vertex algebras, Comm. Math. Phys. 323 (2013), no. 2, 627-633.

    Article  MATH  MathSciNet  Google Scholar 

  13. T. Arakawa, Rationality of W-algebras: principal nilpotent cases, Ann. Math. (2) 182 (2015), no. 2, 565-604.

    Article  MATH  MathSciNet  Google Scholar 

  14. T. Arakawa, Rationality of admissible affine vertex algebras in the category O, Duke Math. J. 165 (2016), no. 1, 67-93.

    Article  MATH  MathSciNet  Google Scholar 

  15. T. Arakawa, T. Creutzig, K. Kawasetsu, A. R. Linshaw, Orbifolds and cosets of minimal W-algebras, Comm. Math. Phys. 355 (2017), no. 1, 339-372.

    Article  MATH  MathSciNet  Google Scholar 

  16. T. Arakawa, T. Creutzig, A. R. Linshaw, Cosets of Bershadsky–Polyakov algebras and rational W-algebras of type A, Selecta Math. (N.S.) 23 (2017), no. 4, 2369-2395.

    Article  MATH  MathSciNet  Google Scholar 

  17. T. Arakawa, T. Creutzig, A. R. Linshaw, W-algebras as coset vertex algebras, https://arxiv.org/abs/1801.03822.

  18. T. Arakawa, V. Futorny, L. E. Ramirez, Weight representations of admissible affine vertex algebras, Comm. Math. Phys. 353 (2017), no. 3, 1151-1178.

    Article  MATH  MathSciNet  Google Scholar 

  19. T. Arakawa, C.-H. Lam, H. Yamada, Zhu's algebra, C 2 -algebra and C 2 -cofiniteness of parafermion vertex operator algebras, Adv. Math. 264 (2014), 261-295.

    Article  MATH  MathSciNet  Google Scholar 

  20. T. Arakawa, A. Molev, Explicit generators in rectangular affine W-algebras of type A, Lett. Math. Phys. 107 (2017), no. 1, 47-59.

    Article  MATH  MathSciNet  Google Scholar 

  21. J. Auger, T. Creutzig, D. Ridout, Modularity of logarithmic parafermion vertex al-gebras, D. Lett Math Phys (2018), https://doi.org/10.1007/s11005-018-1098-4.

  22. A. Babichenko, T. Creutzig, Harmonic analysis and free field realization of the Ta-kiff supergroup of GL(1|1), SIGMA Symmetry Integrability Geom. Methods Appl. 11 (2015), Paper 067.

  23. A. Babichenko, D. Ridout, Takiff superalgebras and conformal field theory, J. Phys. A 46 (2013), no. 12, 125204.

    Article  MATH  MathSciNet  Google Scholar 

  24. D. J. Benson, Representations and Cohomology. I. Basic Representation Theory of Finite Groups and Associative Algebras, Cambridge Studies in Advanced Mathematics, Vol. 30, Cambridge University Press, Cambridge, 1991.

    Google Scholar 

  25. M. Bershadsky, Conformal field theories via Hamiltonian reduction, Comm. Math. Phys. 139 (1991), no. 1, 71-82.

    Article  MATH  MathSciNet  Google Scholar 

  26. S. Carnahan, M. Miyamoto, Regularity of fixed-point vertex operator subalgebras, arXiv:1603.05645v4 (2018).

  27. T. Creutzig, T. Gannon, Logarithmic conformal field theory, log-modular tensor categories and modular forms, J. Phys. A 50 (2017), no. 40, 404004.

    Article  MATH  MathSciNet  Google Scholar 

  28. T. Creutzig, T. Gannon, The theory of C 2 -cofinite VOAs, in preparation.

  29. T. Creutzig, Y.-Z. Huang, J. Yang, Braided tensor categories of admissible modules for affine Lie algebras, Commun. Math. Phys. 362 (2018), no. 3, 827-854.

    Article  MATH  MathSciNet  Google Scholar 

  30. T. Creutzig, S. Kanade, A. R. Linshaw, Simple current extensions beyond semi-simplicity, arXiv:1511.08754 (2015).

  31. T. Creutzig, S. Kanade, R. McRae, Tensor categories for vertex operator superal-gebra extensions, arXiv:1705.05017 (2017).

  32. T. Creutzig, A. R. Linshaw, Cosets of affine vertex algebras inside larger structures, to appear in J. Algebra, arXiv:1407.8512v5 (2018).

  33. T. Creutzig, A. R. Linshaw, The super W 1+∞ algebra with integral central charge, Trans. Amer. Math. Soc. 367 (2015), no. 8, 5521-5551.

    Article  MATH  MathSciNet  Google Scholar 

  34. T. Creutzig, A. R. Linshaw, Orbifolds of symplectic fermion algebras, Trans. Amer. Math. Soc. 369 (2017), no. 1, 467-494.

    Article  MATH  MathSciNet  Google Scholar 

  35. T. Creutzig, A. R. Linshaw, Cosets of the W k(sl 4; f subreg)-algebra, Contemp. Math. 711 (2018), 105-117.

    Article  MATH  Google Scholar 

  36. T. Creutzig, A. Milas, False theta functions and the Verlinde formula, Adv. Math. 262 (2014), 520-545.

    Article  MATH  MathSciNet  Google Scholar 

  37. T. Creutzig, A. Milas, Higher rank partial and false theta functions and represen-tation theory, Adv. Math. 314 (2017), 203-227.

    Article  MATH  MathSciNet  Google Scholar 

  38. T. Creutzig, A. Milas, M. Rupert, Logarithmic link invariants of \( {\overline{U}}_q^H\left(s{l}_2\right) \) and asymptotic dimensions of singlet vertex algebras, J. Pure Appl. Algebra 222 (2016), no. 10, 3224-3247.

  39. T. Creutzig, D. Ridout, Modular data and Verlinde formulae for fractional level WZW models I, Nucl. Phys. B 865 (2012), no. 1, 83-114.

    Article  MATH  MathSciNet  Google Scholar 

  40. T. Creutzig, D. Ridout, Logarithmic conformal field theory: Beyond an introduction, J. Phys. A 46 (2013), no. 49, 494006.

    Article  MATH  MathSciNet  Google Scholar 

  41. T. Creutzig, D. Ridout, Modular data and Verlinde formulae for fractional level WZW models II, Nucl. Phys. B 875 (2013), no. 2, 423-458.

    Article  MATH  MathSciNet  Google Scholar 

  42. T. Creutzig, D. Ridout, Relating the archetypes of logarithmic conformal field theory, Nucl. Phys. B 872 (2013), no. 3, 348-391.

    Article  MATH  MathSciNet  Google Scholar 

  43. T. Creutzig, D. Ridout, W-algebras extending \( \widehat{g}l\left(1|1\right), \) in: Lie Theory and its Applications in Physics, Springer Proc. Math. Stat., Vol. 36, Springer, Tokyo, 2013, pp. 349-367.

  44. T. Creutzig, D. Ridout, S. Wood, Coset constructions of logarithmic (1, p) models, Lett. Math. Phys. 104 (2014), no. 5, 553-583.

    Article  MATH  MathSciNet  Google Scholar 

  45. T. Creutzig, P. B. Rønne, The GL(1|1)-symplectic fermion correspondence, Nucl. Phys. B 815 (2009), no. 1-2, 95-124.

    Article  MATH  MathSciNet  Google Scholar 

  46. P. Di Vecchia, J. L. Petersen, M. Yu, H. B. Zheng, Explicit construction of unitary representations of the N = 2 superconformal algebra, Phys. Lett. B 174 (1986), no. 3, 280-284.

    Article  MathSciNet  Google Scholar 

  47. C. Dong, C.-H. Lam, Q. Wang, H. Yamada, The structure of parafermion vertex operator algebras, J. Algebra 323 (2010), no. 2, 371-381.

    Article  MATH  MathSciNet  Google Scholar 

  48. C. Dong, C.-H. Lam, H. Yamada, W-algebras related to parafermion algebras, J. Algebra 322 (2009), no. 7, 2366-2403.

    Article  MATH  MathSciNet  Google Scholar 

  49. C. Dong, J. Lepowsky, Generalized Vertex Algebras and Relative Vertex Operators, Progress in Mathematics, Vol. 112, Birkhäuser, Boston, 1993.

  50. C. Dong, H. Li, G. Mason, Compact automorphism groups of vertex operator algebras, Int. Math. Res. Not. 1996, no. 18, 913-921.

  51. C. Dong, H. Li, G. Mason, Simple currents and extensions of vertex operator algebras, Comm. Math. Phys. 180 (1996), no. 3, 671-707.

    Article  MATH  MathSciNet  Google Scholar 

  52. C. Dong, H. Li, G. Mason, Vertex operator algebras and associative algebras, J. Algebra 206 (1998), no. 1, 67-96.

    Article  MATH  MathSciNet  Google Scholar 

  53. C. Dong, L. Ren, Representations of the parafermion vertex operator algebras, Adv. Math. 315 (2017), 88-101.

    Article  MATH  MathSciNet  Google Scholar 

  54. C. Dong, Q. Wang, On C 2 -cofiniteness of parafermion vertex operator algebras, J. Algebra 328 (2011), 420-431.

    Article  MATH  MathSciNet  Google Scholar 

  55. C. Dong, Q. Wang, Quantum dimensions and fusion rules for parafermion vertex operator algebras, Proc. Amer. Math. Soc. 144 (2016), no. 4, 1483-1492.

    Article  MATH  MathSciNet  Google Scholar 

  56. P. Etingof, S. Gelaki, D. Nikshych, V. Ostrik, Tensor Categories, Mathematical Surveys and Monographs, Vol. 205, American Mathematical Society, Providence, 2015.

    Book  MATH  Google Scholar 

  57. B. L. Feigin, A. M. Semikhatov, \( {W}_n^{(2)} \) algebras, Nucl. Phys. B 698 (2004), no. 3, 409-449.

  58. I. B. Frenkel, Y.-Z. Huang, J. Lepowsky, On Axiomatic Approaches to Vertex Operator Algebras and Modules, Mem. Amer. Math. Soc., Vol. 494, 1993.

  59. M. R. Gaberdiel, Fusion rules and logarithmic representations of a WZW model at fractional level, Nucl. Phys. B 618 (2001), no. 3, 407-436.

    Article  MATH  MathSciNet  Google Scholar 

  60. N. Geer, J. Kujawa, B. Patureau-Mirand, Ambidextrous objects and trace functions for nonsemisimple categories, Proc. Amer. Math. Soc. 141 (2013), no. 9, 2963-2978.

    Article  MATH  MathSciNet  Google Scholar 

  61. N. Genra, Screening operators for W-algebras, Selecta Math. (N.S.) 23 (2017), no. 3, 2157-2202.

    Article  MATH  MathSciNet  Google Scholar 

  62. D. Gepner, New conformal field theories associated with Lie algebras and their partition functions, Nucl. Phys. B 290 (1987), no. 1, 10-24.

    Article  MathSciNet  Google Scholar 

  63. R. Howe, Remarks on classical invariant theory, Trans. Amer. Math. Soc. 313 (1989), no. 2, 539-570.

    Article  MATH  MathSciNet  Google Scholar 

  64. Y.-Z. Huang, Rigidity and modularity of vertex tensor categories, Commun. Contemp. Math. 10 (2008), suppl. 1, 871-911.

  65. Y.-Z. Huang, Cofiniteness conditions, projective covers and the logarithmic tensor product theory, J. Pure Appl. Algebra 213 (2009), no. 4, 458-475.

    Article  MATH  MathSciNet  Google Scholar 

  66. Y.-Z. Huang, A. Kirillov Jr., J. Lepowsky, Braided tensor categories and extensions of vertex operator algebras, Comm. Math. Phys. 337 (2015), no. 3, 1143-1159.

    Article  MATH  MathSciNet  Google Scholar 

  67. Y.-Z. Huang, J. Lepowsky, L. Zhang, Logarithmic tensor category theory for generalized modules for a conformal vertex algebra I{VIII, arXiv:1012.4193v7 (2013), arXiv:1012.4196v2 (2012), arXiv:1012.4197v2 (2012), arXiv:1012.4198v2 (2012), arXiv:1012.4199v3 (2012), arXiv:1012.4202v3 (2012), arXiv:1110.1929v2 (2012), arXiv:1110.1931v2 (2012).

  68. V. Kac, A. Radul, Representation theory of the vertex algebra W1+∞, Transform. Groups 1 (1996), no. 1-2, 41-70.

    Article  MATH  MathSciNet  Google Scholar 

  69. V. G. Kac, M.Wakimoto, Modular invariant representations of infinite-dimensional Lie algebras and superalgebras, Proc. Nat. Acad. Sci. U.S.A. 85 (1988), no. 14, 4956-4960.

    Article  MATH  MathSciNet  Google Scholar 

  70. V. G. Kac, M. Wakimoto, Integrable highest weight modules over affine super-algebras and Appell's function, Comm. Math. Phys. 215 (2001), no. 3, 631-682.

    Article  MATH  MathSciNet  Google Scholar 

  71. V. G. Kac, M. Wakimoto, Quantum reduction and representation theory of super-conformal algebras, Adv. Math. 185 (2004), no. 2, 400-458.

    Article  MATH  MathSciNet  Google Scholar 

  72. H. G. Kausch, Extended conformal algebras generated by a multiplet of primary fields, Phys. Lett. B 259 (1991), no. 4, 448-455.

    Article  MathSciNet  Google Scholar 

  73. M. Krauel, M. Miyamoto, A modular invariance property of multivariable trace functions for regular vertex operator algebras, J. Algebra 444 (2015), 124-142.

    Article  MATH  MathSciNet  Google Scholar 

  74. C.-H. Lam, Induced modules for orbifold vertex operator algebras, J. Math. Soc. Japan 53 (2001), no. 3, 541-557.

    Article  MATH  MathSciNet  Google Scholar 

  75. J. Lepowsky, M. Primc, Structure of the Standard Modules for the Affine Lie Algebra \( {A}_1^{(1)}, \) Contemp. Math. American Mathematical Society, Vol. 46, Providence, 1985.

  76. J. Lepowsky, R. L. Wilson, A new family of algebras underlying the Rogers-Rama-nujan identities and generalizations, Proc. Nat. Acad. Sci. USA 78 (1981), no. 12, part 1, 7254-7258.

  77. J. Lepowsky, R. L. Wilson, A Lie theoretic interpretation and proof of the Rogers-Ramanujan identities, Adv. Math. 45 (1982), no. 1, 21-72.

    Article  MATH  MathSciNet  Google Scholar 

  78. J. Lepowsky, R. L. Wilson, The structure of standard modules. I. Universal algebras and the Rogers-Ramanujan identities, Invent. Math. 77 (1984), no. 2, 199-290.

    Article  MATH  MathSciNet  Google Scholar 

  79. J. Lepowsky, R. L. Wilson, The structure of standard modules. II. The case \( {A}_1^{(1)}, \) principal gradation, Invent. Math. 79 (1985), no. 3, 417-442.

  80. H. Li, Symmetric invariant bilinear forms on vertex operator algebras, J. Pure Appl. Algebra 96 (1994), no. 3, 279-297.

    Article  MATH  MathSciNet  Google Scholar 

  81. H. Li, On abelian coset generalized vertex algebras, Commun. Contemp. Math. 3 (2001), no. 2, 287-340.

    Article  MATH  MathSciNet  Google Scholar 

  82. H. Li, J. Lepowsky, Introduction to Vertex Operator Algebras and Their Representations, Progress in Mathematics, Vol. 227, Birkhäuser, Boston, 2004.

    MATH  Google Scholar 

  83. H. Li, X. Xu, A characterization of vertex algebras associated to even lattices, J. Algebra 173 (1995), no. 2, 253-270.

    Article  MATH  MathSciNet  Google Scholar 

  84. X. Lin, Mirror extensions of rational vertex operator algebras, Trans. Amer. Math. Soc. 369 (2017), no. 6, 3821-3840.

    Article  MATH  MathSciNet  Google Scholar 

  85. A. R. Linshaw, Invariant chiral differential operators and the W 3 algebra, J. Pure Appl. Algebra 213 (2009), no. 5, 632-648.

    Article  MATH  MathSciNet  Google Scholar 

  86. A. R. Linshaw, A Hilbert theorem for vertex algebras, Transform. Groups 15 (2010), no. 2, 427-448.

    Article  MATH  MathSciNet  Google Scholar 

  87. A. R. Linshaw, Invariant theory and the W 1+∞ algebra with negative integral central charge, J. Eur. Math. Soc. 13 (2011), no. 6, 1737-1768.

    MATH  MathSciNet  Google Scholar 

  88. A. R. Linshaw, Invariant theory and the Heisenberg vertex algebra, Int. Math. Res. Not. 2012, no. 17, 4014-4050.

  89. A. R. Linshaw, Invariant subalgebras of affine vertex algebras, Adv. Math. 234 (2013), 61-84.

    Article  MATH  MathSciNet  Google Scholar 

  90. A. R. Linshaw, The structure of the Kac-Wang-Yan algebra, Comm. Math. Phys. 345 (2016), no. 2, 545-585.

    Article  MATH  MathSciNet  Google Scholar 

  91. A. Milas, Logarithmic intertwining operators and vertex operators, Comm. Math. Phys. 277 (2008), no. 2, 497-529.

    Article  MATH  MathSciNet  Google Scholar 

  92. M. Miyamoto, Modular invariance of vertex operator algebras satisfying C 2 -cofiniteness, Duke Math. J. 122 (2004), no. 1, 51-91.

    Article  MATH  MathSciNet  Google Scholar 

  93. M. Miyamoto, Flatness and semi-rigidity of vertex operator algebras, arXiv:1104. 4675 (2011).

  94. M. Miyamoto, C 1 -cofiniteness and fusion products for vertex operator algebras, in: Conformal Field Theories and Tensor Categories, Proceedings of a workshop held at Beijing International Center for Mathematical Research, Beijing, China, June 13-17, 2011, Springer, Heidelberg, 2014, pp. 271-279.

  95. M. Miyamoto, C 2 -cofiniteness of cyclic-orbifold models, Comm. Math. Phys. 335 (2015), no. 3, 1279-1286.

    Article  MATH  MathSciNet  Google Scholar 

  96. A. M. Polyakov, Gauge transformations and diffeomorphisms, Internat. J. Modern Phys. A 5 (1990), no. 5, 833-842.

    Article  MATH  MathSciNet  Google Scholar 

  97. D. Ridout, \( \widehat{s}l{(2)}_{-1/2}: \) a case study, Nucl. Phys. B 814 (2009), no. 3, 485521.

  98. D. Ridout, \( \widehat{s}l{(2)}_{-1/2} \) and the triplet model, Nucl. Phys. B 835 (2010), no. 3, 314-342.

  99. D. Ridout, Fusion in fractional level \( \widehat{s}l(2) \) -theories with \( k=-\frac{1}{2}, \) Nucl. Phys. B 848 (2011), no. 1, 216-250.

  100. D. Ridout, S. Wood, Modular transformations and Verlinde formulae for logarith-mic (p + ; p – )-models, Nucl. Phys. B 880 (2014), 175-202.

    Article  MATH  Google Scholar 

  101. D. Ridout, S. Wood, Relaxed singular vectors, Jack symmetric functions and fractional level \( \widehat{s}l(2) \) models, Nucl. Phys. B 894 (2015), 621-664.

  102. D. Ridout, S. Wood, The Verlinde formula in logarithmic CFT, Journal of Physics: Conference Series 597 (2015), no. 1, 012065.

    Google Scholar 

  103. I. Runkel, A braided monoidal category for free super-bosons, J. Math. Phys. 55 (2014), no. 4, 041702.

    Article  MATH  MathSciNet  Google Scholar 

  104. R. Sato, Equivalences between weight modules via N = 2 coset constructions, arXiv: 1605.02343(2016).

  105. A. N. Schellekens, S. Yankielowicz, Extended chiral algebras and modular invariant partition functions, Nucl. Phys. B 327 (1989), no. 3, 673-703.

    Article  MathSciNet  Google Scholar 

  106. A. Tsuchiya, S. Wood, The tensor structure on the representation category of the W p triplet algebra, J. Phys. A 46 (2013), no. 44, 445203.

    Article  MATH  MathSciNet  Google Scholar 

  107. A. Tsuchiya, S. Wood, On the extended W-algebra of type sl 2 at positive rational level, Int. Math. Res. Not. 2015, no. 14, 5357-5435.

  108. W. Wang, W 1+∞ algebra, W 3 algebra, and Friedan–Martinec–Shenker bosonization, Comm. Math. Phys. 195 (1998), no. 1, 95-111.

    Article  MATH  MathSciNet  Google Scholar 

  109. H. Weyl, The Classical Groups, Princeton Landmarks in Mathematics, Princeton University Press, Princeton, 1997.

    Google Scholar 

  110. А. Б. Замолодчиков, В. А. фатеев, Нелокальные (парафермионные) токи в двумерной конформной квантовой теории поля и самодуальные критические точки в Z N -симметричных статистических системах, ЖЭТФ 380–399. Engl. transl.: A. B. Zamolodchikov, V. A. Fateev, Nonlocal (parafermion) currents in two-dimensional conformal quantum field theory and self-dual critical points in Z N -symmetric statistical systems, Soviet Phys. JETP 62 (1985), no. 2, 215–225.

Download references

Author information

Author notes

  1. S. KANADE

    Present address: Department of Mathematics, University of Denver, Denver, 80208, USA

Authors and Affiliations

  1. Department of Mathematical and Statistical Sciences, University of Alberta, Edmonton, T6G2G1, Canada

    T. CREUTZIG & S. KANADE

  2. Department of Mathematics, University of Denver, Denver, 80208, USA

    A. R. LINSHAW

  3. School of Mathematics and Statistics, The University of Melbourne, Parkville, 3010, Australia

    D. RIDOUT

Authors
  1. T. CREUTZIG
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. S. KANADE
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. A. R. LINSHAW
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. D. RIDOUT
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to S. KANADE.

Additional information

Supported by the NSERC discovery grant #RES0020460.

Supported by PIMS postdoctoral fellowship.

Supported by the Simons Foundation Grant #318755.

Supported by the Australian Research Council Discovery Projects DP1093910 and DP160101520.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

CREUTZIG, T., KANADE, S., LINSHAW, A.R. et al. SCHUR–WEYL DUALITY FOR HEISENBERG COSETS. Transformation Groups 24, 301–354 (2019). https://doi.org/10.1007/s00031-018-9497-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00031-018-9497-2

Search

Navigation