An Investigation into the Rheology of Pharmaceutical Inter-granular Material Bridges at High Shear Rates

Abstract

Purpose

This study was undertaken to investigate the rheological properties of inter-granular material bridges on the nano-scale when strained at high shear rates.

Materials and Methods

Atomic force microscopy (AFM) was used as a rheometer to measure the viscoelasticity of inter-granular material bridges for lactose:PVP K29/32 and lactose:PVP K90 granules, produced by wet granulation.

Results

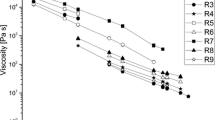
The loss tangent (tan δ) and both the storage (G′) and loss shear moduli (G″) of inter-granular material bridges were measured as a function of the probe–sample separation distance, oscillation frequency and relative humidity (RH). As the probe was withdrawn from the granule surface tan δ initially increased rapidly from zero to a plateau phase. G″ became increasingly dominant as the bridge was further extended and eventually exceeded G′. At high RH, capillary forces were foremost at bridge rupture, whereas at low RH elastic forces dominated. The effect of increasing frequency was to increase the effective elasticity of the bridge at high RH.

Conclusions

AFM has been employed as a rheometer to investigate the nano-scale rheology of inter-granular material bridges. This novel method may be used to obtain a fundamental understanding how different binders, granulated with different diluent fillers, behave at high shear rates.

APPENDIX

Application of the Voigt model in deriving tan δ, G′ and G″ values of granular material bridges from dynamic AFM measurements

When the cantilever, driven at a frequency well below its resonant frequency, is not in contact with the substrate it is static, not oscillating and its deflection signal is at the “free level”. When the colloid probe of mass m is at the original position it is static and experiences no force (Fig. 1: Static state):

$$z = z_0 $$

(5)

$$d = z_0 + d_0 $$

(6)

where z and d are the piezo drive and probe detected spatial positions respectively, z ₀ is the original position of the piezo and d ₀ is the cantilever length when static (Fig. 1). It follows that c (cantilever compression or extension) may be defined as:

$$c = z - d$$

(7)

In the static state c ₀ may therefore be derived as:

$$c_0 = z_0 - \left( {z_0 + d_0 } \right) = - d_0 $$

(8)

It is noteworthy that Eq. 8 results from defining cantilever compression as positive and extension as negative (Fig. 1). Cantilever extension may also be defined as positive (with compression being negative); this leads to c ₀ = d ₀, without affecting the derivation of rheological parameters.

When the probe is brought into contact with the substrate and the cantilever deflects, the cantilever is in the pseudo-static state. The piezo position is equal to its initial position plus its change in position:

$$z = z_0 + \Delta z_0 $$

(9)

Similarly,

$$d = z_0 + d_0 + \Delta d_0 $$

(10)

and

$$\Delta c = c - c_0 = \Delta z_0 - \Delta d_0 $$

(11)

Assuming harmonic oscillation of the piezo, when the probe is in contact with the substrate, and a resultant forced harmonic oscillation of mass m at the same harmonic frequency, the piezo oscillating with an amplitude z ₁ drives the cantilever at amplitude d ₁. In the dynamic state, when the cantilever oscillates, z may therefore be expressed as:

$$z = z_0 + \Delta z_0 + z_1 \times e^{j\omega t} $$

(12)

Since the cantilever oscillation lags behind that of the piezo by phase angle θ, owing to the loss component of the sample, d may be written as:

$$d = z_0 + d_0 + \Delta d_0 + d_1 \times e^{j\left( {\omega t + \theta } \right)} $$

(13)

and

$$\Delta c = \Delta z_0 - \Delta d_0 + z_1 \times e^{j\omega t} - d_1 \times e^{j\left( {\omega t + \theta } \right)} $$

(14)

In addition,

$$\Delta c = \Delta c_0 + c_1 \times e^{j\left( {\omega t + \varphi } \right)} $$

(15)

with Δc ₀ the DC component of the cantilever deflection, and $c_1 \times e^{j\left( {\omega t + \varphi } \right)}$ the AC component. The phase lag of the deflection signal is denoted by φ. Using Eqs. 7–13 the DC and AC components of the cantilever deflection signal may be separated and cantilever deflection may then be expressed as:

$$\Delta c_0 = \Delta z_0 - \Delta d_0 $$

(16)

and

$$c_1 \times e^{j\left( {\omega t + \varphi } \right)} = z_1 \times e^{j\omega t} - d_1 \times e^{j\left( {\omega t + \theta } \right)} $$

(17)

Furthermore, the change in position (i.e., the amplitude signal) of the piezo (Δz) and mass m (Δd) is equal to the difference between their position in the dynamic and static states:

$$\Delta z = z_{{\text{dynamic}}} - z_{{\text{static}}} $$

(18)

and

$$\Delta d = d_{{\text{dynamic}}} - d_{{\text{static}}} $$

(19)

Therefore,

$$\Delta z = \Delta z_0 + z_1 \times e^{j\omega t} $$

(20)

$$\Delta d = \Delta z_0 + \Delta d_0 + d_1 \times e^{j\left( {\omega t + \theta } \right)} $$

(21)

Differentiation of Eqs. 12, 13 and 14 yields:

$$\dot z = j\omega z_1 \times e^{j\omega t} $$

(22)

$$\ddot z = - \omega ^2 z_1 \times e^{j\omega t} $$

(23)

$$\dot d = j\omega d_1 \times e^{j\left( {\omega t + \theta } \right)} $$

(24)

$$\ddot d = - \omega ^2 d_1 \times e^{j\left( {\omega t + \theta } \right)} $$

(25)

$$\dot c = j\omega c_1 \times e^{j\left( {\omega t + \varphi } \right)} $$

(26)

$$\ddot c = - \omega ^2 c_1 \times e^{j\left( {\omega t + \varphi } \right)} $$

(27)

Applying Newton’s third law of motion, the forces acting on the probe mass m (i.e., the product of its mass and acceleration (Newton’s second law) added to the product of the cantilever spring constant and deflection (Hooke’s law) is equal but opposite to the force arising from the sample spring and dashpot:

$$m\ddot d + k\Delta c = D\dot d + K\Delta d$$

(28)

where D is the dashpot dampening coefficient and K denotes the spring restoring coefficient.

By substitution:

$$- m\omega ^2 d_1 \times e^{j\left( {\omega t + \theta } \right)} + k\left[ {\Delta c_0 + c_1 \times e^{j\left( {\omega t + \varphi } \right)} } \right] = j\omega Dd_1 \times e^{j\left( {\omega t + \theta } \right)} + K\left[ {\Delta d_0 + d_1 \times e^{j\left( {\omega t + \theta } \right)} } \right]$$

(29)

In AFM, c ₁ may be measured directly from deflection signal and z ₁ calibrated using a non-deformable elastic surface, but d ₁ cannot be measured; substituting for d ₁, using Eq. 14:

$$\begin{aligned}& - m\omega ^2 \left[ {z_1 \times e^{j\omega t} - c_1 \times e^{j\left( {\omega t + \varphi } \right)} } \right] + k\Delta c_0 + kc_1 \times e^{j\left( {\omega t + \varphi } \right)} = \\& j\omega D\left[ {z_1 \times e^{j\omega t} - c_1 \times e^{j\left( {\omega t + \varphi } \right)} } \right] + K\Delta d_0 + K\left[ {z_1 \times e^{j\omega t} - c_1 \times e^{j\left( {\omega t + \varphi } \right)} } \right] \\ \end{aligned} $$

(30)

In the pseudo-static state, Newton’s 3rd Law of motion applies:

$$k\Delta c_0 = K\Delta d_0 $$

(31)

If γ is the ratio of c ₁ to z ₁, and Eq. 29 is divided by z ₁ and e ^jωt:

$$- m\omega ^2 \left( {1 - \gamma \times e^{j\varphi } } \right) + k\gamma e^{j\varphi } = j\omega D\left( {1 - \gamma \times e^{j\varphi } } \right) + K\left( {1 - \gamma \times e^{j\varphi } } \right)$$

(32)

Dividing by $\left( {1 - \gamma \times e^{j\varphi } } \right)$:

$$- m\omega ^2 + k \times \frac{{\gamma e^{j\varphi } }}{{1 - \gamma e^{j\varphi } }} = j\omega D + K$$

(33)

$$- m\omega ^2 + k \times \frac{{\gamma e^{j\varphi } \left( {1 - \gamma e^{ - j\varphi } } \right)}}{{1 - 2\gamma \cos \varphi + \gamma ^2 }} = j\omega D + K$$

(34)

$$- m\omega ^2 + k \times \frac{{\gamma e^{j\varphi } - \gamma ^2 }}{{1 - 2\gamma \cos \varphi + \gamma ^2 }} = j\omega D + K$$

(35)

On rearranging, with $e^{j\varphi } = \cos \varphi + j\sin \varphi$:

$$- m\omega ^2 + k \times \frac{{\gamma \cos \varphi - \gamma ^2 + j\gamma \sin \varphi }}{{1 - 2\gamma \cos \varphi + \gamma ^2 }} = j\omega D + K$$

(36)

Splitting Eq. 36 into real and imaginary components:

$$K = - m\omega ^2 + k \times \frac{{\gamma \cos \varphi - \gamma ^2 }}{{1 - 2\gamma \cos \varphi + \gamma ^2 }}$$

(37)

and

$$\omega D = k \times \frac{{\gamma \sin \varphi }}{{1 - 2\gamma \cos \varphi + \gamma ^2 }}$$

(38)

The mass of the borosilicate microsphere was small: the average mass for the ballotini, with an average density of 2,500 kg m⁻³ and average radius of 10 μm, was 1.05 × 10⁻¹¹ g. Therefore mω ² ≪ k at drive frequencies <1,000 Hz (i.e., at 1,000 Hz: 5.56 × 10⁻³ N m⁻¹ ≪ 40 N m⁻¹) and it may be assumed that:

$$K = k \times \frac{{\gamma \cos \varphi - \gamma ^2 }}{{1 - 2\gamma \cos \varphi + \gamma ^2 }}$$

(39)

For a sphere–plane geometry Montfort and Hadziioannou (14) derived that:

$$G\prime = \frac{K}{b}$$

(40)

$$G\prime \prime = \frac{{\omega D}}{b}$$

(41)

where b, a correction factor used to convert deflection measurements into loss (G′) and storage (G″) moduli, is related to the radius of the colloid probe (R) and the distance of separation between the colloid probe and granule surface (s):

$$b = \frac{{6\pi R^2 }}{s}$$

(42)

Therefore, by substitution

$$G\prime = \frac{k}{b}\frac{{\gamma \cos \varphi - \gamma ^2 }}{{1 - 2\gamma \cos \varphi + \gamma ^2 }}$$

(43)

$$G\prime \prime = \frac{k}{b}\frac{{\gamma \sin \varphi }}{{1 - 2\gamma \cos \varphi + \gamma ^2 }}$$

(44)

The rheological parameter tan δ may be defined as:

$$\tan \delta = \frac{{G\prime \prime }}{{G\prime }} = \frac{{\sin \varphi }}{{\cos \varphi - \gamma }}$$

(45)

Comparison of Voigt and Choi models

To allow a comparison between the Voigt and Choi models the experimental APDD data and Voigt derived rheological data shown in Fig. 8 were processed also using the Choi model (Fig. 12). In the case of the Voigt model (Figs. 8 and 12), as the colloid probe moved further away from the granule surface both G profiles decreased. At the crossover point of the G′ and G″ profiles, where tan δ equalled 1: distance of separation ∼13 nm), the deflection signal illustrates that the probe was still in contact with the granule surface, the signal-to-noise ratio of the phase signal was still high and the amplitude signal was high; therefore the model is valid at this point. In contrast, the Choi model (Fig. 12) which incorporates the elastic meniscus force computed an elevated G′. Whilst G″ decreased with increasing separation, G′ remained high and was dominant until bridge rupture. As a result the bridges were predominantly elastic with tan δ being less than 1 throughout. In comparing the models, the peak of the G profiles occurred at the same distance of separation as maximum adhesion (i.e., minimum deflection). Thereafter, traces for G′ differ between Voigt and Choi models, however this does not effect the conclusions drawn in this work.

Fig. 12.

Conversion of APDD curves to rheological measurement using the Voigt and Choi models. The Choi model accounts for the capillary force of the inter-granular bridge (Eq. 4). G′, G″ and tan δ profiles of an inter-granular material bridge formed at 80% RH, between a spherical cantilever probe oscillating at 1,000 Hz and the surface of a lactose:PVP K29/32 granule, are plotted as a function of probe–sample separation distance and based upon the unload part of the force curve.